Journal of Jilin University(Engineering and Technology Edition) ›› 2024, Vol. 54 ›› Issue (4): 1136-1143.doi: 10.13229/j.cnki.jdxbgxb.20221526

Previous Articles    

Improved algorithm based on self-corrected min-sum decoding for LDPC codes

Hong JIANG(),Hong-liang XU   

  1. College of Information Engineering，Southwest University of Science and Technology，Mianyang 621000，China
  • Received:2022-11-29 Online:2024-04-01 Published:2024-05-17

RICH HTML

 0   

PDF (PC)

220

Abstract:

Low density parity check code is a kind of linear block code. Due to the low content of non-zero elements， there is a problem that the node information reliability determination is not accurate enough in the decoding process. Therefore， an improved LDPC decoding algorithm based on self correcting minimum sum is proposed. The iteration rule of BP decoding algorithm is analyzed. The first minimum and the second minimum of the minimum sum algorithm are used to set the correction threshold of variable node information， and the self-corrected min-sum algorithm is improved. The order statistics theory is used to obtain the normalization factor corresponding to the two minimum values to prevent the transmission and diffusion of unreliable variable node information in the iterative decoding process. Simulation results show that when the bit error rate is 10-5， the proposed algorithm can obtain about 0.2 dB of decoding performance gain， and the average number of iterations can be reduced by 18.2% at most， which proves that the proposed algorithm can effectively improve the decoding performance and iterative convergence performance.

Key words: LDPC code, self-corrected min-sum algorithm, modified threshold, order statistic, belief propagation algorithm

CLC Number: 

  • TN911.22

Fig.1

SCMS algorithm variable node information erase threshold"

Fig.2

M-SCMS algorithm variable node information erase threshold"

Fig.3

Percentage change in symbols for the algorithm"

Fig.4

Bit error rate of different algorithm with code length 2400"

Fig.5

Bit error rate of different algorithm with code length 3000"

Table 1

Computational complexity of different algorithm in a single iteration"

算 法MultiplicationDivisionAddiction
BP11NW-6（N+K）N（W+1）N（3W+1）
MS00N（4W-1）+K（lbW-2）
OMS00N（4W-1）+K（lbW-2）
SCMS00N（4W-1）+K（lbW-2）
DE-NMSNW0N（4W-1）+K（lbW-2）
LMMSE-MSNW0N（4W-1）+K（lbW-2）
M-SCMS2NW0N（5W-1）+K（lbW-2）

Fig.6

Average number of iterations of different decoding algorithm"

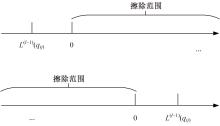
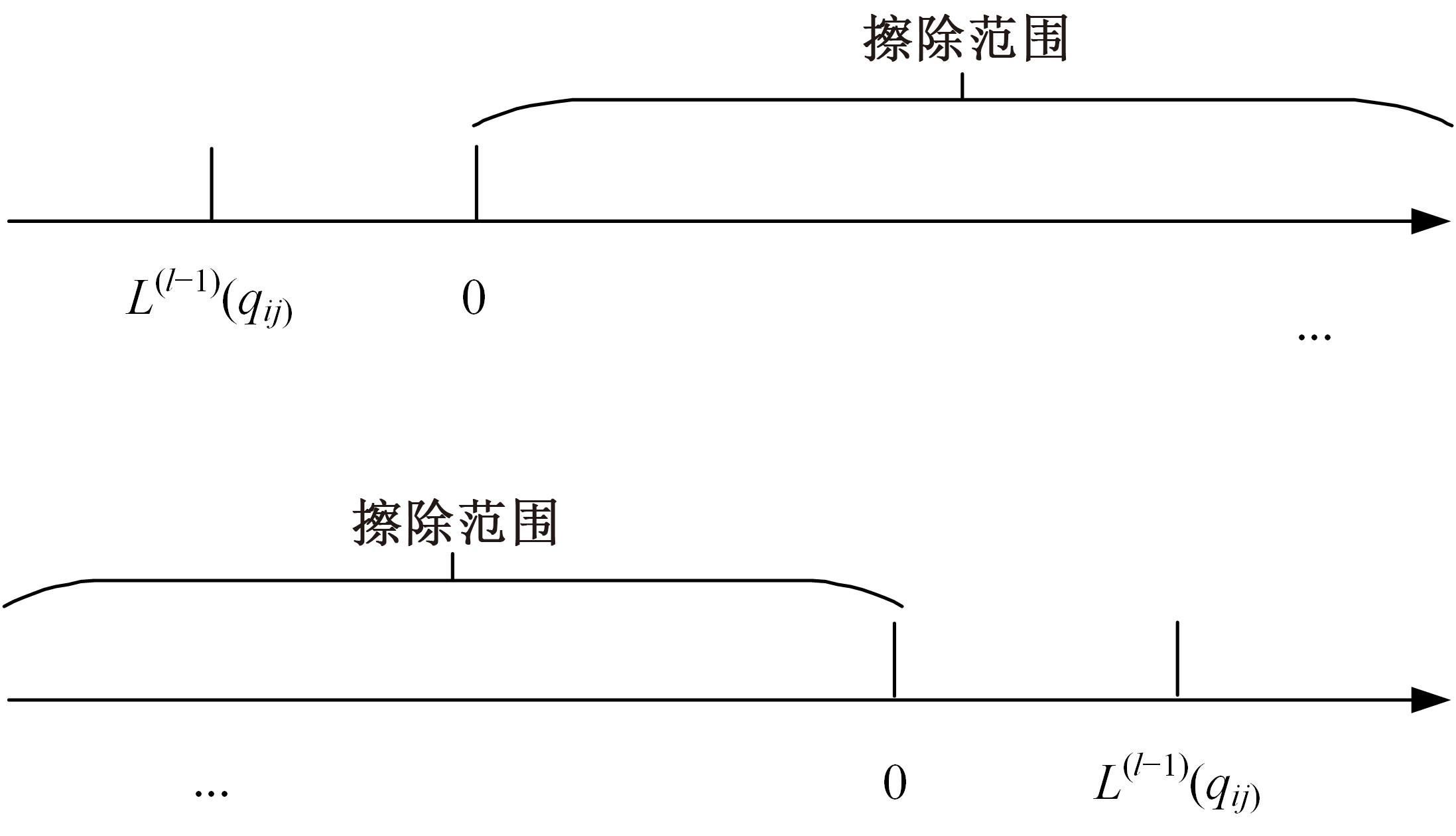
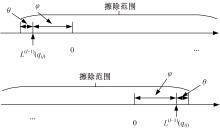
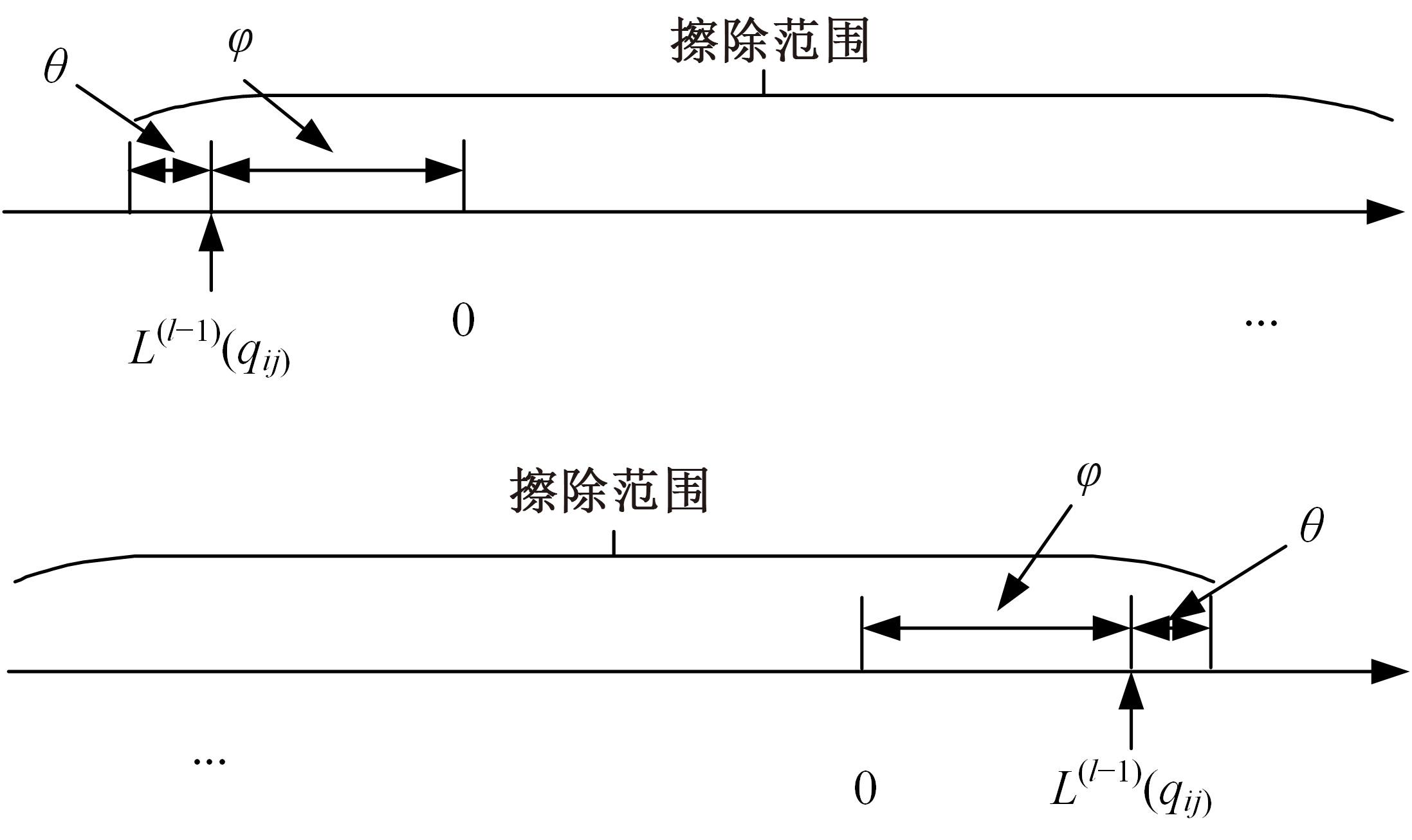
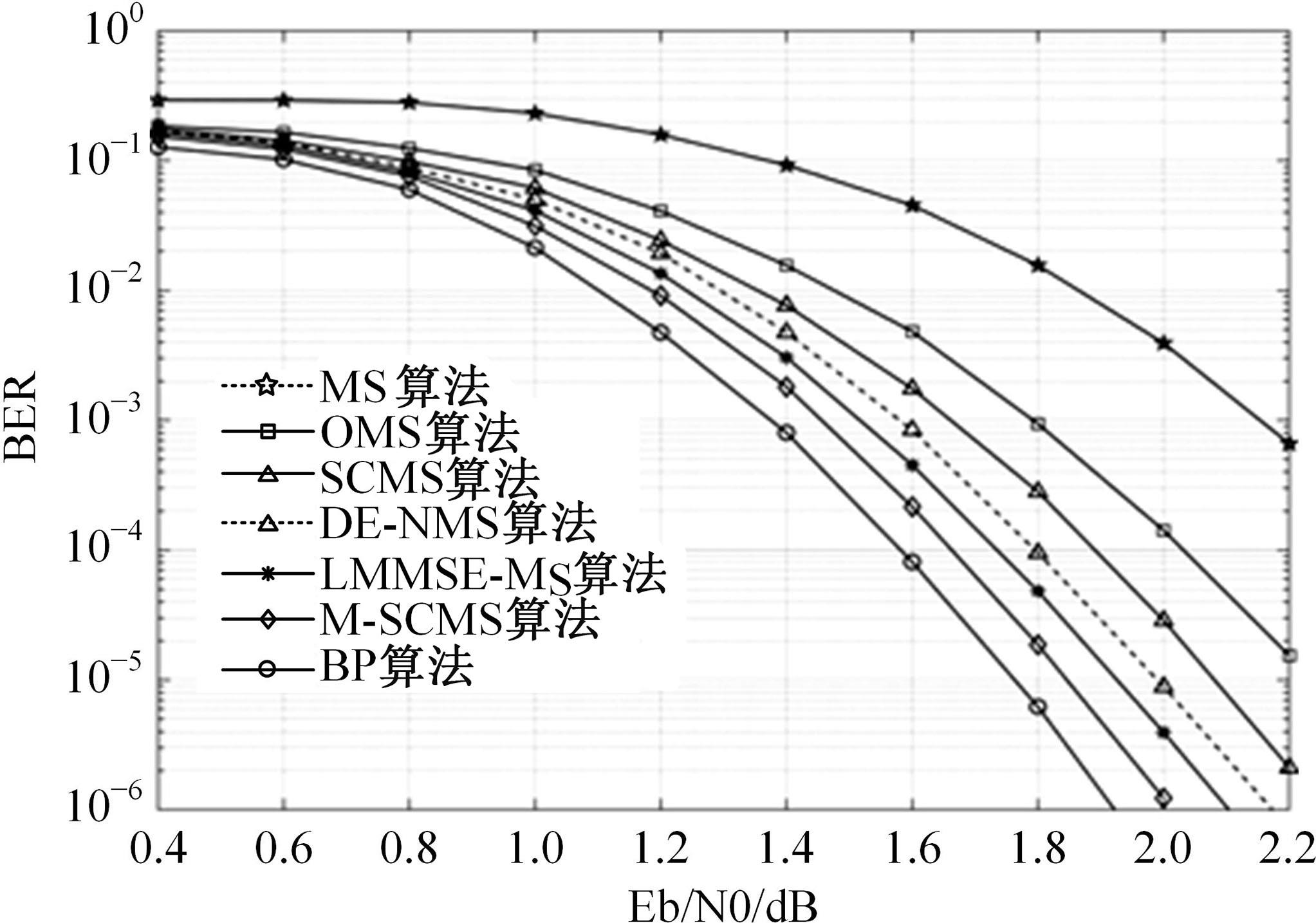
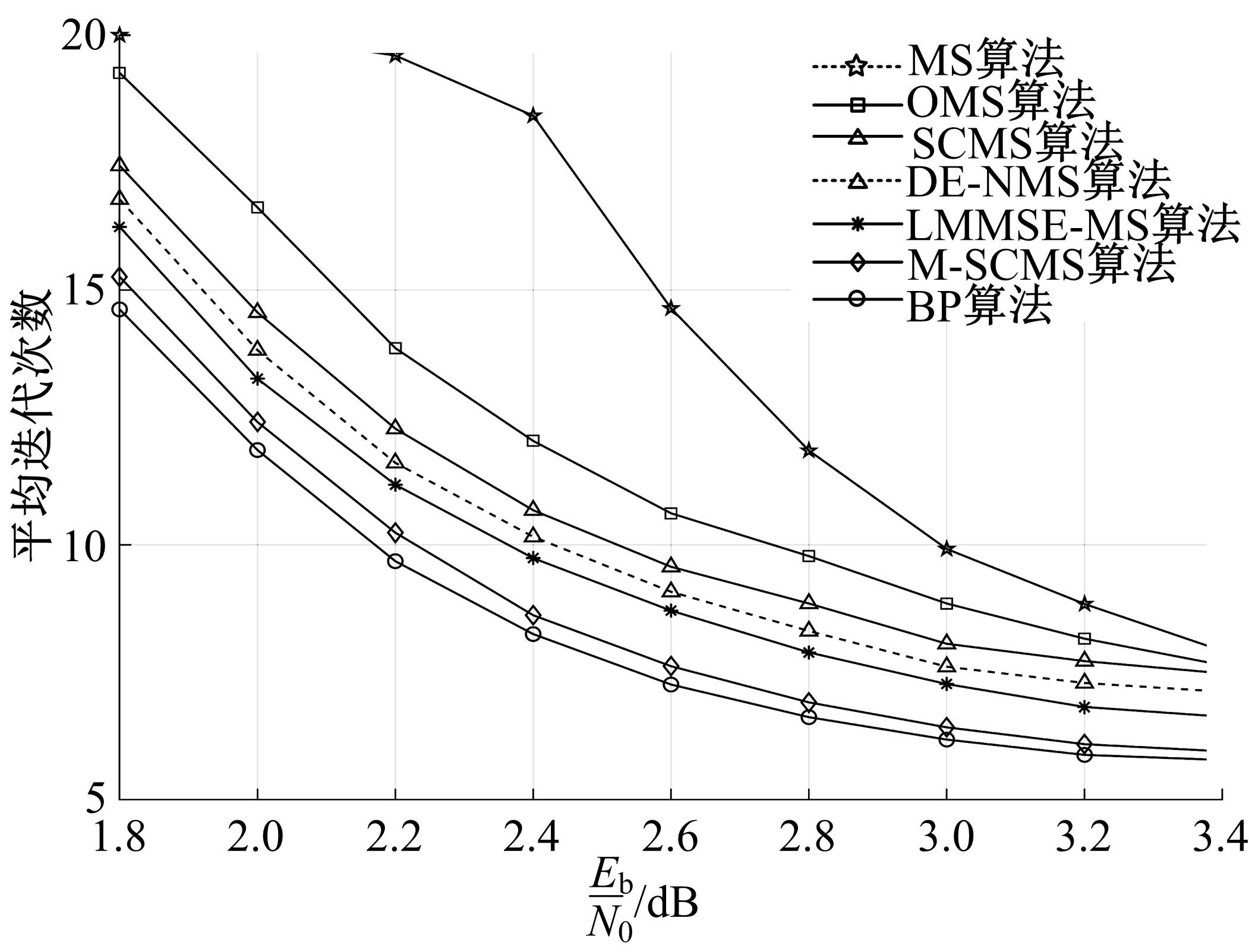
1 Indoonundon M, Pawan F T. Overview of the challenges and solutions for 5G channel coding schemes[J]. Journal of Information and Telecommunication, 2021, 5(4): 460-483.
2 HU D W. On the implementation of 5G LDPC decoder[J]. Journal of Electronics & Information Technology,2021, 43(4): 1112-1119.
3 Wu X, Tian J Q, Yang X Y. Fast encoding algorithm for 5G new radio LDPC code[J]. Communications Technology, 2022, 55(1): 30-35.
4 Myung S, Park S I, Kim K J, et al. Offset and normalized min-sum algorithms for ATSC 3.0 LDPC decoder[J]. IEEE Trans on Broadcasting, 2017, 63(4): 734-739.
5 TRAN-THI B N, NGUYEN-LY T, Hong H N, et al. An improved offset min-sum LDPC decoding algorithm for 5G new radio[C]∥International Symposium on Electrical and Electronics Engineering (ISEE), Piscataway, USA, 2021: 106-109.
6 Shrinidhi J, Krishna P S, Yamuna B, et al. Modified min sum decoding algorithm for low density parity check codes[J]. Procedia Computer Science, 2020, 171: 2128-2136.
7 Wang X, Cao W, Li J, et al. Improved min-sum algorithm based on density evolution for low-density parity check codes[J]. IET Communications, 2017, 11(10): 1582-1586.
8 Chen F T, Zhang Y S, Du Z. Low complexity offset min-sum algorithm for 5G low density parity check codes[J]. Journal of Computer Applications, 2020, 40(7): 2028-2032
9 Nam-Ii K I M, Seung-Que L E, Jin-Up K I M. A modified min sum decoding algorithm based on approximation enhancement for LDPC codes[C]∥International Conference on Information and Communication Technology Convergence, Jeju, Korea (South), 2020: 1407-1410.
10 Wang D T, Zhou H, Qian H Y. LDPC Adaptive minimum sum decoding algorithm and its FPGA implementation[J]. Computer Science, 2021, 48(Sup.1): 608-612.
11 Zavertkin K, Panarina A, Ovinnikov A, et al. Efficient BP-based decoding algorithms for QC-LDPC codes[C]∥The 10th Mediterranean Conference on Embedded Computing, Budva, Montenegro,2021: 1-4.
12 Nimara S. Reliability assessment of flooded min-sum LDPC decoders based on sub-threshold processing units[C]∥The 22nd Euromicro Conference on Digital System Design, Kallithea, Greece, 2019: 620-623.
13 Sun R, Hou X, Sun J, et al. Reliability-based-layered belief propagation for iterative decoding of LDPC codes[C]∥IEEE International Symposium on Information Theory, Vail, USA, 2018: 1156-1160.
14 LIU X, YANG D, WANG Z. Improved decoding algorithms of LDPC codes based on reliability metrics of variable nodes[J]. IEEE Access, 2019, 7: 35769-35778.
15 Yacoub E B. Matched quantized min-sum decoding of low-density parity-check codes[C]∥IEEE Information Theory Workshop, Riva del Garda, Italy, 2021: 1-5.
16 Chen R, Chen L. Dual threshold self-corrected minimum sum algorithm for 5G LDPC decoders[J]. Information, 2020, 11(7): 355.
17 Li X, Zhang L, Tan J, et al. An improved self-corrected min-sum decoding for LDPC codes[C]∥The 16th International Conference on Intelligent Systems and Knowledge Engineering, Chengdu, China, 2021: 152-156.
18 Chen R, Chen L. A dynamic self-corrected minimum sum decoding algorithm for LDPC codes[J]. Journal of Beijing University of Posts and Telecommunications, 2020, 43(4): 15-20.
19 Chen F T, Li H B, Li P A. Improved algorithm based on offset min-sum decoding for LDPC codes[J]. Systems Engineering and Electronics, 2022, 44(7): 2350-2356.
20 Wu H, Wang H. A high throughput implementation of QC-LDPC codes for 5G NR[J]. IEEE Access, 2019, 7: 185373-185384.
[1] WU Xiao-lan, WANG Shi-gang, JI Teng-fei. The application of the sampling techniques in the DSP-based video object extraction [J]. 吉林大学学报(工学版), 2013, 43(增刊1): 217-220.
[2] HAN Wei, HUANG Jian-Guo, HAN Jing. Performance of LDPC code in underwater speech communication system [J]. 吉林大学学报(工学版), 2010, 40(05): 1377-1380.
[3] YANG Wen-Hong, WANG Ke, LIU Cheng-Cai, GAO Qing. Spark ignition engine knock detection and intensity determination based on higherorder statistics [J]. 吉林大学学报(工学版), 2010, 40(02): 376-0381.
[4] ZHAO Dan-feng，TONG Ning-ning，WU Yu-ping . Class of irregular LDPC codes with low encoding complexity [J]. 吉林大学学报(工学版), 2009, 39(02): 504-0507.
[5] Zhang He-sheng, Zhang Yi, Wen Hui-min, Hu Dong-cheng . Estimation approaches of average link travel time using GPS data [J]. 吉林大学学报(工学版), 2007, 37(03): 533-0537.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
Copyright © Journal of Jilin University(Engineering and Technology Edition), All Rights Reserved.
Tel: +86-431-85095297　Fax: +86-431-85094074　E-mail: xbgxb@jlu.edu.cn
Powered by Beijing Magtech Co. Ltd