吉林大学学报(工学版) ›› 2019, Vol. 49 ›› Issue (4): 1357-1362.doi: 10.13229/j.cnki.jdxbgxb20180185

• • 上一篇    

基于双混沌振子的最小频移键控信号频率估计方法

刘勇1(),邓方顺1,刘小林1,闵思婕2,王鹏3   

  1. 1. 中国船舶重工集团公司第七二二研究所 低频电磁通信技术实验室, 武汉 430079
    2. 武汉纺织大学 电子电气工程学院，武汉 430200
    3. 海军航空大学 信号与信息处理山东省重点实验室, 山东 烟台 264001
  • 收稿日期:2018-02-08 出版日期:2019-07-01 发布日期:2019-07-16
  • 作者简介:刘勇（1968?），男，研究员. 研究方向：低频通信. E?mail:llect722@163.com
  • 基金资助:
    国家自然科学基金项目(41606117, 41476089, 61671016)

Frequency estimation of minimum shift keying signal based on dual chaotic oscillator

Yong LIU1(),Fang⁃shun DENG1,Xiao⁃lin LIU1,Si⁃jie MIN2,Peng WANG3   

  1. 1. Laboratory of Low?frequency Electro?magnetic Communication Technology with the 722 Research Institute, China Shipbuilding Industry Corporation, Wuhan 430079, China
    2. School of Electronic and Electrical Engingeering，Wuhan Textile University，Wuhan 430200, China
    3. Signal and Information Processing Provincial Key Laboratory in Shandong, Naval Aviation University, Yantai 264001, China
  • Received:2018-02-08 Online:2019-07-01 Published:2019-07-16

RICH HTML

 4   

PDF (PC)

71

摘要：

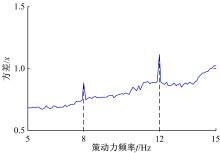
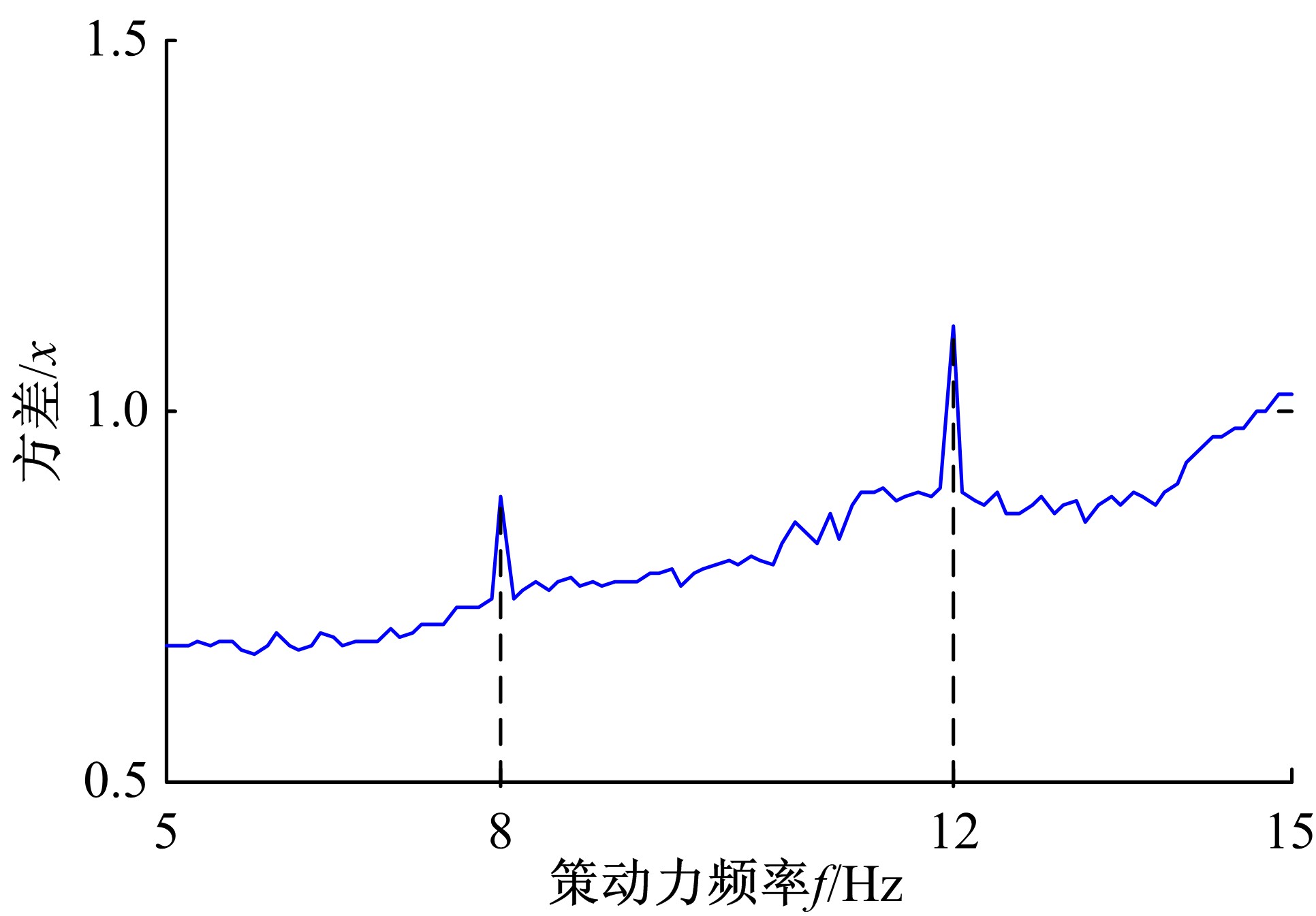
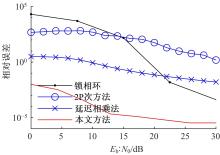
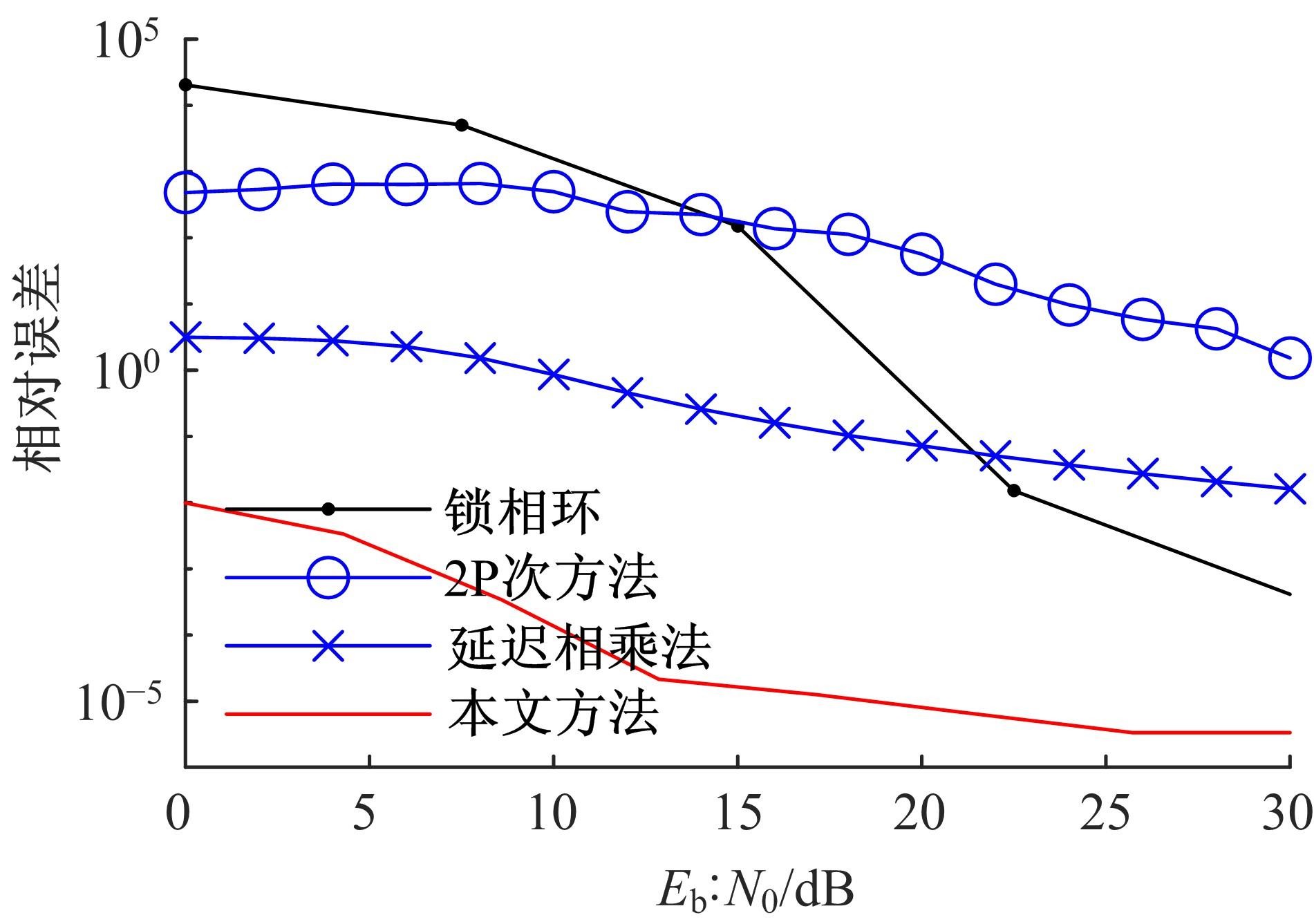
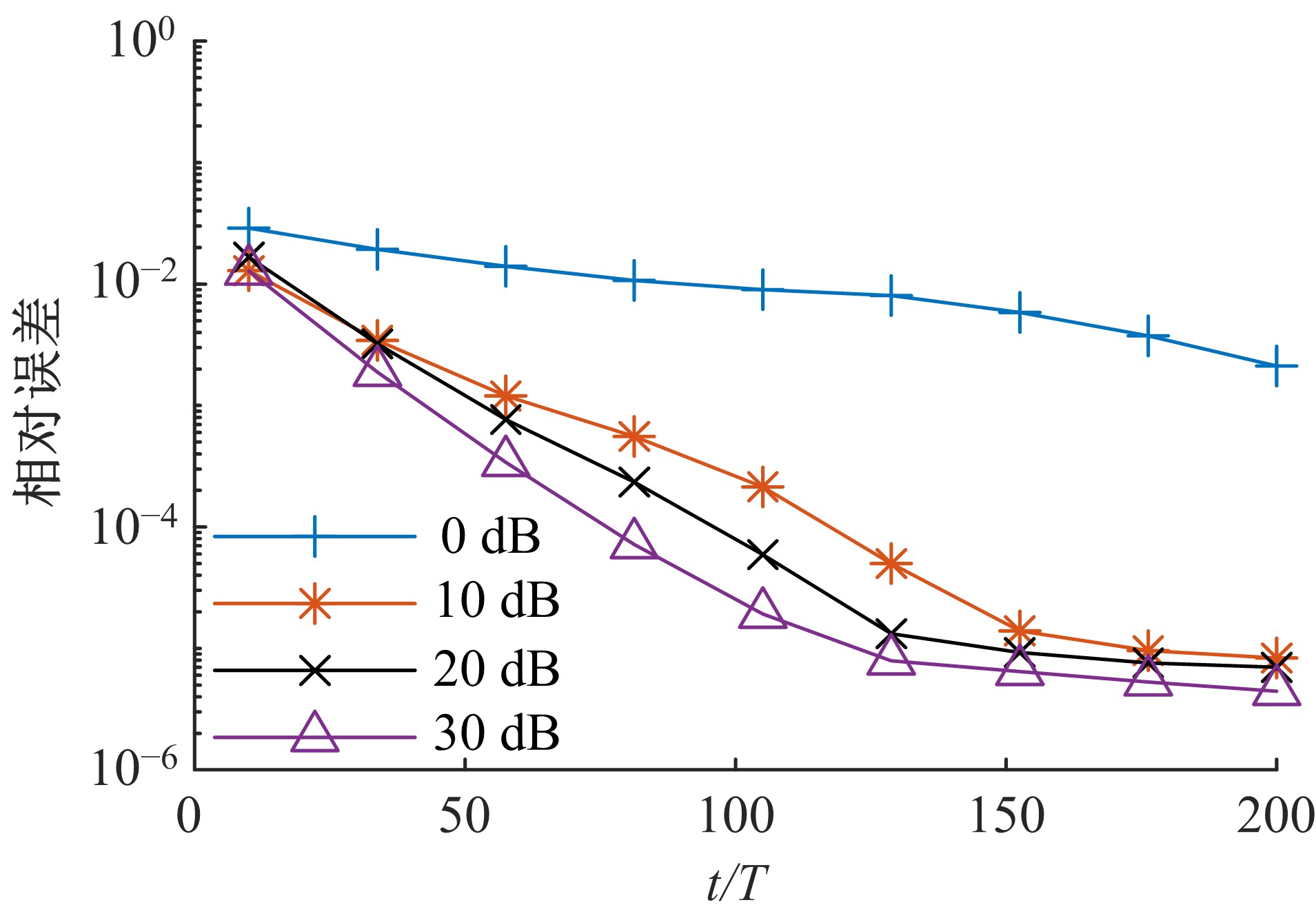
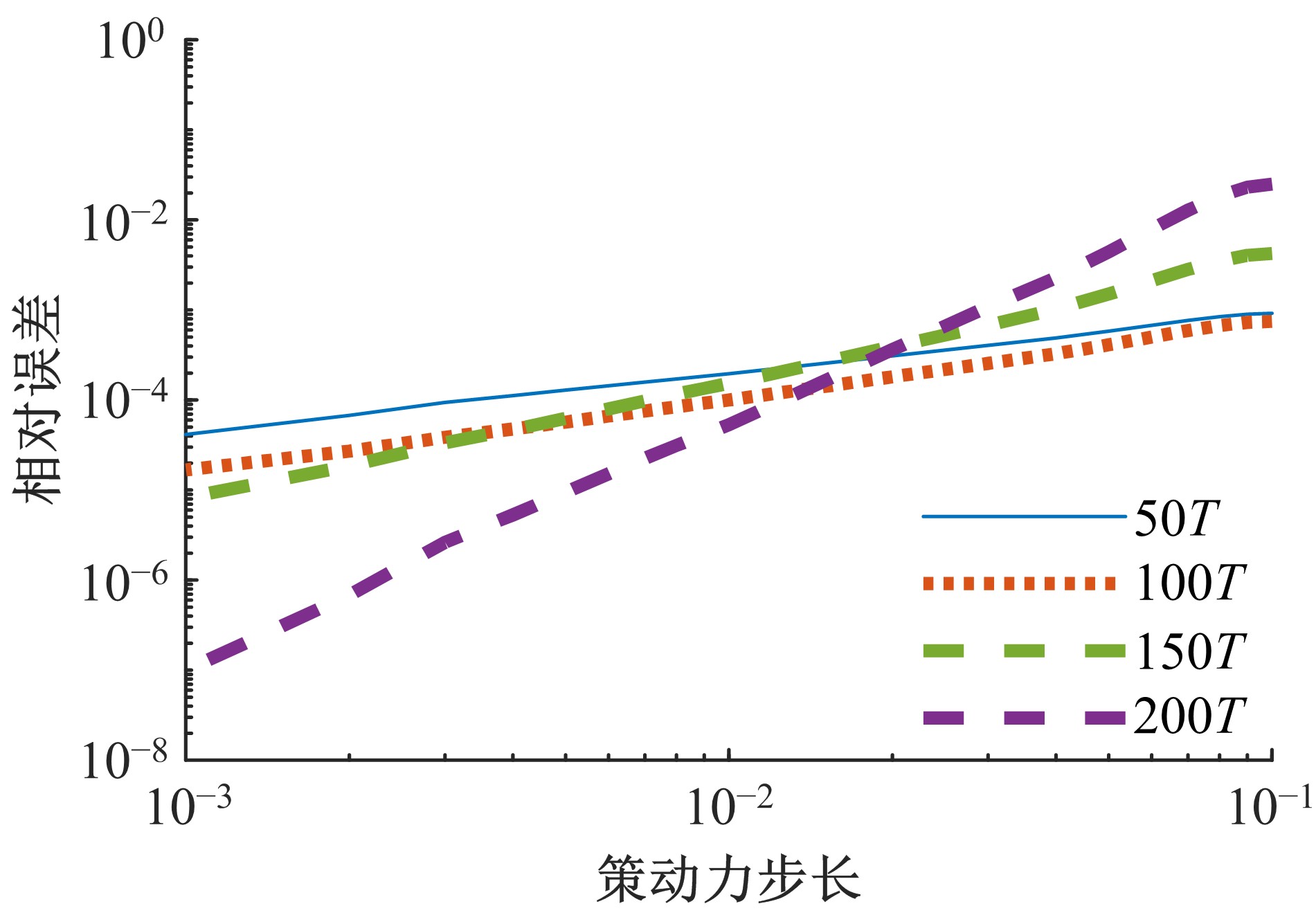
针对低信噪比条件下最小频移键控（MSK）信号频率估计的问题，提出混沌双振子频率估计算法，通过混沌振子系统策动力频率与待测信号频率的相互配合，充分利用混沌振子~信号敏感、噪声免疫的特性，实现在低信噪比条件下对待测信号频率的有效估计。理论分析和实验证明了本文算法的有效性。相对于经典的锁相环法和2P次方法，本文算法在低信噪比条件下（如Eb/N0=0 dB时）也能准确估计信号频率，且相对误差在10-2量级以下。

关键词: 通信技术, 频率估计, 混沌, Duffing振子, 最小频移键控, 多普勒频移

Abstract:

To solve the problems in the existing methods on estimating the frequency of MSK signals, especially under the condition of low signal-to-noise ratio (SNR), an algorithm of frequency estimation based on dual chaotic oscillator is proposed. By considering both the dynamic frequency of the chaotic oscillator system and the frequency of the signal to be measured, the chaotic oscillator’s characteristics of signal sensitivity and noise immunity are fully utilized to realize the signal frequency effective estimation under the low SNR conditions. Theoretical analysis and experiments show the effectiveness of the algorithm. Compared with the classical phase locked loop (PLL) and 2P-POWER methods, the new algorithm can accurately estimate the signal frequency under the condition of low SNR (eg. Eb/N0= 0 dB), and the relative error is under 10-2 orders of magnitude.

Key words: communication technology, frequency estimation, chaos, Duffing oscillator, minimum shift keying(MSK), Doppler shift

中图分类号: 

  • TN911.72

图1

双混沌振子MSK频率估计"

图2

策动力频率与方差关系图"

图3

相对误差随信噪比的变化趋势"

图4

相对误差随信号长度的变化趋势"

图5

相对误差随策动力步长的变化趋势"

1 YangG, WangJ, ZhangG, et al. Joint estimation of timing and carrier phase offsets for MSK signals in alpha⁃stable noise[J]. IEEE Communications Letters, 2018, 22(1): 89⁃92.
2 LiuH, LiaoR, WeiZ, et al. BER analysis of a hybrid modulation scheme based on PPM and MSK subcarrier intensity modulation[J]. IEEE Photonics Journal, 2015, 7(4): 1⁃10.
3 López⁃RieraA, Águila⁃LópezF D, Palá⁃SchönwälderP, et al. Joint symbol and chip synchronization for a burst⁃mode⁃communication superregenerative MSK receiver[J]. IEEE Transactions on Circuits and Systems I: Regular Papers, 2017, 64(5): 1260⁃1269.
4 GudovskiyD A, ChuL, LeeS. A novel nondata⁃aided synchronization algorithm for MSK⁃type⁃modulated signals[J]. IEEE Communications Letters, 2015, 19(9): 1552⁃1555.
5 ZhangYang⁃yong, ZhengHuan, DingKui, et al. Code⁃aided interference suppression for communication system with MSK modulation[C]∥IEEE International Symposium on Signal Processing and Information Technology (ISSPIT), Limassol, Cyprus，2016: 274⁃279.
6 芮国胜, 张洋, 苗俊, 等. 联合增益递推的duffing系统弱信号检测算法[J]. 电子学报, 2012, 40(6): 1269⁃1273.
RuiGuo⁃Sheng, ZhangYang, MiaoJun, et al. A weak signal detection method by duffing system with the gain[J]. Acta Electronica Sinica, 2012, 40(6): 1269⁃1273.
7 WangXiao⁃yu, MortazawiA. Bandwidth enhancement of RF resonators using duffing nonlinear resonance for wireless power applications[J]. IEEE Transactions on Microwave Theory and Techniques, 2016, 64(11): 3695⁃3702.
8 BajajN, SabaterA B, HickeyJ N, et al. Design and implementation of a tunable, Duffing⁃like electronic resonator via nonlinear feedback[J]. Journal of Microelectromechanical Systems, 2016, 25(1): 2⁃10.
9 孙晓东，石要武，于晓辉. 混沌干扰背景下的正弦频率估计新方法[J]. 吉林大学学报: 工学版, 2009, 39(5): 1353⁃1357.
SunXiao⁃dong, ShiYao⁃wu, YuXiao⁃hui. New frequency estimation method for sinusoidal signal submerged in strong chaotic background[J]. Journal of Jilin University(Engineering and Technology Edition), 2009, 39(5): 1353⁃1357.
10 孙晓东，权爱娟，李勇. 复杂噪声背景下谐波信号频率估计新方法[J]. 吉林大学学报: 工学版, 2015, 45(2): 653⁃657.
SunXiao⁃dong, QuanAi⁃juan, LiYong. New frequency estimation method for harmonic signal submerged in complex noise background[J]. Journal of Jilin University(Engineering and Technology Edition), 2015, 45(2): 653⁃657.
11 李国正，张波. 基于Duffing振子检测频率未知微弱信号的新方法[J]. 仪器仪表学报, 2017, 38(1): 181⁃189.
LiGuo⁃zheng, ZhangBo. Novel method for detecting weak signal with unknown frequency based on Duffing oscillator[J]. Chinese Journal of Scientific Instrument, 2017, 38(1): 181⁃189.
12 LaiZhi⁃hui, LengYong⁃gang. Weak⁃signal detection based on the stochastic resonance of bistable Duffing oscillator and its application in incipient fault diagnosis[J]. Mechanical Systems and Signal Processing, 2016, 81: 60⁃74.
13 LiGang⁃sheng, ZengLi⁃ping, ZhangLing, et al. State identification of duffing oscillator based on extreme learning machine[J]. IEEE Signal Processing Letters, 2018, 25(1): 25⁃29.
14 WangGeng, LiQiang, LiShao⁃qian. Partial⁃band jamming suppression with a noncoherent CPM detector[C]∥WRI International Conference on Communications and Mobile Computing, Kunming, China,2009: 204⁃208.
15 D'AmicoA, D'AndreaA N, MengaliU. Feedforward synchronization schemes for MSK⁃type signals[J]. Transactions on Emerging Telecommunications Technologies, 1999, 10(6): 597⁃607.
[1] 周彦果,张海林,陈瑞瑞,周韬. 协作网络中采用双层博弈的资源分配方案[J]. 吉林大学学报(工学版), 2018, 48(6): 1879-1886.
[2] 孙晓颖, 扈泽正, 杨锦鹏. 基于分层贝叶斯网络的车辆发动机系统电磁脉冲敏感度评估[J]. 吉林大学学报(工学版), 2018, 48(4): 1254-1264.
[3] 董颖, 崔梦瑶, 吴昊, 王雨后. 基于能量预测的分簇可充电无线传感器网络充电调度[J]. 吉林大学学报(工学版), 2018, 48(4): 1265-1273.
[4] 牟宗磊, 宋萍, 翟亚宇, 陈晓笑. 分布式测试系统同步触发脉冲传输时延的高精度测量方法[J]. 吉林大学学报(工学版), 2018, 48(4): 1274-1281.
[5] 姚海洋, 王海燕, 张之琛, 申晓红. 双Duffing振子逆向联合信号检测模型[J]. 吉林大学学报(工学版), 2018, 48(4): 1282-1290.
[6] 丁宁, 常玉春, 赵健博, 王超, 杨小天. 基于USB 3.0的高速CMOS图像传感器数据采集系统[J]. 吉林大学学报(工学版), 2018, 48(4): 1298-1304.
[7] 关济实, 石要武, 邱建文, 单泽彪, 史红伟. α稳定分布特征指数估计算法[J]. 吉林大学学报(工学版), 2018, 48(2): 618-624.
[8] 陈瑞瑞, 张海林. 三维毫米波通信系统的性能分析[J]. 吉林大学学报(工学版), 2018, 48(2): 605-609.
[9] 张超逸, 李金海, 阎跃鹏. 双门限唐检测改进算法[J]. 吉林大学学报(工学版), 2018, 48(2): 610-617.
[10] 孙晓颖, 王震, 杨锦鹏, 扈泽正, 陈建. 基于贝叶斯网络的电子节气门电磁敏感度评估[J]. 吉林大学学报(工学版), 2018, 48(1): 281-289.
[11] 武伟, 王世刚, 赵岩, 韦健, 钟诚. 蜂窝式立体元图像阵列的生成[J]. 吉林大学学报(工学版), 2018, 48(1): 290-294.
[12] 李炜, 李亚洁. 基于离散事件触发通信机制的非均匀传输网络化控制系统故障调节与通信满意协同设计[J]. 吉林大学学报(工学版), 2018, 48(1): 245-258.
[13] 袁建国, 张锡若, 邱飘玉, 王永, 庞宇, 林金朝. OFDM系统中利用循环前缀的非迭代相位噪声抑制算法[J]. 吉林大学学报(工学版), 2018, 48(1): 295-300.
[14] 王金鹏, 曹帆, 贺晓阳, 邹念育. 基于多址干扰和蜂窝间互扰分布的多载波系统联合接收方法[J]. 吉林大学学报(工学版), 2018, 48(1): 301-305.
[15] 单泽彪, 刘小松, 王春阳, 石屹然, 石要武. 相干/同向信号波达方向与多普勒频率的联合估计[J]. 吉林大学学报(工学版), 2017, 47(6): 1949-1956.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
版权所有 © 《吉林大学学报(工学版)》编辑部
地址：长春市人民大街5988号　电话：+86-431-85095297　传真：+86-431-85094074　E-mail: xbgxb@jlu.edu.cn
本系统由北京玛格泰克科技发展有限公司设计开发