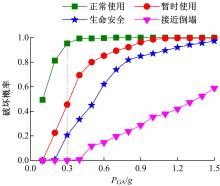
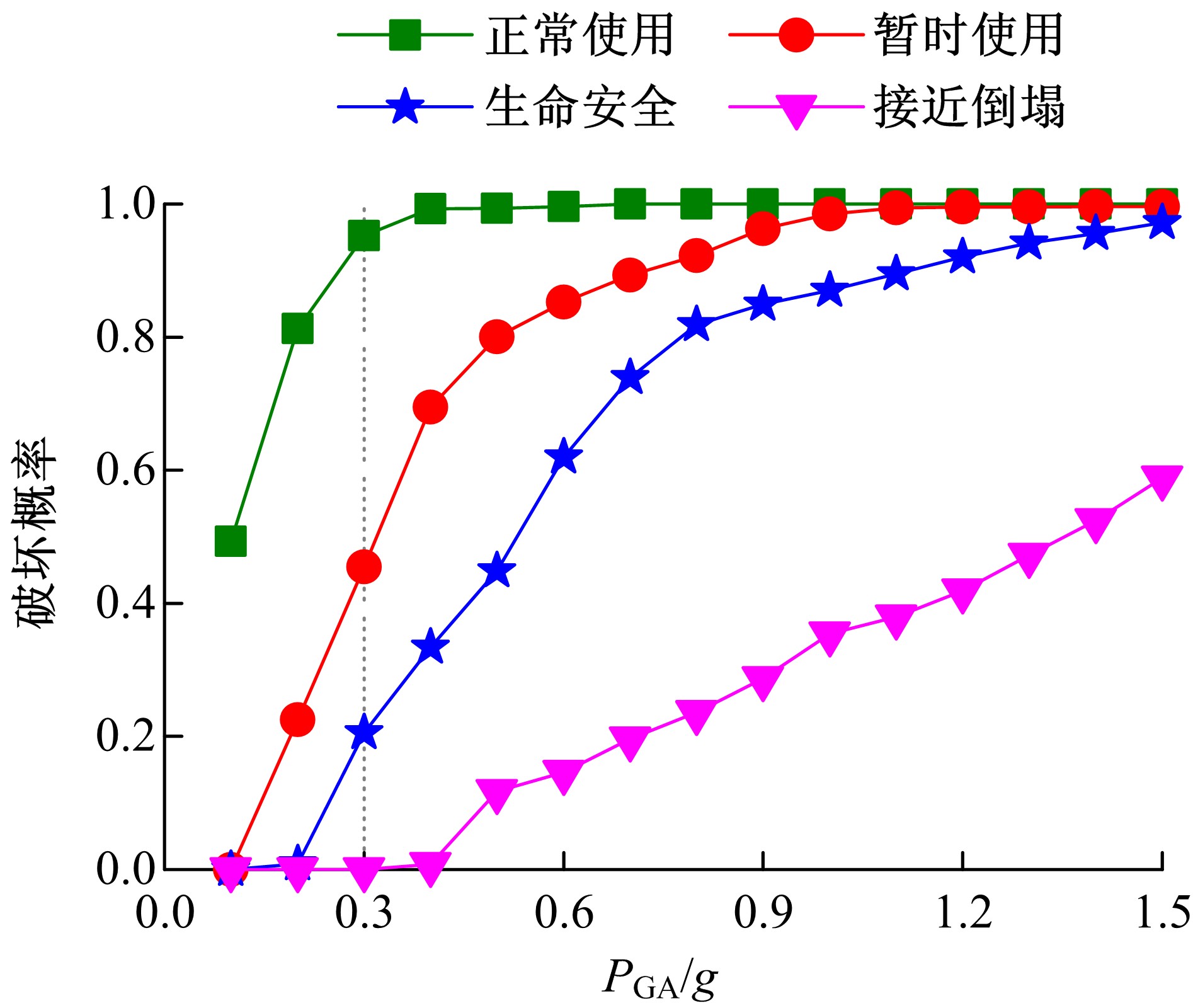
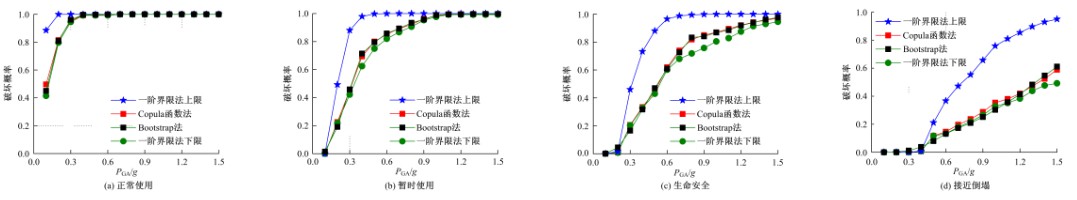
Journal of Jilin University(Engineering and Technology Edition) ›› 2020, Vol. 50 ›› Issue (1): 202-209.doi: 10.13229/j.cnki.jdxbgxb20181138

Previous Articles    

Seismic vulnerability analysis of frame structure considering floor correlation

Xiu-zhen WANG1,2(),Yong-jiu QIAN1(),Chang-jiang SHAO1,Shuai SONG3   

  1. 1. School of Civil Engineering，Southwest Jiaotong University，Chengdu 610031，China
    2. School of Civil Engineering and Hydraulic Engineering，Ningxia University，Yinchuan 750021，China
    3. School of Architecture and Civil Engineering，Taiyuan University of Technology, Taiyuan 030024，China
  • Received:2018-11-16 Online:2020-01-01 Published:2020-02-06
  • Contact: Yong-jiu QIAN E-mail:xiuzhen@live.cn;yjqian@sina.com

RICH HTML

 1   

PDF (PC)

356

Abstract:

In order to consider the correlation between each floor′s seismic demand in the vulnerability analysis of the frame structure, a frame structure vulnerability analysis method, which can consider the correlation of each floor seismic demand was proposed. The multiple Copula function method was used to deal with the correlation and edge distribution function of each floor’s seismic demand respectively. The incremental dynamic analysis method was used to get the vulnerability curve of each floor. The edge distribution function of each floor’s seismic demand was obtained by using the kernel density estimation method, which is a nonparametric method, and the corresponding parameters of the alternative Copula function were obtained. Then the optimal Copula function was selected by the minimum distance method, and the joint distribution function of each floor′s seismic demand was obtained, and the vulnerability curves of the frame structure were obtained. The results of the first order boundary method and the Bootstrap resampling method were compared. It is shown that the results of the frame structure vulnerability analysis method proposed in this paper are both located between the two boundaries of the first order boundary method, and are in good agreement with the results of the Bootstrap resampling method, which proves the accuracy of the method to a certain extent.

Key words: engineering structure, frame structure, multivariate Copula function, nonlinear correlation, Bootstrap resampling method, joint probability distribution function

CLC Number: 

  • TU375

Fig.1

"

Table 1

Statistical parameters of random parameters"

随机变量 符号 均值 变异系数 分布类型
钢筋弹性模量/MPa E s 228559 0.033[13] 正态分布
型钢弹性模量/MPa E ss 228559 0.033 正态分布
混凝土弹性模量/MPa E c 33904 0.08[14] 正态分布
钢筋屈服强度/MPa f y 384 0.078[15] 对数正态分布
型钢屈服强度/MPa f ys 384 0.078 正态分布
混凝土强度/MPa f c 34.82 0.14[15] 正态分布
阻尼比 D A 0.05 0.2[16] 正态分布
结构质量/(kN·m-2) M s 6 0.1[15] 正态分布

Table 2

Ground motion records"

地震 记录次数 发生年份 震级
Taiwan ART1(45) 34 1986 7.3
Hector Mine 6 1999 7.1
San Fernando 6 1971 6.6
Northridge?01 44 1994 6.7
Kocaeli_ urkey 4 1999 7.5
Santa Barbara 2 1978 5.9
Mammoth akes?06 2 1980 5.9
Cape Mendocino 6 1992 7.0
Big Bear?01 16 1992 6.5
Southern Calif 2 1952 6.0
Friuli_ Italy?02 4 1976 5.9

Fig.2

"

Fig.3

"

Fig.4

"

Table 3

Euclidean distance of Copula function"

P GA Gauss Copula t?Copula P GA Gauss Copula t?Copula
0.1g 0.163 0.126 0.9g 0.246 0.215
0.2g 0.237 0.162 1.0g 0.220 0.195
0.3g 0.297 0.202 1.1g 0.205 0.153
0.4g 0.325 0.236 1.2g 0.186 0.134
0.5g 0.273 0.215 1.3g 0.212 0.145
0.6g 0.232 0.182 1.4g 0.217 0.164
0.7g 0.251 0.221 1.5g 0.232 0.182
0.8g 0.256 0.213

Fig.5

Structural vulnerability curves"

Fig.6

"

1 王丹 . 钢框架结构的地震易损性及概率风险分析[D]. 哈尔滨: 哈尔滨工业大学土木工程学院, 2006.
Wang Dan . Seismic fragility analysis and probabilistic risk analysis of steel frame structures[D]. Harbin: School of Civil Engineering, Harbin Institute of Technology, 2006.
2 Gardoni P , Kiureghian A D , Mosalam K M . Probabilistic capacity models and fragility estimates for reinforced concrete columns based on experimental observations[J]. Journal of Engineering Mechanics, 2002, 128(10): 1024-1038.
3 Shinozuka M , Feng M Q , Lee J , et al . Statistical analysis of fragility curves[J]. Journal of Engineering Mechanics, 2000, 126(12): 1224-1231.
4 吕大刚, 宋鹏彦, 于晓辉, 等 . 结构动力抗震可靠度理论的研究进展[J]. 西南交通大学学报, 2015, 50(6): 1025-1038.
Lv Da-gang , Song Peng-yan , Yu Xiao-hui , et al . Research progress of structural dynamic seismic reliability theory[J]. Journal of Southwest Jiaotong University, 2015, 50(6): 1025-1038.
5 吕大刚, 于晓辉, 陈志恒 . 钢筋混凝土框架结构侧向倒塌地震易损性分析[J]. 哈尔滨工业大学学报, 2011, 43(6): 1-5.
Lv Da-gang , Yu Xiao-hui , Chen Zhi-heng . Seismic vulnerability analysis of reinforced concrete frame structures subjected to lateral collapse[J]. Journal of Harbin Institute of Technology, 2011, 43 (6): 1-5.
6 宋帅, 钱永久, 吴刚 . 基于Copula函数的桥梁系统地震易损性方法研究[J]. 工程力学, 2016, 33(11): 193-200.
Song Shuai , Qian Yong-jiu , Wu Gang . Study on seismic vulnerability of bridge system based on Copula function[J]. Engineering Mechanics, 2016, 33(11): 193-200.
7 Hong H P , Zhou W , Zhang S , et al . Optimal condition-based maintenance decisions for systems with dependent stochastic degradation of components[J]. Reliability Engineering and System Safety, 2014, 121: 276-288.
8 李鹏举, 朱辉 . 基于Copula函数的金融理财产品风险度量[J]. 系统工程理论与实践, 2014, 34(3): 663-667.
Li Peng-ju , Zhu Hui . Risk measurement of financial products based on Copula function[J]. System Engineering Theory and Practice, 2014, 34 (3): 663-667.
9 张英芝, 刘津彤, 申桂香, 等 . 基于故障相关性分析的数控机床系统可靠性建模[J]. 吉林大学学报:工学版, 2017, 47(1): 169-173.
Zhang Ying-zhi , Liu Jin-tong , Shen Gui-xiang , et al . Reliability modeling of CNC machine tools system based on failure correlation analysis[J]. Journal of Jilin University (Engineering and Technology Edition), 2017, 47(1): 169-173.
10 张英芝, 郑锐, 申桂香, 等 . 基于COPULA理论的数控装备故障相关性[J]. 吉林大学学报:工学版, 2011, 41(6): 1636-1640.
Zhang Ying-zhi , Zheng Rui , Shen Gui-xiang , et al . Failure dependency of CNC equipment based on copula theory[J]. Journal of Jilin University (Engineering and Technology Edition), 2011, 41(6): 1636-1640.
11 曹晋华, 程侃 . 可靠性数学引论[M]. 北京：高等教育出版社, 2012.
12 刘月飞 . 考虑失效模式和验证模式相关性的桥梁结构体系可靠度分析[D]. 哈尔滨: 哈尔滨工业大学土木工程学院, 2015.
Liu Yue-fei . Reliability analysis of bridge structural system considering failure mode and verification mode correlation [D]. Harbin：School of Civil Engineering， Harbin Institute of Technology, 2015.
13 Mirza S A , Macgregor J G . Variability of mechanical properties of reinforced bars[J]. Journal of Structural Division, 1979, 105(5): 921-937.
14 Mirza S A , Hatzinikolas M , Macgregor J G . Statistical descriptions of strength of concrete[J]. Journal of the Structural Division, 1979, 105(6): 1021-1037.
15 GB50010―2010. 钢筋混凝土结构设计规范[S].
16 Porter K A , Beck J L , Shaikhutdinov R V . Sensitivity of building loss estimates to major uncertain variables[J]. Earthquake Spectra, 2002, 18(4):719-743.
17 Nielson B , Pang W . Effect of ground motion suite size on uncertainty estimation in seismic bridge fragility modeling[C]∥Structures Congress 2011 of ASCE, Las Vegas, Nevada, United States, 2011: 23-34.
18 陈波 . 结构非线性动力分析中地震动记录的选择和调整方法研究[D]. 北京: 中国地震局地球物理研究所, 2014.
Chen Bo .Ground motion selection and modification methods for performing nonlinear dynamic analysis of buildings[D]. Beijing: Institute of Geophysics, China Seismological Bureau, 2014.
19 GB50011―2010. 建筑抗震设计规范[S].
20 王秋维, 史庆轩, 杨坤 . 型钢混凝土结构抗震性态水平和容许变形值的研究[J]. 西安建筑科技大学学报:自然科学版, 2009, 41(1): 82-87.
Wang Qiu-wei , Shi Qing-xuan , Yang Kun . Research on the seismic behavior and admissible deformation of steel reinforced concrete structures [J]. Journal of Xi'an University Of Architecture and Technology (Natural Science Edition), 2009, 41(1): 82-87.
21 Scaillet O , Fermanian J D . Nonparametric estimation of copulas for time series[J]. Journal of Risk, 2003, 5(3): 25-54.
22 谢中华 . MATLAB统计分析与应用: 40个案例分析[M]. 北京: 北京航空航天大学出版社, 2012.
23 胡云昌, 郭振邦 . 结构系统可靠性分析原理及应用[M].天津： 天津大学出版社, 1992.
24 Lee H K H . Elements of computational statistics[J]. Journal of the American Statistical Association, 2003, 98(463): 772-773.
[1] NI Ying-sheng, SUN Qi-xin, MA Ye, XU Dong. Calculation of capacity reinforcement about composite box girder with corrugated steel webs based on tensile stress region theory [J]. 吉林大学学报(工学版), 2018, 48(1): 148-158.
[2] ZHANG Yan-ling, SUN Tong, HOU Zhong-ming, LI Yun-sheng. Bending-torsion characteristics of steel-concrete curved composite beams stiffened with diaphragms [J]. 吉林大学学报(工学版), 2015, 45(4): 1107-1114.
[3] WANG Chun-gang, ZHANG Zhuang-nan, ZHAO Da-qian, CAO Yu-fei. Experimental investigation of Σ-section channel steel with complex edge stiffeners and web holes under axial compression [J]. 吉林大学学报(工学版), 2015, 45(3): 788-796.
[4] LI Hai-feng,GUO Xiao-nong,LUO Yong-feng,GAO Xuan-neng. Collapse resistance behavior of Ferris wheel with stay cables [J]. 吉林大学学报(工学版), 2015, 45(2): 406-413.
[5] GUO Jun-ping, DENG Zong-cai, LU Hai-bo, LIN Jin-song. Experiment on shear behavior of reinforced concrete beams strengthened with prestressed high strength steel wire mesh [J]. 吉林大学学报(工学版), 2014, 44(4): 968-977.
[6] JIANG Feng-guo, ZHAO Jing-lu, ZHENG Zhong-yuan. Reliability analysis of steel columns exposed to fire by load and resistance factor design [J]. 吉林大学学报(工学版), 2014, 44(4): 978-984.
[7] JIANG Feng-guo, ZHAO Jing-lu. Reliability analysis of reinforced concrete member under fire load [J]. 吉林大学学报(工学版), 2013, 43(06): 1500-1503.
[8] BO Ming-Yuan, YAO Ji-Tao. Reliability of reinforced concrete structural element [J]. 吉林大学学报(工学版), 2010, 40(增刊): 218-0221.
[9] ZHANG Huan, WANG Wen-Bo, GAO Wei-Dong, BENG Mu-Gen. Resource allocation for multihop relay links with adaptive frame structure [J]. 吉林大学学报(工学版), 2010, 40(02): 571-0575.
[10] LI Li-ming,CHEN Yi-yi,LI Ning,CAI Yu-chun. Seismic performance of outer-shell connection of cold-formed square tubular column and H-shaped steel beam [J]. 吉林大学学报(工学版), 2010, 40(01): 67-0071.
[11] ZHANG Su-mei, YUN Di . Lateral brace arrangement for large-span half-through concrete filled steel tube arch bridge [J]. 吉林大学学报(工学版), 2009, 39(01): 108-112.
[12] SHI Gang,SHI Yong-jiu,WANG Yuan-qing . Analysis on overall buckling behaviour of ultra-high strength steel columns by ANSYS [J]. 吉林大学学报(工学版), 2009, 39(01): 113-118.
[13] LIANG Ping, ZHANG Xu-li, XU Tao . Weighted generalized inverse method for topological modification of frame structures [J]. 吉林大学学报(工学版), 2008, 38(06): 1366-1370.
[14] Shi Gang, Shi Yong-jiu, Wang Yuan-qing. Calculational method of shear deformation for beam-to-column connection panel zone in steel frames [J]. 吉林大学学报(工学版), 2006, 36(04): 462-466.
[15] SHI Gang, SHI Yongjiu, WANG Yuanqing. Momentrotation Hysteretic Model of Semirigid Endplate Connections in Steel Frames [J]. 吉林大学学报(工学版), 2005, 35(06): 654-0660.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
Copyright © Journal of Jilin University(Engineering and Technology Edition), All Rights Reserved.
Tel: +86-431-85095297　Fax: +86-431-85094074　E-mail: xbgxb@jlu.edu.cn
Powered by Beijing Magtech Co. Ltd