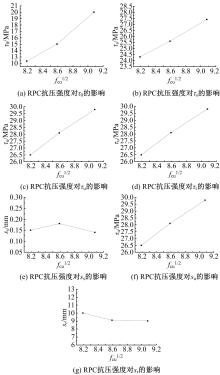
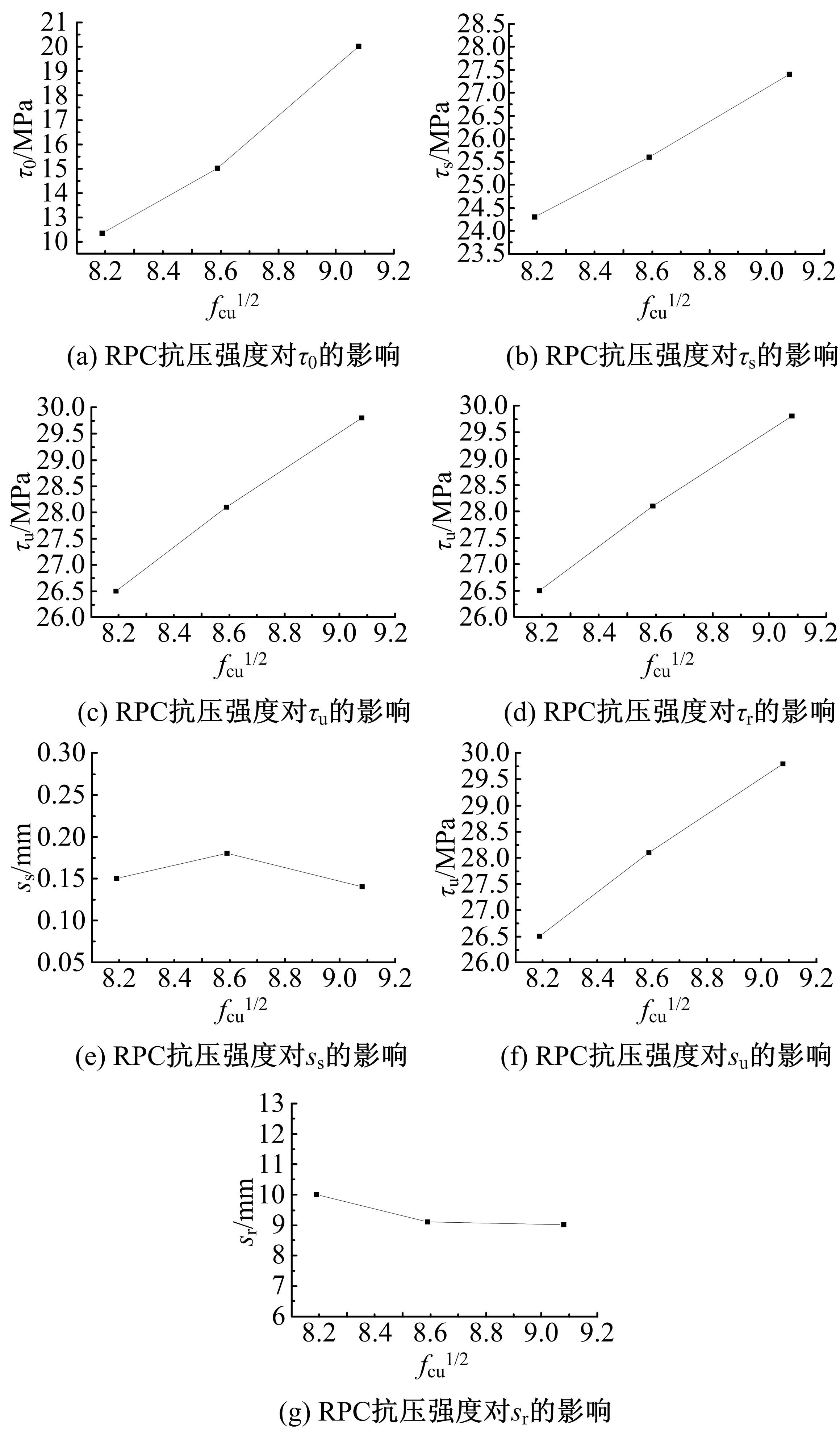
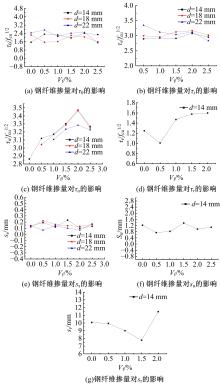
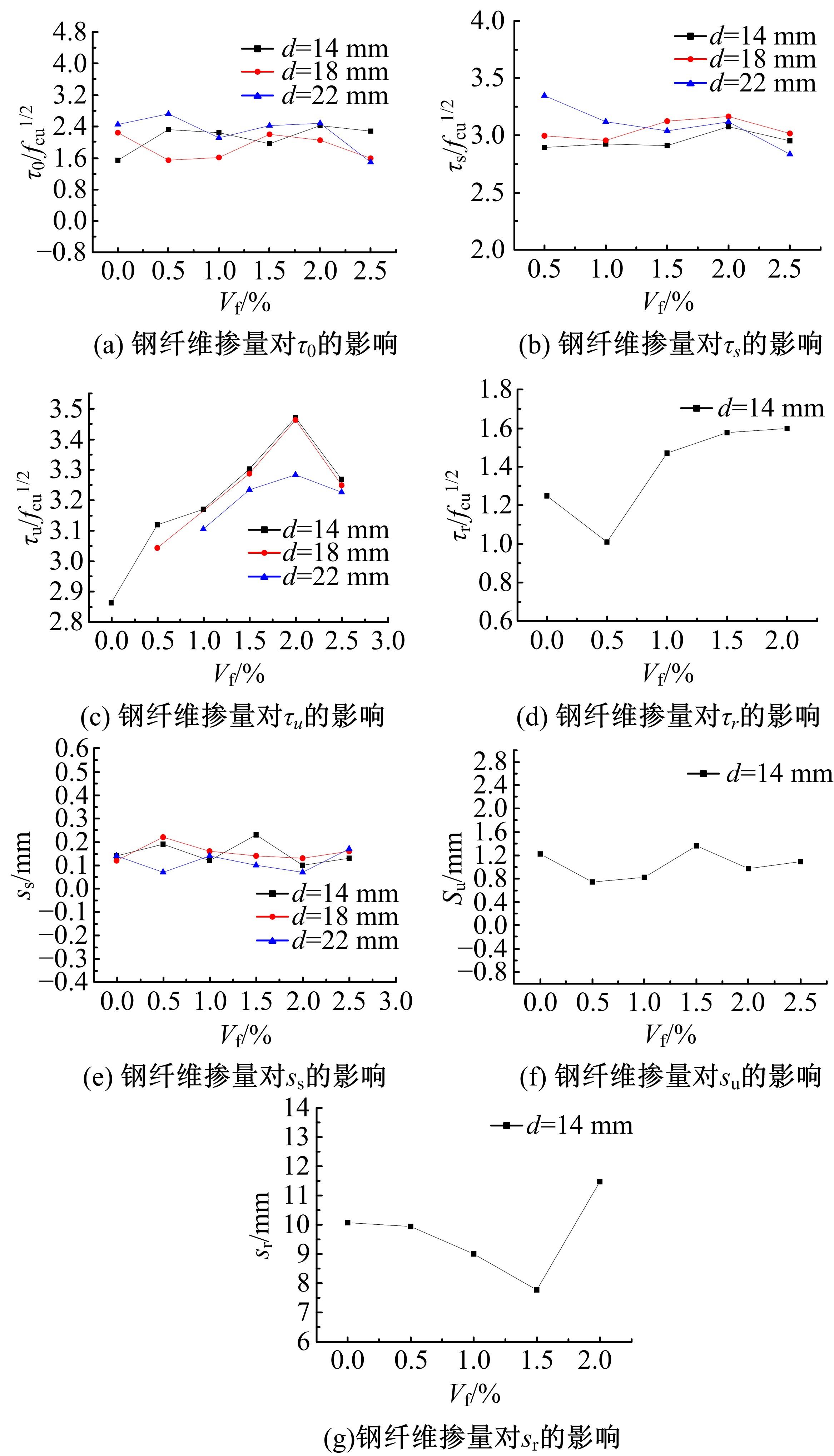
Journal of Jilin University(Engineering and Technology Edition) ›› 2021, Vol. 51 ›› Issue (4): 1317-1330.doi: 10.13229/j.cnki.jdxbgxb20200427
Bond-slip constitutive model of steel bars and reactive powder concrete under standard curing
Dong-hui CHENG( ),Yong-xuan FAN,Yan-song WANG
),Yong-xuan FAN,Yan-song WANG
- School of Civil Engineering,Northeast Forestry University,Harbin 150040,China
CLC Number:
- TU378
| 1 | Richard P, Cheyrezy M H.Composition of reactive powder concretes[J]. Cement and Concrete Research, 1995, 25(7) : 1501-1511. |
| 2 | Richard Pierre. Reactive powder concrete: a new ultra high strength cementitious Material[C]∥The 4th International Symposium On Utilization of High Strength High Performance Concrete, Paris,France,1996:1343-1349. |
| 3 | 吴中伟,廉慧珍. 高性能混凝土[M].北京:中国铁道出版社,1999. |
| 4 | 柯开展,蔡文尧. 活性粉末混凝士(RPC)在工程结构中的应用与前景[J].福建建材, 2006,24(2): 17-19. |
| Ke Kai-zhan,Cai Wen-yao. Application and prospect of reactive powder concrete (RPC) in engineering structure[J]. Fujian Building Materials, 2006,24(2): 17-19. | |
| 5 | 杨志慧.不同钢纤维掺量活性粉末混凝土的抗拉力学特性研究[D]. 北京:北京交通大学土木工程学院,2006. |
| Yang Zhi-hui. Study on tensile mechanical properties of reactive steel reinforced concrete with different steel fibers[D]. Beijing: School of Civil Engineering,Beijing Jiaotong University,2006. | |
| 6 | 周一桥,杜亚凡. 世界上第一座预制预应力活性粉混凝土结构—舍布鲁克人行桥[J].国外桥梁,2000,27(3):18-23. |
| Zhou Yi-qiao, Du Ya-fan. The world's first precast prestressed reactive powder concrete structure - Sherbrooke footbridge[J]. Foreign Bridges, 2000,27(3): 18-23. | |
| 7 | Adeline R, Lachemi M, Blais P. Design and behaviour of the Sherbrooke Footbridge[C]∥Proceedings of International Symposium on High-performance and Reactive Powder Concrete,Sherbrooke,Canada,1998:89-97. |
| 8 | 贾方方. 钢筋与活性粉末混凝土粘结性能的试验研究[D].北京:北京交通大学土木工程学院,2013. |
| Jia Fang-fang. Experimental study on the bonding properties of steel bars and reactive powder concrete[D]. Beijing:School of Civil Engineering,Beijing Jiaotong University, 2013. | |
| 9 | An Ming-zhe,Ji Wen-yu,Zhong Tie-yi,et al. Application of UHPC in railway construction[C]∥International Workshop on Sustainable and Science-Driven Engineering of Cement-based Materials,Beijing,China,2011:141-148. |
| 10 | . 活性粉末混凝土[S]. |
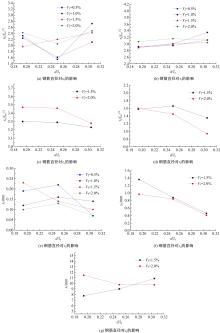
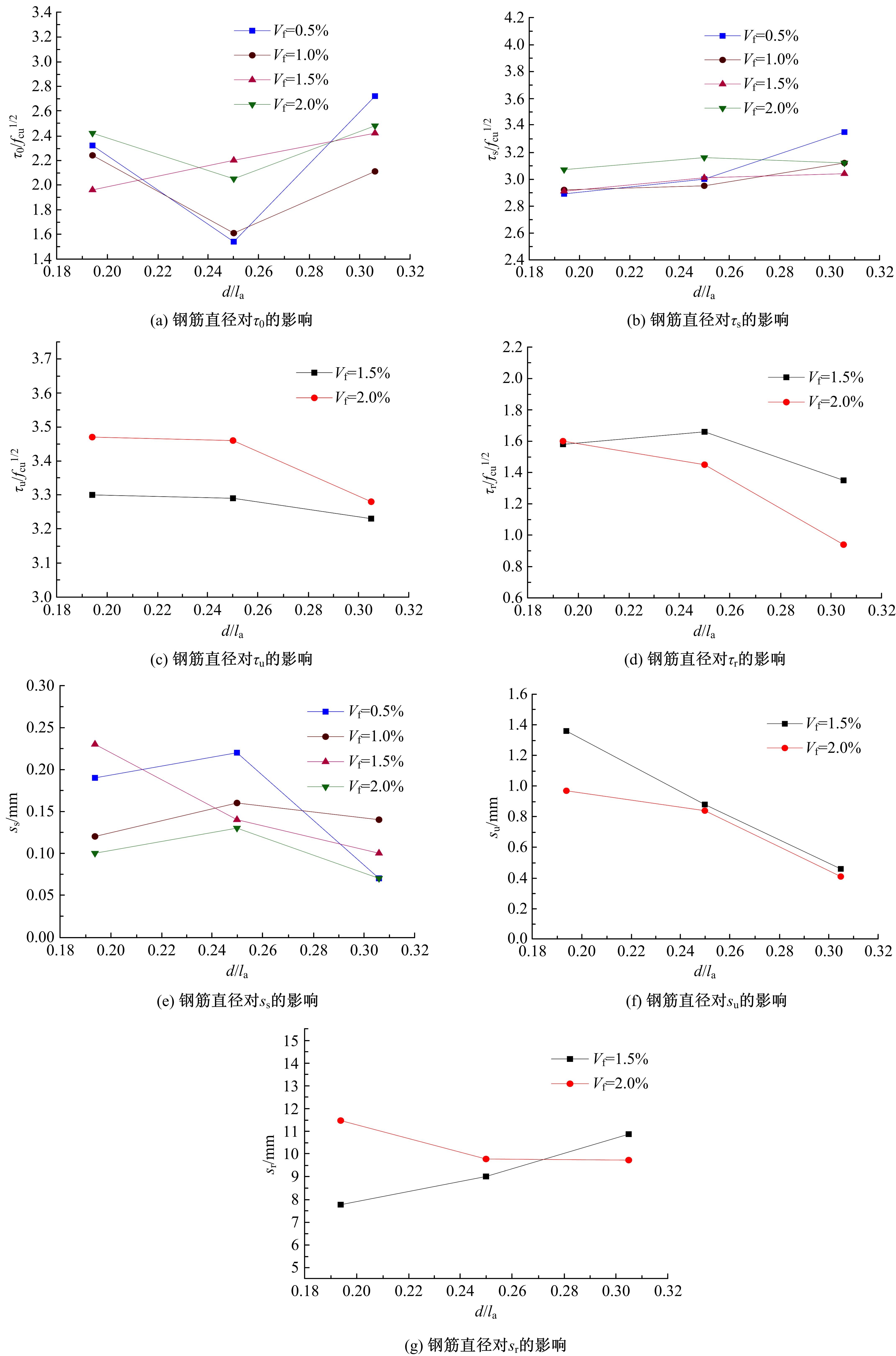
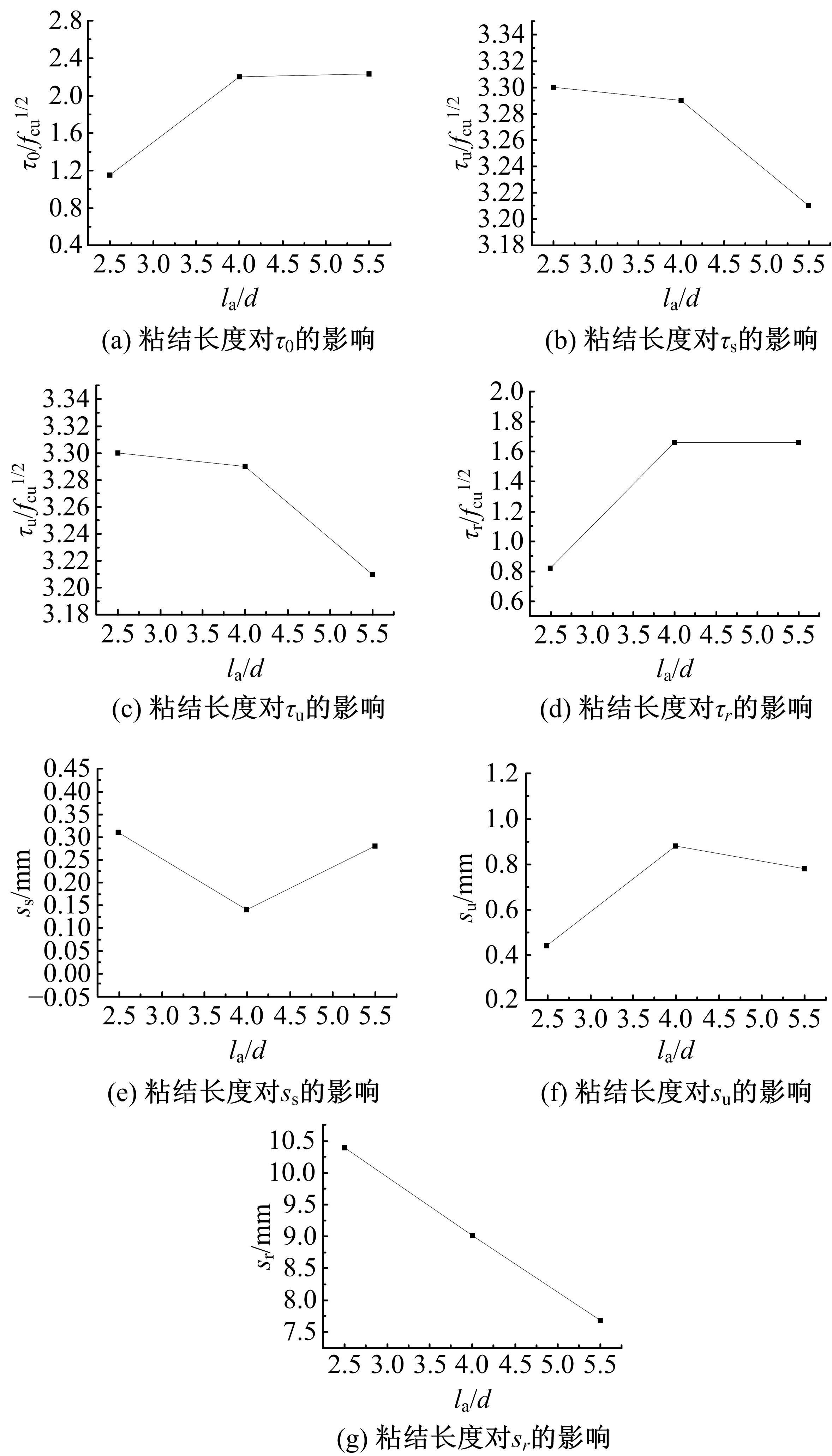
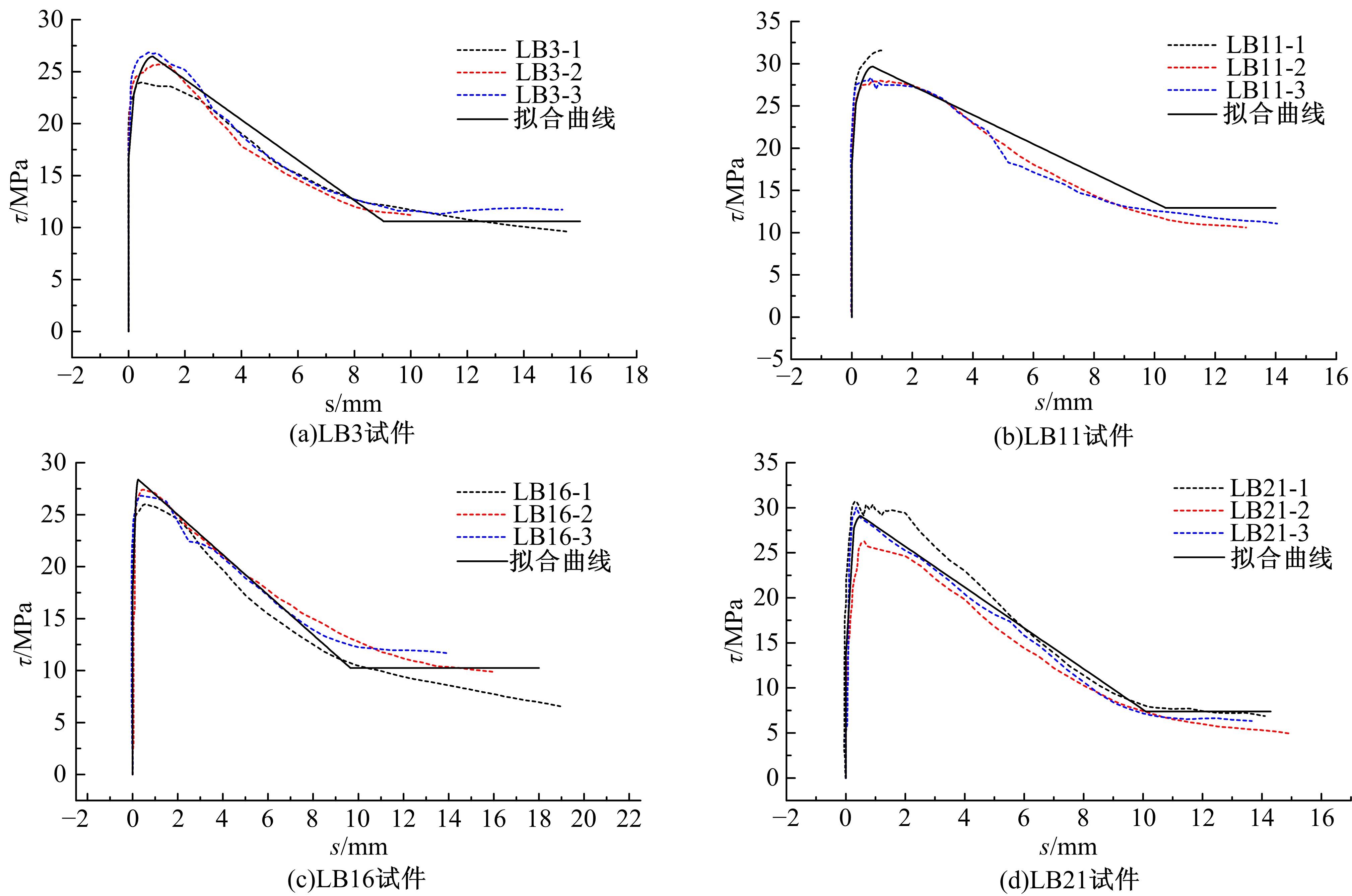
| 11 | 李新星. 大流动性活性粉末混凝土与钢筋的粘结滑移本构理论及试验研究[D].湘潭:湘潭大学土木工程与力学学院,2019. |
| Li Xin-xing. The theory and experimental study of the adhesion slip structure of large liquid active powder concrete and steel bar[D]. Xiangtan:School of Civil Engineering and Mechanics, Xiangtan University, 2019. | |
| 12 | 赵北祥,王晓慧,张延亿.钢筋与活性粉末混凝土粘结滑移本构关系研究[J].科技资讯,2011,8(22):85-88. |
| Zhao Bei-xiang,Wang Xiao-hui,Zhang Yan-yi. A study on the relationship between rebar and active powder concrete bond slip[J]. Science & Technology Information,2011,8(22):85-88. | |
| 13 | CECS13:89 钢纤维混凝土试验方法[M].北京:中国建筑工业出版社,1989. |
| 14 | Xiao Jian-zhuang, Falkner H. Bond behavior between recycled aggregate concrete and steel rebars[J]. Construction and Building Materials,2007,21(2):395-401. |
| 15 | 徐有邻,沈文都. 钢筋外形对粘结性能的影响[J]. 工业建筑, 1987, 17(3): 26-30. |
| Xu You-lin, Shen Wen-du. Effect of reinforcement shape on bonding properties[J]. Industrial Construction, 1987, 17(3): 26-30. | |
| 16 | 卫军,王艺霖,罗晓辉.钢筋拉拔试验中粘结应力分布的曲线拟合[J].铁道科学与工程学报,2005(2):22-24. |
| Wei Jun,Wang Yi-lin,Luo Xiao-hui. Curve fitting of bond stress distribution in steel bar drawing test[J]. Journal of Railway Science and Engineering,2005(2):22-24. | |
| 17 | 徐有邻.变形钢筋-混凝土粘结锚固性能的试验研究[D]. 北京:清华大学土木工程学院,1990. |
| Xu You-lin. Experimental study on the anchoring performance of deformed steel-concrete bonding[D]. Beijing: School of Civil Engineering and Engineering,Tsinghua University, 1990. |
| [1] | Han-bing LIU,Xin GAO,Ya-feng GONG,Shi-qi LIU,Wen-jun LI. Influence of surface treatment on basalt fiber reactive powder concrete mechanical properties and fracture characteristics [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(3): 936-945. |
| [2] | Wei-min ZHUANG,Peng-yue WANG,Rui-juan GAO,Dong-xuan XIE. Effect of hot forming on static mechanical properties of AA5754 aluminum alloy [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(3): 847-854. |
| [3] | Xue-wen CHEN,Ji-ye WANG,Xi-qing YANG,Tao HUANG,Ke-xing SONG. Hot deformation behavior and dislocation density evolution regularity of Cr8 alloy [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(1): 91-99. |
| [4] | Hui YE,Yan-rong ZHU,Yong-feng PU. Numerical simulation of strain rate effect of fiber reinforced composites [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(5): 1622-1629. |
| [5] | JI Wen-yu, LI Wang-wang, GUO Min-long, WANG Jue. Experimentation and calculation methods of prestressed RPC-NC composite beam deflection [J]. 吉林大学学报(工学版), 2018, 48(1): 129-136. |
| [6] | ZHUANG Wei-min, XIE Dong-xuan, YU Tian-ming, YU Wan-dong. Numerical simulation of hot forming of high-strength steel based on damage-phase transformation constitutive model [J]. 吉林大学学报(工学版), 2015, 45(4): 1206-1212. |
| [7] | XIAO Rui, DENG Zong-cai, LAN Ming-zhang, SHEN Chen-liang. Experiment research on proportions of reactive powder concrete without silica fume [J]. 吉林大学学报(工学版), 2013, 43(03): 671-676. |
|
||