基于CA的无信号灯控制路段行人过街横道处动态博弈礼让行为

doi:10.13229/j.cnki.jdxbgxb20200954

Abstract

Abstract:

This paper analyzes the traffic characteristics of motor vehicle drivers and pedestrians in the crosswalk traffic conflict area. The drivers are divided into two types： comity and impoliteness， and the ratio of motor vehicle comity are introduced. At the same time， on the basis of the Non-game theory cellular automata （NCA） model， the dynamic game update rules are introduced to establish the Game theory cellular automata （GCA） model， which overcomes the need to describe the two parties through the spatial position relationship in NCA model. Comprehensive analysis shows that the GCA model is more realistic than the NCA model， and can better simulate the traffic conflict between drivers and pedestrians at crosswalks without signal lights.

Key words: urban traffic, pedestrian′s crossing, traffic conflict, cellular automata model, dynamic game theory

CLC Number:

U268.6

Xian-yan KUANG,Zi-ru CHEN. Dynamic game comity behavior at pedestrians′ crossing on unsignal-controlled roads based on cellular automata[J].Journal of Jilin University(Engineering and Technology Edition), 2022, 52(4): 837-846.

Figures/Tables 11

Fig.1

Fig.2

Fig.3

Table 1

Speed classification"

车速等级	车速/ $(k m ? h - 1)$	元胞速度/ $(c e l l ? s - 1)$
低速	$v ≤ 35$	$v ≤ 6$
中速	$35 < v ≤ 55$	$6 < v ≤ 10$
高速	$v > 55$	$v > 10$

Table 1

Table 2

Delay and risk settings of drivers"

损失设定	低速	中速	高速
延误 $u 1 d$	-1	-2	-3
风险 $u 1 c$	-2	-6	-10

Table 2

Table 3

Delay and risk settings of pedestrians"

损失设定	低速	中速	高速
延误 $u 2 d$	-1	-3	-5
风险 $u 2 c$	-2	-6	-8

Table 3

Fig.4

Fig.5

Fig.6

Fig.7

Fig.8

References 30

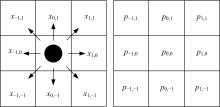
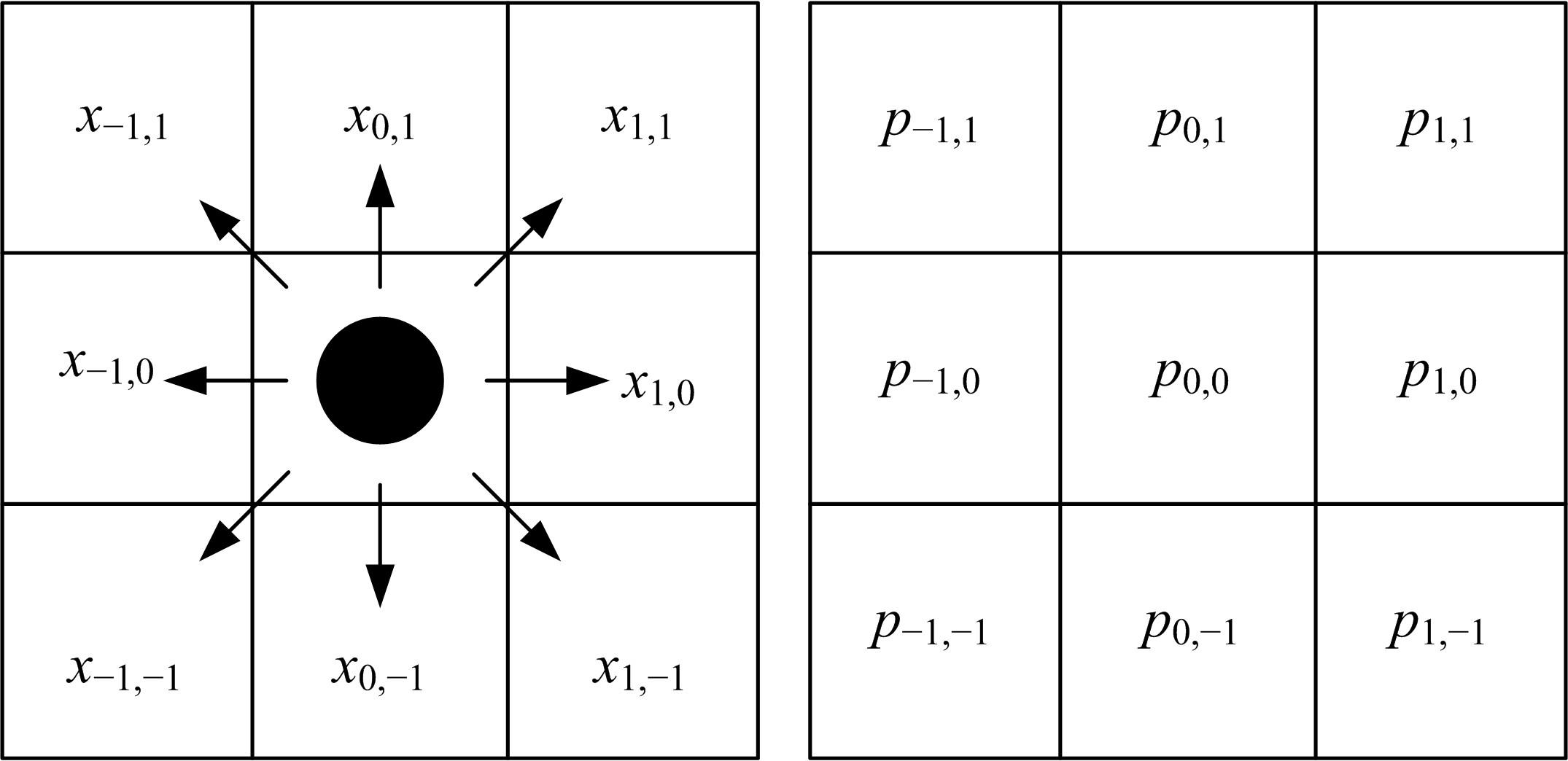
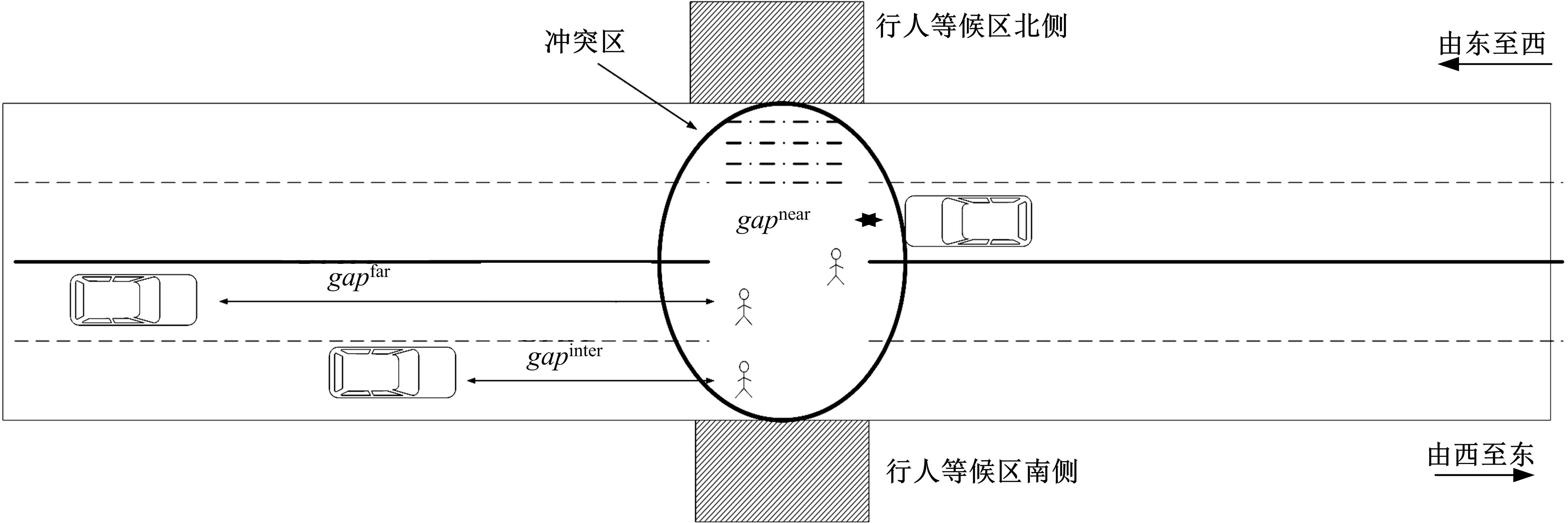
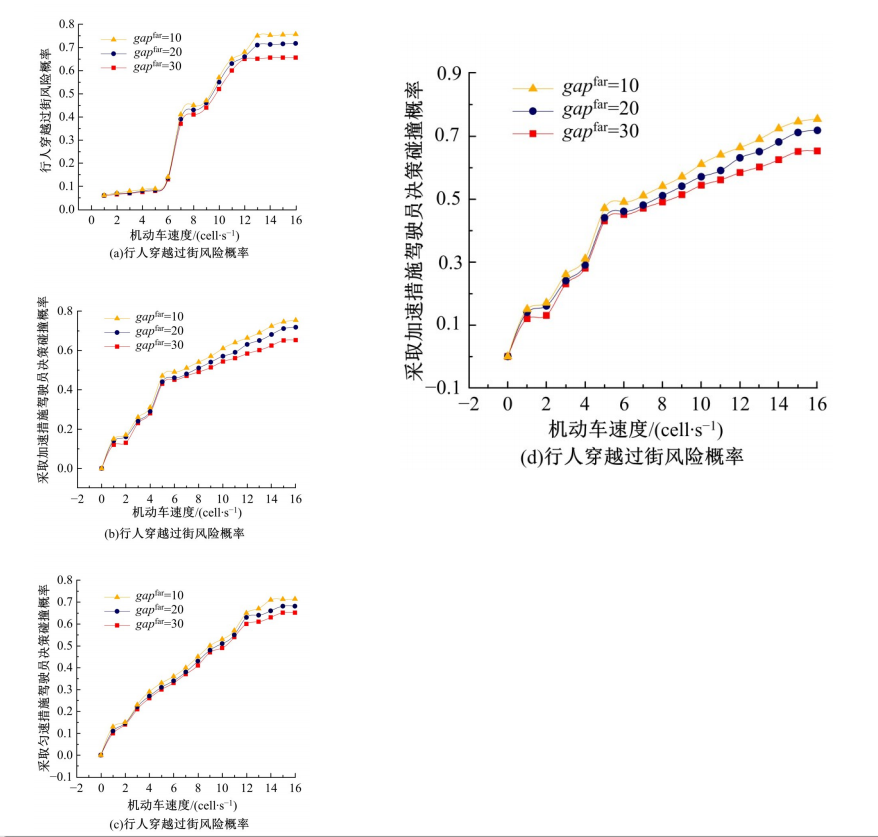
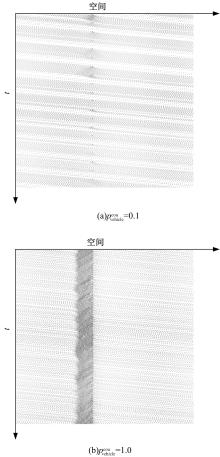
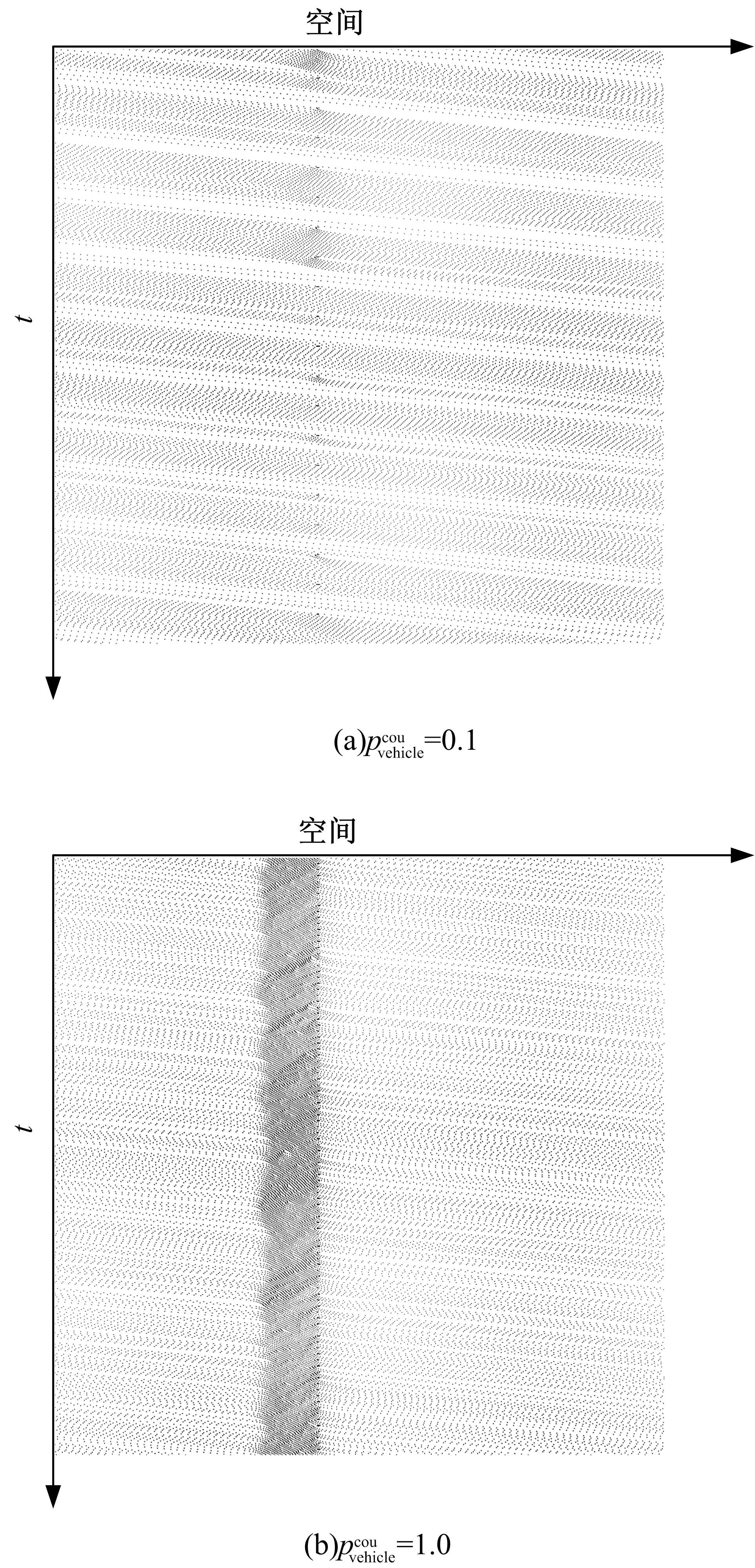
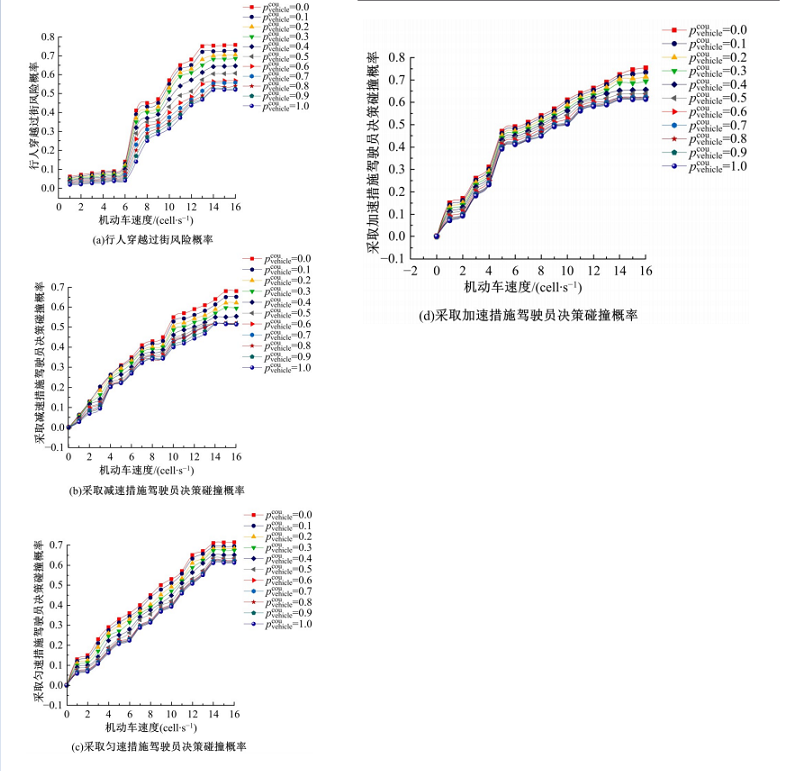
1	Nagel K, Schreckenberg M. A cellular automaton model for freeway traffic[J].Journal de Physique I, 1992, 2(12): 2221-2229.
2	Tian J F, Li G Y, Treiber M, et al. Cellular automaton model simulating spatiotemporal patterns, phase transitions and concave growth pattern of oscillations in traffic flow[J]. Transportation Research Part B: Methodological, 2016, 93: 560-575.
3	Hu X, Hao X, Wang H, et al. Research on on-street temporary parking effects based on cellular automaton model under the framework of Kerner's three-phase traffic theory[J]. Physica A: Statistical Mechanics and its Applications, 2020, 545: No. 123725.
4	Jin S, Xu L, Xu C, et al. Lane width‐based cellular automata model for mixed bicycle traffic flow[J]. Computer-Aided Civil and Infrastructure Engineering, 2019, 34(8): 696-712.
5	Liu Q, Sun J, Tian Y, et al. Modeling and simulation of nonmotorized vehicles' dispersion at mixed flow intersections[J]. Journal of Advanced Transportation, 2019, 5:1-19.
6	岳昊, 邵春福, 陈晓明, 等. 基于元胞自动机的对向行人交通流仿真研究[J]. 物理学报, 2008, 57(11): 6901-6908.
	Yue Hao, Shao Chun-fu, Chen Xiao-ming, et al. Simulation of bi-drectional pedestrian flow based on cellular automata model[J]. Acta Physica Sinica,2008, 57(11): 6901-6908.
7	Tao Y Z, Dong L Y. A cellular automaton model for pedestrian counterflow with swapping[J]. Physica A Statistical Mechanics and Its Applications, 2017, 475: 155-168.
8	陈沂川. 基于元胞自动机的城市轨道交通流特性研究[D]. 北京:北京交通大学电子信息工程学院, 2011.
	Chen Yi-chuan. Research on property of train flow in city express railway system based on cellular automata[D]. Beijing: School of Electronic and Information Engineering, Beijing Jiaotong University, 2011.
9	齐姗姗, 彭其渊. 改进的元胞自动机列车交通流仿真模型研究[J]. 计算机工程与应用, 2019, 55(24): 254-258.
	Qi Shan-shan, Peng Qi-yuan. Train flow research of moving block system based on improved cellular automation model[J]. Computer Engineering and Applications, 2019, 55(24):254-258.
10	Lu L, Ren G, Wang W, et al. A cellular automaton simulation model for pedestrian and vehicle interaction behaviors at unsignalized mid-block crosswalks[J]. Accident Analysis & Prevention, 2016, 95(B): 425-437.
11	Zhao H T, Liu X R, Chen X X, et al. Cellular automata model for traffic flow at intersections in internet of vehicles[J]. Physica A: Statistical Mechanics and its Applications, 2017, 494: 40-51.
12	Liu Q Y, Sun J, Tian Y, et al. Modeling and simulation of nonmotorized vehicles' dispersion at mixed flow intersections[J]. Journal of Advanced Transportation, 2019, 5: 1-19.
13	王迪. 基于CA模型的城市道路多路合流路段交通流特性研究[D]. 重庆: 重庆交通大学交通学院,2016.
	Wang Di. The traffic characteristics of multichannel Megre one road with cellular automata model[D]. Chongqing: School of Transportation, Chongqing Jiaotong University, 2016.
14	黄青霞, 贾斌, 强生杰, 等. 基于元胞自动机的高速公路施工区交通影响分析[J]. 长安大学学报: 自然科学版, 2015, 35(): 254-258.
	Huang Qing-xia, Jia Bin, Qiang Sheng-jie, et al. Analysis of traffic influence of freeway work zone based on cellular automata model[J]. Journal of Chang'an University(Natural Science Edition), 2015, 35(S1): 254-258.
15	田晟, 许凯, 马美娜. 基于元胞自动机的城市施工路段车辆变道行为研究[J]. 重庆交通大学学报: 自然科学版, 2019, 38(1): 113-118, 124.
	Tian Sheng, Xu Kai, Ma Mei-na. Lane changing behavior of vehicles in urban road work zone based on cellular automata[J]. Journal of Chongqing Jiaotong University(Natural Science), 2019, 38(1): 113-118.
16	邝先验, 陈自如. 设置非机动车等候区的信号交叉口交通流模型[J]. 交通运输系统工程与信息, 2019, 19(4): 179-186.
	Kuang Xian-yan, Chen Zi-ru. Traffic flow model of signalized intersection with non-motor vehicle waiting area[J]. Journal of Transportation Systems Engineering and Information Technology, 2019, 19(4): 179-186.
17	邝先验, 陈自如. 考虑礼让行人的交叉口机非混合交通流模型[J]. 广西师范大学学报: 自然科学版, 2019, 37(4): 1-15.
	Kuang Xian-yan, Chen Zi-ru. Mixed traffic flow model of signalized intersections involving pedestrian comity[J]. Journal of Guangxi Normal University(Natural Sicience Edition), 2019, 37(4): 1-15.
18	Kuang X Y, You L Q, Chen Z R, et al. Modelling and simulation for two-wheel vehicle flow based on cellular automaton[J]. Advances in Transportation Studies, 2016, 38:91-106.
19	Zhao H T, Yang S, Chen X X. Cellular automata model for urban road traffic flow considering pedestrian crossing street[J]. Physica A: Statistical Mechanics and its Applications, 2016, 462: 1301-1313.
20	Wardrop J G. Some theoretical aspects of road traffic reesarch[J]. Proceeding of the Institute of Civil Engineers, Part II, 1952, 1(36): 352-362.
21	李平. 基于博弈论的行人过街冲突模型研究[D]. 哈尔滨:哈尔滨工业大学深圳研究生院, 2011.
	Li Ping. Research on the conflict model of pedestrian crossing based on game theory[D]. Harbin: Shenzhen Graduate School of Harbin Institute of Technology, 2011.
22	刘丽娟, 杨澄雄. 基于博弈论的城市道路行人过街决策研究[J]. 公路与汽运, 2014, 29(1): 54-56.
	Liu Li-juan, Yang Cheng-xiong. Stuy of pedestrian Crossing in urban road based on game theory[J]. Highway & Automotive Applications, 2014, 29(1):54-56.
23	杨玉婷. 过街行人与机动车冲关的博弈模型[D]. 北京:北京理工大学机械与车辆学院, 2016.
	Yang Yu-ting. Game theory model of conflicts between pedestrians and vehicles at crosswalk[D]. Beijing: School of Mechanical Engineering and Vehicles, Beijing Institute of Technology, 2016.
24	李新波. 基于博弈论的路段行人过街决策研究[D]. 长春: 吉林大学通信工程学院, 2016.
	Li Xing-bo. Research on pedestrian crossing decision making of road section based on game theory[D]. Changchun: College of Communication Engineering, Jilin University, 2018.
25	马平. 基于博弈论的信号交叉口行人与机动车冲突分析研究[D]. 北京:中国人民公安大学治安与交通管理学院, 2018.
	Ma Ping. Reseach of confilct between pedestrians and vehicles at signalized intersection based on game theory[D]. Beijing: School of Public Security and Traffic Management, Chinese Public Security University, 2016.
26	徐良杰, 王炜. 信号交叉口行人过街时间模型[J]. 交通运输工程学报, 2005, 5(1): 111-115.
	Xu Liang-jie, Wang Wei. Model of pedestrians crossing time at signalized intersection[J]. Journal of Traffic and Transportation Engineering, 2005, 5(1): 111-115.
27	程国柱, 刘博通, 吴立新. 城市道路过街行人风险感知及决策损失研究[J]. 交通运输系统工程与信息, 2014, 14(3): 131-136.
	Cheng Guo-zhu, Liu Bo-tong, Wu Li-xin. Urban road crossing pedestrian risk perception and decision loss[J]. Journal of Transportation Systems Engineering and Information Technology, 2014, 14(3): 131-136.
28	高利平, 付春杰, 何会军, 等. 基于过街方式的信控路段行人-机动车冲突分析与延误模型[J]. 交通运输系统工程与信息, 2011, 11(): 14-21.
	Gao Li-ping, Fu Chun-jie, He Hui-jun, at et. Delay model of ped-veh system based on pedestrian crossing signalized roads[J]. Journal of Transportation Systems Engineering and Information Technology, 2011, 11(S1): 14-21.
29	齐薪玉. 城市道路车辆换道行为的博弈分析与仿真研究[D]. 北京:北京交通大学交通运输学院, 2019.
	Qi Xin-yu. Game analysis and simulation research on vehcile lane changing behavior on urban roads[D]. Beijing: School of Transportation, Beijing Jiaotong University, 2019.
30	刘博通. 驾驶员与过街行人非合作动态博弈模型研究[D]. 哈尔滨:哈尔滨工业大学交通科学与工程学院, 2013.
	Liu Bo-tong. Non-cooperative dynamic game model between drivers and crossing pedestrians[D]. Harbin: School of Transportation Science and Engineering, Harbin Institute of Technology, 2013.

Related Articles 14

[1]	Hong-fei JIA,Zi-han SHAO,Li-li YANG. Ride⁃sharing matching model and algorithm of online car⁃hailing under condition of uncertain destination [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(3): 564-571.
[2]	Shi-jun YANG,Yu-long PEI,Heng-yan PAN,Guo-zhu CHENG,Wen-hui ZHANG. Characteristics analysising and prediction of dwelling time of urban bus [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(6): 2031-2039.
[3]	Lei CHEN,Jiang⁃feng WANG,Yuan⁃li GU,Xue⁃dong YAN. Multi⁃source traffic data fusion algorithm based onmind evolutionary algorithm optimization [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(3): 705-713.
[4]	LI Shi-wu, XU Yi, SUN Wen-cai, WANG Lin-hong, GUO Meng-zhu, CHAI Meng. Pupil diameter based construction conflict self-feedback discrimination method [J]. 吉林大学学报(工学版), 2016, 46(2): 418-425.
[5]	GUO Wei-wei, QU Zhao-wei, WANG Dian-hai. Traffic conflict discrimination model [J]. 吉林大学学报(工学版), 2011, 41(01): 35-0040.
[6]	YU De-xin,YANG Zhao-sheng,GAO Peng. Constrained K-shortest paths algorithm within \|dynamic restricted searching area [J]. 吉林大学学报(工学版), 2009, 39(增刊2): 172-0176.
[7]	YANG Zhao-sheng, YU Yue, YANG Wei. Acquisition of travel time based on inductive coil detector and probe vehicle [J]. 吉林大学学报(工学版), 2009, 39(增刊2): 168-0171.
[8]	CHEN Jun,XIE Zhi-quan. Cycle traffic conflict model on urban pedestrian bicycle paths [J]. 吉林大学学报(工学版), 2009, 39(增刊2): 121-0125.
[9]	QU Zhao-wei, LI Zhi-hui, HU Hong-yu, GUO Wei-wei, WEI Wei. Traffic conflict automatic discrimination at non-signalized intersection based on video processing [J]. 吉林大学学报(工学版), 2009, 39(增刊2): 163-0167.
[10]	Lu Shoufeng, Yang Zhaosheng, Liu Ximin. Research on urban traffic system based on complexity theory [J]. 吉林大学学报(工学版), 2006, 36(增刊1): 153-0156.
[11]	Dai Hong,, Yang Zhaosheng, Xiao Pingping. Application of genetic algorithm in coordination optimization model between traffic flow guidance and traffic control [J]. 吉林大学学报(工学版), 2006, 36(增刊1): 157-0160.
[12]	Ji Changxu, Yang Nan, Hu Juanjuan,Chen Xin. Information processing in integration of urban traffic flow guidance system and traffic control system [J]. 吉林大学学报(工学版), 2006, 36(增刊1): 140-0143.
[13]	Yu Dexin，Yang Zhaosheng，Wang Yuan，Sun Jianping. Urban Road Traffic Control System and Its Coordinate Optimization Based on MultiAgent System [J]. 吉林大学学报(工学版), 2006, 36(01): 113-0118.
[14]	TANG Yangshan ，， LI Jiang ， TIAN Yugeng ， CHEN Xin. Chaos Forecast for Traffic Conflict Flow [J]. 吉林大学学报(工学版), 2005, 35(06): 646-0648.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

Comments

Recommended 0

No Suggested Reading articles found!

Dynamic game comity behavior at pedestrians′ crossing on unsignal-controlled roads based on cellular automata

RICH HTML

PDF (PC)