延误条件下综合多种策略的城轨列车运行调整优化

doi:10.13229/j.cnki.jdxbgxb20200467

吉林大学学报(工学版) ›› 2021, Vol. 51 ›› Issue (5): 1664-1672.doi: 10.13229/j.cnki.jdxbgxb20200467

• 交通运输工程·土木工程 • 上一篇

延误条件下综合多种策略的城轨列车运行调整优化

户佐安^1,²(),夏一鸣¹,蔡佳¹,薛锋^1,²()

^1.西南交通大学交通运输与物流学院，成都 611756
^2.西南交通大学综合交通大数据应用技术国家工程实验室，成都 611756

收稿日期:2020-06-28 出版日期:2021-09-01 发布日期:2021-09-16
通讯作者: 薛锋 E-mail:huzuoan@swjtu.edu.cn;xuefeng.7@swjtu.edu.cn
作者简介:户佐安（1979-），男，副教授，博士.研究方向：运输组织理论及系统优化.E-mail：huzuoan@swjtu.edu.cn
基金资助:
国家重点研发计划项目(2018YFB1601402);国家自然科学基金项目(61104175);四川省科技计划项目(2021YJ0067)

Optimization of urban rail transit operation adjustment based on multiple strategies under delay

Zuo-an HU^1,²(),Yi-ming XIA¹,Jia CAI¹,Feng XUE^1,²()

^1.School of Transportation and Logistics，Southwest Jiaotong University，Chengdu 611756，China
^2.National Engineering Laboratory for Integrated Transportation Big Data Application Technology，Southwest Jiaotong University，Chengdu 611756，China

Received:2020-06-28 Online:2021-09-01 Published:2021-09-16
Contact: Feng XUE E-mail:huzuoan@swjtu.edu.cn;xuefeng.7@swjtu.edu.cn

摘要/Abstract

摘要：

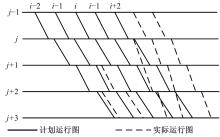
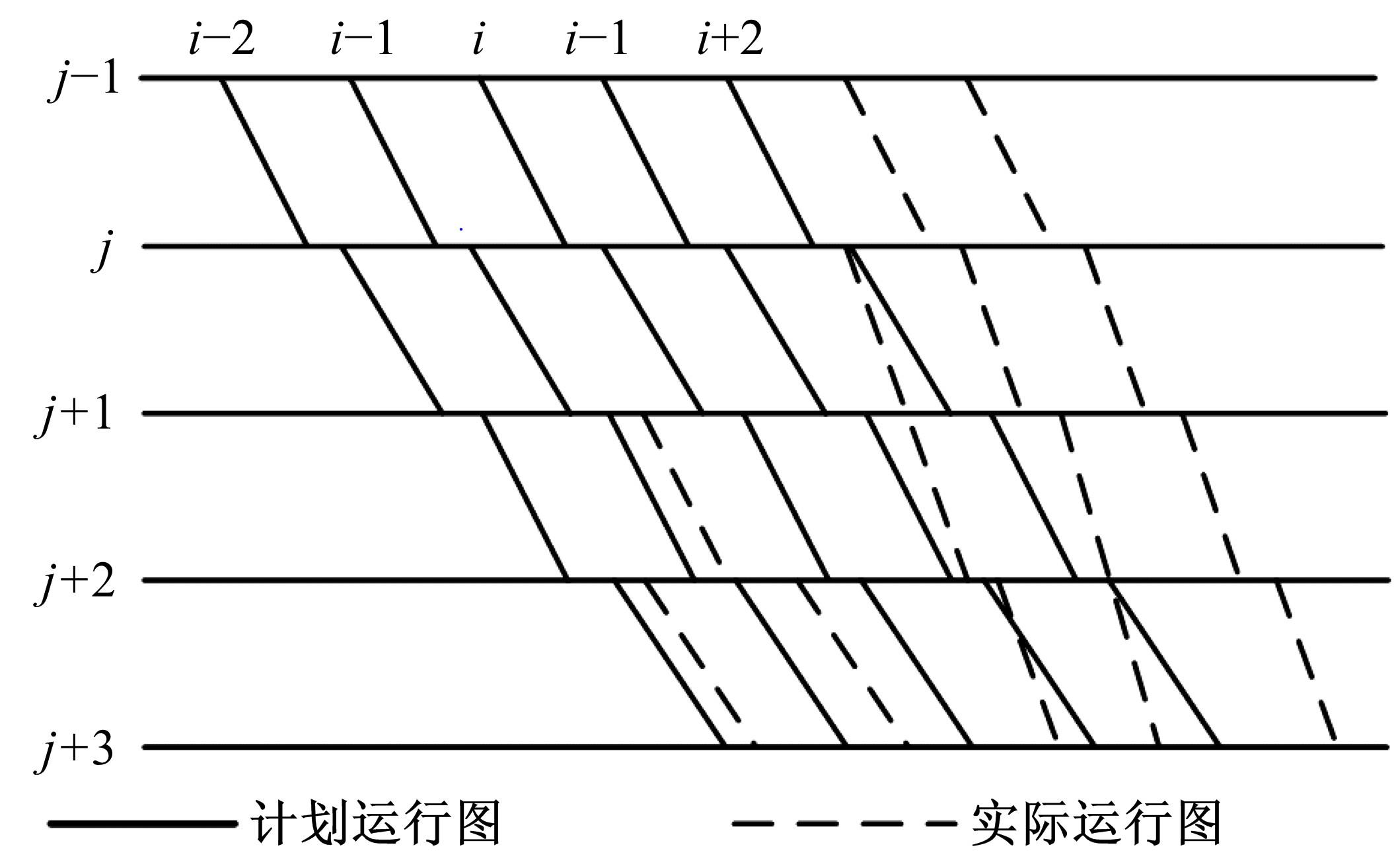
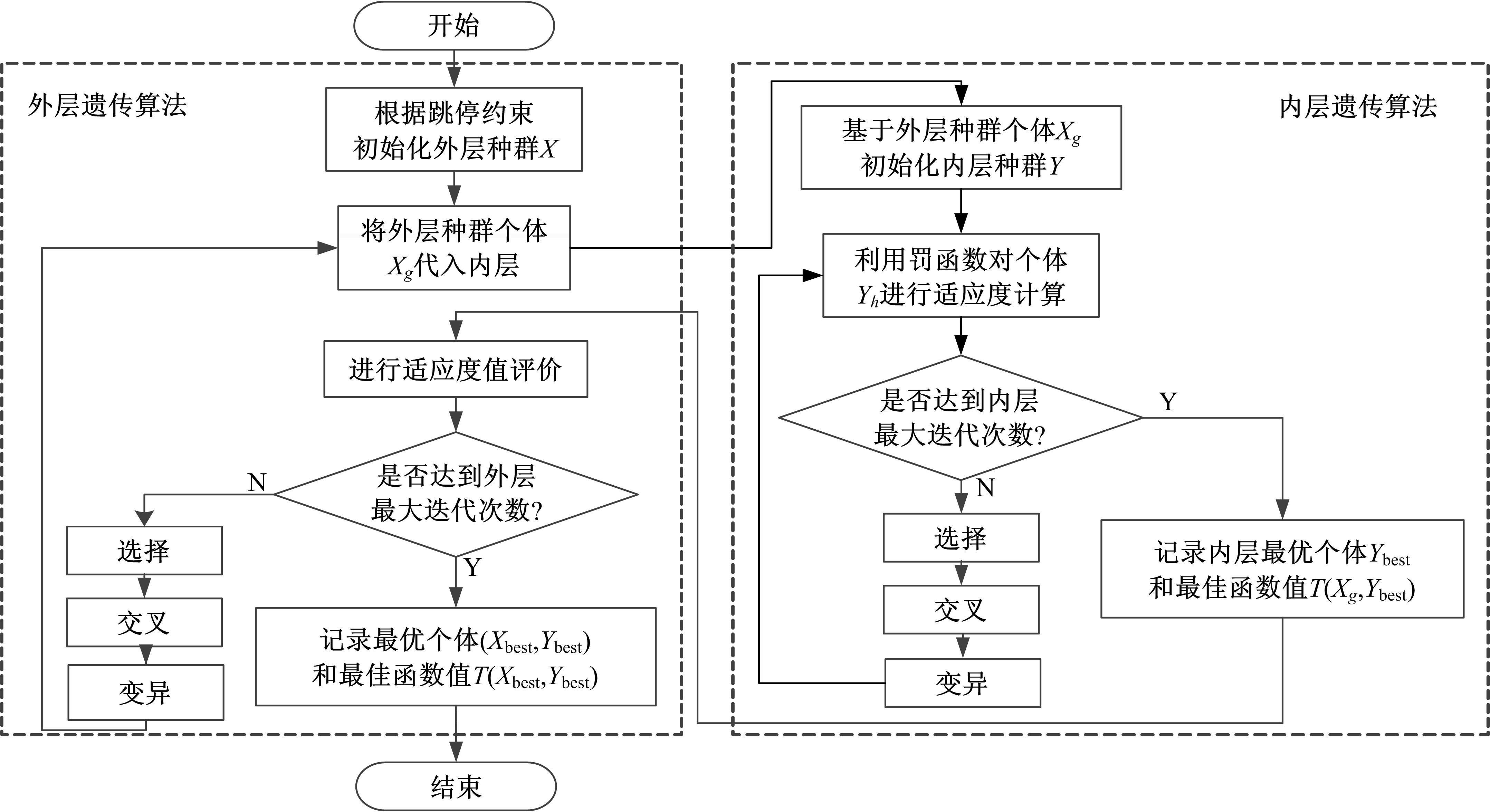
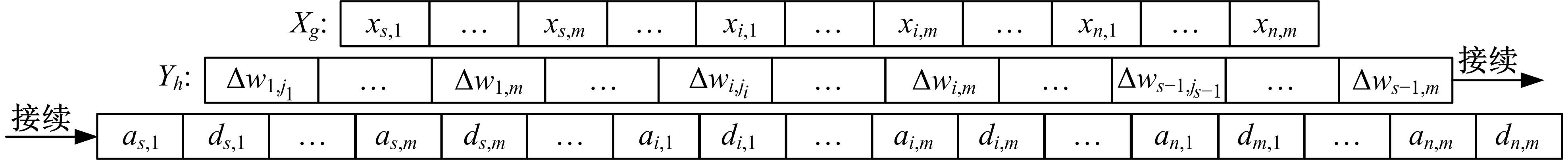
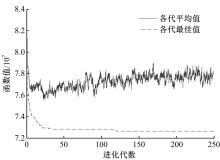
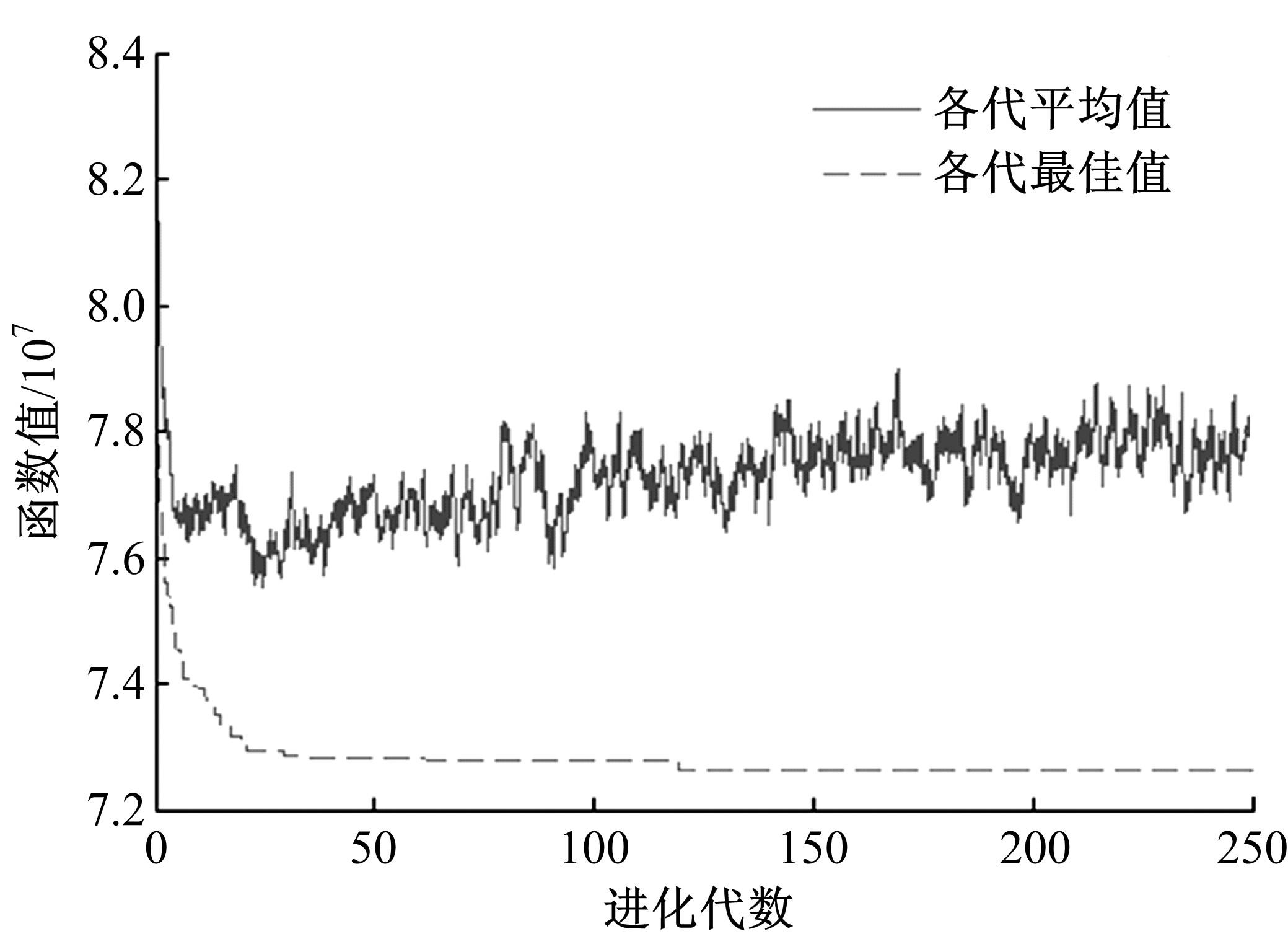
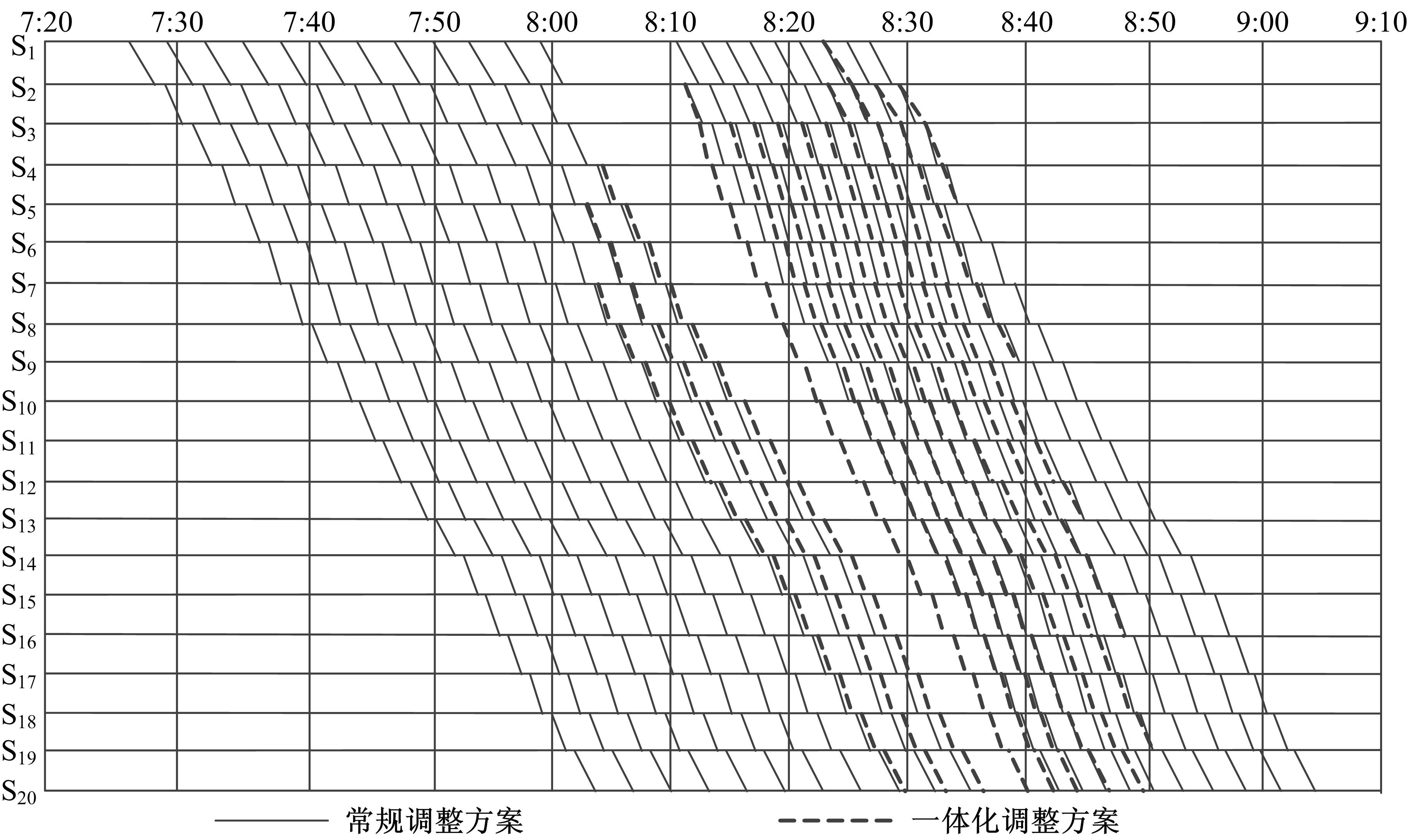
为制定在晚点恢复和缓解乘客滞留两方面更高效的列车运行调整方案，提出包括赶点、扣车、跳停策略及实行动态停站时间在内的列车运行一体化调整方法，结合列车实际运行情况设立运行和调整约束，以乘客总旅行时间最小为目标建立模型，并设计嵌套式遗传算法进行求解。案例结果表明：本文方法相比常规调整方法在各种情形下均能使受延误直接影响的列车提前完成晚点恢复，可有效缓解因延误造成的乘客候车时间过长或乘客滞留情况。

关键词: 交通运输规划与管理, 列车延误, 运行调整, 嵌套式遗传算法

Abstract:

In order to develop a more efficient train operation adjustment scheme in terms of recovering train delay and easing of passenger detention， an integrated train operation adjustment method was put forward， which includes a time-exceeding strategy， a train deduction strategy， a skip-stop strategy and a dynamic dwell time. Then， combined with the actual operating conditions of the train， the operation constraints and adjustment constraints were set up， and a model with the target of minimizing the total travel time was established. Finally， according to the characteristics of the model， a corresponding nested genetic algorithm was designed to solve the model. The results of the case show that compared with the conventional train operation adjustment method， the proposed integrated adjustment method can make the train which was directly affected by delay complete the delay recovery in advance in various kinds of situations， and can effectively alleviate the long waiting time or detention of passengers caused by the delay.

Key words: transportation planning and management, train delay, operation adjustment, nested genetic algorithm

中图分类号:

U231.92

户佐安,夏一鸣,蔡佳,薛锋. 延误条件下综合多种策略的城轨列车运行调整优化[J]. 吉林大学学报(工学版), 2021, 51(5): 1664-1672.

Zuo-an HU,Yi-ming XIA,Jia CAI,Feng XUE. Optimization of urban rail transit operation adjustment based on multiple strategies under delay[J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(5): 1664-1672.

图/表 10

图1

表1

参数定义"

参数	定义
$N$	受延误影响列车集合， $N = {1, ?, i, ?, n}$
$M$	线路车站集合， $M = {1, ?, k, ?, j, ?, m}$
$s, m 0$	初始延误列车及初始延误发生站点， $s ∈ N, m 0 ∈ M$
$t s, t g$	初始延误发生时刻及调度人员对初始延误持续时间做出预估所需时间
$N 1$	初始延误列车前行列车集合， $N 1 = {1,2, ?, s - 1}$
$N 2$	初始延误列车及其后行列车集合， $N 2 = {s, s + 1, ?, n}$
$a i, j, d i, j$	列车 $i$ 在 $j$ 站的实际到达及出发时刻
$A i, j, D i, j$	列车 $i$ 在 $j$ 站的计划到达及出发时刻
$R j, R j'$	列车在 $j$ 至 $j + 1$ 站之间的最小及计划区间运行时间
$I 0, I 1, I 2$	列车计划追踪间隔、最小发到间隔及最小追踪间隔
$w i, j, W i, j$	列车 $i$ 在 $j$ 站的实际和计划停站时间
$Δ w i, j$	列车 $i$ 在 $j$ 站的停站时间增加量
$P i, j$	列车 $i$ 在 $j$ 站出发时车内人数
$B i, j, E i, j$	列车 $i$ 在 $j$ 站的实际上车及下车人数
$B k O D, E k O D$	OD表中在 $k$ 站的总上车及下车人数
$E k, j O D$	OD表中在 $k$ 站上车并在 $j$ 站下车的人数
$q i, j$	列车 $i$ 在 $j$ 站面临的上车需求人数
$l i, j$	列车 $i$ 在 $j$ 站出发时，仍在 $j$ 站滞留的人数
$δ j$	$j$ 站的乘客到达速率，人/s
$θ j$	站站停模式下 $j$ 站乘客下车比例
$ξ i, j$	列车 $i$ 在 $j$ 站的下车比例调整因数
$C m a x, D O$	列车最大载客人数及一侧车门数
$τ 1, τ 2$	列车起动及停车附加时间
$D E$	最大总扣车时间
$λ 1, λ 2$	出行终到站被跳停的乘客选择在出行终到站的前一站及后一站下车的比例
$x i, j$	列车 $i$ 在 $j$ 站跳停决策的0-1变量， $x i, j = 1$ 为列车 $i$ 在 $j$ 站跳停， $x i, j = 0$ 则为未跳停

表1

图2

图3

图4

图5

图6

表2

表3

表4

三种情形下调整方案及优化效果"

延误情形	初始延误列车	影响列车集合	扣车方案	跳停方案	优化效果/%
延误情形	初始延误列车	影响列车集合	扣车方案	跳停方案	乘客总旅行时间	站台乘客候车时间	乘客乘车时间
高峰+一般延误	T12	$N = {1,2, ?, 21}$	T10在S5扣车11 s，T11在S4扣车19 s	均未跳停	42.2	33.5	-10.8
平峰+较长延误	T7	$N = {1,2, ?, 10}$	T5在S7扣车8 s，T6在S5扣车70 s	T7跳停S3及S6	66.2	33.2	21.1
平峰+一般延误	T7	$N = {1,2, ?, 8}$	均未扣车	均未跳停	57.4	26.3	1400

表4

参考文献 16

1	2019年度城市轨道交通运营情况[J]. 城市轨道交通, 2020(6): 37-41.
2	Jamili A, Aghaee M P. Robust stop-skipping patterns in urban railway operations under traffic alteration situation[J]. Transportation Research Part C, 2015, 61: 63-74.
3	刘祥喜. 突发大客流城市轨道交通列车停站方案优化研究[D]. 北京: 北京交通大学土木建筑与工程学院, 2012.
	Liu Xiang-xi. Study on stop-schedule plan optimization for urban rail transit under outburst mass passenger flow[D]. Beijing: School of Civil and Architectural Engineering, Beijing Jiaotong University, 2012.
4	Suh W, Chon K S, Rhee S M. Effect of skip-stop policy on a Korean subway system[J]. Transportation Research Record, 2002, 1793(1): 33-39.
5	Niu H M, Zhou X S, Gao R H. Train scheduling for minimizing passenger waiting time with time-dependent demand and skip-stop patterns: nonlinear integer programming models with linear constraints[J]. Transportation Research Part B, 2015, 76: 117-135.
6	郑锂, 宋瑞, 何世伟, 等. 城市轨道交通跨站停车方案优化模型及算法[J]. 铁道学报, 2009, 31(6): 1-8.
	Zheng Li, Song Rui, He Shi-wei, et al. Optimization model and algorithm of skip-stop strategy for urban rail transit[J]. Journal of the China Railway Society, 2009, 31(6): 1-8.
7	Cao Z C, Yuan Z Z, Li D W. Estimation method for a skip-stop operation strategy for urban rail transit in China[J]. Journal of Modern Transportation, 2014, 22(3): 174-182.
8	王婵婵, 陈菁菁. 城市轨道交通列车延误时多线换乘站跳停方案研究[J]. 城市轨道交通研究, 2014, 17(12): 69-72.
	Wang Chan-chan, Chen Jing-jing. On the skip-stop schemes at rail transit transfer station in case of train delay[J]. Urban Mass Transit, 2014, 17(12): 69-72.
9	何占元, 阴佳腾, 王明主. 基于线性规划模型的城轨列车运行图调整策略[J]. 太原科技大学学报, 2019(40): 296-301.
	He Zhan-yuan, Yin Jia-teng, Wang Ming-zhu. Urban rail train diagram adjustment strategy based on linear programming model[J]. Journal of Taiyuan University of Science and Technology, 2019(40): 296-301.
10	阴佳腾. 基于近似动态规划的城轨列车运行一体化调整方法研究[D]. 北京: 北京交通大学电子信息工程学院, 2018.
	Yin Jia-teng. Intergrated metro train rescheduling optimization based on approximate dynamic programming[D]. Beijing: School of Electronic Information Engineering, Beijing Jiaotong University, 2018.
11	乔珂, 赵鹏, 禹丹丹. 基于乘客等待时间的城市轨道交通列车运行调整模型[J]. 北京交通大学学报, 2014, 38(6): 32-38.
	Qiao Ke, Zhao Peng, Yu Dan-dan. Train regulation model of urban rail transit based on passenger waiting time[J]. Journal of Beijing Jiaotong University(对应英文??), 2014, 38(6): 32-38.
12	Xu X, Li K, Yang L. Rescheduling subway trains by a discrete event model considering service balance performance[J]. Applied Mathematical Modelling, 2016, 40: 1446-1466.
13	Yang X, Chen A, Ning B, et al. Bi-objective programming approach for solving the metro timetable optimization problem with dwell time uncertainty[J]. Transportation Research Part E Logistics & Transportation Review, 2017, 97: 22-37.
14	孙锋, 王殿海, 胡宏宇, 等. 基于出行时间分析的公交跳站运行方案优化[J]. 吉林大学学报: 工学版, 2012, 42(): 179-183.
	Sun Feng, Wang Dian-hai, Hu Hong-yu, et al. Optimizing skip-stop operation of bus transit based on analysis of travel time[J]. Journal of Jilin University (Engineering and Technology Edition), 2012, 42(Sup.1): 179-183.
15	赵航, 安实, 金广君, 等. 考虑车辆运输能力限制的公交换乘优化[J]. 吉林大学学报: 工学版, 2012, 42(3): 606-611.
	Zhao Hang, An Shi, Jin Guang-jun, et al. Optimization of transit transfer with vehicle capacity constrains[J]. Journal of Jilin University(Engineering and Technology Edition), 2012, 42(3): 606-611.
16	Vuchic V R. Urban Transit: Operation, Planning and Economics[M]. New Jersey: John Wiley & Sons, USA, 2004.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

调整方案	乘客总旅行时间/h			站台乘客候车时间/h			乘客乘车时间/h
调整方案	计算结果	与计划值之差	优化效果	计算结果	与计划值之差	优化效果	计算结果	与计划值之差	优化效果
计划运行方案	19 605	-	-	2 097	-	-	17 508	-	-
常规调整方案	20 997	1 392	-	4 305	2 208	-	16 692	-816	-
一体化调整方案	20 179	574	58.8%	3 815	1 718	22.2%	16 364	-1 144	40.2%

[1]	朱才华,孙晓黎,李岩. 站点分类下的城市公共自行车交通需求预测[J]. 吉林大学学报(工学版), 2021, 51(2): 531-540.
[2]	罗清玉,田万利,贾洪飞. 考虑通勤需求的电动汽车充电站选址与定容模型[J]. 吉林大学学报(工学版), 2019, 49(5): 1471-1477.
[3]	曹骞, 李君, 刘宇, 曲大为. 基于马尔科夫链的长春市乘用车行驶工况构建[J]. 吉林大学学报(工学版), 2018, 48(5): 1366-1373.
[4]	孙宝凤, 高坤, 申琇秀, 梁婷. 基于能力平衡和变覆盖半径的加油站网络扩充选址模型[J]. 吉林大学学报(工学版), 2018, 48(3): 704-711.
[5]	徐亮,程国柱. 基于车速离散度和经济车速的高速公路最低车速限制[J]. 吉林大学学报(工学版), 2010, 40(03): 661-0665.

延误条件下综合多种策略的城轨列车运行调整优化

Optimization of urban rail transit operation adjustment based on multiple strategies under delay

RICH HTML

PDF (PC)

摘要/Abstract

引用本文

使用本文

图/表 10

参考文献 16

相关文章 5

Metrics

本文评价

推荐阅读 0

列车	车站
列车	S4	S5	S6	S7	S8	S9	S10	S11	S12	S13	…	S19
T9	-	-	-	12	0	0	9	0	0	0	…	0
T10	-	8	5	0	16	0	10	7	8	0	…	0
T11	23	0	0	0	0	0	26	5	7	0	…	0