支管受轴向受拉工况下CHS-CFSHS T型节点应力集中系数计算公式

doi:10.13229/j.cnki.jdxbgxb20180737

摘要/Abstract

摘要：

基于具有可靠精度的圆管-方管混凝土T型节点应力集中系数（SCF）的有限元计算方法进行了160个有限元模型的SCF计算，对影响SCF的参数进行了分析，掌握了各参数对SCF 的影响规律，提出了T型焊接节点在支管受轴向受拉工况下SCF的计算公式，并且将公式计算值与有限元数据进行了比较。分析表明：在支管受轴向受拉工况下，支管上和主管上0°、60°、90°这6个位置的SCF基本包括SCF最大值可能发生的位置；T型节点SCF计算公式中应包含β的二次函数、2γ的二次函数和τ的幂函数，且三者的影响耦合；回归的SCF计算公式具有可靠的精度。

关键词: 结构工程, 疲劳性能, SCF计算公式, 参数分析, 圆管?方管混凝土T型节点, 应力集中系数

Abstract:

Based on the finite element method of SCF of CHS-CFSHS T-joints with reliable accuracy, 160 finite element models were established to calculate SCF of CHS-CFSHS T-joints when brace is under axial tension which are made up of circular hollow section (CHS) braces and concrete-filled square hollow section (CFSHS) chords. Parameters affecting SCF of the joint were analyzed. In addition, while grasping the influence law of each parameter on SCF, it proposed a calculation formula for SCF when brace is under axial tension, and made a comparison between the calculated value and finite element data. The results show that the six positions of 0°, 60° and 90° on the brace and the chord basically cover the position at which maximum SCF may occur when brace is under axial tension; and quadratic functions of β and 2γ as well as power function of τ should be included in the calculation formula of SCF. Moreover, the influences of the three parameters are coupled with each other, and the regressive calculation formulas of SCF have reliable accuracy.

Key words: structural engineering, fatigue properties, calculation formula of SCF, parameters analysis, CHS-CFSHS welded T-joints, stress concentration factors (SCF)

中图分类号:

TU392.3

杨德磊,童乐为. 支管受轴向受拉工况下CHS-CFSHS T型节点应力集中系数计算公式[J]. 吉林大学学报(工学版), 2019, 49(6): 1891-1899.

De-lei YANG,Le-wei TONG. Calculation formula of SCF for CHS⁃CFSHS welded T⁃joints with brace under axial tension[J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(6): 1891-1899.

图/表 14

图1

图2

图3

图4

图5

表1

图6

图7

图8

图9

图10

图11

图12

表2

参考文献

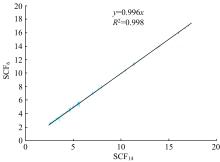
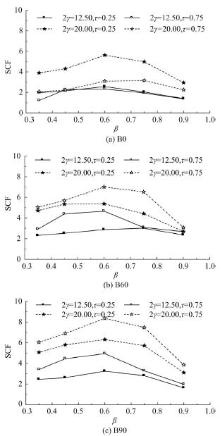
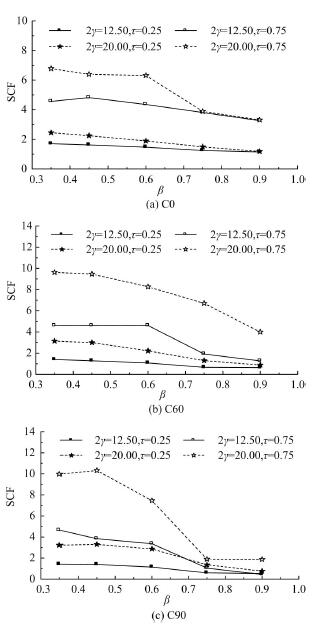
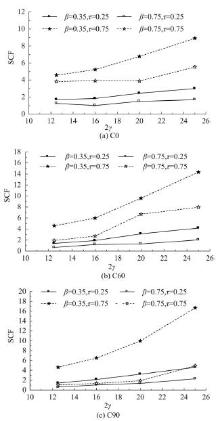
1	IIW：Recommendations for fatigue design of welding joints and components[S].International Institute of Welding, XIII-2151-07/XV-1254 -07, 2008.
2	Packer JA . Concrete-filled rectangular hollow section X and T connections[S]. Delft University Press (Netherlands), 1991382-91.
3	van Wingerde A M . The fatigue behaviour of T-and X-joints made of square hollow sections[D]. Netherlands： Dlft University of Technology， 1992： 1-180.
4	van Wingerde A M , Packer J A , Wardenier J . Criteria for the fatigue assessment of hollow structural section connections[J]. Journal of Constructional Steel Research, 1995, 35(1): 71-115.
5	Zhao Xiao-lin , Herion S , Packer J A , et al . Design Guide for Circular and Rectangular Hollow Section Welded Joints under Fatigue Loading[M]. Germany: TüV-Verlag, 2001.
6	曹珊珊, 雷俊卿 . 考虑区间不确定性的钢结构疲劳寿命分析[J]. 吉林大学学报:工学版, 2016, 46(3): 804-810.
6	Cao Shan-shan , Lei Jun-qing . Fatigue life prediction of steel structure considering interval uncertainty[J] Journal of Jilin University(Engineering and Technology Edition), 2016, 46(3): 804-810.
7	Sakai Y , Hosaka T , Isoe A , et al . Experiments on concrete filled and reinforced tubular K-joints of truss girder[J]. Journal of Constructional Steel Research, 2004, 60(3-5):683-699.
8	Tong Le-wei , Wang Ke . Experimental study on stress concentration factors of concrete-filled circular hollow section T-joints under axial loading[C]∥China：Pacific Structural Steel Conference2007, in New Zealand, Vol.2.
9	Tong Le-wei , Sun Chuan-qi , Chen Yi-yi , et al . Experimental comparison in hot spot stress between CFCHS and CHS K-joints with gap[C]∥Proceedings of 12th International Symposium on Tubular Structures, Shanghai, China, 2008: 389-395.
10	Gu M , Tong L W , Zhao X L , et al . Stress intensity factors of surface cracks in welded T-joints between CHS brace and concrete-filled CHS chord[C]∥Tubular Structures XII: Proceedings of Tubular Structures XII, Shanghai, China, 2008: 359.
11	Chen J , Chen J , Jin W L . Experiment investigation of stress concentration factor of concrete-filled tubular T joints[J]. Journal of Constructional Steel Research, 2010, 66(12): 1510-1515.
12	Han Lin-hai , Li Wei , Bjorhovde R . Developments and advanced applications of concrete-filled steel tubular (CFST) structures: members[J]. Journal of Constructional Steel Research, 2014, 100: 211-228.
13	Kim I G , Chung C H , Shim C S , et al . Stress concentration factors of N-joints of concrete-filled tubes subjected to axial loads[J]. International Journal of Steel Structures, 2014, 14(1): 1-11.
14	Qian Xu-dong , Jitpairod K , Marshall P , et al . Fatigue and residual strength of concrete-filled tubular X-joints with full capacity welds[J]. Journal of Constructional Steel Research, 2014, 100: 21-35.
15	Xu Fei , Chen Ju , Jin Wei-liang . Experimental investigation of SCF distribution for thin-walled concrete-filled CHS joints under axial tension loading[J]. Thin-Walled Structures, 2015, 93: 149-157.
16	SIMULIA . Abaqus 6.12.Analysis User’s Manual[Z]. USA, Dassault Systems Simulia Corp., 2013.
17	Machiri F R , Zhao X L , Grundy P . Stress concentration factors and fatigue behaviour of welded thin-walled CHS-SHS T-joints under in-plane bending[J]. Engineering Structures, 2004, 26(13): 1861-1875.
18	童乐为, 杨德磊, Zhao X L .圆管-方管混凝土T型节点疲劳性能试验研究[J]. 振动与冲击, 2013(2): 99-105.
18	Tong Le-wei , Yang De-lei , Zhao X L . Experimental study on fatigue behaviour of welded CHS-to-Concrete filled SHS T-joints [J]. Journal of Vibration and Shock, 2013(2): 99-105.
19	王柯 . 圆管-圆管混凝土T型焊接节点热点应力和疲劳强度研究[D]. 上海：同济大学土木工程学院, 2008.
19	Wang Ke . Fatigue behaviour of welded CHS-to-Concrete filled CHS T-joints[D]. Shanghai: School of Civil Engineering, Tongji University, 2008.
20	Tong Le-wei , Zheng Hong-Zhi , Mashiri F R , et al . Stress-concentration factors in circular hollow section and square hollow section T-connections: experiments, finite-element analysis, and formulas[J]. Journal of Structural Engineering, 2013, 139(11): 66-81.
21	Yang De-lei , Tong Le-wei . Research on hot spot stress calculation method of CHS-CFSHS joints [J]. Jordan Journal of Civil Engineering, 2018, 12(2): 323-332.
22	中国工程建设标准化协会 .CECS280. 钢管结构技术规程[S]. 北京：中国建筑工业出版社, 2010.
23	中国工程建设标准化协会 .GB 50017—2003. 钢结构设计规范[S]. 北京：中国建筑工业出版社, 2003.
24	中华人民共和国建设部 .JGJ 81—2002.建筑钢结构焊接技术规程[S]. 北京：中国建筑工业出版社, 2002.
25	van Wingerde A M , Packer J A ， Wardenier J . Criteria for the fatigue assessment of hollow structural section connections[J]. Journal of Constructional Steel Research, 1995, 35(1): 71-115.
26	Yang Dei-lei , Tong Le-wei , Zhao Xiao-lin . Experimental study on SNCF of welded CHS-CFSHS T-Joints under Axial loading[J]. Advanced Materials Research, 2011, 163-167: 127-131.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

位置	支管/%	主管/%	位置	支管/%	主管/%
0°	0.00	11.25	60°	15.00	17.50
15°	0.00	5.00	75°	2.50	3.75
30°	0.00	5.00	90°	75.75	47.50
45°	6.25	10.00

项目		不区分焊缝类型			角焊缝			全熔透焊缝
项目		B0	B60	B90	C0	C60	C90	C0	C60	C90
公式值/ 有限元值	均值	1.116	1.046	1.039	1.016	1.084	0.988	1.015	0.971	0.949
	方差	0.033	0.023	0.019	0.023	0.064	0.057	0.043	0.071	0.066
	离散度	0.030	0.022	0.018	0.023	0.059	0.057	0.042	0.073	0.070
比值范围	<0.8	2.50%	0.00%	2.50%	5.00%	5.00%	11.25%	10.00%	15.00%	17.50%
	0.8~0.9	6.25%	11.25%	5.00%	8.75%	11.25%	17.50%	11.25%	13.75%	11.25%
	0.9~1.0	21.25%	33.75%	33.75%	36.25%	22.50%	36.25%	23.75%	28.75%	26.25%
	1.0~1.1	35.00%	31.25%	37.50%	33.75%	32.50%	15.00%	31.25%	22.50%	26.25%
	1.1~1.2	18.75%	13.75%	13.75%	12.50%	13.75%	8.75%	15.00%	11.25%	10.00%
	>1.2	16.25%	10.00%	7.50%	3.75%	12.50%	11.25%	8.75%	8.75%	8.75%

[1]	石舟,寇淑清. 36MnVS4裂解连杆性能分析及轻量化设计[J]. 吉林大学学报(工学版), 2019, 49(6): 1992-2001.
[2]	罗开玉,邢月华,柴卿锋,吴世凯,尹叶芳,鲁金忠. 激光冲击强化对2Cr13不锈钢腐蚀疲劳性能的影响[J]. 吉林大学学报(工学版), 2019, 49(3): 850-858.
[3]	戴岩, 聂少锋, 周天华. 带环梁的方钢管约束钢骨混凝土柱-钢梁节点滞回性能有限元分析[J]. 吉林大学学报(工学版), 2018, 48(5): 1426-1435.
[4]	杨昕卉, 薛伟, 郭楠. 钢板增强胶合木梁的抗弯性能[J]. 吉林大学学报(工学版), 2017, 47(2): 468-477.
[5]	王少杰, 徐赵东, 李舒, 王凯洋，Dyke Shirley J. 基于应变监测的连续梁支承差异沉降识别[J]. 吉林大学学报(工学版), 2016, 46(4): 1090-1096.
[6]	宿晓萍，王清. 复合盐浸-冻融-干湿多因素作用下的混凝土腐蚀破坏[J]. 吉林大学学报(工学版), 2015, 45(1): 112-120.
[7]	于燕, 张小盟, 刘云旭. TRIP汽车钢板的高周疲劳性能[J]. 吉林大学学报(工学版), 2014, 44(5): 1371-1374.
[8]	张阔, 谭庆昌, 王顺, 王聪慧, 王春艳. 表面粗糙度幅值对点接触混合润滑的影响[J]. 吉林大学学报(工学版), 2012, 42(02): 377-381.
[9]	姜浩, 郭学东. 基于地震激励的混凝土桥梁模态参数识别[J]. 吉林大学学报(工学版), 2011, 41(增刊2): 185-188.
[10]	陈俊, 黄晓明. 基于离散元法的沥青混合料虚拟疲劳试验方法[J]. 吉林大学学报(工学版), 2010, 40(02): 435-0440.