吉林大学学报(工学版) ›› 2021, Vol. 51 ›› Issue (2): 638-649.doi: 10.13229/j.cnki.jdxbgxb20191171

• 交通运输工程·土木工程 • 上一篇    

多次冲击下砂岩粘弹性损伤本构关系

杜瑞锋1,2(),裴向军1(),贾俊1,3,张晓超1,陈俊宇1,张国华1   

  1. 1.成都理工大学 地质灾害防治与地质环境保护国家重点实验室，成都 610059
    2.内蒙古建筑职业技术学院 建筑工程与测绘学院，呼和浩特 010070
    3.国土资源部黄土地质灾害重点实验室/中国地质调查局西安地质调查中心，西安 710054
  • 收稿日期:2019-12-23 出版日期:2021-03-01 发布日期:2021-02-09
  • 通讯作者: 裴向军 E-mail:dddrrrfeng@126.com;peixj0119@tom.com
  • 作者简介:杜瑞锋（1978-），男，博士研究生.研究方向：地质灾害防治与治理.E-mail:dddrrrfeng@126.com
  • 基金资助:
    国家重点研发计划项目(2017YFC1501002);青年科学基金项目(41702335);川藏铁路重大工程风险识别与对策研究项目(2019YFG0460)

Viscoelastic damage constitutive relation of sandstone under multiple impact load

Rui-feng DU1,2(),Xiang-jun PEI1(),Jun JIA1,3,Xiao-chao ZHANG1,Jun-yu CHEN1,Guo-hua ZHANG1   

  1. 1.State Key Laboratory of Geohazard Prevention and Geoenvironment Protection，Chengdu University of Technology，Chengdu 610059，China
    2.Civil Engineering and Surveying & Mapping Department，Inner Mongolia Technical College of Construction，Hohhot 010070，China
    3.Key Laboratory for Geo-hazards in Loess Area，MLR/Xi'an Center of Geological Survey，China Geological Survey，Xi'an 710054，China
  • Received:2019-12-23 Online:2021-03-01 Published:2021-02-09
  • Contact: Xiang-jun PEI E-mail:dddrrrfeng@126.com;peixj0119@tom.com

RICH HTML

 4   

PDF (PC)

272

摘要：

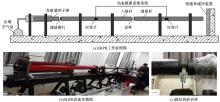
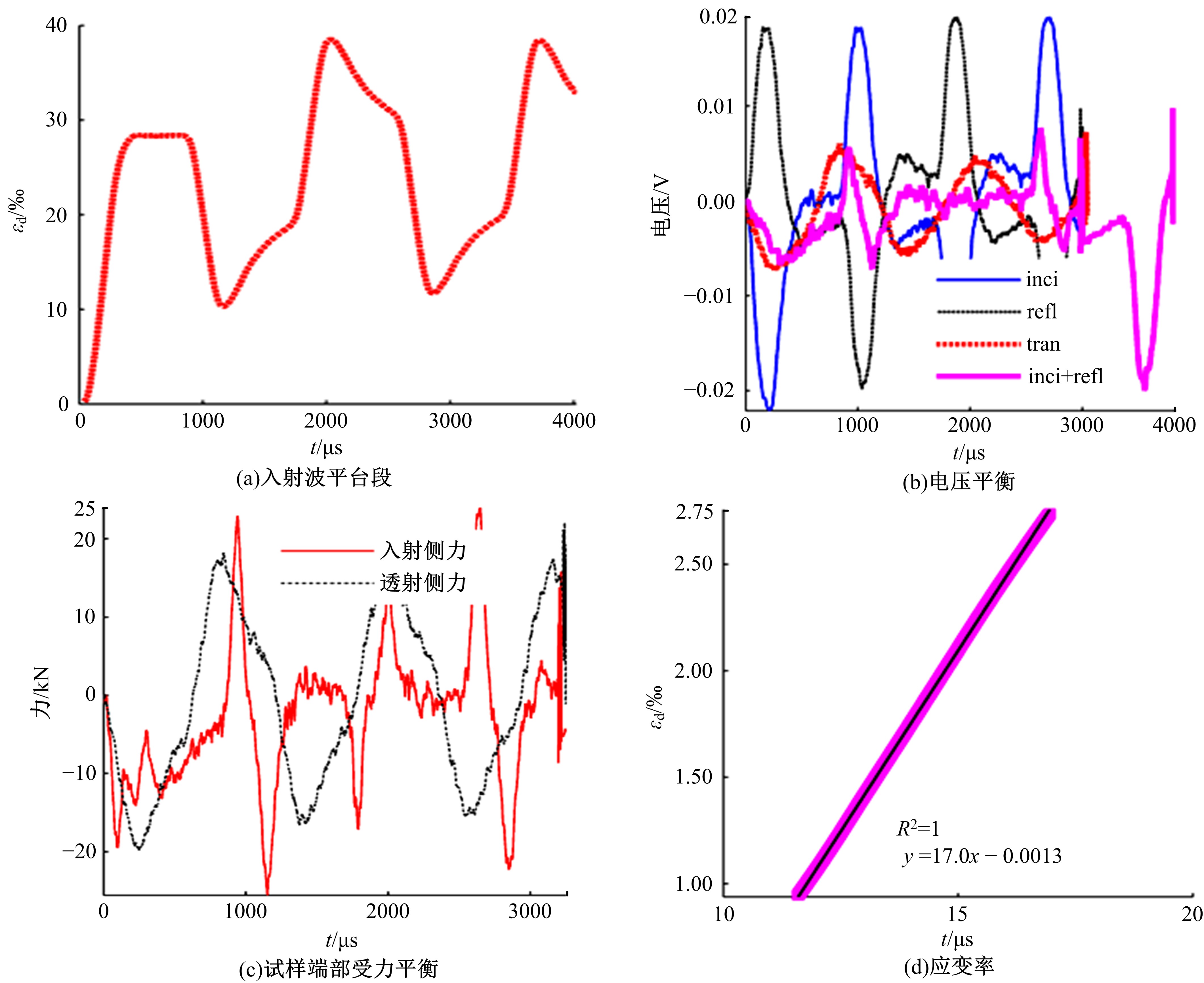
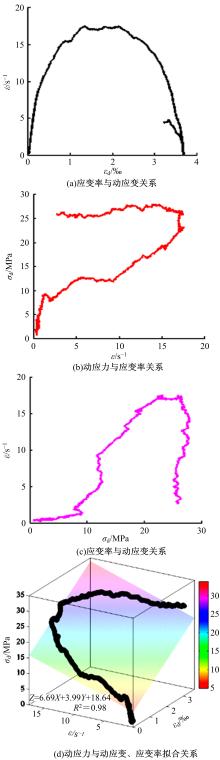
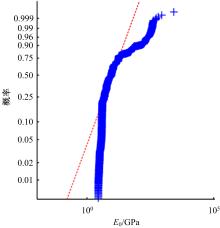
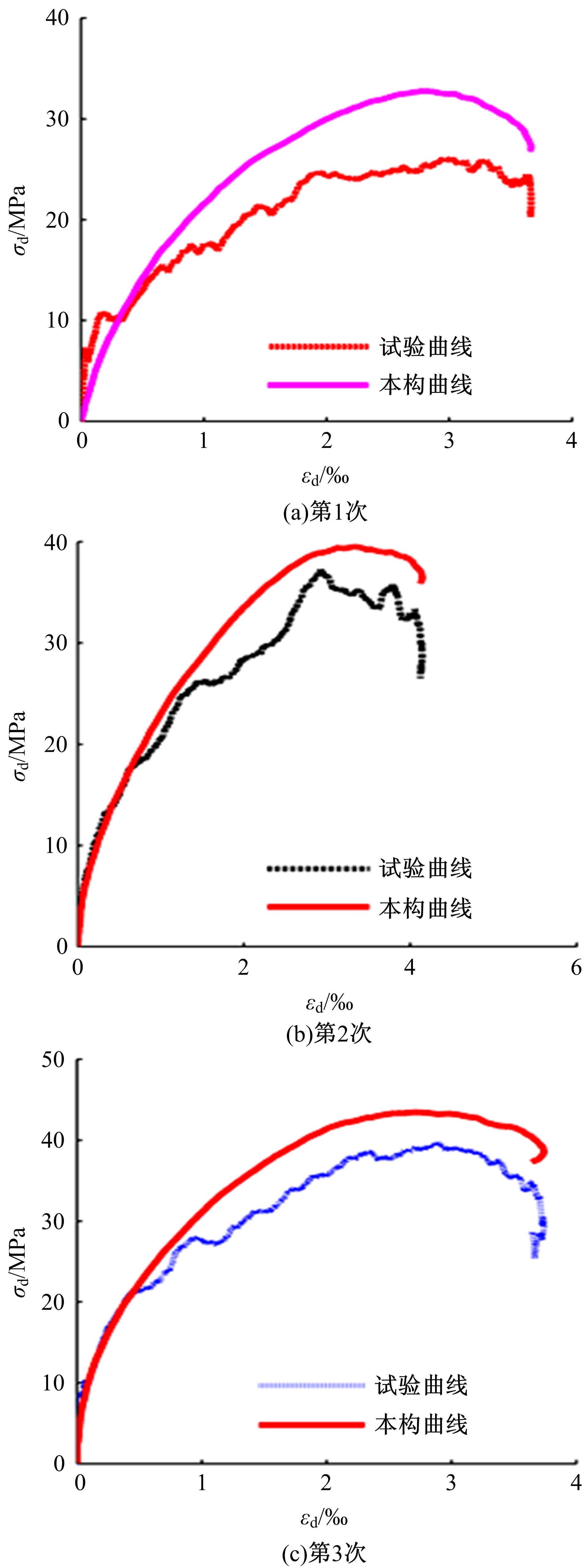
为研究砂岩的粘弹性损伤特性，开展了多次冲击压缩下的霍普金森杆试验。通过砂岩动应力应变曲线分析了中应变率范围内两种动变形模量的定义方法，表明采用等效动变形模量能反映砂岩在动态冲击压缩作用下的损伤变化特性。从微观角度出发，砂岩的统计损伤变量可由破坏的微元体数量占全部微元体数量的比例定义；从宏观角度出发，这种统计损伤变量也可由微元体破坏前后的动变形模量定义，并验证了动变形模量服从Weibull统计分布。微元体受力模型由粘性体和损伤体并联而成。粘性体的力学机制由砂岩动应力与动应变、应变率之间高度线性相关的拟合关系得到合理的解释；损伤体的应力应变关系由Drucker-Prager强度准则和Lemaitre应变等效原理基础上推导而来。通过对建立的粘弹性本构关系曲线与试验曲线进行验证，两者之间的变化趋势一致，具有较好的代表性，表明建立的粘弹性损伤本构关系是合理的；两者之间的偏差可通过增加砂岩试样数量、优化试验方案以及应用概率统计等途径解决；在获得一定概率保证率的本构关系参数后方可将粘弹性损伤本构关系用于分析和评价煤矿采动区岩质边坡的稳定性，以及为相关的地质工程安全评价提供必要的理论分析基础。

关键词: 地质工程, 砂岩, 应变率, 动变形模量, 粘弹性, 损伤本构关系

Abstract:

In order to study the viscoelastic damage characteristics of sandstone， the Hopkinson test under multiple impact compression was carried out. Based on the dynamic stress-strain curve of sandstone， two definitions of dynamic deformation modulus in the range of middle strain rate are analyzed. It is shown that the equivalent dynamic deformation modulus can reflect the damage characteristics of sandstone under dynamic impact compression. Microscopically， the statistical damage variable of sandstone can be defined by the ratio of the number of damaged microelements to the total number of microelements； and macroscopically it can also be defined by the dynamic deformation modulus before and after the microelement failure. It is verified that the dynamic deformation modulus obeys the Weibull statistical distribution. The force model of the microelement is composed of a viscous body and a damaged body in parallel. The mechanical mechanism of the viscoelastic body is reasonably explained by the highly linear correlation between the dynamic stress， the dynamic strain and the strain rate of sandstone. The stress-strain relation of the damaged body is derived based on the Drucker-Prager strength criterion and the strain equivalent principle proposed by Lemaitre. Through the verification of the introduced viscoelastic constitutive relation curve and the test curve of sandstone， the trend of change between them is consistent， which has good representativeness， indicating that the established viscoelastic damage constitutive relation is reasonable. The deviation between them can be solved by increasing the number of sandstone samples， optimizing the test scheme and applying probability statistics. After obtaining the viscoelastic constitutive relationship parameters with a certain guarantee rate of probability， the viscoelastic damage constitutive relation can be used to analyze and evaluate the stability of the rock slope in the coal mining area， and provide the necessary theoretical analysis basis for the related geological engineering evaluation of safety.

Key words: geological engineering, sandstone, strain rate, dynamic deformation modulus, viscoelasticity, damage constitutive relation

中图分类号: 

  • TB122

图1

砂岩岩样准备"

图2

SHPB试验原理图、实物图和就位的砂岩样"

图3

砂岩SHPB试验相关图件"

图4

多次冲击压缩下砂岩动应力应变曲线"

图5

等效变形模量示意图"

图6

两种动变形模量定义的对比"

图7

砂岩S-2动变形模量变化规律"

图8

微元体示意图"

图9

砂岩S-1的动应力、动应变与应变率之间关系"

图10

砂岩动变形模量的Weibull分布概率"

图11

砂岩S-1试样本构关系曲线与试验曲线对比"

图12

砂岩S-2试样本构关系曲线与试验曲线对比"

图13

砂岩粘性系数变化对本构曲线的影响"

1 Zhang H, Wang L, Bai L Y, et al. Research on the impact response and model of hybrid basalt-macro synthetic polypropylene fiber reinforced concrete[J]. Construction and Building Materials, 2019, 204(20): 303-316.
2 王礼立, 朱兆祥. 应力波基础[M]. 北京: 国防工业出版社, 2005.
3 Zhang Q B, Zhao J. Determination of mechanical properties and full-field strain measurements of rock material under dynamic loads[J]. International Journal of Rock Mechanics & Mining Sciences, 2013, 60: 423-439.
4 Sunita M, Asce S M, Tanusree C, et al. Determination of high-strain-rate stress-strain response of granite for blast analysis of tunnels[J]. Journal of Engineering Mechanics, 2019, 145(8): 04019057.
5 Fakhimi A, Azhari P, Kimberley J. Physical and numerical evaluation of rock strength in split hopkinson pressure bar testing[J]. Computers and Geotechnics, 2018, 102: 1-11.
6 Zhou Z L, Zhao Y, Jiang Y H, et al. Dynamic behavior of rock during its post failure stage in SHPB test[J]. Transaction of Nonferrous Metals Society of China, 2017, 27(1): 184-196.
7 陈俊宇, 裴向军, 杜瑞锋, 等. 冲击载荷作用下砂岩的动力学特性及能耗规律[J]. 科学技术与工程, 2019, 19(31): 304-310.
Chen Jun-yu, Pei Xiang-jun, Du Rui-feng, et al. Dynamic characteristic and energy consumption of sandstone under impact loading[J]. Science Technology and Engineering, 2019, 19(31): 304-310.
8 梁宁慧, 缪庆旭, 刘新荣, 等. 聚丙烯纤维增强混凝土断裂韧度及软化本构曲线确定[J]. 吉林大学学报: 工学版, 2019, 49(4): 1144-1152.
Liang Ning-hui, Miao Qing-xu, Liu Xin-rong, et al. Determination of fracture toughness and softening traction-separation law of poly propylene fiber reinforced concrete[J]. Journal of Jilin University (Engineering and Technology Edition), 2019, 49(4): 1144-1152.
9 王子文, 郭学东, 郭威, 等. 疏水性纳米白炭黑改性沥青及沥青混合料的粘弹特性[J]. 吉林大学学报: 工学版, 2020, 50(5): 1709-1717.
Wang Zi-wen, Guo Xue-dong, Guo Wei, et al. Research on viscoelasticity of hydrophobic nano-silica modified asphalt and asphalt mixture[J]. Journal of Jilin University (Engineering and Technology Edition), 2020, 50(5): 1709-1717.
10 郭辉. 典型粘弹性材料力学特征及率温耦合本构关系[D]. 西安: 西北工业大学航空学院, 2018.
Guo Hui. Mechanical characteristics and constitutive relation of strain rate and temperature dependence of typical viscoelastic materials[D]. Xi'an: School of Aeronautics, Northwestern Polytechnical University, 2018.
11 王恩元, 孔祥国, 何学秋, 等. 冲击荷载下三轴煤体动力学分析及损伤本构方程[J]. 煤炭学报, 2019, 44(7): 2049-2056.
Wang En-yuan, Kong Xiang-guo, He Xue-qiu, et al. Dynamic analysis and damage constitute equation of triaxial coal mass under impact load[J]. Journal of China Coal Society, 2019, 44(7): 2049-2056.
12 Kargar A R. An analytical solution for circular tunnels excavated in rock masses exhibiting viscous elastic-plastic behavior[J]. International Journal of Rock Mechanics and Mining Sciences, 2019, 124: 104128.
13 朱晶晶. 循环冲击荷载下岩石力学特性与损伤模型试验研究[D]. 长沙: 中南大学资源与安全工程学院, 2012.
Zhu Jing-jing. Experimental study of rock mechanical properties and damage model under cyclical dynamic loads[D]. Changsha: School of Resources and Safety Engineering, Central South University, 2012.
14 凌天龙, 刘殿书, 梁书锋, 等. 花岗岩损伤型黏弹性动态本构模型研究[J]. 矿业科学学报, 2019, 4(5): 403-409.
Ling Tian-long, Liu Dian-shu, Liang Shu-feng, et al. Research on damage viscoelastic dynamic constitutive model of granite[J]. Journal of Mining Science and Technology, 2019, 4(5): 403-409.
15 蒋邦友, 谭云亮, 王连国, 等. 基于Mogi-Coulomb准则的弹塑性损伤本构模型及其数值实现[J]. 中国矿业大学学报, 2019, 48(4): 784-792.
Jiang Bang-you, Tan Yun-liang, Wang Lian-guo, et al. Development and numerical implementation of elastoplastic damage constitutive model for rock based on Mogi-Coulomb criterion[J]. Journal of China University of Mining & Technology, 2019, 48(4): 784-792.
16 李地元, 孙小磊, 周子龙, 等. 多次冲击荷载作用下花岗岩动态累计损伤特性[J]. 实验力学, 2016, 31(6): 827-835.
Li Di-yuan, Sun Xiao-lei, Zhou Zi-long, et al. On the dynamic accumulated damage characteristic of granite subjected to impact load action[J]. Journal of Experimental Mechanics, 2016, 31(6): 827-835.
17 Shan R L, Song Y W, Song L W, et al. Dynamic property tests of frozen red sandstone using a split hopkinson pressure bar[J]. Earthquake Engineering and Engineering Vibration, 2019, 18(3): 511-519.
18 Fu T T, Zhu Z W, Zhang D, et al. Research on damage viscoelastic dynamic constitutive model of frozen soil[J]. Cold Regions Science and Technology, 2019, 160: 209-221.
19 李天涛. 基于能量耗散的强震岩体震裂损伤特性及其孕灾机理研究[D]. 成都: 成都理工大学环境与土木工程学院, 2017.
Li Tian-tao. Seismic damage characteristics of rock based on energy dissipation and disaster-pregnant mechanism of strong earthquake[D]. Chengdu: College of Environment and Civil Engineering, Chengdu University of Technology, 2017.
20 杜瑞锋, 裴向军, 张晓超, 等. 泥质砂岩动变形模量的演化规律试验研究[J]. 成都理工大学学报: 自然科学版, 2020, 47(1): 92-101.
Du Rui-feng, Pei Xiang-jun, Zhang Xiao-chao, et al. Experimental study on evolution of dynamic deformation modulus of argillaceous sandstone[J]. Journal of Chengdu University of Technology (Science & Technology Edition), 2020, 47(1): 92-101.
21 陈松, 乔春生, 叶青, 等. 基于摩尔-库伦准则的断续节理岩体复合损伤本构模型[J]. 岩土力学, 2018, 39(10): 3612-3622.
Chen Song, Qiao Chun-sheng, Ye Qing, et al. Composite damage constitutive model of rock mass with intermittent joints based on Mohr-Coulomb criterion[J]. Rock and Soil Mechanics, 2018, 39(10): 3612-3622.
22 曹文贵, 张超, 贺敏, 等. 基于微观力学特征的脆性岩石变形过程模拟[J]. 岩土力学, 2016, 37(10): 2753-2760.
Cao Wen-gui, Zhang Chao, He Min, et al. Deformation simulation of brittle rock based on micromechanical properties[J]. Soil and Rock Mechanics, 2016, 37(10): 2753-2760.
23 潘青松, 彭刚, 胡伟华, 等. Weibull统计理论的参数对混凝土全曲线模型的影响[J]. 长江科学院院报, 2015, 32(4): 120-124.
Pan Qing-song, Peng Gang, Hu Wei-hua, et al. Application of Weibull statistical theory in concrete's parameter curve model[J]. Journal of Yangtze River Scientific Research Institute, 2015, 32(4): 120-124.
[1] 王子文,郭学东,郭威,常孟元,戴文亭. 疏水性纳米白炭黑改性沥青及混合料的粘弹特性[J]. 吉林大学学报(工学版), 2020, 50(5): 1709-1717.
[2] 叶辉,朱艳荣,蒲永锋. 纤维增强复合材料应变率效应的数值仿真[J]. 吉林大学学报(工学版), 2019, 49(5): 1622-1629.
[3] 赵长福, 高中礼, 马中胜, 马洪顺. 股骨上端松质骨压缩粘弹性实验研究[J]. 吉林大学学报(工学版), 2002, (2): 87-90.
[4] 李洪, 王玉臣, 马洪顺. 腹主动脉粘弹性实验研究[J]. 吉林大学学报(工学版), 2001, (4): 38-41.
[5] 董心, 贺家宁, 罗民, 马洪顺. C3-C7段颈椎粘弹性实验研究[J]. 吉林大学学报(工学版), 2001, (2): 35-39.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
版权所有 © 《吉林大学学报(工学版)》编辑部
地址：长春市人民大街5988号　电话：+86-431-85095297　传真：+86-431-85094074　E-mail: xbgxb@jlu.edu.cn
本系统由北京玛格泰克科技发展有限公司设计开发