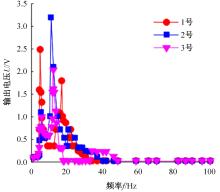
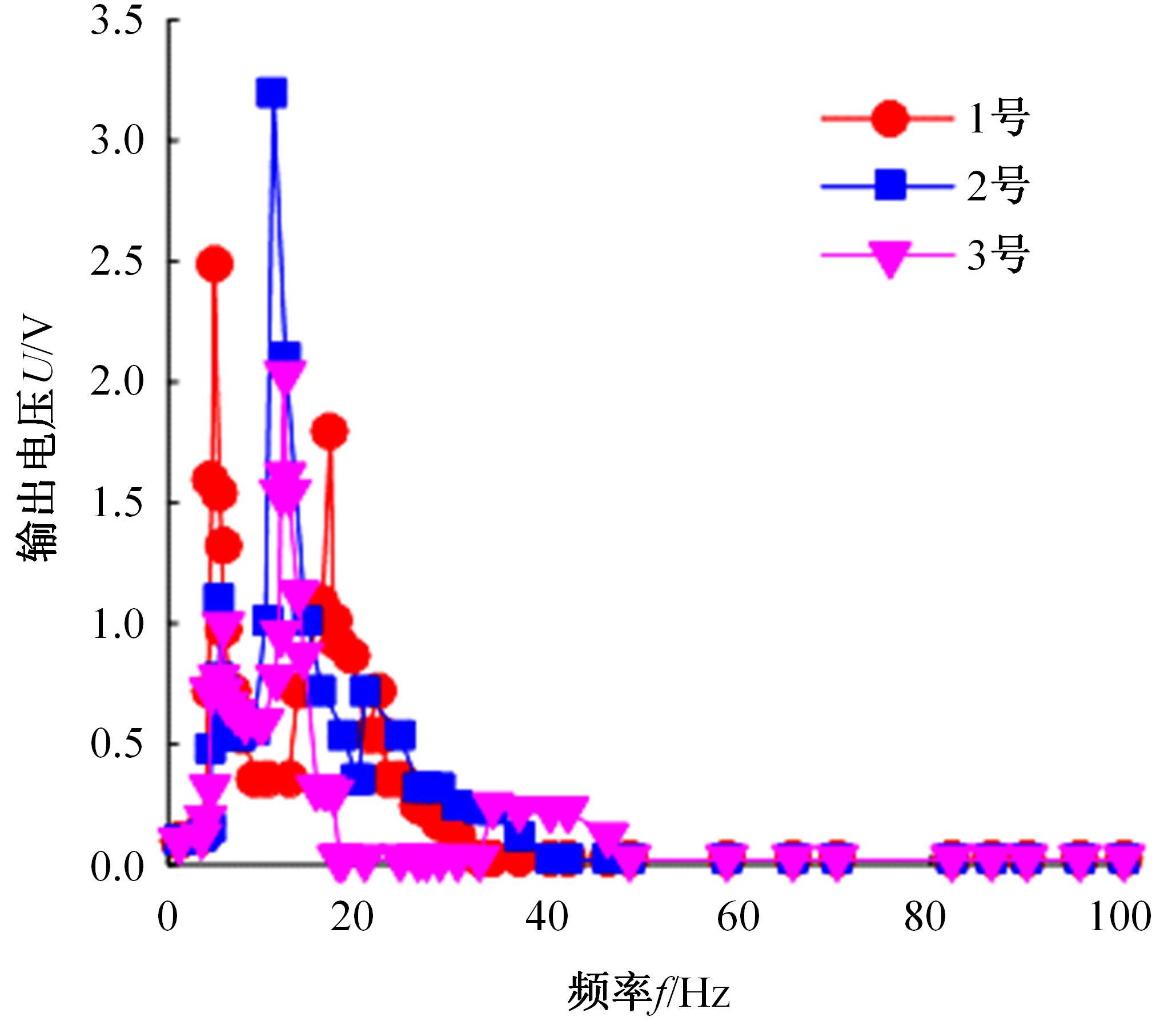
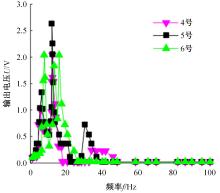
Journal of Jilin University(Engineering and Technology Edition) ›› 2023, Vol. 53 ›› Issue (10): 2807-2816.doi: 10.13229/j.cnki.jdxbgxb.20211323
Previous Articles Next Articles
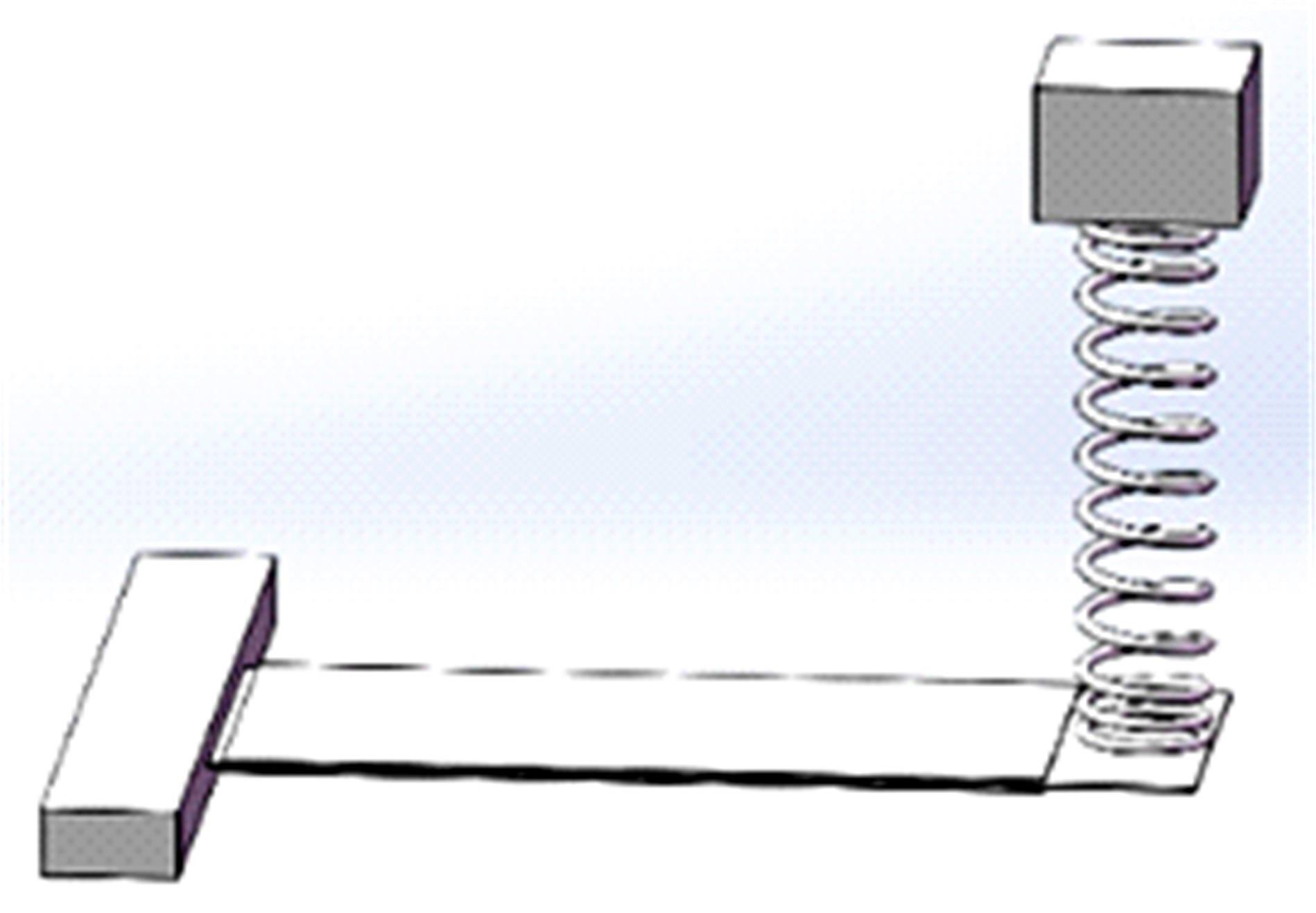
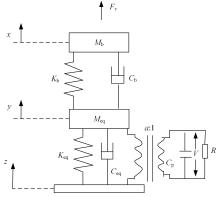
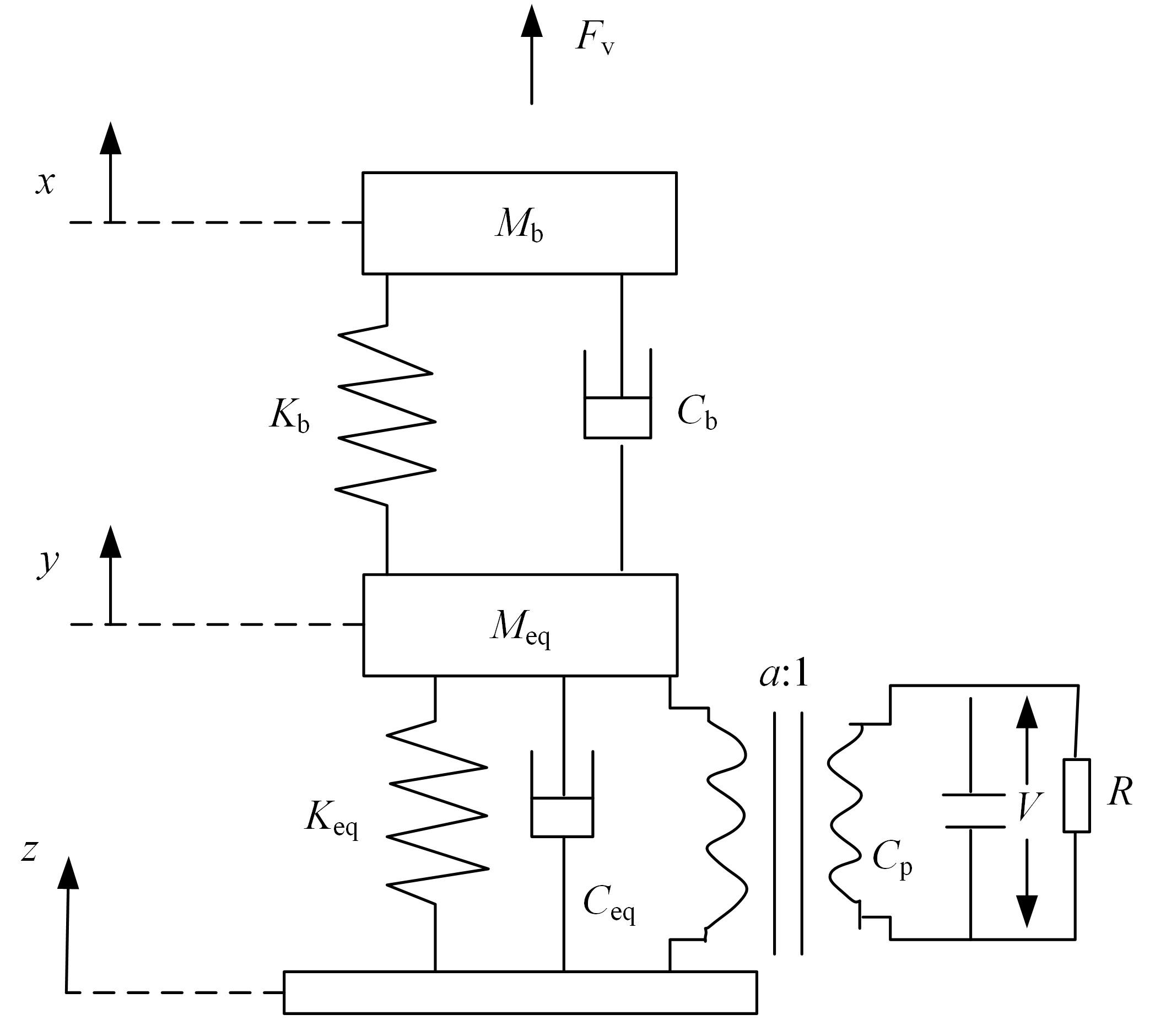
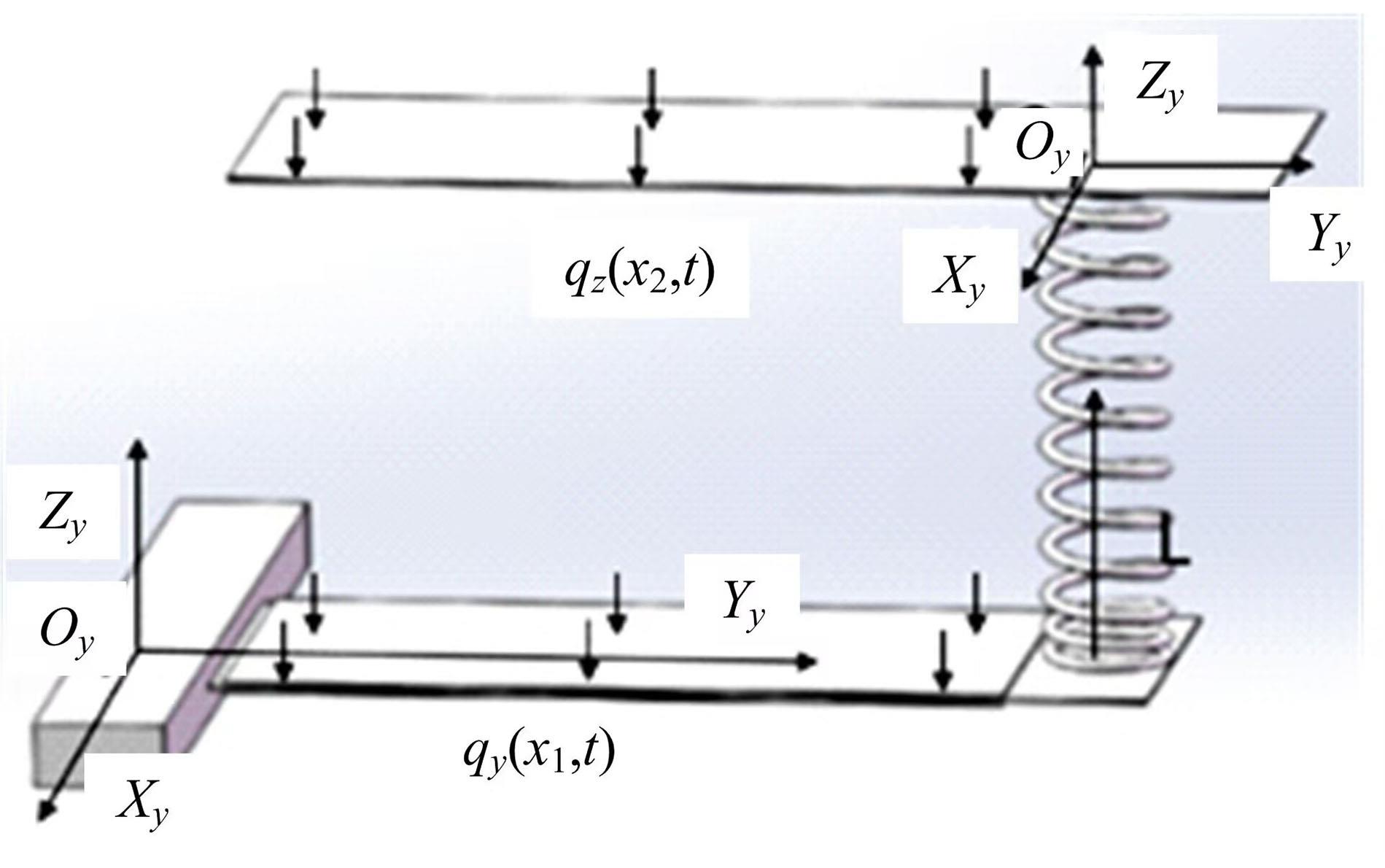
Low and ultra-wideband performance optimization of spring-connected mass piezoelectric cantilever
Jian-dong JIANG( ),Zhen YE,Xin QIAO(
),Zhen YE,Xin QIAO( )
)
- Key Laboratory of Special Purpose Equipment and Advanced Manufacturing Technology,Ministry of Education,Zhejiang University of Technology,Hangzhou 310014,China
CLC Number:
- TN712
| 1 | 钱志鸿,王义君. 面向物联网的无线传感器网络综述[J]. 电子与信息学报,2013,35(1): 215-227. |
| Qian Zhi-hong, Wang Yi-jun. Internet of things-oriented wireless sensor networks review[J]. Journal of Electronics & Information Technology, 2013, 35(1): 215-227. | |
| 2 | Mazumdar N, Nag A. Cache-aware mobile data collection schedule for IOT enabled multi-rate data generator wireless sensor network[J]. Sustainable Computing: Informatics and Systems, 2021(31):No. 100583. |
| 3 | Worlu C, Jamal A A, Mahiddin N A. Wireless sensor networks, internet of things, and their challenges[J]. International Journal of Innovative Technology and Exploring Engineering, 2019, 8(12): 556-566. |
| 4 | Safaei M, Sodano H A, Anton S R. A review of energy harvesting using piezoelectric materials: state-of-the-art a decade later (2008–2018)[J]. Smart Materials and Structures, 2019, 28(11): No.113001. |
| 5 | 徐振龙,单小彪,谢涛,等. 宽频压电振动俘能器的研究现状综述[J]. 振动与冲击,2018,7(8): 190-199, 205. |
| Xu Zhen-long, Shan Xiao-biao, Xie Tao, et al. A review of broadband piezoelectric vibration energy harvester[J]. Journal of Viration and Shock, 2018, 37(8): 190-199, 205. | |
| 6 | Shen D, Park J H, Ajitsaria J, et al. The design, fabrication and evaluation of a MEMS PZT cantilever with an integrated Si proof mass for vibration energy harvesting[J]. Journal of Micromechanics and Microengineering, 2008, 18(5): No.055017. |
| 7 | Li W G, He S, Yu S. Improving power density of a cantilever piezoelectric power harvester through a curved L-shaped proof mass[J]. IEEE Transactions on Industrial Electronics, 2009, 57(3): 868-876. |
| 8 | Challa V R, Prasad M G, Shi Y, et al. A vibration energy harvesting device with bidirectional resonance frequency tunability[J]. Smart Materials and Structures, 2008, 17(1): No.015035. |
| 9 | Liu J Q, Fang H B, Xu Z Y, et al. A MEMS-based piezoelectric power generator array for vibration energy harvesting[J]. Microelectronics Journal, 2008, 39(5): 802-806. |
| 10 | Cao D X, Leadenham S, Erturk A. Internal resonance for nonlinear vibration energy harvesting[J]. The European Physical Journal Special Topics, 2015, 224(14): 2867-2880. |
| 11 | Chen L Q, Jiang W A, Panyam M, et al. A broadband internally resonant vibratory energy harvester[J]. Journal of Vibration and Acoustics, 2016, 138(6):No.061007. |
| 12 | Roundy S, Leland E S, Baker J, et al. Improving power output for vibration-based energy scavengers[J]. IEEE Pervasive Computing, 2005, 4(1): 28-36. |
| 13 | Erturk A, Inman D J.压电能量采集(Piezoelectric energy harvesting) [M]. 北京: 国防工业出版社, 2015. |
| 14 | 蒋建东,张玖利,牛瑞征,等. 耦合弯曲-剪切载荷L型压电振子的低宽频特性[J]. 浙江大学学报:工学版,2021 55(1): 162-168. |
| Jian-dong Jang, Zhang Jiu-li, Niu Rui-zheng, et al. Low broadband characteristics of L-shaped piezoelectric cantilever beam with bending shear load[J]. Journal of Zhejiang University (Engineering Science), 2021, 55(1): 162-168. | |
| 15 | 杨斌强,徐文潭,陆国丽,等. 宽频压电振动能量采集器的分布参数模型与实验[J]. 中国机械工程,2017,28(2): 127-134. |
| Yang Bin-qiang, Xu Wen-tan, Lu Guo-li, et al. Distributed parameter model and experiments of a broadband piezoelectric vibration energy harvester[J]. Chinese Journal of Mechanical Engineering, 2017, 28(2): 127-134. | |
| 16 | 展永政,王光庆. 压电振动能量采集器的性能分析与功率优化[J]. 浙江大学学报:工学版,2014,48(7): 1248-1253. |
| Zhan Yong-zheng, Wang Guang-qing. Performance analysis and power optimization of piezoelectric vibration engineering harvester[J]. Journal of Zhejiang University (Engineering Science), 2014, 48(7): 1248-1253. | |
| 17 | 杨斌强,徐文潭,王学保,等. 带弹性放大器的双稳态压电振动能量采集器[J]. 传感技术学报,2017(30): 684-691. |
| Yang Bin-qiang, Xu Wen-tan, Wang Xue-bao, et al. A bistable piezoelectric vibration energy harvester with an elastic magnifier[J]. Chinese Journal of Sensors and Actuators, 2017(30): 684-691. | |
| 18 | Aldraihem O J, Wetherhold R C, Singh T. Distributed control of laminated beams: Timoshenko theory vs Euler-Bernoulli theory[J]. Journal of Intelligent Material Systems and Structures, 1997, 8(2): 149-157. |
| 19 | Liu H, Xu T, Huang Z, et al. Parametric design for a piezoelectric cantilever carrying oscillators to harvest multi-frequency vibration energy[J]. International Journal of Applied Electromagnetics and Mechanics, 2013, 41(4): 389-405. |
| 20 | Tang L H, Yang Y W. A nonlinear piezoelectric energy harvester with magnetic oscillator[J]. Applied Physics Letters,2012, 101(9): 094102. |
| 21 | 夏呈. 修正铁摩辛柯梁受迫振动响应分析及其应用[D]. 南京: 东南大学机械工程学院,2017. |
| Xia Cheng. The analysis of forced vibration response of modified Timoshenko beam theory and its application [D]. Nanjing: College of Mechanical Engineering, Southeast University,2017. |
| [1] | Ming-wei HU,Hong-guang WANG,Xin-an PAN. Global structural optimization design of collaborative robots using orthogonal design [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(1): 370-378. |
| [2] | Chun-ling ZHONG,Dong LIANG,Yun-long ZHANG,Jing WANG. Calculation of natural vibration frequency of simply supported beam strengthened by external prestressing [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(6): 1884-1890. |
| [3] | WANG Xiu-gang, SU Jian, CAO Xiao-ning, LIN Hui-ying, ZHANG Yi-rui, YANG Xiao-min. Experiment on natural vibration characteristics of suspension based on simulation of semi-vehicle [J]. 吉林大学学报(工学版), 2014, 44(6): 1583-1590. |
| [4] | ZHANG Tian-xiao, LIU Xin-hui, ZHANG Nong. Vibration analysis of hydraulic relief valve [J]. 吉林大学学报(工学版), 2014, 44(01): 91-94. |
| [5] | LIU Han-bing, WANG Long-lin, TAN Guo-jin, CHENG Yong-chun, WU Chang-xia. Effect of prestress force on natural frequency of external prestressed simply supported steel beam [J]. 吉林大学学报(工学版), 2013, 43(01): 81-85. |
| [6] | HAN Zhi-Wu, LV You, DONG Li-Chun, ZHANG Jun-Qiu, NIU Shi-Chao, MA Rong-Feng, REN Lou-Quan. Modal analysis of gear with bionic surface morphology [J]. 吉林大学学报(工学版), 2010, 40(06): 1604-1608. |
| [7] | JIN Wen-ming, LI Hua-jun, KOU Shu-qing, YANG Sheng-hua. Modal analysis of frame of cantilever NC assembling machine for assembled camshaft [J]. 吉林大学学报(工学版), 2009, 39(增刊2): 319-0323. |
| [8] | SU Tie-jian, GUAN Ai-hua, MAI Li. Dynamic Analysis of Elastic Plate with Dispersing Pressure [J]. 吉林大学学报(工学版), 2000, (3): 72-74. |
| [9] | LIU Han-bing, GONG Guo-qing, WEI Yuan. Improved r-and h-adaptive Finite Element Method Based on Perturbation and Element Energy Ratio [J]. 吉林大学学报(工学版), 2000, (3): 56-60. |
|
||