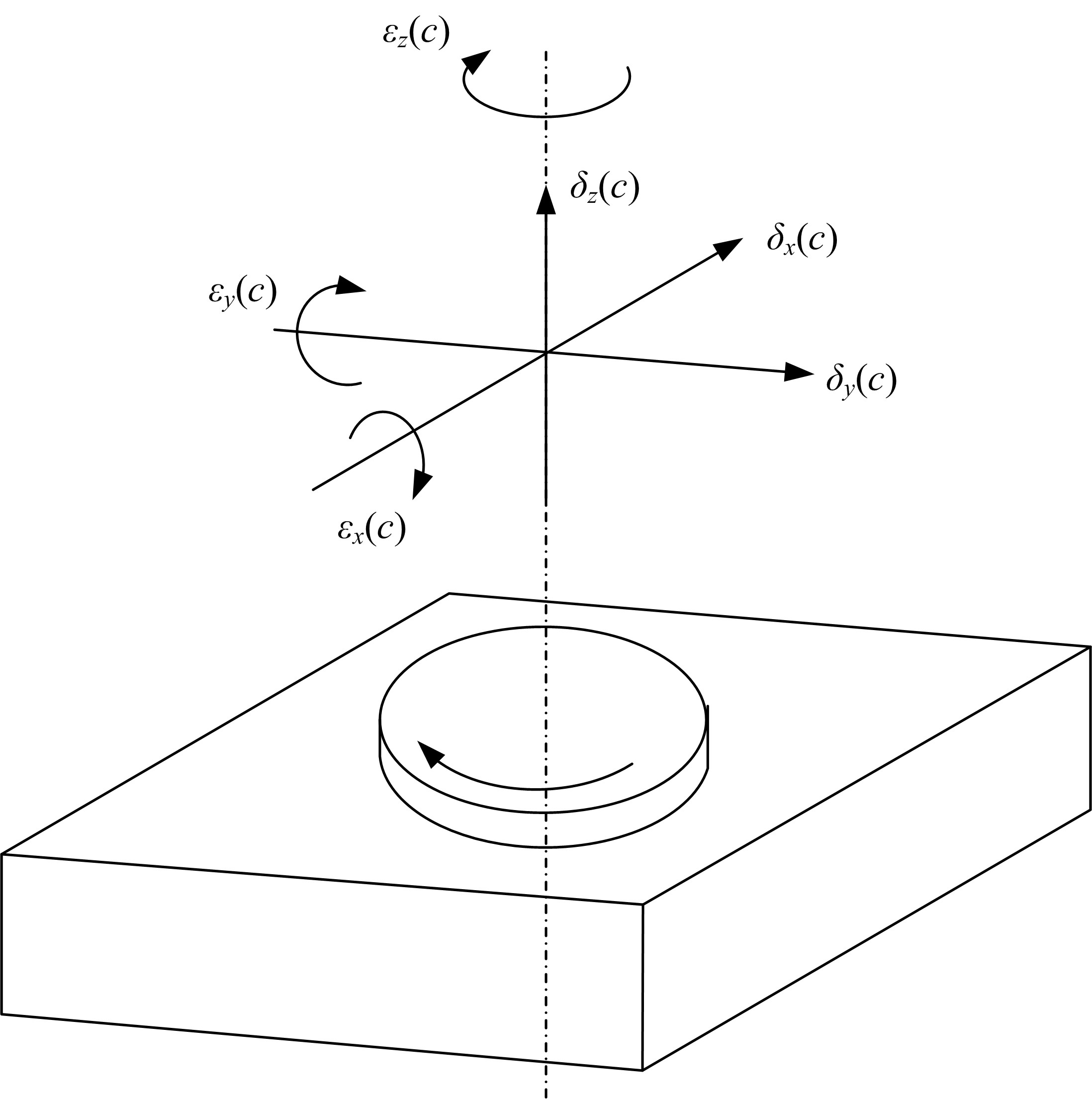
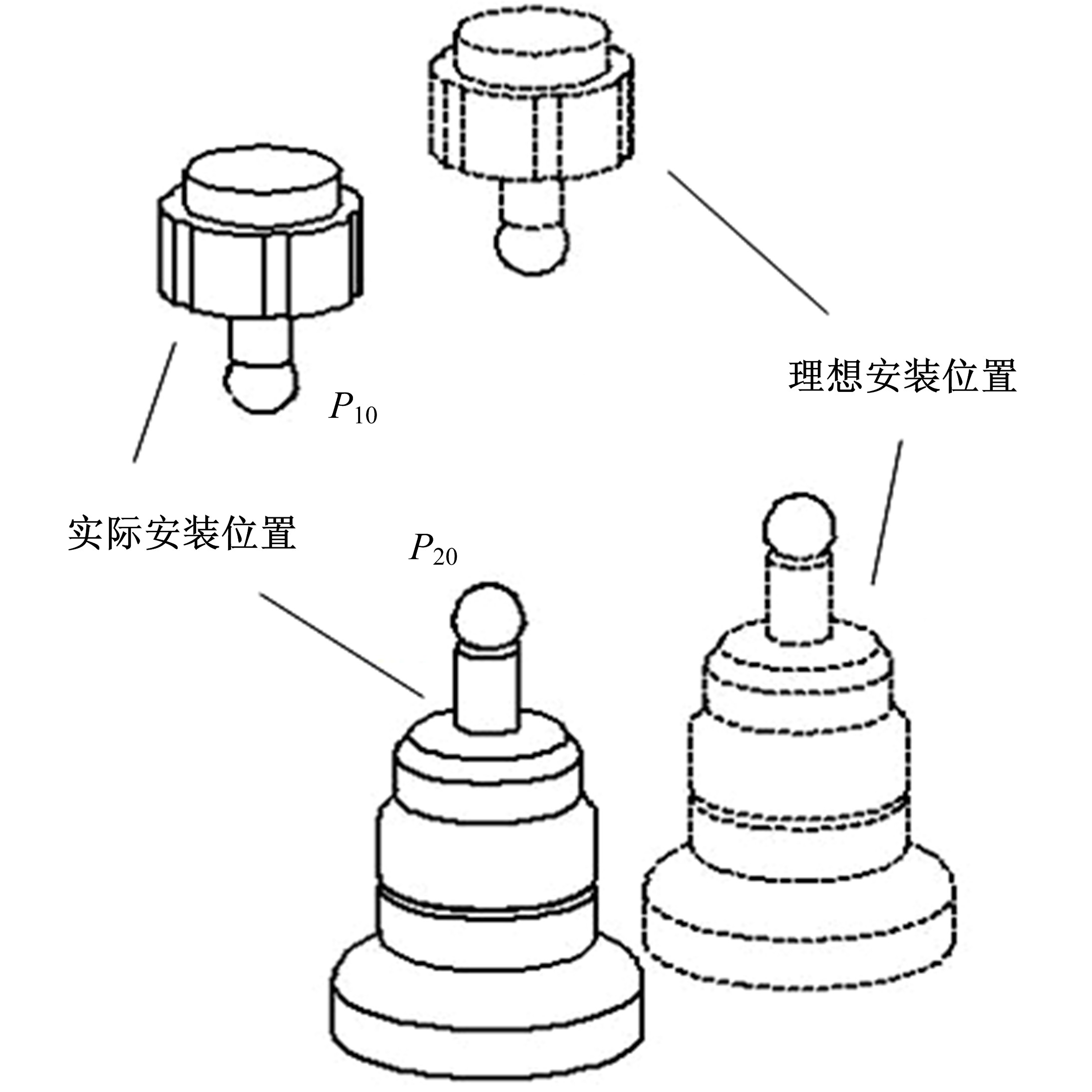
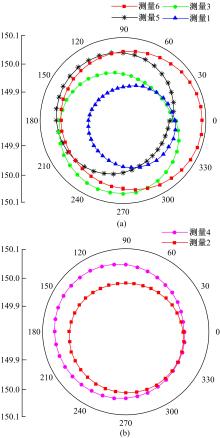
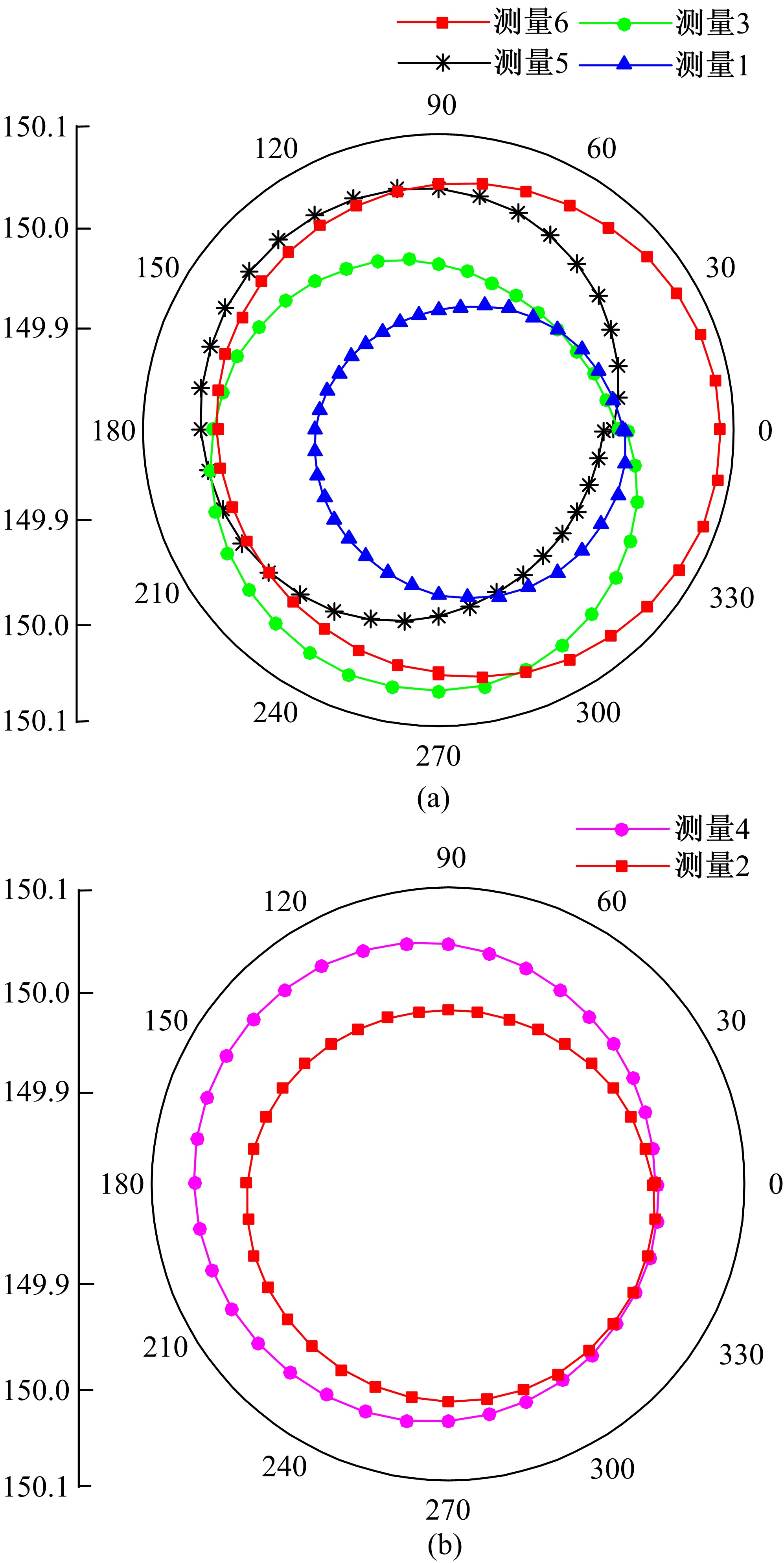
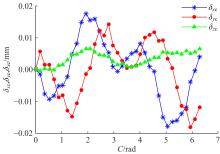
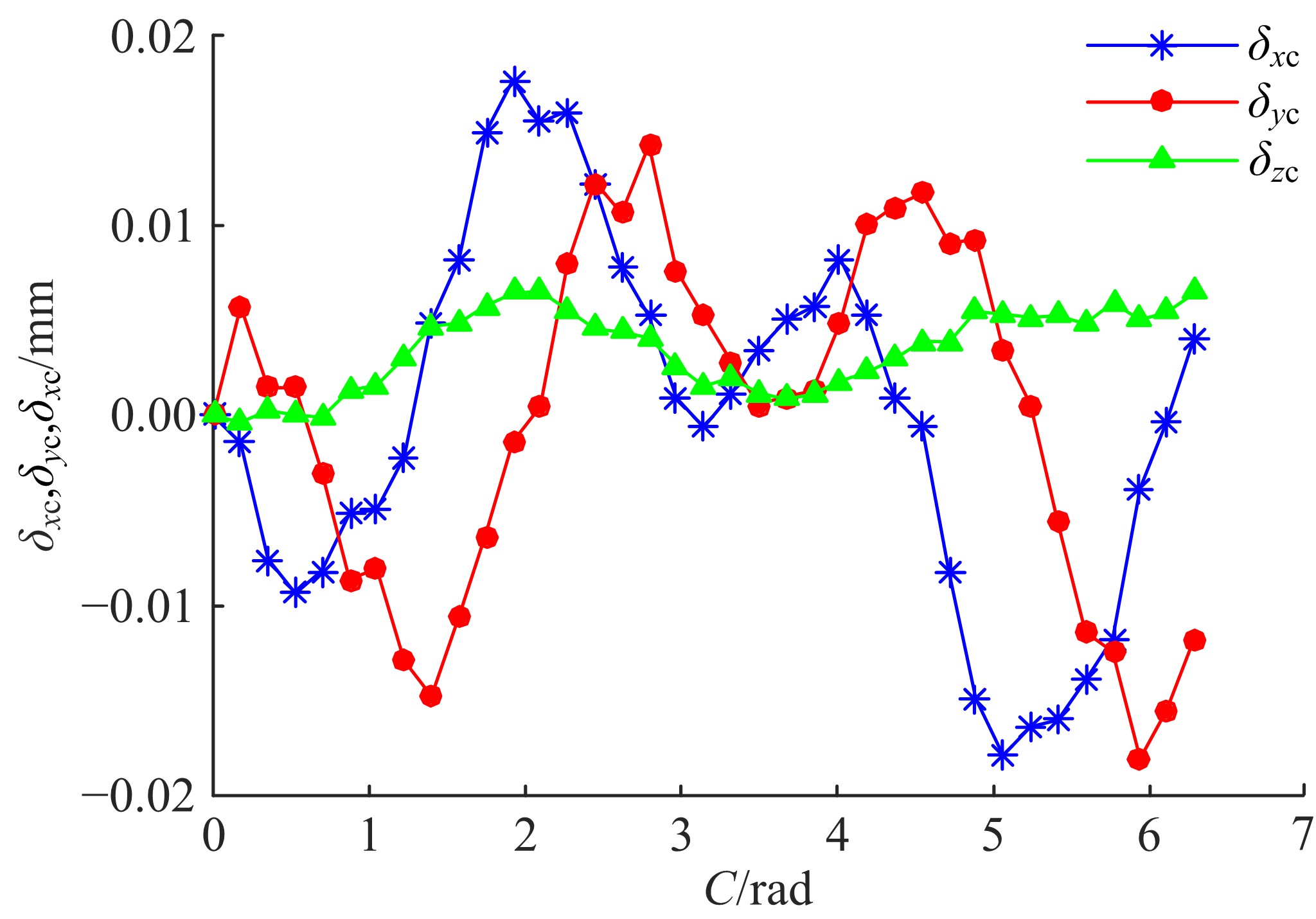
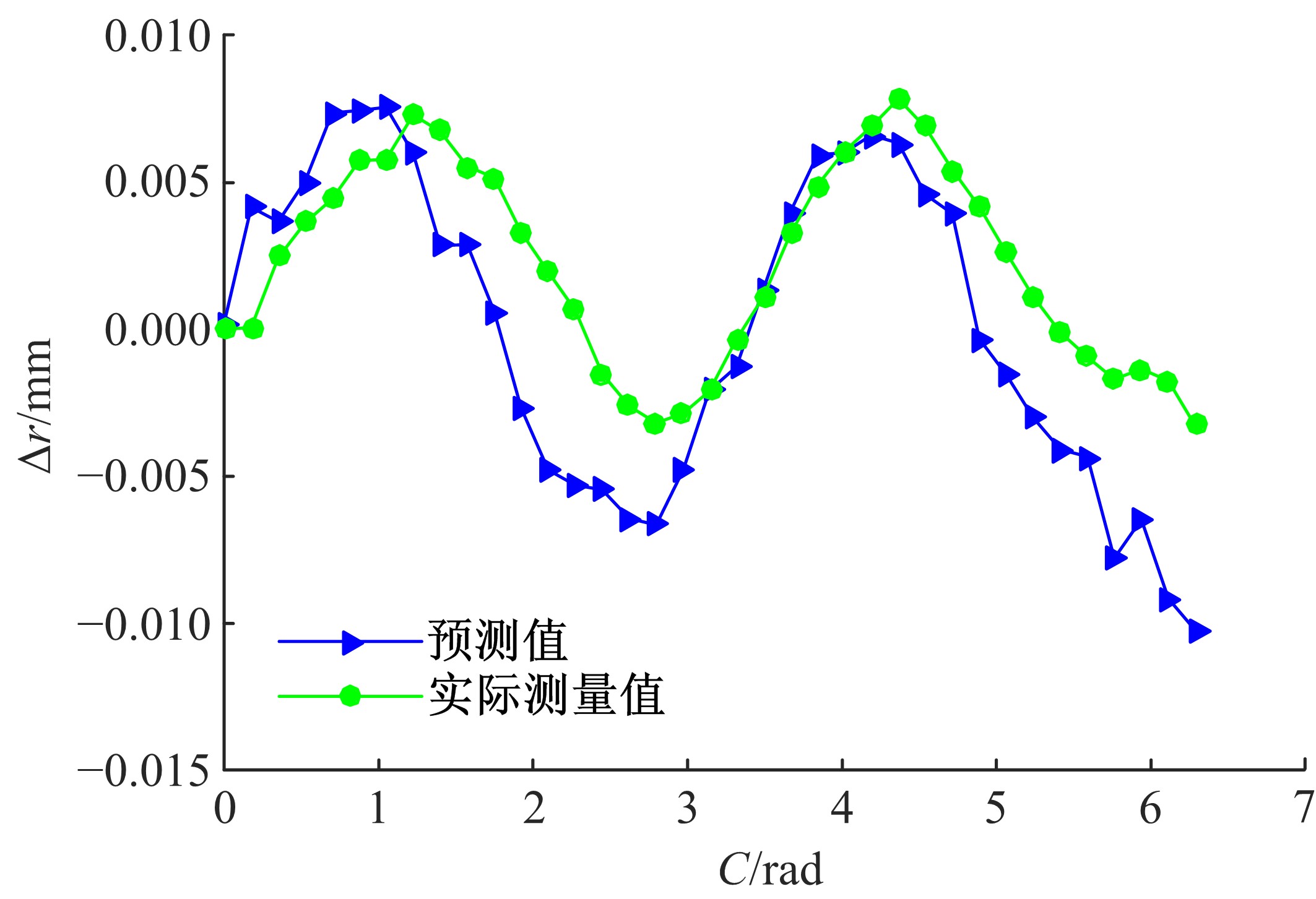
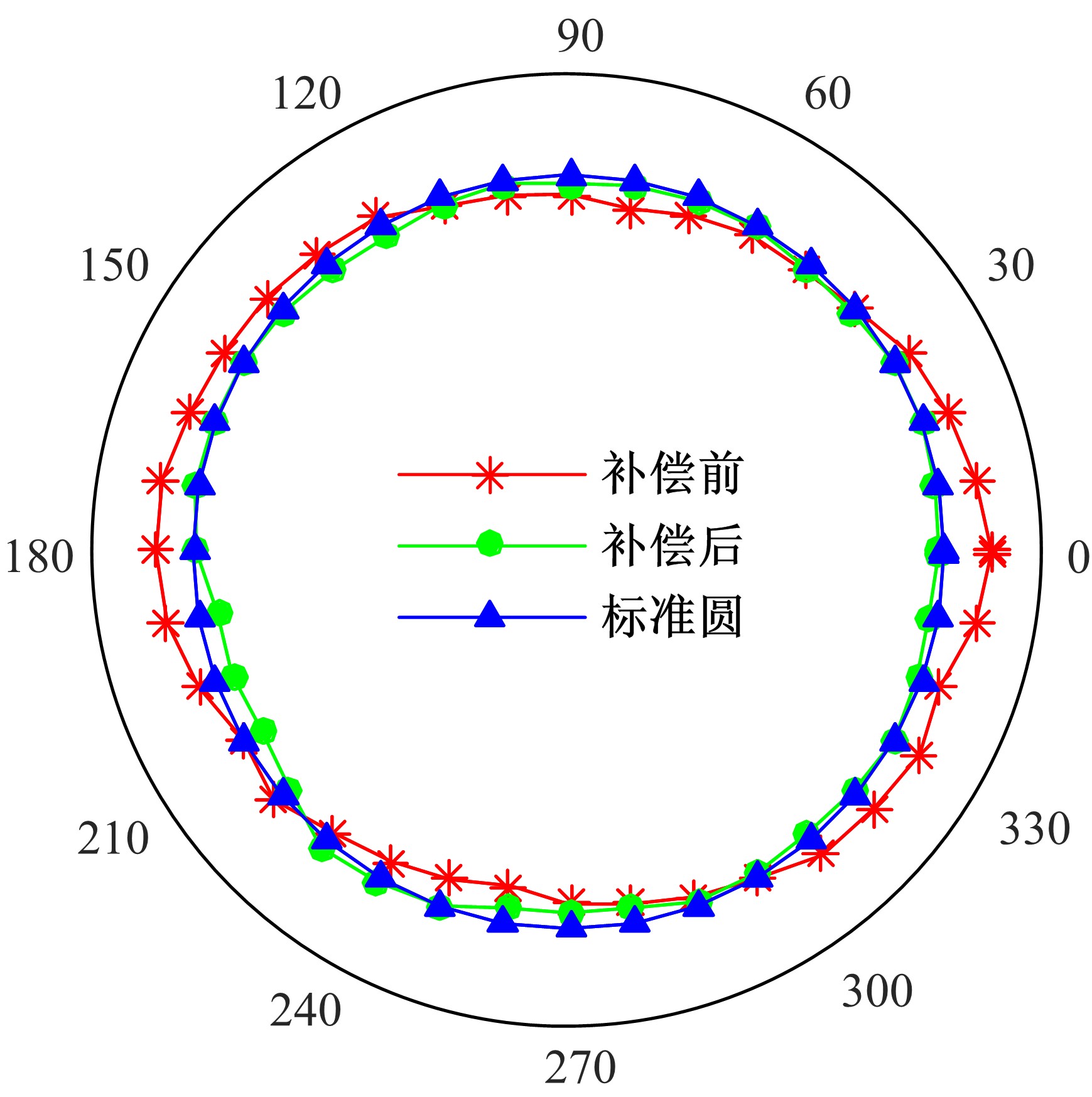
Journal of Jilin University(Engineering and Technology Edition) ›› 2021, Vol. 51 ›› Issue (2): 458-467.doi: 10.13229/j.cnki.jdxbgxb20200031

Previous Articles    

Rapid measurement and identification of position dependent geometric errors of CNC machine tool turntable

Guo-long LI(),Xiao-hui TAO,Kai XU,Zhe-yu LI   

  1. State Key Laboratory of Mechanical Transmission，Chongqing University，Chongqing 400044，China
  • Received:2020-01-11 Online:2021-03-01 Published:2021-02-09

RICH HTML

 6   

PDF (PC)

425

Abstract:

In order to quickly and systematically identify the position dependent geometric error terms of CNC machine tool turntable， a rapid measurement and identification method of the geometric error of the turntable based on a double ballbar is proposed. Firstly， based on the homogeneous coordinate transformation theory， the position dependent geometric error model of the machine tool turntable is established， and the relationship between the change in ballbar length and the geometric error during the movement of the ballbar is obtained. Then， a six-time tapered installation method of the ballbar is designed， the error identification matrix expression is direved， the change in the length of the ballbar during the movement of the ballbar is record， and the position dependent geometric error terms of the turntable can be quickly identified. Then， a method for eliminating the installation error of the double ballbar is proposed， which can effectively eliminate the influence of the installation error in the raw data of the ballbar measurement. Finally， based on the identification results of the position dependent geometric errors of the turntable， the change in the length of the ballbar in the additional experiment is predicted， and compared with the experimental results， the prediction accuracy is high. On this basis， the position dependent geometric error of the turntable is compensated. It is show that after compensation， the accuracy is significantly improved compared with that before compensation. The experimental results show that the method can accurately and quickly identify the position dependent geometric error terms of the turntable， which has important significance for improving the accuracy of the machine tool.

Key words: mechanical manufacturing and automation, CNC machine tool turntable, position dependent geometric errors, the double ballbar, installation error

CLC Number: 

  • TH161

Fig.1

PDGES of C-axis"

Fig.2

Double ballbar test device"

Fig.3

Double ballbar test mode"

Fig.4

Double ballbar setup error"

Table 1

Radius calculation results and simulation results"

测量模式拟合圆半径
计算值/mm仿真值/mm差值/mm
1100.0480100.04530.0027
2150.0498150.04310.0067
3100.0520100.04580.0062
4150.0525150.04380.0087
5100.0480100.04530.0027
6100.0514100.0593-0.0079

Table 2

Center x coordinate calculation results and simulation results"

测量模式拟合圆圆心x坐标
计算值/mm仿真值/mm差值/mm
1-0.0400-0.0333-0.0067
2-0.0267-0.0184-0.0083
3-0.0480-0.05030.0023
4-0.0320-0.03670.0047
5-0.0400-0.0336-0.0064
6-0.0295-0.03600.0065

Table 3

Center y coordinate calculation results and simulation results"

测量模式拟合圆圆心y坐标
计算值/mm仿真值/mm差值/mm
1-0.0480-0.05500.0070
2-0.0320-0.04370.0117
30.04000.02890.0111
40.02670.01230.0144
5-0.0400-0.04890.0089
6-0.0386-0.0300-0.0086

Table 4

Double ballbar setup position"

测量刀具球P1工件球P2
1（0,0,60）（80,0,0）
2（0,0,126.886）（80,0,0）
3（0,0,60）（0,80,0）
4（0,0,126.886）（0,80,0）
5（0,0,60）（-80,0,0）
6（-60,80,80）（0,80,0）

Fig.5

Double ballbar test picture"

Fig.6

Double ballbar test result"

Fig.7

Linear error identification results"

Fig.8

Angle error identification result"

Fig.9

Double ballbar forecast and test results"

Fig.10

Results of double ballbar test before and after compensation"

1 杨建国, 范开国, 杜正春. 数控机床误差实时补偿技术[M]. 北京: 机械工业出版社, 2013.
2 Abbaszadeh-Mir Y, Mayer J R R, Cloutier G, et al. Theory and simulation for the identification of the link geometric errors for a five-axis machine tool using a telescoping magnetic ball-bar[J]. International Journal of Production Research, 2002, 40(18): 4781-4797.
3 . Test code for machine tools—part 7: geometric accuracy of axes of rotation[S].
4 Zargarbashi S H H, Mayer J R R. Single setup estimation of a five-axis machine tool eight link errors by programmed end point constraint and on the fly measurement with capball sensor[J]. International Journal of Machine Tools and Manufacture, 2009, 49(10): 759-766.
5 Wang M, Hu J Z, Zan T. Kinematic error separation on five-axis NC machine tool based on telescoping double ball bar[J]. Frontiers of Mechanical Engineering, 2010, 5(4): 431-437.
6 He Z, Fu J, Zhang L, et al. A new error measurement method to identify all six error parameters of a rotational axis of a machine tool[J]. International Journal of Machine Tools and Manufacture, 2015, 88: 1-8.
7 Hong C, Ibaraki S, Oyama C. Graphical presentation of error motions of rotary axes on a five-axis machine tool by static R-test with separating the influence of squareness errors of linear axes[J]. International Journal of Machine Tools and Manufacture, 2012, 59: 24-33.
8 Ibaraki S, Oyama C, Otsubo H. Construction of an error map of rotary axes on a five-axis machining center by static R-test[J]. International Journal of Machine Tools and Manufacture, 2011, 51(3): 190-200.
9 Bi Q, Huang N, Sun C, et al. Identification and compensation of geometric errors of rotary axes on five-axis machine by on-machine measurement[J]. International Journal of Machine Tools and Manufacture, 2015, 89: 182-191.
10 Ibaraki S, Iritani T, Matsushita T. Calibration of location errors of rotary axes on five-axis machine tools by on-the-machine measurement using a touch-trigger probe[J]. International Journal of Machine Tools and Manufacture, 2012, 58: 44-53.
11 Uddin M S, Ibaraki S, Matsubara A, et al. Prediction and compensation of machining geometric errors of five-axis machining centers with kinematic errors[J]. Precision Engineering, 2009, 33(2): 194-201.
12 Xiang S, Yang J, Zhang Y. Using a double ball bar to identify position-independent geometric errors on the rotary axes of five-axis machine tools[J]. The International Journal of Advanced Manufacturing Technology, 2014, 70(9-12): 2071-2082.
13 Zhang Y, Yang J, Zhang K. Geometric error measurement and compensation for the rotary table of five-axis machine tool with double ballbar[J]. International Journal of Advanced Manufacturing Technology, 2013, 65(1-4): 275-281.
14 Zargarbashi S H H, Mayer J R R. Assessment of machine tool trunnion axis motion error, using magnetic double ball bar[J]. International Journal of Machine Tools and Manufacture, 2006, 46(14): 1823-1834.
15 Tsutsumi M, Tone S, Kato N, et al. Enhancement of geometric accuracy of five-axis machining centers based on identification and compensation of geometric deviations[J]. International Journal of Machine Tools and Manufacture, 2013, 68(5): 11-20.
16 Lee K I, Lee D M, Yang S H. Parametric modeling and estimation of geometric errors for a rotary axis using double ball-bar[J]. International Journal of Advanced Manufacturing Technology, 2012, 62(5-8): 741-750.
17 徐凯, 李国龙, 何坤, 等. 基于球杆仪的直线轴位置相关误差辨识研究[J]. 仪器仪表学报, 2019, 40(5): 1-9.
Xu Kai, Li Guo-long, He Kun, et. al. Research on dependent error identification of linear axes based on double ball-bar[J]. Chinese Journal of Scientific Instrument, 2019, 40(5): 1-9.
18 付国强, 傅建中, 沈洪垚. 五轴数控机床旋转轴几何误差辨识新方法[J]. 浙江大学学报: 工学版, 2015, 49(5): 848-857.
Fu Guo-qiang, Fu Jian-zhong, Shen Hong-yao. One novel geometric error identification of rotary axes for five-axis machine tool[J]. Journal of Zhejiang University (Engineering Science), 2015, 49(5): 848-857.
19 郭世杰, 姜歌东, 梅雪松. 摆头转台型五轴机床旋转轴运动误差测量与辨识[J]. 农业机械学报, 2019, 50(2): 402-410, 426.
Guo Shi-jie, Jiang Ge-dong, Mei Xue-song. Motion error measurement and identification of rotary axis of five-axis machine tool[J]. Transactions of the Chinese Society for Agricultural Machinery, 2019, 50(2): 402-410, 426.
20 Ding S, Wu W, Huang X, et al. Single-axis driven measurement method to identify position-dependent geometric errors of a rotary table using double ball bar[J]. The International Journal of Advanced Manufacturing Technology, 2019,101(5-8): 1715-1724.
21 Zhu S, Ding G, Qin S, et al. Integrated geometric error modeling, identification and compensation of CNC machine tools[J]. International Journal of Machine Tools & Manufacture, 2012, 52(1): 24-29.
22 刘志峰, 刘广博, 程强, 等. 基于多体系统理论的精密立式加工中心精度建模与预测[J]. 吉林大学学报: 工学版, 2012, 42(2): 388-391.
Liu Zhi-feng, Liu Guang-bo, Cheng Qiang, et al. Precision modeling and prediction of precise vertical machining center based on theory of multi-body system[J]. Journal of Jilin University (Engineering and Technology Edition), 2012, 42(2): 388-391.
23 曲兴田, 赵永兵, 刘海忠, 等. 基串并混联机床几何误差建模与实验[J]. 吉林大学学报: 工学版, 2017, 47(1): 137-144.
Qu Xing-tian, Zhao Yong-bing, Liu Hai-zhong, et al. Modeling and experiment of spatial geometric errors of hybrid serial parallel machine tool[J]. Journal of Jilin University (Engineering and Technology Edition), 2017, 47(1): 137-144.
24 Lee K I, Yang S H. Measurement and verification of position-independent geometric errors of a five-axis machine tool using a double ball-bar[J]. International Journal of Machine Tools and Manufacture, 2013, 70(4): 45-52.
[1] Yun-wei ZHAO,De-xu GENG,Xiao-min LIU,Qi LIU. Implementation and stability on turning with constant radius of pneumatic flexible hexapod robot [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 472-482.
[2] Chun-zheng DUAN,Fang-yuan ZHANG,Wen-neng KOU,Bin WEI. Martensitic transformation of surface white layer in high speed hard cutting [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(5): 1575-1583.
[3] LIU Guo-zheng, SHI Wen-ku, Chen Zhi-yong. Finite element analysis of transmission error for hypoid gears considering installation error [J]. 吉林大学学报(工学版), 2018, 48(4): 984-989.
[4] REN Shu-nan, YANG Xiang-dong, WANG Guo-lei, LIU Zhi, CHEN Ken. Base position planning of mobile manipulator for large parts painting [J]. 吉林大学学报(工学版), 2016, 46(6): 1995-2002.
[5] GUO Li-bin, ZHANG Bin, CUI Hai, ZHANG Zhi-hang. Structural parameters of 3D roughness for micro wire electrical discharge machining surface [J]. 吉林大学学报(工学版), 2015, 45(3): 851-856.
[6] ZHANG Lei, ZHAO Yun-wei, YANG Zhuo, ZHAO Ji. Shear yield property of electrorheological polishing fluid [J]. , 2012, 42(05): 1145-1150.
[7] ZHANG Lei,HE Xin-sheng,ZHANG Ying,YANG He-ran. Development of new type ER fluid-assisted polishing  tool and its polishing experiment [J]. 吉林大学学报(工学版), 2010, 40(04): 1009-1014.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
Copyright © Journal of Jilin University(Engineering and Technology Edition), All Rights Reserved.
Tel: +86-431-85095297　Fax: +86-431-85094074　E-mail: xbgxb@jlu.edu.cn
Powered by Beijing Magtech Co. Ltd