基于弹塑性应力场理论的钢筋混凝土梁受剪承载力

doi:10.13229/j.cnki.jdxbgxb20190940

摘要/Abstract

摘要：

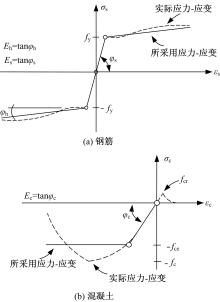
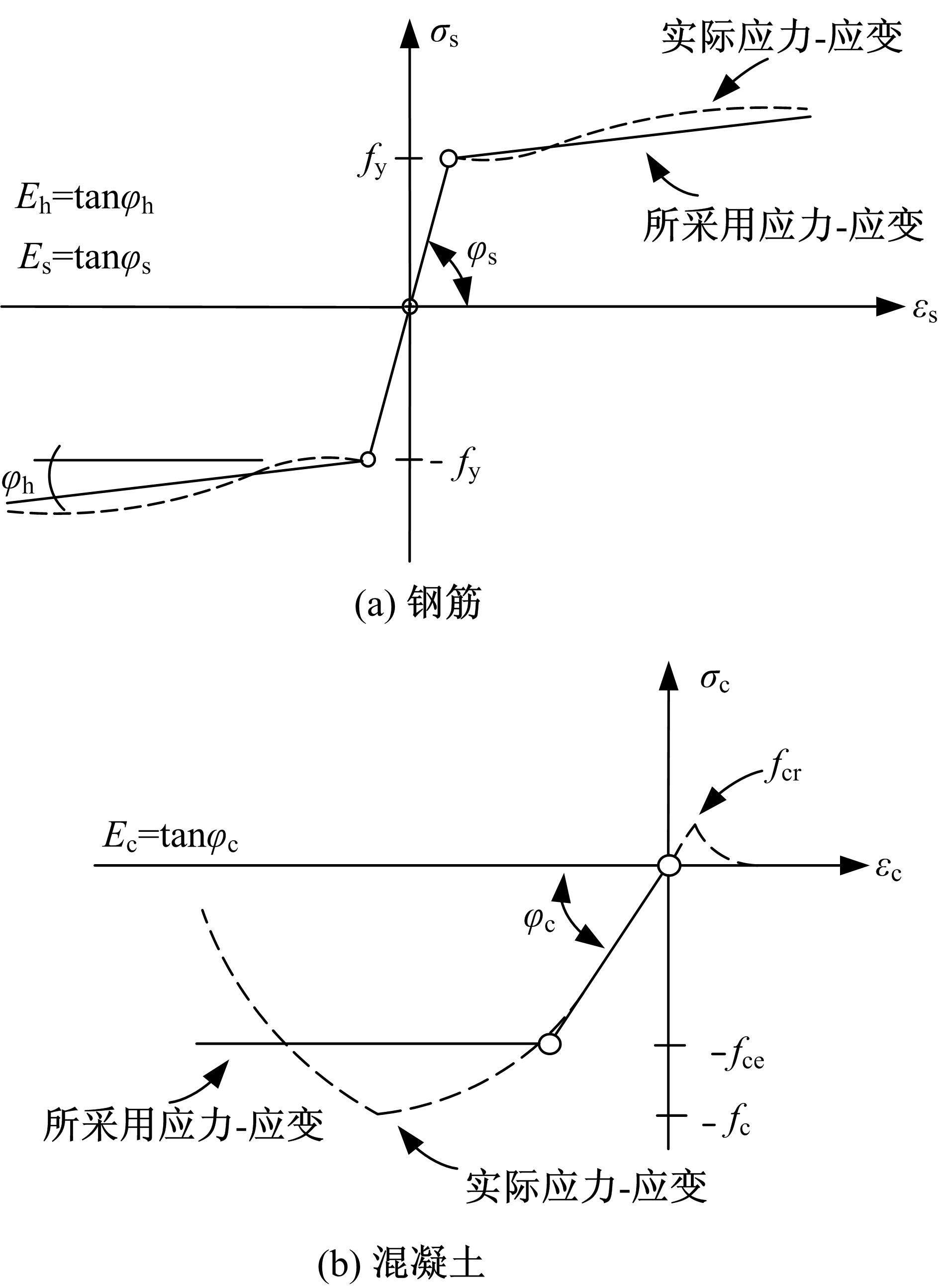
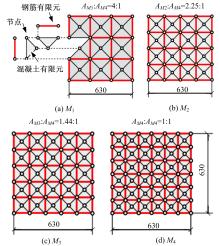
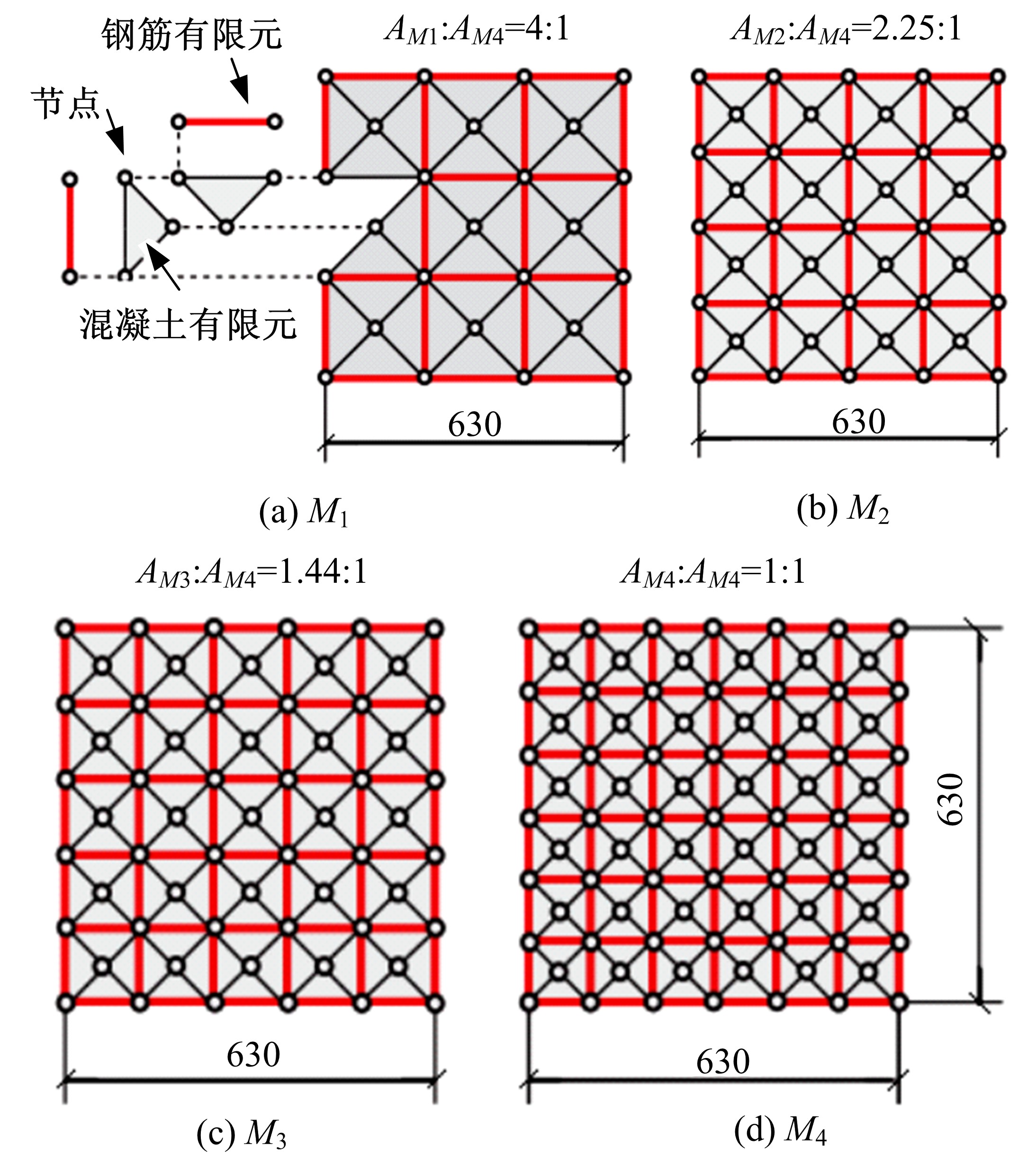
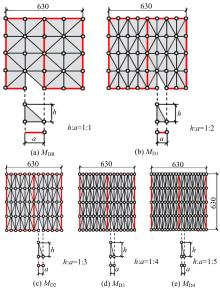
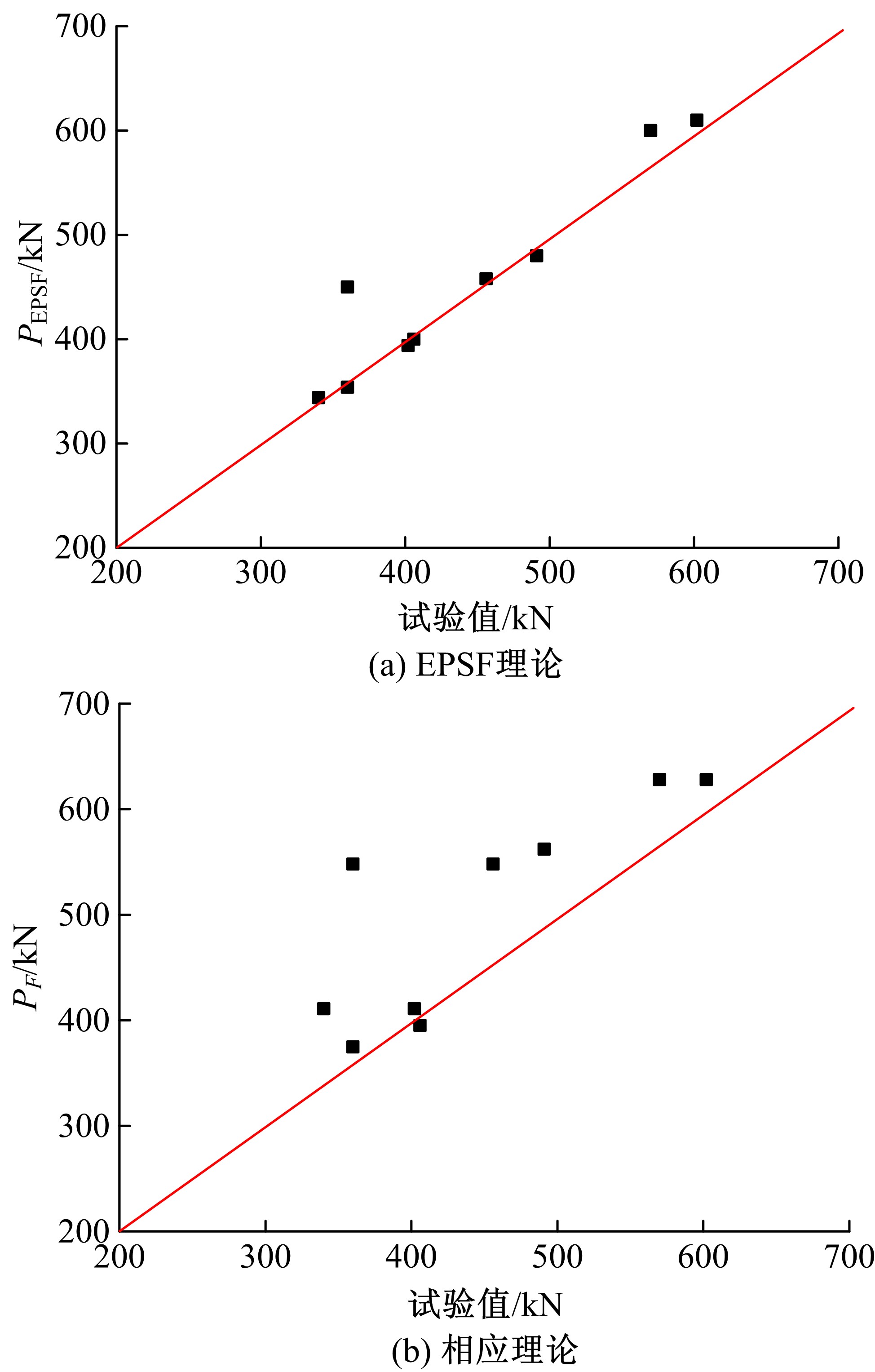
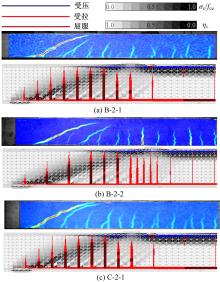
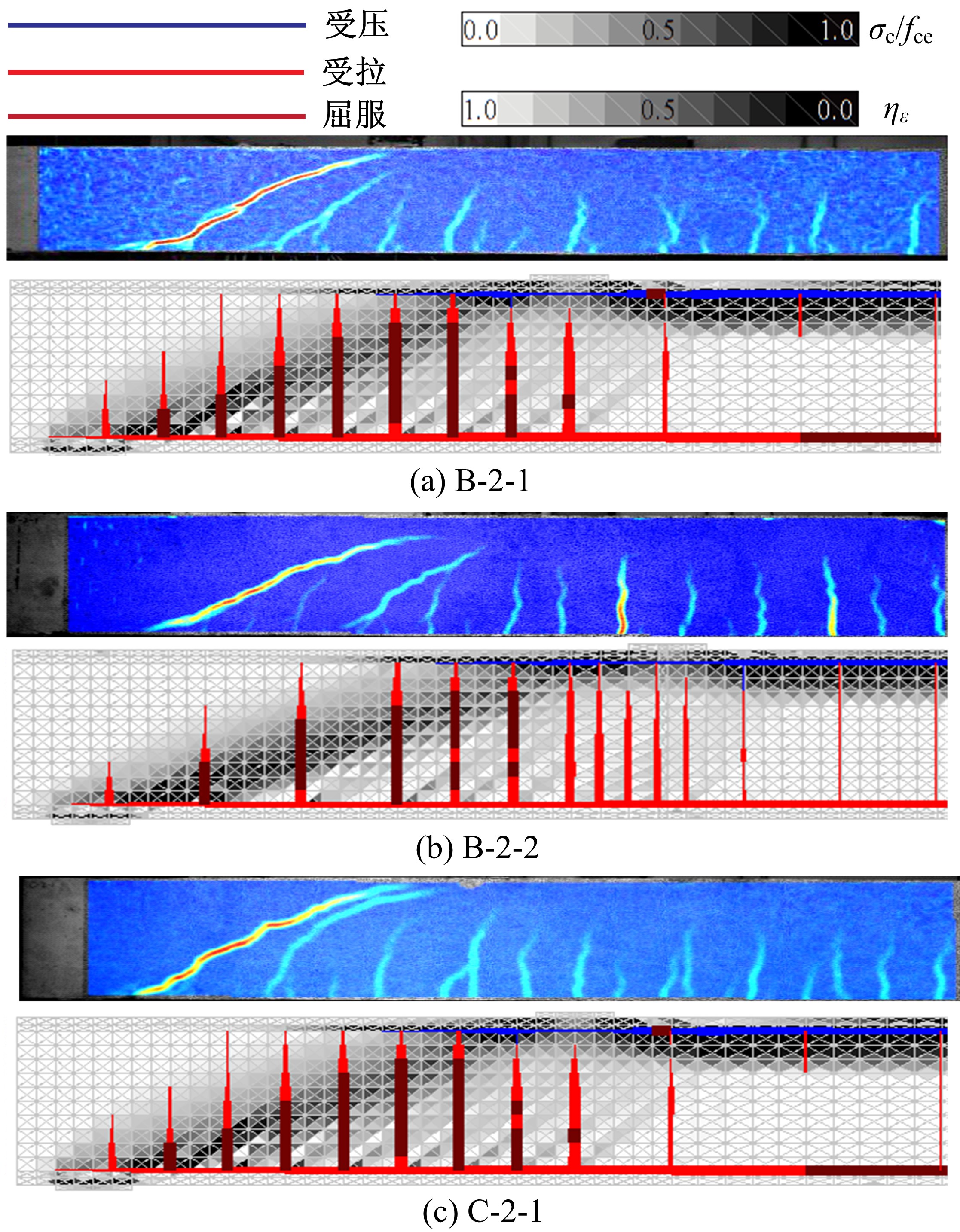
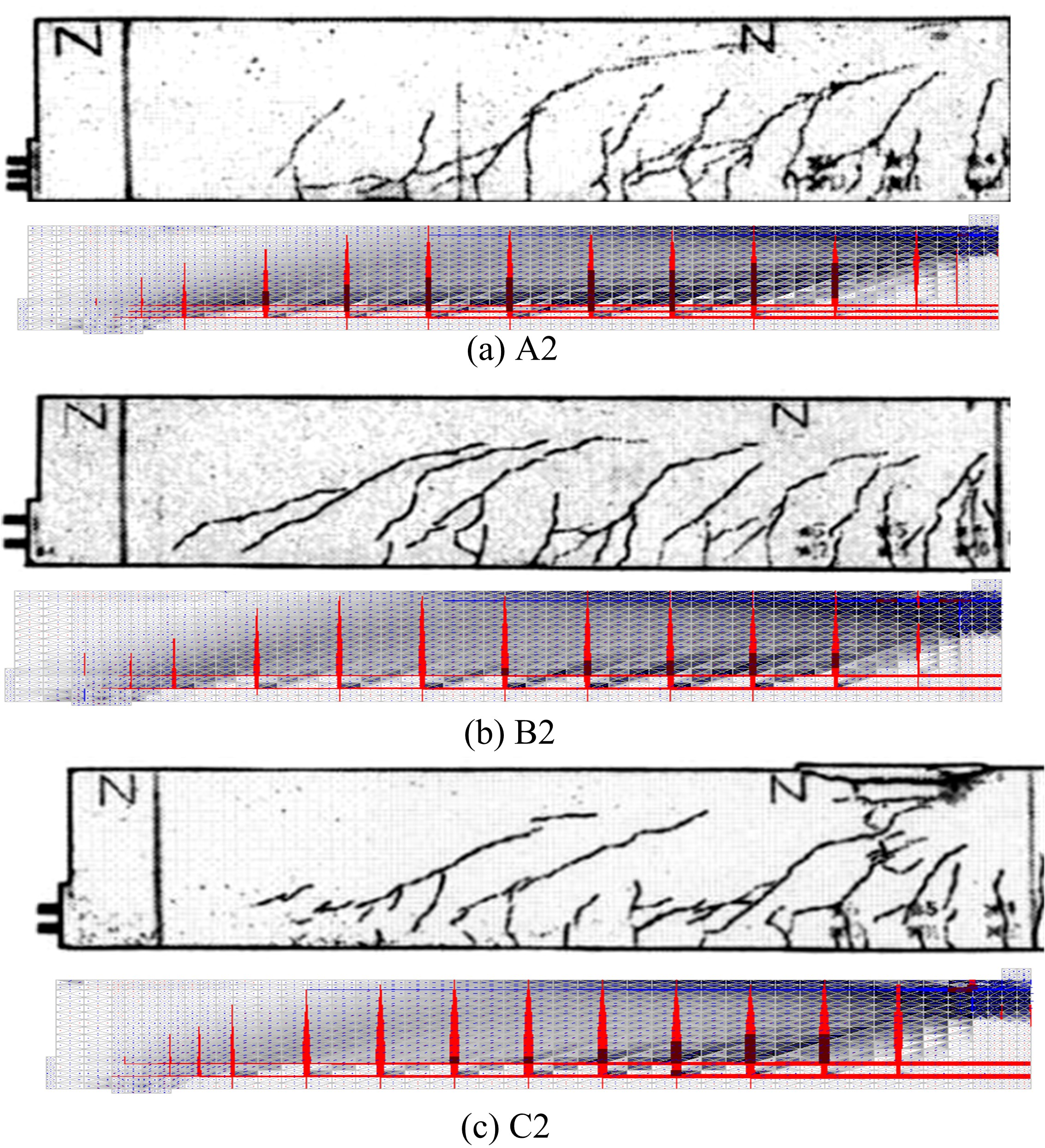
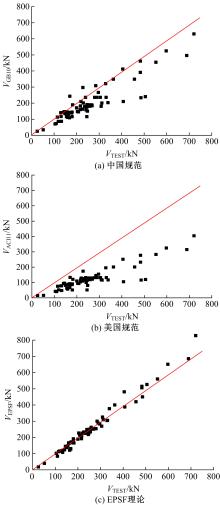
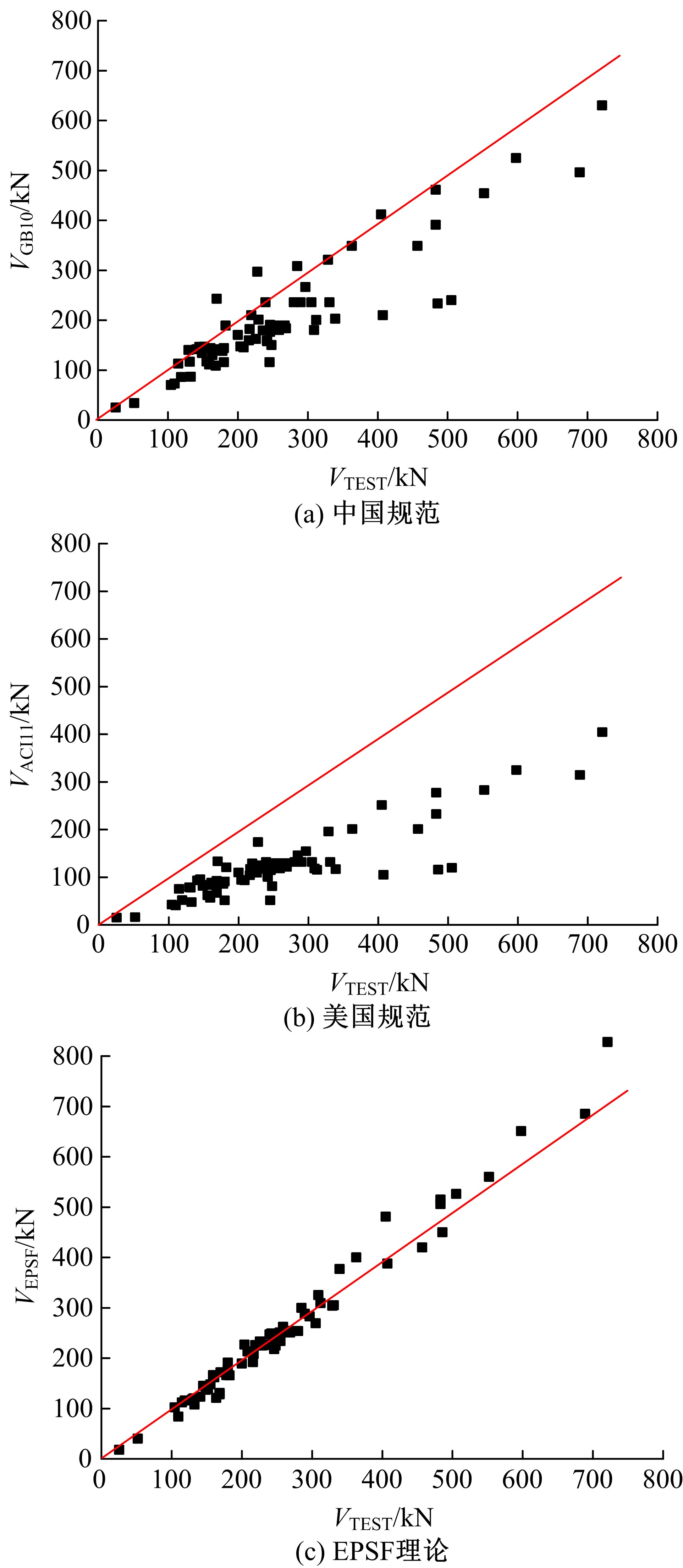
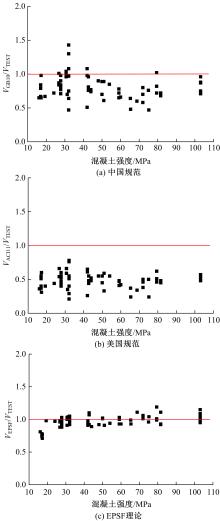
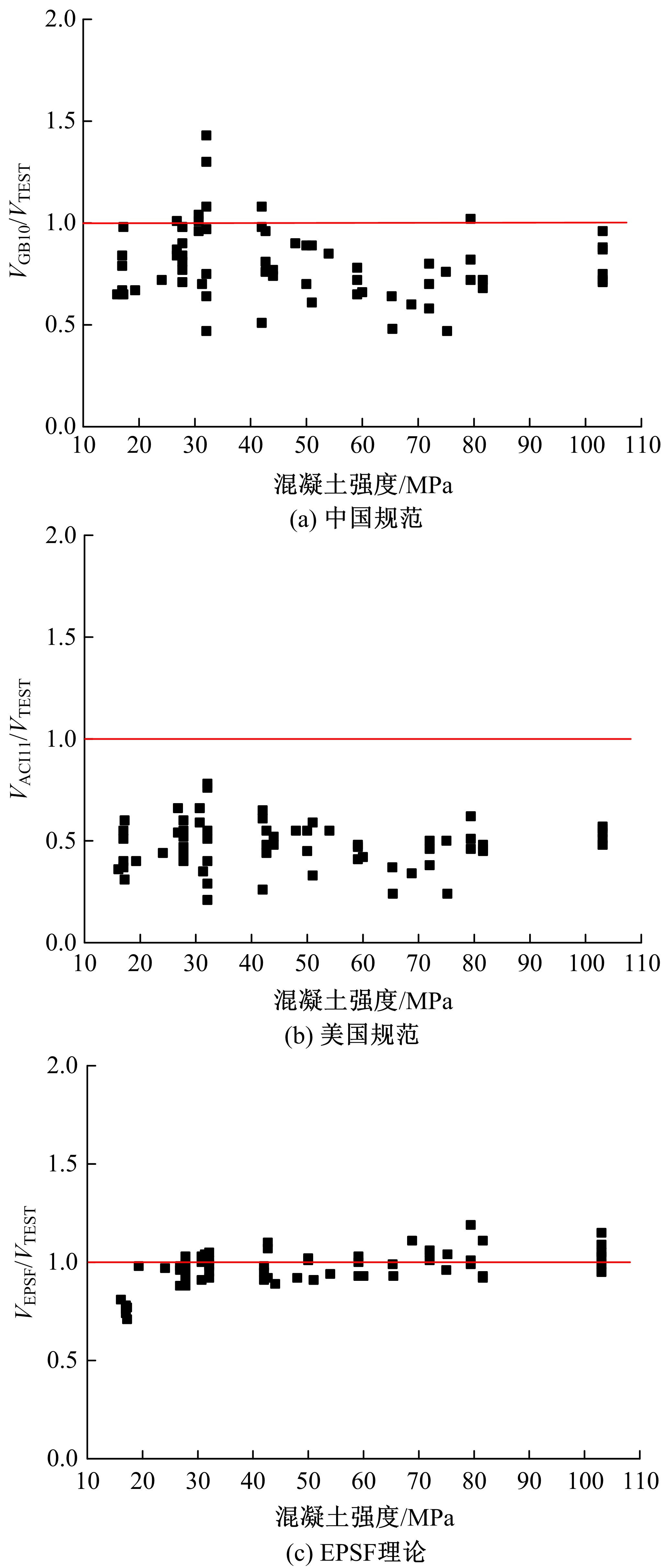
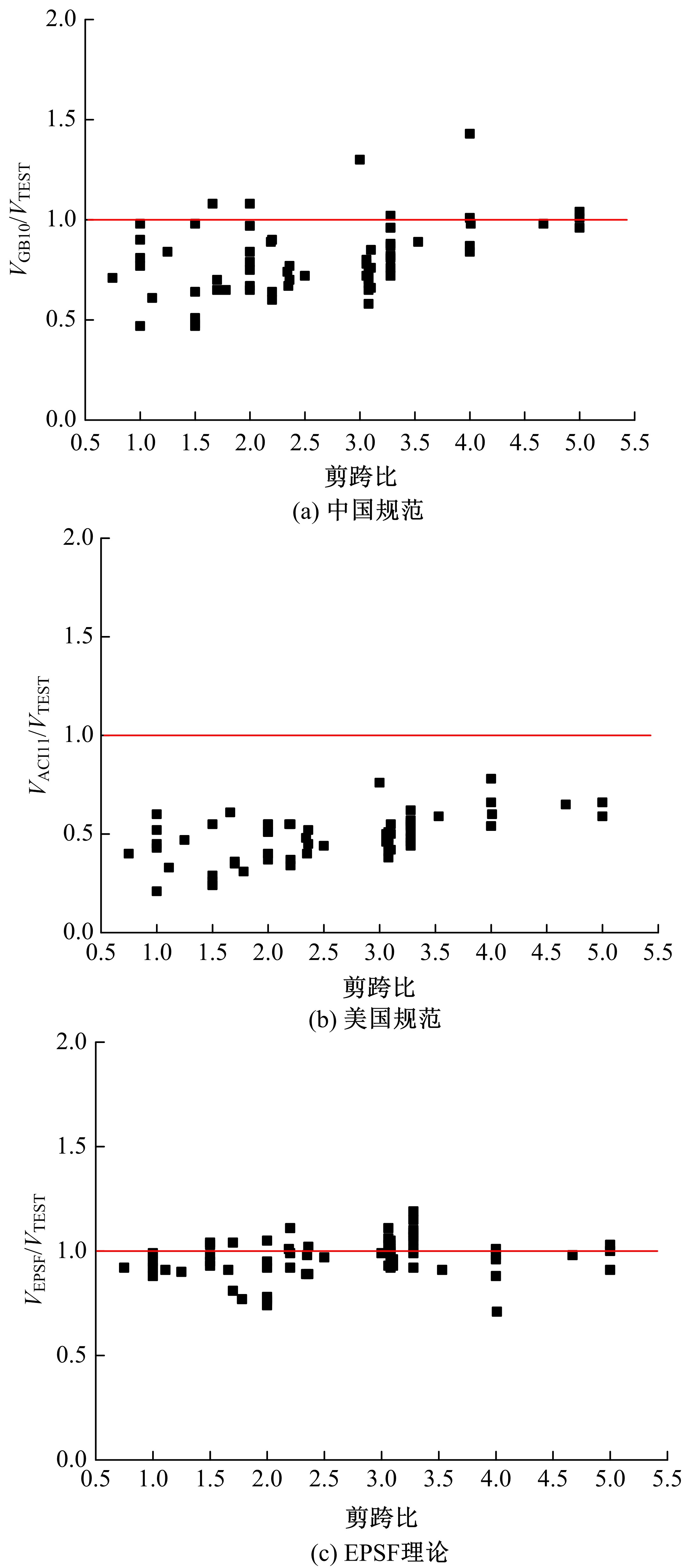
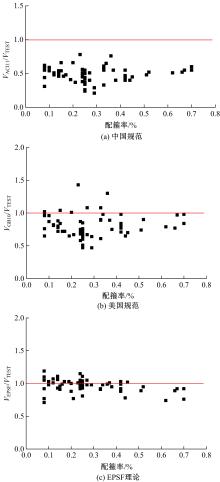
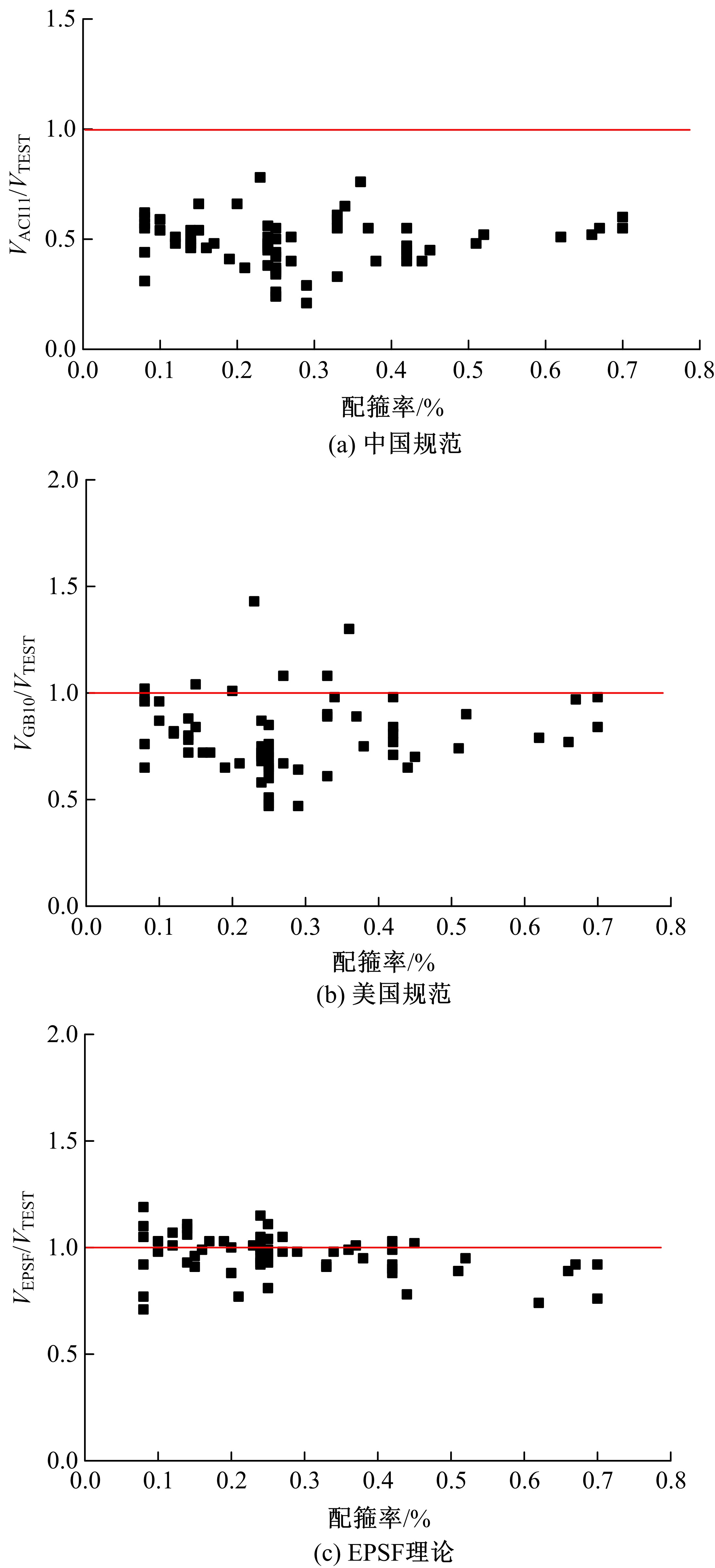
为了研究基于弹塑性应力场理论（EPSF）的钢筋混凝土梁的抗剪性能，采用基于EPSF理论的有限元程序ICONC对混凝土构件进行建模计算，探讨了有限元网格大小、形状和迭代步数对模拟结果的影响；选取12根试验梁，对比基于EPSF理论的模拟结果、试验结果和ABAQUS模拟结果，验证了基于EPSF理论计算结果的准确性。利用中美规范公式和EPSF理论对70根剪切破坏梁的受剪承载力进行了计算，并与试验值进行了对比。结果表明：有限元网格大小、形状和迭代步数对模拟结果影响甚小；程序ICONC可以较准确地模拟钢筋混凝土梁破坏现象、钢筋的屈服和混凝土的裂缝分布；与ABAQUS模拟相比，EPSF理论可以更精确、更方便、更快速地预测钢筋混凝土梁的受剪承载力；混凝土强度、剪跨比、配箍率变化对ICONC程序模拟结果影响较小，本文方法具有一定的准确性和稳定性。

关键词: 结构工程, 钢筋混凝土梁, 弹塑性应力场理论, ICONC程序, 受剪承载力

Abstract:

In order to study the shear behavior of reinforced concrete beams based on the elastoplastic stress field theory （EPSF）， ICONC， a finite element program based on the EPSF theory， was used to model the concrete members. The influences of finite element mesh size， shape and iterative step number on the simulation results were investigated. Twelve test beams were selected. The simulation results based on EPSF theory， experimental results and ABAQUS simulation results were compared to verify the accuracy of the results based on EPSF theory. The shear capacities of 70 beams subject to shear failure were calculated by the formulas in the Chinese and American code， and also calculated by EPSF theory， then those calculated values were compared with the experimental values. The results show that the mesh size， shape and iteration steps have little influence on the simulation results. ICONC， an applicable program based on EPSF theory， can accurately simulate the failure phenomena of reinforced concrete beams， the yield of reinforcement and the crack distributions of concrete. Compared to ABAQUS， EPSF theory can permit an accurate， convenient and rapid prediction of the ultimate shear capacity for reinforced concrete beams. The changes in concrete strength， shear span ratio and stirrup ratio have little impact on ICONC program simulation results. So the proposed method has certain accuracy and stability.

Key words: structural engineering, reinforced concrete beams, elastoplastic stress field theory, ICONC program, shear capacity

中图分类号:

TU375.1

熊二刚,徐涵,谭赐,王婧,丁若愚. 基于弹塑性应力场理论的钢筋混凝土梁受剪承载力[J]. 吉林大学学报(工学版), 2021, 51(1): 259-267.

Er-gang XIONG,Han XU,Ci TAN,Jing WANG,Ruo-yu DING. Shear strength of reinforced concrete beams based on elastoplastic stress field theory[J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(1): 259-267.

图/表 17

图1

图2

表1

表2

图3

表3

表4

试验梁模拟和实测极限承载力"

试件编号	$P T E S T$ /kN	$P E P S F$ /kN	$P F$ /kN	$P E P S F P T E S T$	$P F P T E S T$	破坏形式
E?1.5	360	354	374.63	0.98	1.04	剪压破坏
F?1.0	491	480	561.94	0.98	1.14	斜压破坏
A?2?2	402	394	411	0.98	1.02	弯曲破坏
A'?2?1	406	400	395	0.99	0.97	弯曲破坏
C?2?2	602	610	628	1.01	1.04	弯曲破坏
A?2?1	340	344	411	1.01	1.19	剪切破坏
B?2?1	456	458	548	0.99	1.20	剪切破坏
B?2?2	360	450	548	1.25	1.52	剪切破坏
C?2?1	570	600	628	1.05	1.10	剪切破坏

表4

图4

图5

表5

试验梁模拟和实测极限承载力"

试件编号	P_TEST/kN	P_EPSF/kN	$P E P S F P T E S T$	破坏形式
A2	439	452	1.03	剪切破坏
B2	365	331	0.91	剪切破坏
C2	290	290	1	剪切破坏

表5

图6

表6

各方法得到的极限承载力对比"

梁编号	P_TEST/kN	P_EPSF/kN	P_ABS/kN	$P E P S F P T E S T$	$P A B S P T E S T$
E?1.5	360	354	382.0	0.98	1.06
F?1.0	491	480	554.4	0.98	1.13
A?2?2	402	394	403.9	0.98	1.00
A′?2?1	406	400	430.4	0.99	1.06
C?2?2	602	600	621.2	1.01	1.03
A?2?1	340	344	357.8	1.01	1.05
B?2?1	456	458	531.3	0.99	1.16
B?2?2	360	450	514.4	1.25	1.43
C?2?1	570	600	638.4	1.05	1.12

表6

图7

表7

图8

图9

图10

参考文献 18

1	Niketić F. Development of a consistent approach for design and assessment of structural concrete members using stress fields and strut-and-tie models[D]. Lausanne: EPFL, Switzerland, 2017.
2	Ruiz M F, Muttoni A. On development of suitable stress fields for structural concrete[J]. ACI Structural Journal, 2007, 104(4): 495-502.
3	Vecchio F J, Collins M P. The modified compression-field theory for reinforced concrete elements subjected to shear[J]. ACI Structural Journal, 1986, 83(2): 219-231.
4	Muttoni A, Schwartz J, Thürlimann B. Design of Concrete Structures with Stress Fields[M]. Basel:Birkhäuser Verlag, 1997.
5	Muttoni A. Die Anwendbarkeit der Plastizitätstheorie in der Bemessung von Stahlbeton[M]. Basel:Birkhäuser Verlag, 1990.
6	CEB-FIB. Model code 2010 First final draft –Volumes 1 fib Bulletin 65[Z].
7	Vecchio F J, Shim W. Experimental and analytical investigation of classic concrete beam tests[J]. Journal of Structural Engineering, 2004, 130(3): 460-469.
8	Frey F, Jirousek J. Méthode des éléments finis Analyse des structures et milieux continues[M]. Lausanne, Suisse: Presses Polytechnique et Universitaires Romandes, 2001.
9	赵娜娜. 基于压力路径法钢筋混凝土梁的抗剪试验研究[D]. 西安:长安大学建筑工程学院, 2017.
	Zhao Na-na. Behaviour of reinforced concrete beams for shear in compliance with compressive force path method[D]. Xi'an: School of Architectural Engineering, Chang'an University, 2017.
10	―2010. 混凝土结构设计规范[S].
11	阎昭琦. 基于压力路径法的大尺寸钢筋混凝土梁斜截面抗剪性能研究[D]. 西安:长安大学建筑工程学院, 2018.
	Yan Zhao-qi. Behaviour of big size reinforced concrete beams for shear in compliance with compressive force path method[D]. Xi'an: School of Architectural Engineering, Chang'an University, 2018.
12	易伟建, 吕艳梅. 高强箍筋高强混凝土梁受剪试验研究[J]. 建筑结构学报, 2009, 30(4): 94-101.
	Yi Wei-jian, Lv Yan-mei. Experimental study on shear behavior of high-strength concrete beams with high-strength stirrups[J]. Journal of Building Structures, 2009, 30(4): 94-101.
13	中国建筑科学研究院. 钢筋混凝土构件试验数据集——85年设计规范背景资料续编[M]. 北京:中国建筑工业出版社,1985.
14	Cladera Bohigas A. Shear design of reinforcement high-strength concrete beams[D]. Barcelona: Universitat Politècnica de Catalunya, 2002.
15	Yoon Y S, Cooc W D, Mitchell D. Minimum shear reinforcement in normal, medium and high-strength concrete beams[J]. ACI Structural Journal, 1996, 93(5): 576-584.
16	Hong S G, Kim D J, Kim S Y. Shear strength of reinforced concrete deep beams with end anchorage failure[J]. ACI Structural Journal, 2002, 99(1): 12-22.
17	李娟. HRB500级箍筋混凝土梁斜截面受力性能试验研究[D]. 长沙:湖南大学土木工程学院,2007.
	Li Juan. Experimental Study on mechanical behavior of diagonal section of reinforced concrete beams with HRB500 stirrups[D]. Changsha: School of Civil Engineering, Hunan University, 2007.
18	ACI 318M―14. Building code requirements for structural concrete and commentary[S].

相关文章 13

[1]	樊学平,屈广,刘月飞. 应用新数据同化算法的桥梁极值应力预测[J]. 吉林大学学报(工学版), 2020, 50(2): 572-580.
[2]	杨德磊,童乐为. 支管受轴向受拉工况下CHS-CFSHS T型节点应力集中系数计算公式[J]. 吉林大学学报(工学版), 2019, 49(6): 1891-1899.
[3]	于天来,李海生,黄巍,王思佳. 预应力钢丝绳加固钢筋混凝土梁桥抗剪性能[J]. 吉林大学学报(工学版), 2019, 49(4): 1134-1143.
[4]	戴岩, 聂少锋, 周天华. 带环梁的方钢管约束钢骨混凝土柱-钢梁节点滞回性能有限元分析[J]. 吉林大学学报(工学版), 2018, 48(5): 1426-1435.
[5]	杨昕卉, 薛伟, 郭楠. 钢板增强胶合木梁的抗弯性能[J]. 吉林大学学报(工学版), 2017, 47(2): 468-477.
[6]	于天来, 刘兴国, 姚爽, 穆罕默德马苏. 碳纤维筋体外预应力加固钢筋混凝土梁的疲劳性能[J]. 吉林大学学报(工学版), 2016, 46(6): 1867-1873.
[7]	王少杰, 徐赵东, 李舒, 王凯洋，Dyke Shirley J. 基于应变监测的连续梁支承差异沉降识别[J]. 吉林大学学报(工学版), 2016, 46(4): 1090-1096.
[8]	苏迎社, 杨媛媛. 高温对建筑混凝土材料抗震抗压的作用及原理[J]. 吉林大学学报(工学版), 2015, 45(5): 1436-1442.
[9]	宿晓萍，王清. 复合盐浸-冻融-干湿多因素作用下的混凝土腐蚀破坏[J]. 吉林大学学报(工学版), 2015, 45(1): 112-120.
[10]	郭俊平¹, 邓宗才¹, 卢海波², 林劲松². 预应力高强钢绞线网抗剪加固钢筋混凝土梁试验[J]. 吉林大学学报(工学版), 2014, 44(4): 968-977.
[11]	姜浩, 郭学东. 基于地震激励的混凝土桥梁模态参数识别[J]. 吉林大学学报(工学版), 2011, 41(增刊2): 185-188.
[12]	孙绪杰，潘景龙，郑文忠 . 玻璃纤维增强聚合物混凝土小型空心砌块复合墙片的抗震性能[J]. 吉林大学学报(工学版), 2008, 38(05): 1054-1059.
[13]	李春良，程永春 . 碳纤维布加固钢筋混凝土梁的预应力控制过程[J]. 吉林大学学报(工学版), 2008, 38(02): 393-0398.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

极限承载力/（kN·m^-1）	M₁	M₂	M₃	M₄
对称配筋均布压应力	3564	3564	3564	3564
对称配筋均布拉应力	182	182	182	182
对称配筋均布剪应力	179	179	179	179
非对称配筋均布剪应力	129	129	129	129

极限承载力/kN	ρ_w=0.20%(b_w=150)	ρ_w=0.31%(b_w=100)	ρ_w=0.61%(b_w=50)	ρ_w=1.02%(b_w=30)
M₁	266	224	145	97
M₂	262	221	141	94
M₃	262	218	136	90
M₄	261	215	134	89

网格类型	极限承载力	网格类型	极限承载力
M_DR	266	M_D3	261
M_D1	270	M_D4	255
M_D2	264

参数	EPSF理论	中国规范	美国规范
均值	0.96	0.80	0.48
变异系数	0.09	0.23	0.25
标准差	0.09	0.18	0.12