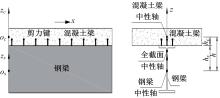
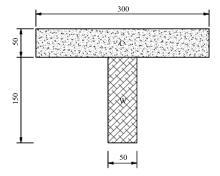
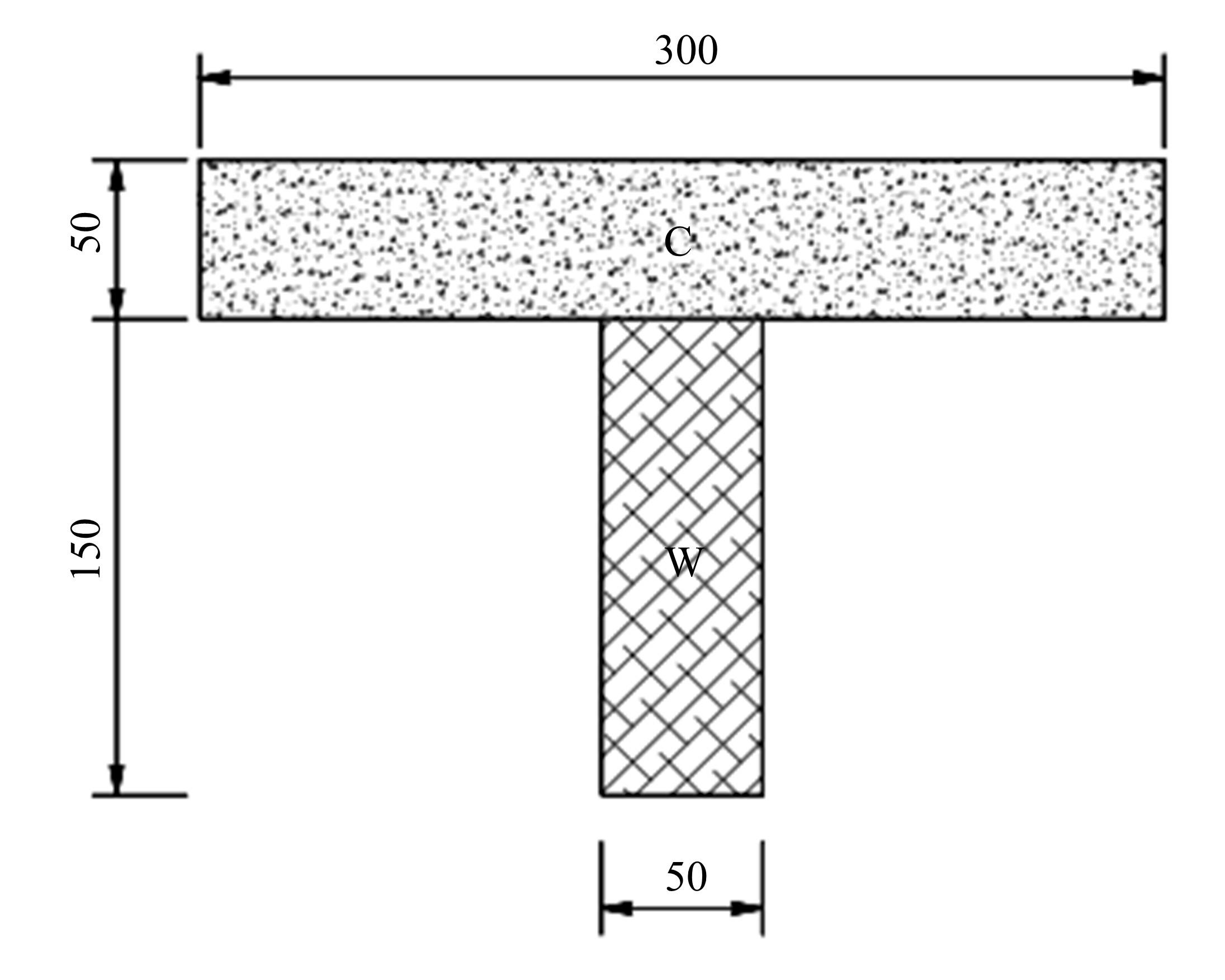
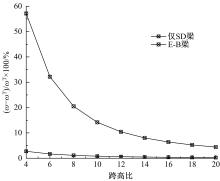
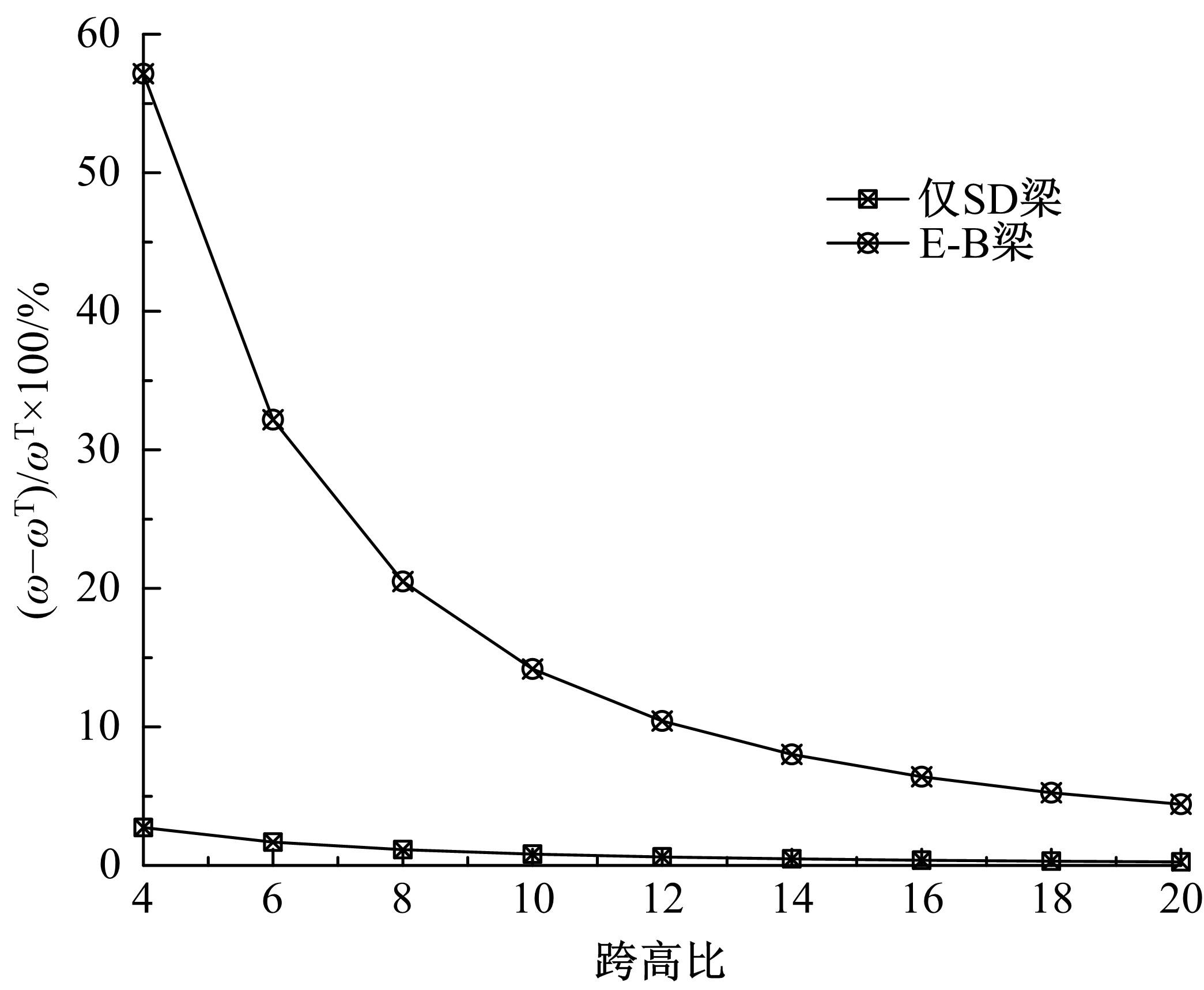
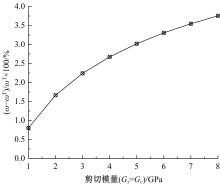
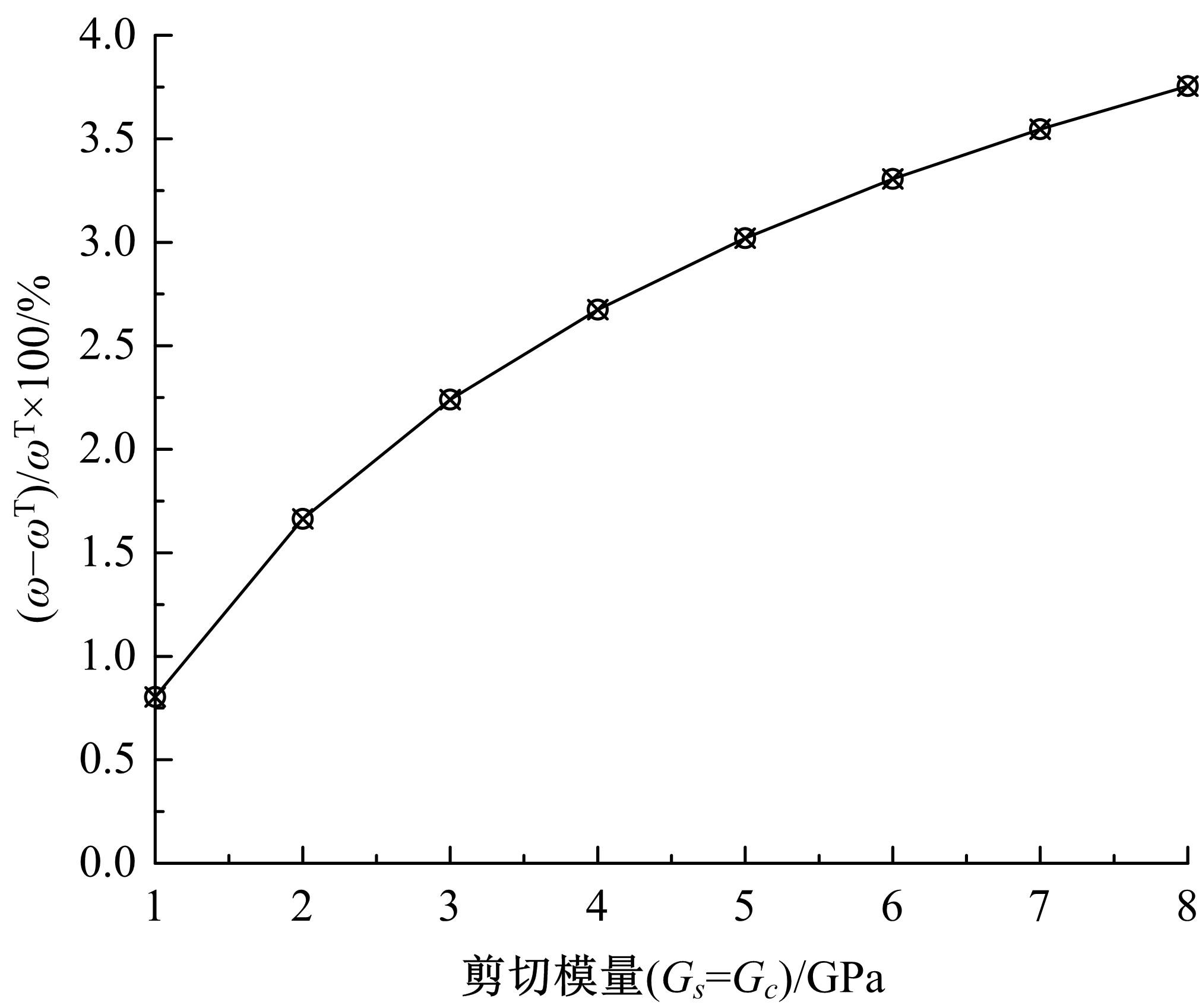
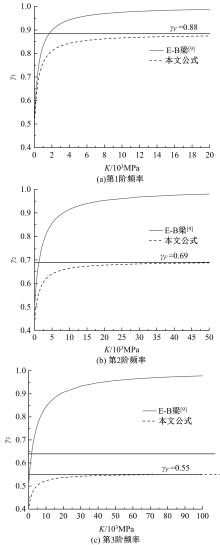
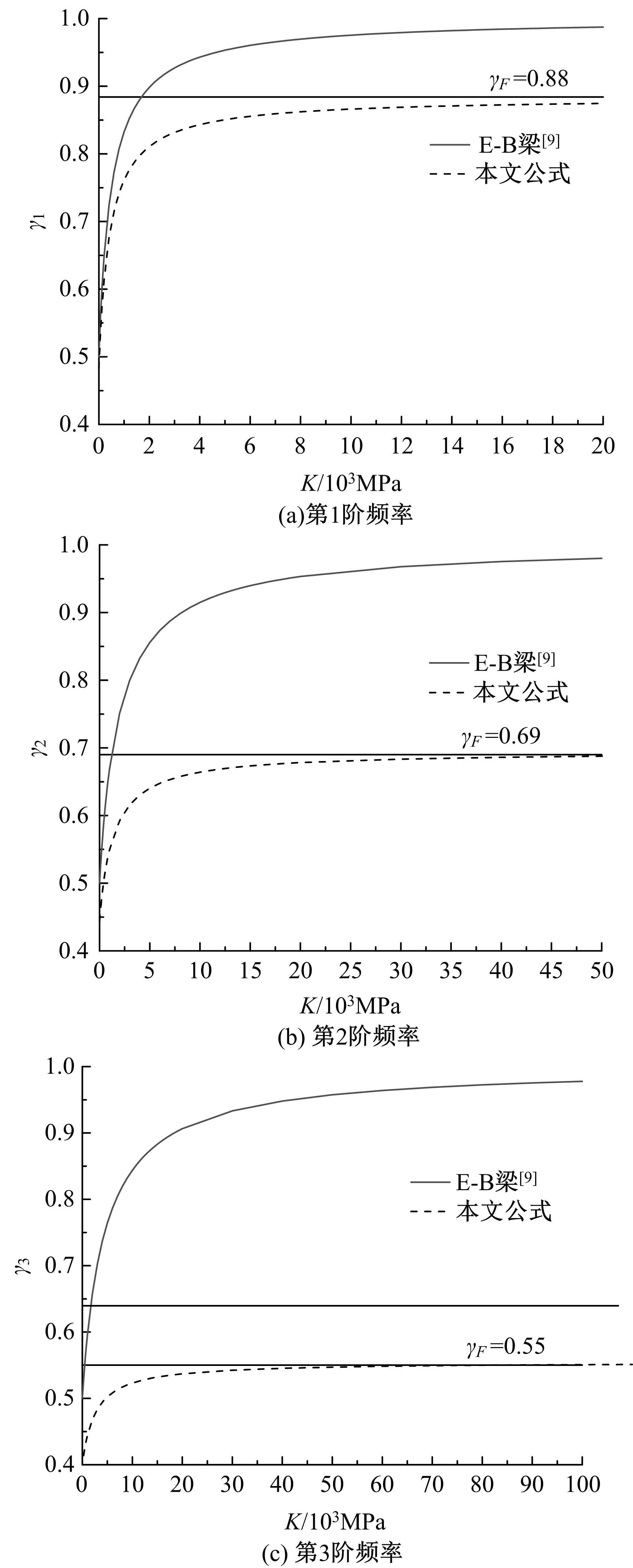
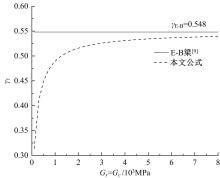
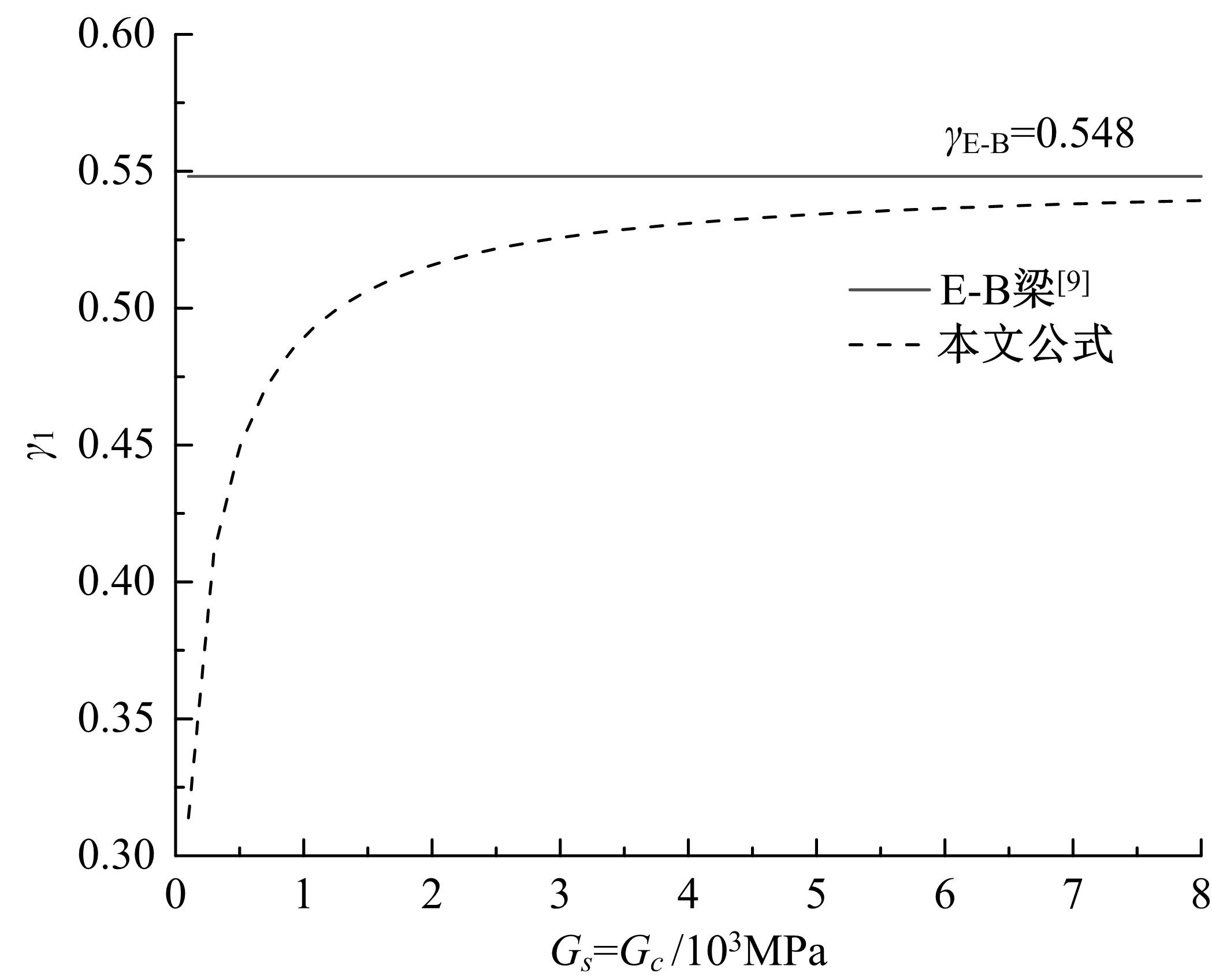
Journal of Jilin University(Engineering and Technology Edition) ›› 2023, Vol. 53 ›› Issue (2): 488-495.doi: 10.13229/j.cnki.jdxbgxb20210742
Previous Articles Next Articles
Dynamic reduction coefficients of steel⁃concrete composite beam based on Timoshenko beam theory
Qi-kai SUN( ),Nan ZHANG(
),Nan ZHANG( ),Xiao LIU,Zi-ji ZHOU
),Xiao LIU,Zi-ji ZHOU
- School of Civil Engineering,Beijing Jiaotong University,Beijing 100044,China
CLC Number:
- U441
| 1 | 聂建国,余志武. 钢-混凝土组合梁在我国的研究及应用[J]. 土木工程学报, 1999, 32(2): 3-8. |
| Nie Jian-guo, Yu Zhi-wu. Research and practice of composite steel-concrete beams in China[J]. China Civil Engineering Journal, 1999, 32(2): 3-8. | |
| 2 | 聂建国, 陶慕轩, 吴丽丽, 等. 钢-混凝土组合结构桥梁研究新进展[J]. 土木工程学报, 2012, 45(6): 110-122. |
| Nie Jian-guo, Tao Mu-xuan, Wu Li-li,et al.Advances of research on steel-concrete composite bridges[J]. China Civil Engineering Journal, 2012, 45(6): 110-122. | |
| 3 | 刘永健,刘江.钢-混凝土组合梁桥温度作用与效应综述[J]. 交通运输工程学报, 2020, 20(1): 42-59. |
| Liu Yong-jian, Liu Jiang. Review on temperature action and effect of steel-concrete composite girder bridge[J]. Journal of Traffic and Transportation Engineering, 2020, 20(1): 42-59. | |
| 4 | 聂建国,沈聚敏,余志武. 考虑滑移效应的钢-混凝土组合梁变形计算的折减刚度法[J]. 土木工程学报, 1995, 28(6): 11-17. |
| Nie Jian-guo, Shen Ju-min, Yu Zhi-wu. A reduced rigidity method for calculating deformation of composite steel-concrete beams[J]. China Civil Engineering Journal, 1995, 28(6): 11-17. | |
| 5 | 王景全,吕志涛,刘钊. 部分剪力连接钢-混凝土组合梁变形计算的组合系数法[J]. 东南大学学报: 自然科学版, 2005, 35(): 5-10. |
| Wang Jing-quan, Lv Zhi-tao, Liu Zhao. Consistency factor method for calculating deformation of composite steel-concrete girders with partial shear connection[J]. Journal of Southeast University (Natural Science Edition), 2005, 35(S1): 5-10. | |
| 6 | 徐荣桥,陈德权.组合梁挠度计算的改进折减刚度法[J]. 工程力学,2013, 30(2): 285-291. |
| Xu Rong-qiao, Chen De-quan. Modified reduced stiffness method for calculating the deflection of composite beams[J]. Engineering Mechanics, 2013, 30(2): 285-291. | |
| 7 | 张云龙, 刘占莹, 吴春利, 等. 钢-混凝土组合梁静动力响应[J]. 吉林大学学报: 工学版, 2017, 47(3): 789-795. |
| Zhang Yun-long, Liu Zhan-ying, Wu Chun-li,et al. Static and dynamic responses of steel-concrete composite beams[J]. Journal of Jilin University (Engineering and Technology Edition), 2017, 47(3): 789-795. | |
| 8 | 侯忠明,夏禾,张彦玲. 栓钉连接件抗剪刚度对钢-混凝土结合梁自振特性影响研究[J].中国铁道科学, 2012, 33(6): 24-29. |
| Hou Zhong-ming, Xia He, Zhang Yan-ling. The influence of the shear stiffness of stud connectors on the natural vibration characteristics of steel-concrete composite beams[J]. China Railway Science, 2012, 33(6): 24-29. | |
| 9 | 侯忠明,夏禾,王元清,等. 钢-混凝土组合梁动力折减系数研究[J]. 振动与冲击, 2015, 34(4): 74-81. |
| Hou Zhong-ming, Xia He, Wang Yuan-qing, et al. Dynamic redution coefficients for a steel-concrete composite beam[J]. Journal of Vibration and Shock, 2015, 34(4): 74-81. | |
| 10 | 张云龙, 郭阳阳, 王静, 等. 钢-混组合梁的固有频率及其振型[J]. 吉林大学学报: 工学版, 2020, 50(2): 581-588. |
| Zhang Yun-long, Guo Yang-yang, Wang Jing,et al. Natural frequency and mode of vibration of steel-concrete composite beams[J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 581-588. | |
| 11 | 孙琪凯, 张楠, 刘潇, 等. 基于能量法分析考虑纵向刚度分布的钢-混组合梁自振特性[J]. 振动与冲击, 2021, 40(10): 67-72. |
| Sun Qi-kai, Zhang Nan, Liu Xiao, et al. Free vibration characteristics of steel-concrete composite beams considering longitudinal stiffness distribution based on energy method[J]. Journal of Vibration and Shock, 2021, 40(10): 67-72. | |
| 12 | Sun Q K, Zhang N, Liu X, et al. Free vibrations of steel-concrete composite beams by the dynamic direct stiffness method[J]. International Journal of Structural Stability and Dynamic, 2021, 21(4): 2150049. |
| 13 | Berczyński S, Wróblewski T. Vibration of steel-concrete composite beams using the Timoshenko beam model[J]. Journal of Vibration and Control, 2005, 11(6): 829-848. |
| 14 | Xu R Q, Wu Y F. Static dynamic and buckling analysis of partial interaction composite members using Timoshenko's beam theory[J]. International Journal of Mechanical Sciences, 2007, 49(10): 1139-1155. |
| 15 | Nguyen Q H, Hjiaj M, Grognec P L. Analytical approach for free vibration analysis of two-layer Timoshenko beams with interlayer slip[J]. Journal of Sound and Vibration, 2012, 331(12): 2949-2961. |
| 16 | Lin J P, Wang G N, Bao G J, et al. Stiffness matrix for the analysis and design of partial-interaction composite beams[J]. Construction and Building Materials, 2017, 156: 761-772. |
| 17 | Lin J P, Wang G N, Xu R Q. Variational principles and explicit finite-element formulations for the dynamic analysis of partial-interaction composite beams[J]. Journal of Engineering Mechanics-ASCE, 2020, 146(6): 04020055. |
| 18 | Sun Q K, Zhang N, Liu X, et al. An equivalent single-layer theory for free vibration analysis of steel-concrete composite beams[J]. Steel and Composite Structures, 2021, 38(3): 281-291. |
| [1] | Ya-chuan KUANG,Li-bin CHEN,Chao-ju LI,Yu-hao HE. Analysis of mechanical properties of stud shear connectors [J]. Journal of Jilin University(Engineering and Technology Edition), 2023, 53(2): 538-546. |
| [2] | Hua-wen YE,Zhi-chao DUAN,Ji-lin LIU,Yu ZHOU,Bing HAN. Wheel⁃load diffusion effect on orthotropic steel⁃concrete composite bridge deck [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(8): 1808-1816. |
| [3] | Li-feng WANG,Zi-wang XIAO,Sai-sai YU. New risk analysis method based on Bayesian network for hanging basker system of multi-tower cable-stayed bridge [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(4): 865-873. |
| [4] | Yan-ling ZHANG,Can WANG,Xu ZHANG,Ang-yang WANG,Yun-sheng LI. Human⁃induced vibration analysis and pedestrian comfort evaluation for suspension footbridge with different hunger systems [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(11): 2644-2652. |
| [5] | Chang-jun ZHONG,Zhong-bin WANG,Chen-yang LIU. Influencing factors and structural optimization of main cable saddle bearing capacity of suspension bridge [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(6): 2068-2078. |
| [6] | Wei CHEN,Tian-bao WAN,Zhong-bin WANG,Xuan LI,Rui-li SHEN. Design and performance of internal air supply conduit for dehumidification in main cables of suspension bridges [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(5): 1749-1755. |
| [7] | Shu-lun GUO,Tie-yi ZHONG,Zhi-gang YAN. Calculation method of buffeting response for stay cables of long⁃span cable⁃stayed bridge [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(5): 1756-1762. |
| [8] | Kai GAO,Gang LIU. Effective strength improvement of global critical strength branch and bound method [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(2): 597-603. |
| [9] | Ya-feng GONG,Jia-xiang SONG,Guo-jin TAN,Hai-peng BI,Yang LIU,Cheng-xin SHAN. Multi⁃vehicle bridge weigh⁃in⁃motion algorithm [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(2): 583-596. |
| [10] | Qing-wen KONG,Guo-jin TAN,Long-lin WANG,Yong WANG,Zhi-gang WEI,Han-bing LIU. Analysis of free vibration characteristics of cracked box girder bridge based on finite element method [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(1): 225-232. |
| [11] | Hua CHEN,Yao-jia CHEN,Bin XIE,Peng-kai WANG,Lang-ni DENG. Interface failure mechanism and bonding strength calculation of CFRP tendons bonded anchorage system [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(5): 1698-1708. |
| [12] | Ya-feng GONG,Jia-xiang SONG,Hai-peng BI,Guo-jin TAN,Guo-hai HU,Si-yuan LIN. Static test and finite element analysis of scale model of fabricated box culvert [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(5): 1728-1738. |
| [13] | Hao GAO,Jun-jie WANG,Hui-jie LIU,Jian-ming WANG. Design criterion and applied devices for controlled seismic behavior of continuous girder bridges [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(5): 1718-1727. |
| [14] | Qian-hui PU,Jing-wen LIU,Gang-yun ZHAO,Meng YAN,Xiao-bin LI. Theoretical analysis of bearing capacity of concrete eccentric compressive column reinforced by HTRCS [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 606-612. |
| [15] | Yun-long ZHANG,Yang-yang GUO,Jing WANG,Dong LIANG. Natural frequency and mode of vibration of steel⁃concrete composite beam [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 581-588. |
|