路堤挡土墙主动土压力计算方法修正

doi:10.13229/j.cnki.jdxbgxb20210013

摘要/Abstract

摘要：

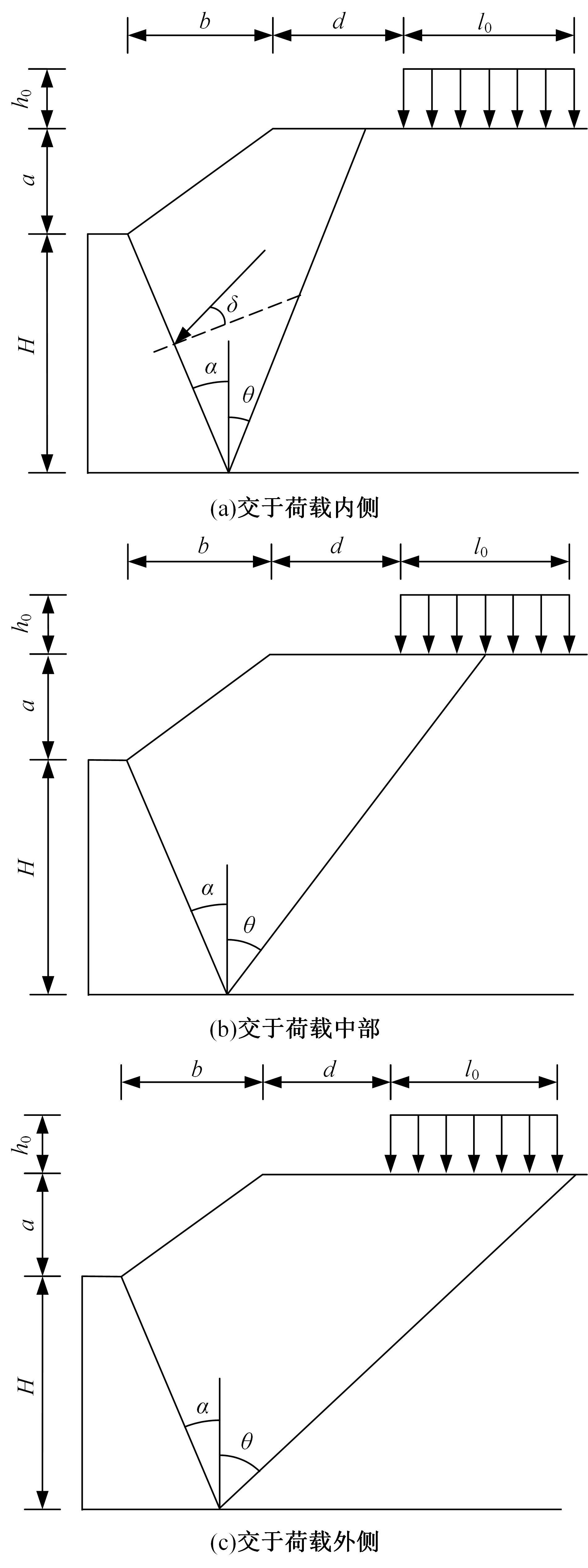
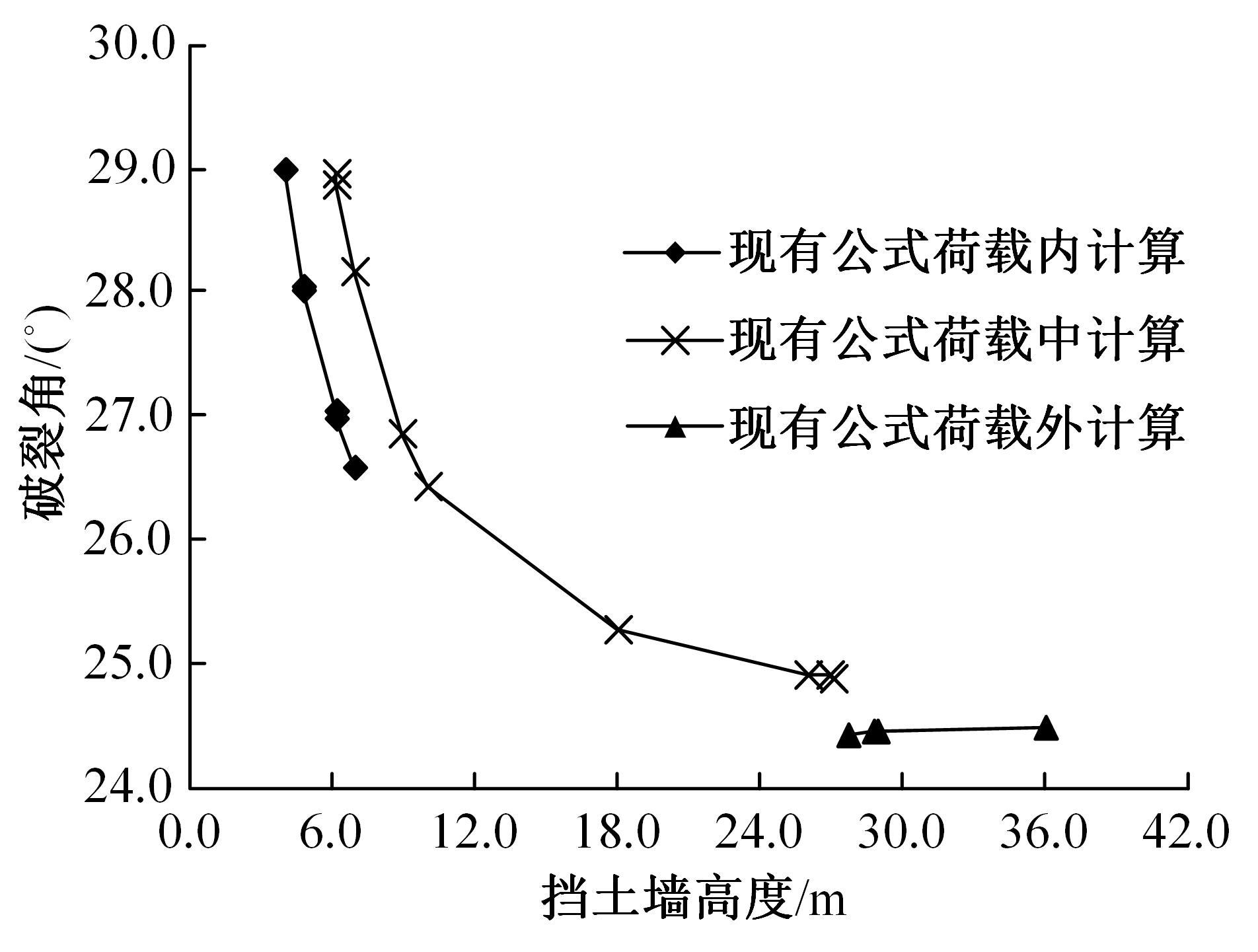
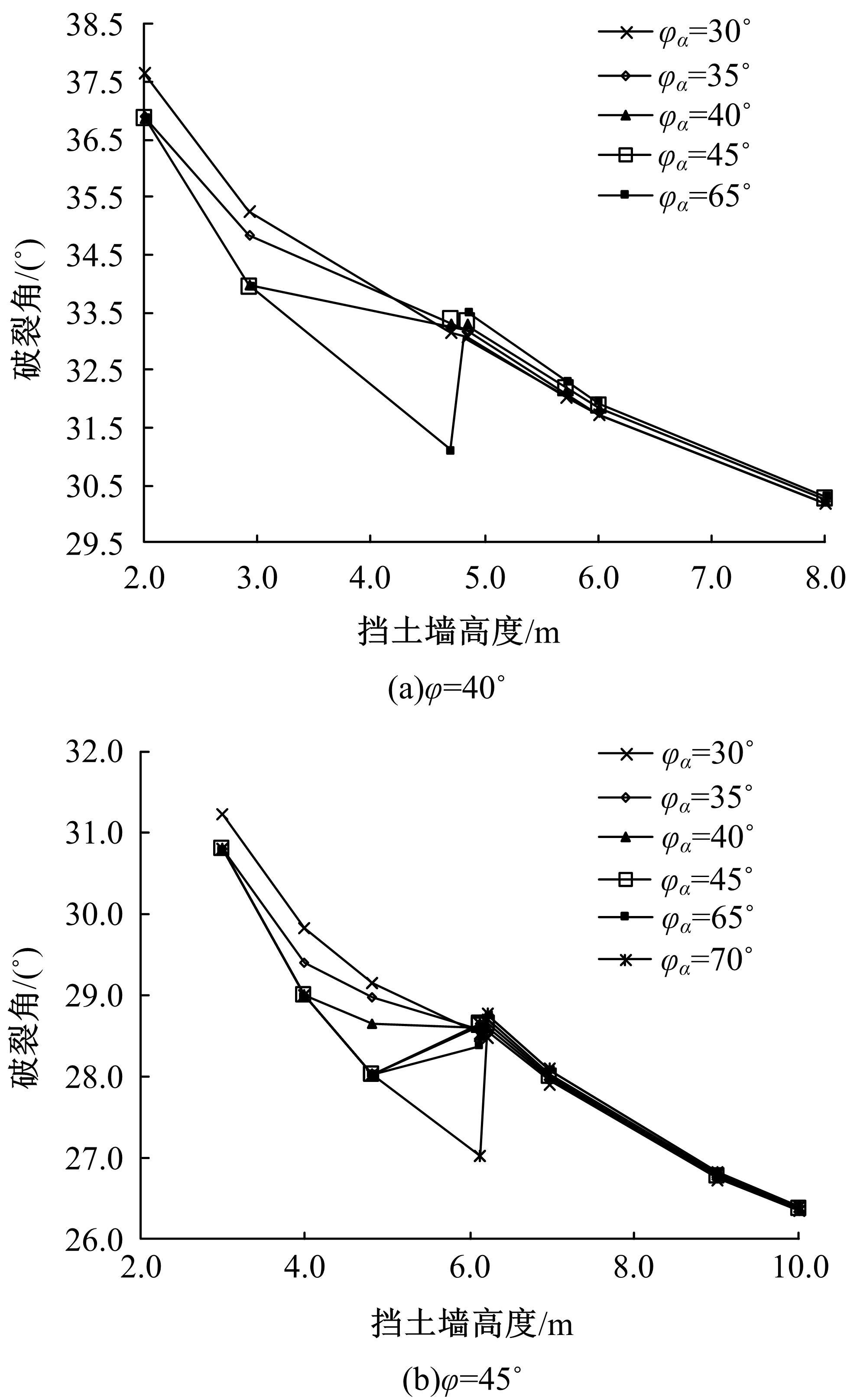
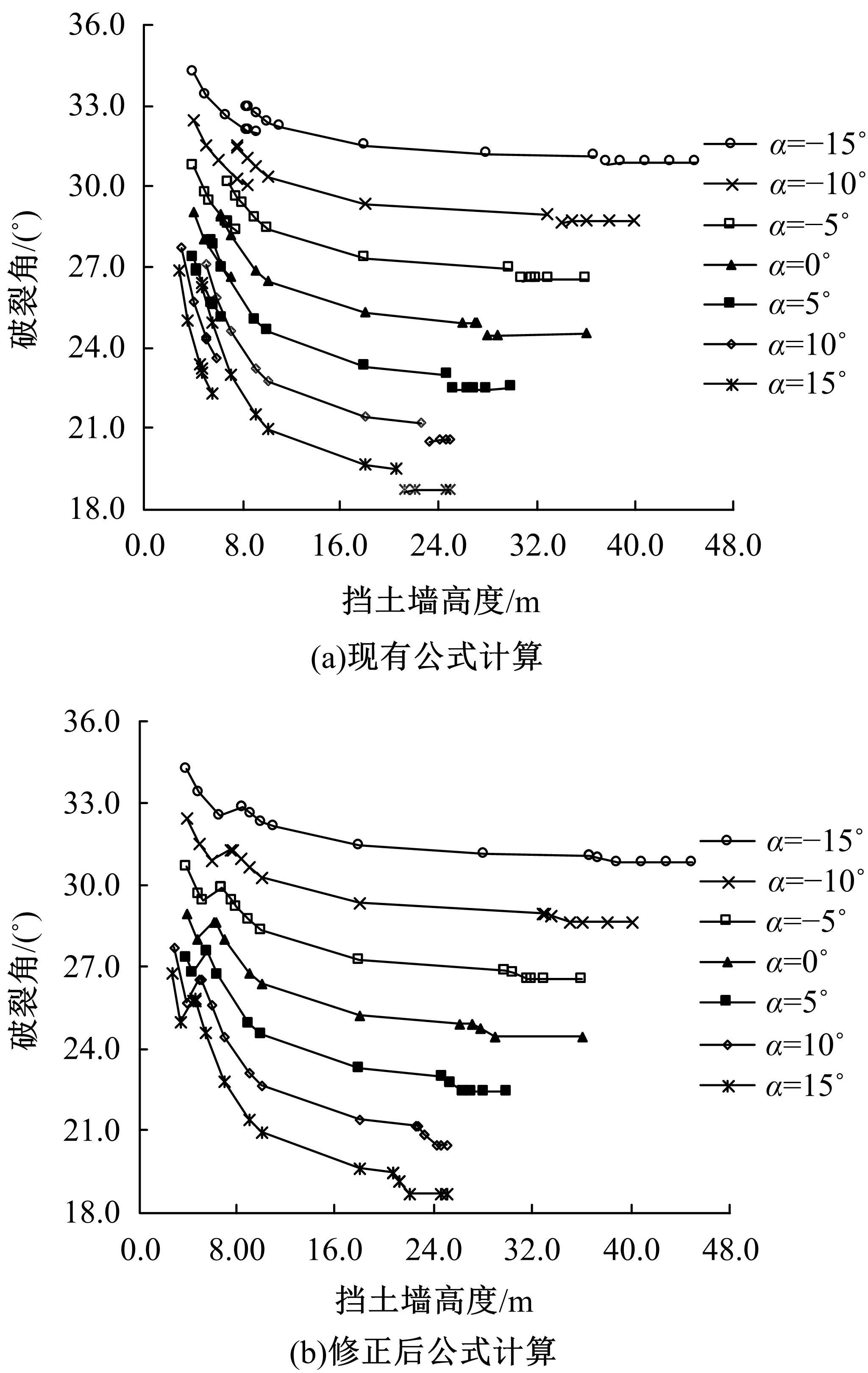
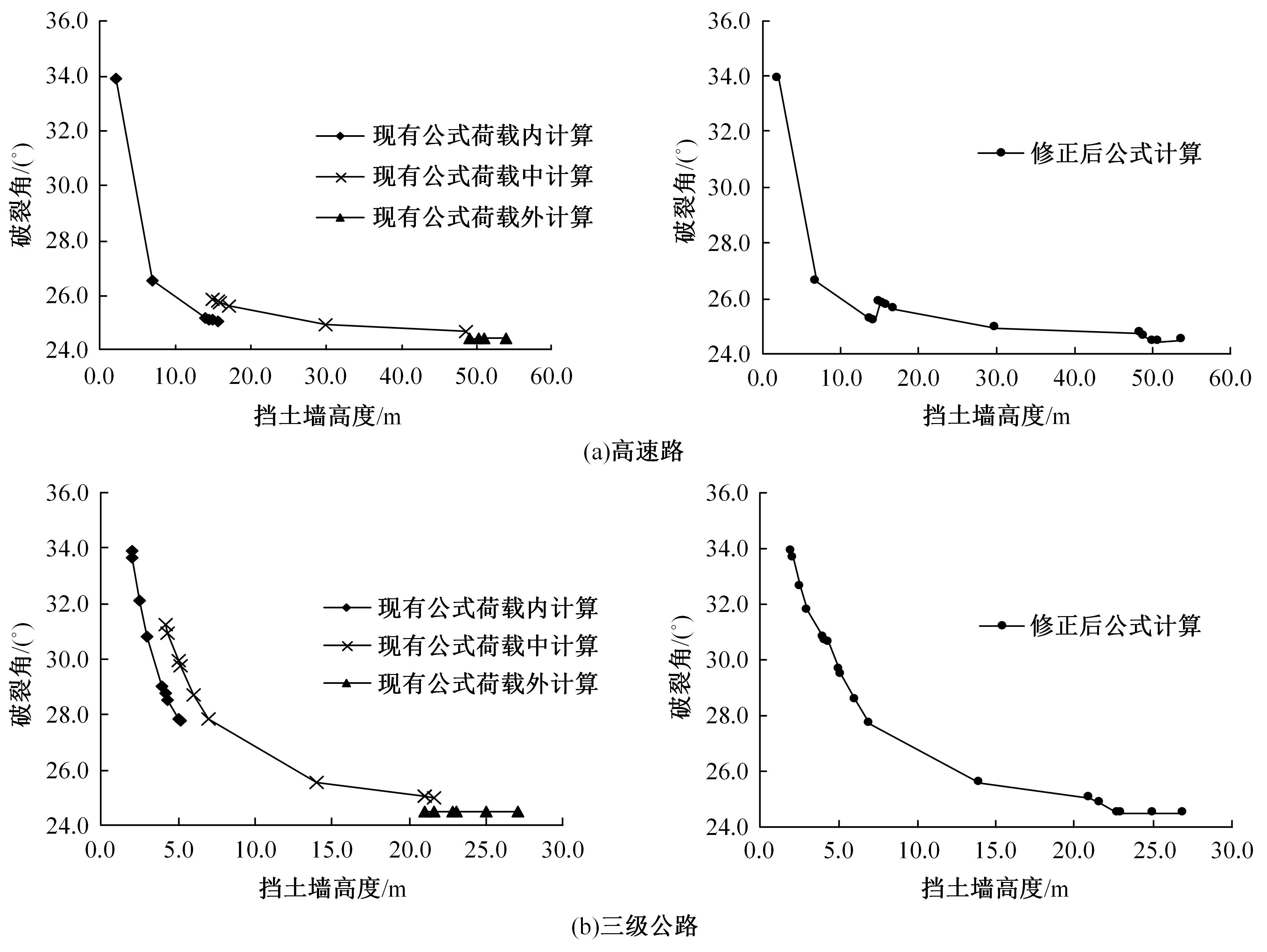
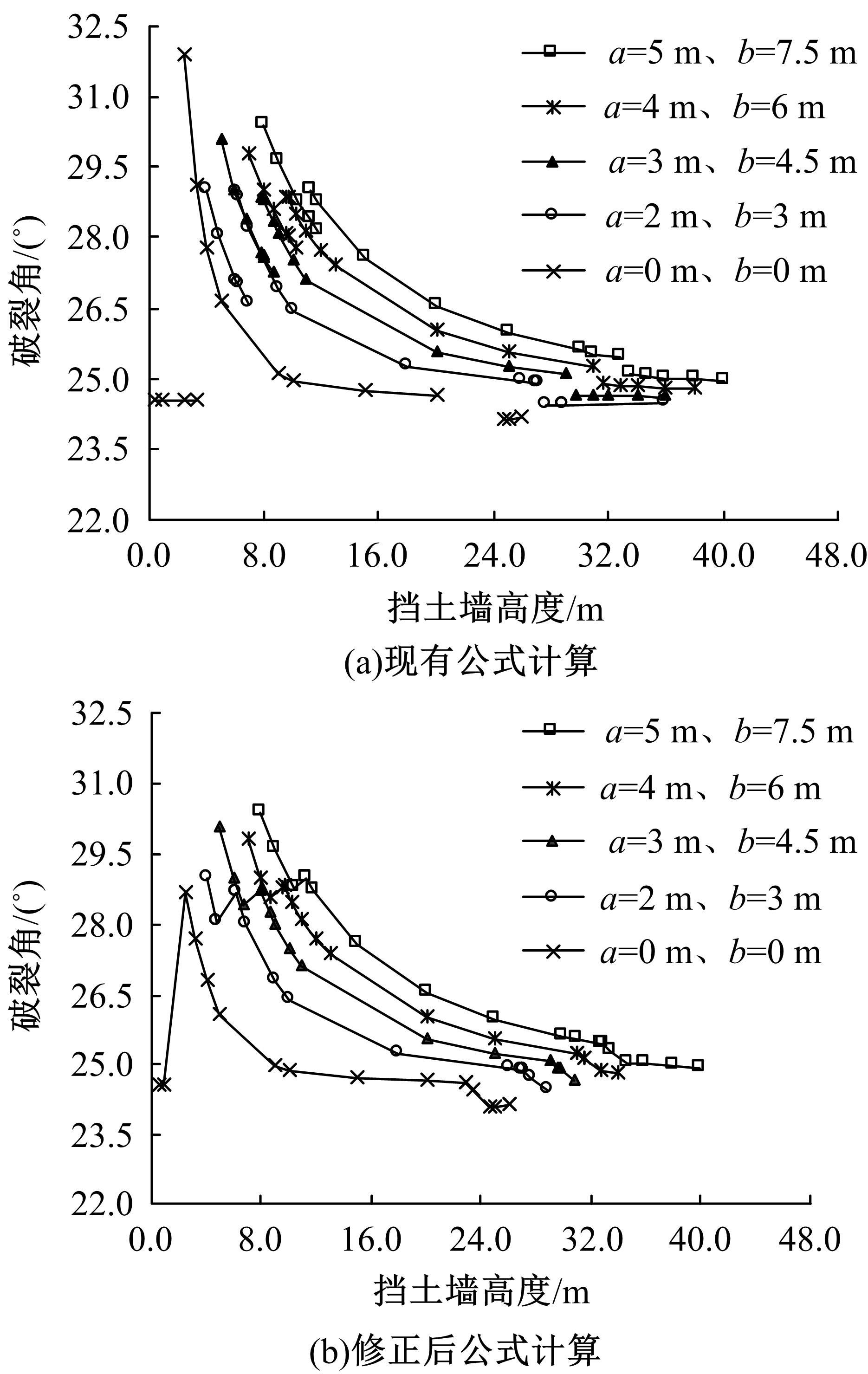
现有路堤挡土墙稳定性计算方法，均先假定墙后土体破裂面的位置，按照对应的公式计算出破裂角θ，再与假定的破裂角对比以确定计算结果。当挡土墙高度 $H$ 变化时，应分别采用破裂面交于荷载外侧、内侧、中部的计算公式，所得θ值在高度的某范围内不唯一或无解，造成假设不成立，无法判断破裂面的具体位置，也就无法计算挡墙的最大主动土压力。针对上述问题，本文提出将挡土墙上的车辆荷载换算为土柱时由矩形荷载改为梯形荷载，使之更接近墙后土体性质，并推导出新的破裂角计算公式。分析了梯形荷载与水平线夹角 $φ α$ 的取值范围，使修改后的公式更具普遍性和适用性。通过改变路基宽度、墙背倾斜角度、边坡高度、坡率等路基参数，经过与规范计算方法对比和实际案例分析，表明采用修正后的计算公式判断破裂面的位置时具有唯一解，验证了修正后公式的正确性和合理性。

关键词: 道路工程, 路堤挡土墙, 修正后破裂角, 稳定性计算

Abstract:

In the existing calculation methods of embankment retaining wall stability， the position of soil fracture surface behind the wall is assumed first， and the fracture angle θ is calculated according to the corresponding formula， then compare it with the assumed rupture angle to determine the calculation results.When the height H of the retaining wall changes， the calculation formula that the fracture surface intersects the outside， inside and middle of the load should be adopted respectively， and the obtained θ value is not unique or has no solution within a certain range of height， the hypothesis is not tenable， the specific position of the fracture surface cannot be judged， and the maximum active earth pressure of the retaining wall cannot be calculated. In view of the above problems， this paper proposes to change the vehicle load on the retaining wall from rectangular load to trapezoidal load when converting it into soil column， so as to make it closer to the nature of the soil behind the wall， and deduces a new formula for calculating the fracture angle. The value range of the angle $φ α$ between the trapezoidal load and the horizontal line is analyzed to make the revised formula more universal and applicable. By changing the subgrade parameters such as subgrade width， wall back inclination angle， slope height and slope rate， through comparison with the standard calculation method and actual case analysis， it is shown that the modified calculation formula has a unique solution to judge the position of the fracture surface， and the correctness and rationality of the modified formula are verified.

Key words: road engineering, embankment retaining wall, corrected fracture angle, stability calculation

中图分类号:

U416

时成林,王勇,吴春利,宋文祝. 路堤挡土墙主动土压力计算方法修正[J]. 吉林大学学报(工学版), 2022, 52(6): 1394-1403.

Cheng-lin SHI,Yong WANG,Chun-li WU,Wen-zhu SONG. Modification of calculation method for active earth pressure on embankment retaining wall[J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(6): 1394-1403.

图/表 12

图1

图2

表1

表2

图3

图4

图5

图6

图7

图8

图9

表3

参考文献 19

1	薛殿基,冯仲林. 挡土墙设计实用手册[M]. 北京: 中国建筑工业出版社,2008.
2	彭明祥. 挡土墙主动土压力的库仑统一解[J]. 岩土力学, 2009, 30(2): 379-386.
	Peng Ming-xiang. Coulumb's unified solution of active earth pressure on retaining wall[J]. Rock and Soil Mechanics, 2009, 30(2): 379-386.
3	顾慰慈. 挡土墙土压力计算手册[M]. 北京: 中国建材工业出版社, 2005.
4	田恒银. 挡土墙主动土压力计算的研究[D]. 重庆:重庆大学土木工程学院, 2016.
	Tian Heng-yin. Study on calculation of active earth pressure on retaining wall[D]. Chongqing: College of Civil Engineering, Chongqing University, 2016.
5	Fathipour H, Siahmazgi A S, Payan M, et al. Limit analysis of modified pseudodynamic lateral earth pressure in anisotropic frictional medium using finite-element and second-order cone programming[J/OL]. [2021-01-05].
6	Rao P P, Chen Q S, Zhou Y T, et al. Determination of active earth pressure on rigid retaining wall considering arching effect in cohesive backfill soil[J]. International Journal of Geomechanics, 2016, 16(3): 1-9.
7	刘洋, 于鹏强. 刚性挡土墙平移模式的土拱形状与主动土压力分析[J].岩土力学, 2019, 40(2): 506-516, 528.
	Liu Yang, Yu Peng-qiang. Analysis of soil arch shape and active earth pressure in translation mode of rigid retaining wall[J]. Rock and Soil Mechanics, 2019, 40(2): 506-516, 528.
8	谢明星, 郑俊杰, 曹文昭, 等.有限填土路堤挡土墙主动土压力研究[J].华中科技大学学报:自然科学版, 2019, 47(2): 1-6.
	Xie Ming-xing, Zheng Jun-jie, Cao Wen-zhao, et al. Research on active earth pressure of retaining wall of limited fill embankment[J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2019, 47(2): 1-6.
9	谢清泉, 刘昌清, 王卉, 等. 悬臂式挡土墙土压力计算方法对比研究[J]. 路基工程, 2020(5): 110-114, 125.
	Xie Qing-quan, Liu Chang-qing, Wang Hui, et al. Comparative study on earth pressure calculation methods of cantilever retaining wall[J]. Subgrade Engineering, 2020(5):110-114, 125.
10	王闫超, 晏鄂川, 陆文博, 等. 无黏性有限土体主动土压力解析解[J]. 岩土力学, 2016, 37(9): 2513-2520.
	Wang Yan-chao, Yan E-chuan, Lu Wen-bo, et al. Analytical solution for active earth pressure of non-cohesive finite soil[J]. Rock and Soil Mechanics, 2016, 37(9): 2513-2520.
11	顾嵋杰. 基于不同破裂面类型的挡土墙土压力的分析计算[J]. 西北水电, 2020(4):52-56.
	Gu Mei-jie. Analysis and calculation of earth pressure on retaining wall based on different fracture surface types[J]. Northwest Hydropower, 2020(4):52-56.
12	竺明星, 姜开渝, 龚维明, 等. 考虑c-φ回填土坡面倾角影响的挡土墙非线性主动土压力研究[J]. 天津大学学报, 2019, 52(): 70-75.
	Zhu Ming-xing, Jiang Kai-yu, Gong Wei-ming, et al. Study on nonlinear active earth pressure of retaining wall considering the influence of slope angle of c-φ backfill [J]. Journal of Tianjin University, 2019, 52(Sup.1): 70-75.
13	黄旺, 杨建军, 黄娟. 几种挡土墙主动土压力理论对比及墙体应力分析[J]. 长沙理工大学学报: 自然科学版, 2017, 14(3): 29-34.
	Huang Wang, Yang Jian-jun, Huang Juan. Theoretical comparison and wall stress analysis of several retaining walls[J]. Journal of Changsha University of science and Technology(Natural Science Edition), 2017, 14(3): 29-34.
14	张东卿, 薛元, 罗强, 等. 中欧(法)挡土墙设计规范对比研究[J]. 铁道工程学报, 2020, 37(2): 30-34, 39.
	Zhang Dong-qing, Xue Yuan, Luo Qiang, et al. Comparative study of China-Europe (French) retaining wall design code[J]. Journal of Railway Engineering Society, 2020, 37(2): 30-34, 39.
15	柯才桐, 陈奕柏, 朱嘉. 挡土墙土压力线性分布解[J]. 岩石力学与工程学, 2014, 33():3312-3317.
	Ke Cai-tong, Chen Yi-bo, Zhu Jia. Linear distribution solution of earth pressure on retaining wall[J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(Sup.1):3312-3317.
16	党发宁, 张乐, 王旭, 等. 基于弹性理论的有限位移条件下挡土墙上土压力解析[J]. 岩石力学与工程学报, 2020, 39(10): 2094-2103.
	Dang Fa-ning, Zhang Le, Wang Xu, et al. Analysis of earth pressure on retaining wall under limited displacement conditions based on elastic theory[J]. Chinese Journal of Rock Mechanics and Engineering, 2020, 39(10): 2094-2103.
17	吴艾祺. 车辆动荷载作用下公路挡土墙的力学响应研究[D]. 西安:长安大学地质工程与测绘学院, 2019.
	Wu Ai-qi. Research on the mechanical response of highway retaining walls under dynamic vehicle load[D]. Xi'an: College of Geological Engineering and Geomatics, Chang'an University, 2019.
18	刘宁, 刘杰. 国内外标准中砂性土内摩擦角确定方法对比[J]. 水运工程, 2021, 45(1): 42-47.
	Liu Ning, Liu Jie. Comparison of methods for determining the internal friction angle of sandy soil in domestic and foreign standards[J]. Water Transport Engineering, 2021, 45(1): 42-47.
19	刘寒冰, 张互助, 王静. 失水干燥对路基压实黏质土抗剪强度特性的影响[J]. 吉林大学学报: 工学版, 2017, 47(2): 446-451.
	Liu Han-bing, Zhang Hu-zhu, Wang Jing. Effect of dehydration and drying on shear strength characteristics of subgrade compacted clayey soil[J]. Journal of Jilin University (Engineering and Technology Edition), 2017, 47(2): 446-451.

相关文章 15

[1]	郭庆林,刘强,吴春利,李黎丽,李懿明,刘富春. 导电沥青及混合料裂缝局部温度场及愈合效果[J]. 吉林大学学报(工学版), 2022, 52(6): 1386-1393.
[2]	姚玉权,仰建岗,高杰,宋亮. 基于性能-费用模型的厂拌再生沥青混合料优化设计[J]. 吉林大学学报(工学版), 2022, 52(3): 585-595.
[3]	夏全平,高江平,罗浩原,张其功,李志杰,杨飞. 用于高模量沥青砼的复合改性硬质沥青低温性能[J]. 吉林大学学报(工学版), 2022, 52(3): 541-549.
[4]	冉武平,陈慧敏,李玲,冯立群. 干湿循环下粗粒土回弹模量演变规律及模型预估和修正[J]. 吉林大学学报(工学版), 2021, 51(6): 2079-2086.
[5]	董伟智,张爽,朱福. 基于可拓层次分析法的沥青混合料路用性能评价[J]. 吉林大学学报(工学版), 2021, 51(6): 2137-2143.
[6]	许哲谱,杨群. 基于实时路况地图的短期养护作业开始时间优化[J]. 吉林大学学报(工学版), 2021, 51(5): 1763-1774.
[7]	文畅平,任睆遐. 基于Lade模型的生物酶改良膨胀土双屈服面本构关系[J]. 吉林大学学报(工学版), 2021, 51(5): 1716-1723.
[8]	王元元,孙璐,刘卫东,薛金顺. 测量路面三维纹理双目重构算法的约束改进[J]. 吉林大学学报(工学版), 2021, 51(4): 1342-1348.
[9]	彭勇,杨汉铎,陆学元,李彦伟. 基于离散元法的空隙特征对沥青混合料虚拟剪切疲劳寿命的影响[J]. 吉林大学学报(工学版), 2021, 51(3): 956-964.
[10]	朱伟刚,朱超,张亚球,魏海斌. 基于卷积格网曲面拟合滤波算法的数字高程模型构建及质量评价[J]. 吉林大学学报(工学版), 2021, 51(3): 1073-1080.
[11]	程永春,李赫,李立顶,王海涛,白云硕,柴潮. 基于灰色关联度的矿料对沥青混合料力学性能的影响分析[J]. 吉林大学学报(工学版), 2021, 51(3): 925-935.
[12]	宫亚峰,逄蕴泽,王博,谭国金,毕海鹏. 基于吉林省路况的新型预制装配式箱涵结构的力学性能[J]. 吉林大学学报(工学版), 2021, 51(3): 917-924.
[13]	阳恩慧,徐加秋,唐由之,李奥,邱延峻. 温拌剂对沥青断裂和老化性能的影响[J]. 吉林大学学报(工学版), 2021, 51(2): 604-610.
[14]	戴文亭,司泽华,王振,王琦. 剑麻纤维水泥加固土的路用性能试验[J]. 吉林大学学报(工学版), 2020, 50(2): 589-593.
[15]	方宇,孙立军. 基于生存分析的城市桥梁使用性能衰变模型[J]. 吉林大学学报(工学版), 2020, 50(2): 557-564.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

边界高度H/m	破裂角θ/（°）			处于荷载中部破裂角θ的变化范围/（°）
边界高度H/m	交于荷载内侧	交于荷载中部	交于荷载外侧	处于荷载中部破裂角θ的变化范围/（°）
6.14	27.03	28.95	-	28.93~58.91
6.98	26.60	28.16	-	26.62~56.37
27.11	-	24.89	24.43	8.79~24.88
27.70	-	24.88	24.44	8.62~24.44

高度/m	计算公式	破裂角/（°）	主动土压力/kN	抗滑动稳定系数	抗倾覆稳定系数
6.14	荷载内侧	27.03	81.75	1.82	2.94
6.14	荷载中部	28.95	81.03	1.84	2.93
6.98	荷载内侧	26.60	103.36	1.75	2.69
6.98	荷载中部	28.16	104.02	1.74	2.68

计算公式		H=6.14 m		H=6.98 m		H=27.11	H=27.70
计算公式		荷载内侧	荷载中部	荷载内侧	荷载中部	H=27.11	H=27.70
现有公式计算	抗滑动稳定系数K_c	1.82	1.84	1.75	1.74	-	-
现有公式计算	抗倾覆稳定系数K₀	2.94	2.93	2.69	2.68	-	-
修正后公式计算	抗滑动稳定系数K_c	1.83		1.74		1.32	1.32
修正后公式计算	抗滑动稳定系数K₀	2.93		2.68		1.56	1.55