吉林大学学报(工学版) ›› 2021, Vol. 51 ›› Issue (6): 2087-2095.doi: 10.13229/j.cnki.jdxbgxb20210471
• 交通运输工程·土木工程 • 上一篇
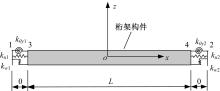
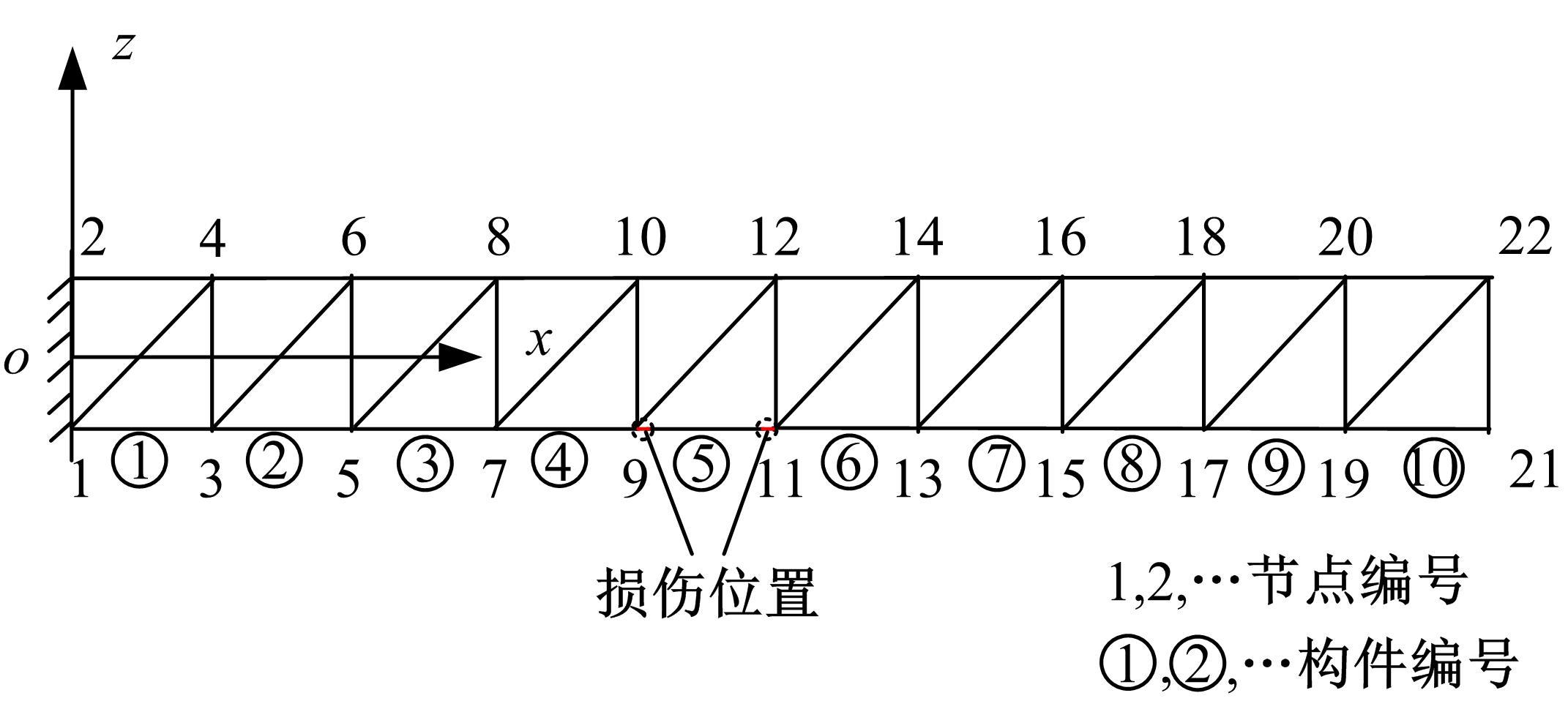
基于弹性波传播和谱单元法的桁架结构损伤检测
- 1.南京林业大学 土木工程学院,南京 210037
2.吉林大学 交通学院,长春 130022
Damage detection of truss structures based on elastic wave propagation and spectral element method
Fu-shou LIU1( ),Qi WEI1,Wen-ting XU1,Guo-jin TAN2(
),Qi WEI1,Wen-ting XU1,Guo-jin TAN2( )
)
- 1.College of Civil Engineering,Nanjing Forestry University,Nanjing 210037,China
2.College of Transportation,Jilin University,Changchun 130022,China
摘要:
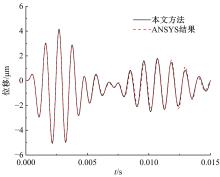
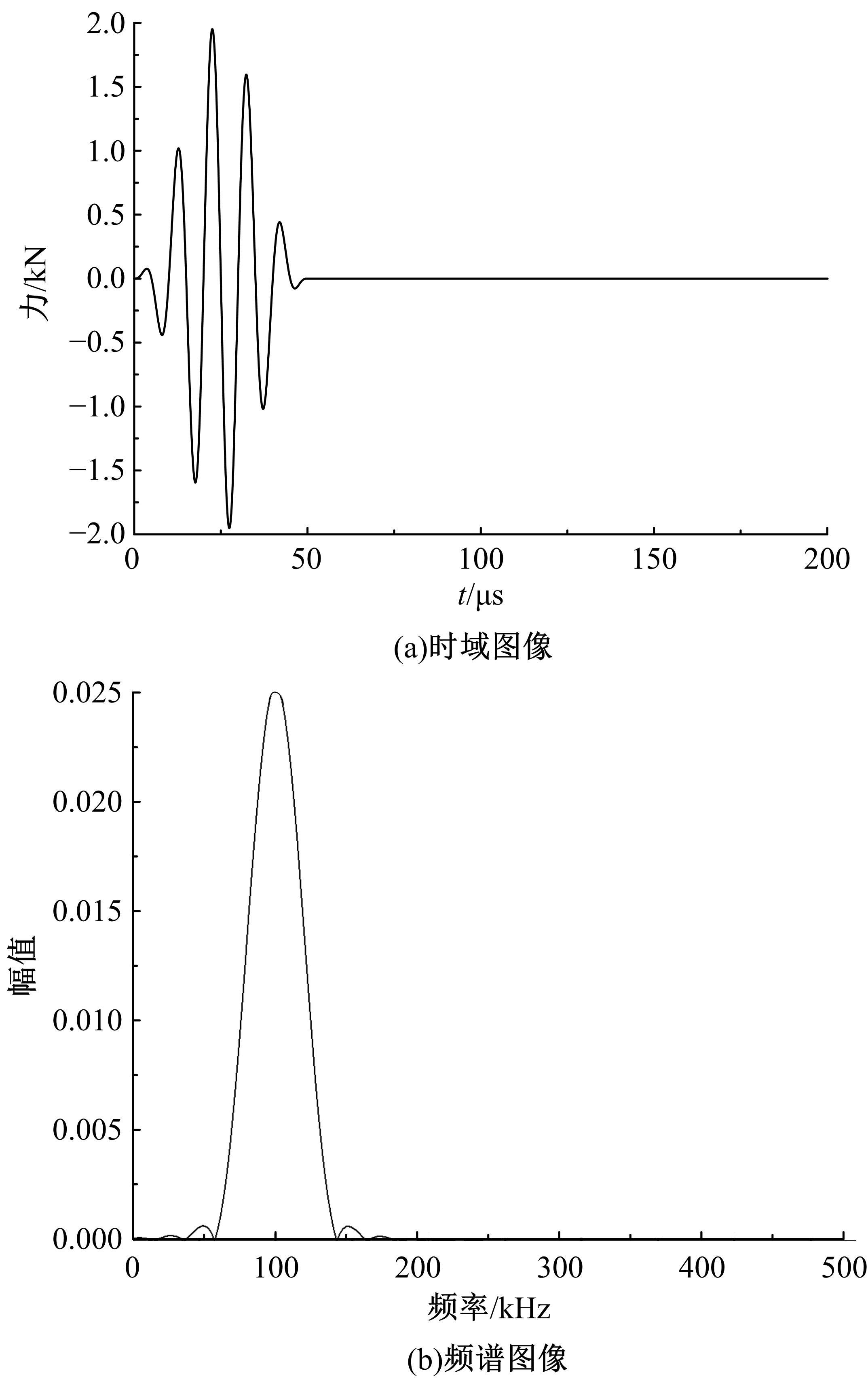
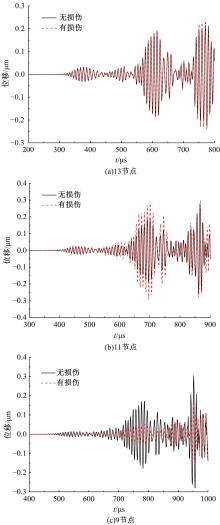
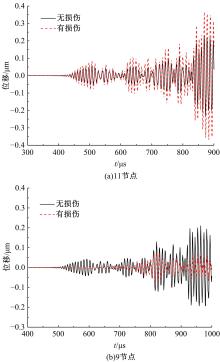
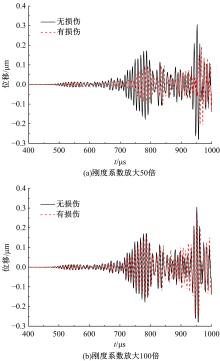
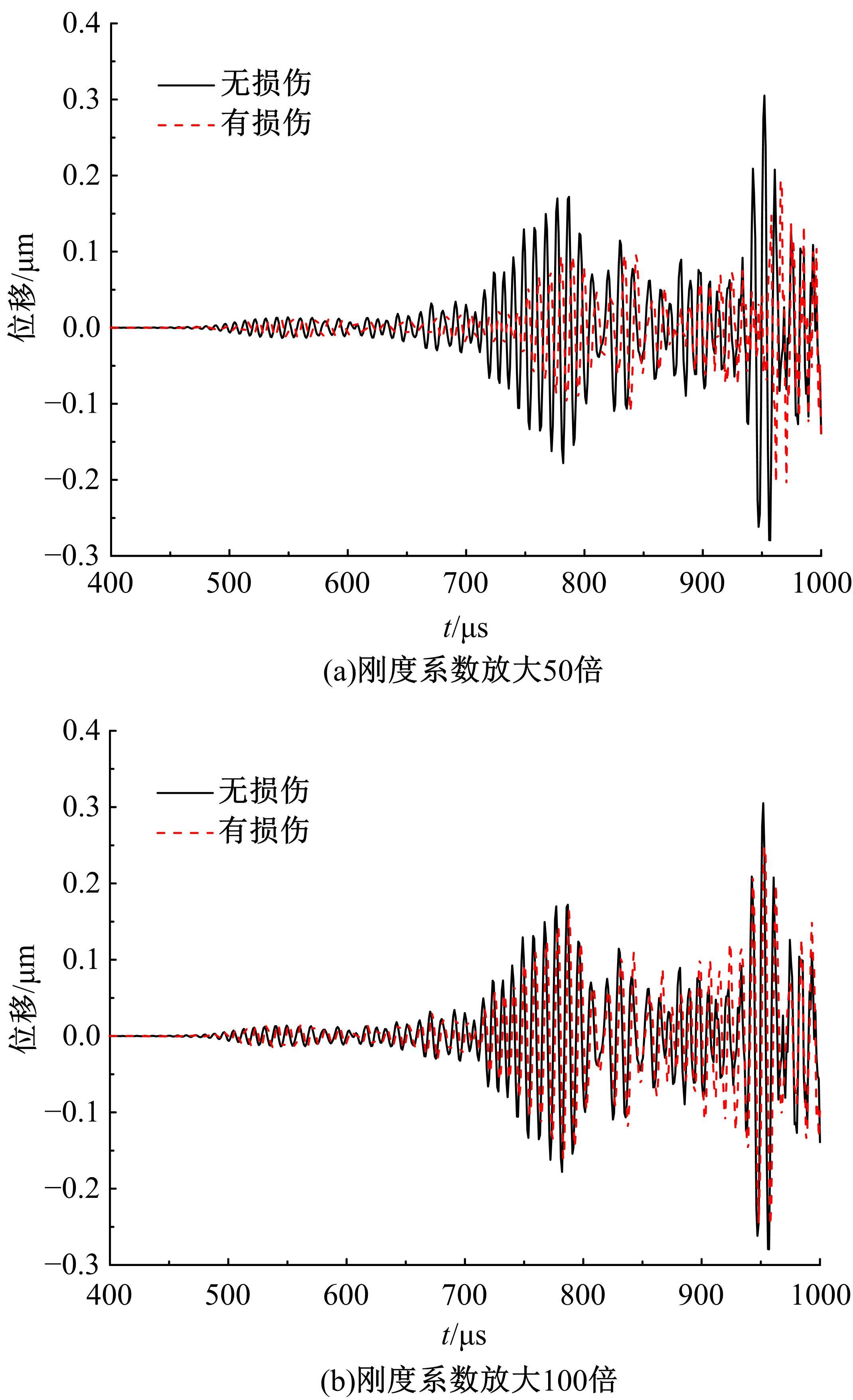
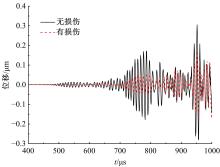
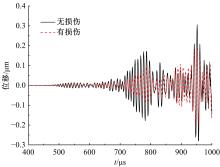
为了准确、及时地对桁架结构在长期服役后出现的构件和节点损伤进行检测,本文考虑了损伤引起的节点刚度下降,采用六自由度方向的线性弹簧模拟损伤后的节点,基于谱单元法建立了带有节点损伤的桁架结构动力学模型。对桁架结构施加窄带脉冲激励以激发结构内高频弹性波的传播,采用数值Laplace逆变换方法获取结构的时域响应。结果表明,弹性波在通过两端含有受损节点的构件时,其传播会受到一定的阻碍,导致桁架节点位移幅值的改变和首波到达节点时间的延迟,通过分析节点损伤引起的弹性波在桁架中传播的变化情况,可以实现桁架结构的损伤检测。
中图分类号:
- O327
| 1 | Liu F S, Wang L B, Jin D P, et al. Equivalent micropolar beam model for spatial vibration analysis of planar repetitive truss structure with flexible joints[J]. International Journal of Mechanical Sciences, 2019, 165:No.105202. |
| 2 | 金栋平, 刘福寿, 文浩, 等. 空间结构动力学等效建模与控制[M]. 北京: 科学出版社, 2021. |
| 3 | 姜东,徐宇,王桂伦,等. 锁定状态下球铰连接桁架的刚度性能[J]. 东南大学学报: 自然科学版, 2019, 49 (5):820-825. |
| Jiang Dong, Xu Yu, Wang Gui-lun, et al. Stiffness performance of ball hinged joint trusses under locking condition[J]. Journal of Southeast University (Natural Science Edition), 2019, 49(5):820-825. | |
| 4 | 周剑萍, 王亮. 大跨度主-副桁架钢结构的地震反应探讨分析[J]. 地震工程学报, 2019, 41(3):626-630. |
| Zhou Jian-ping, Wang Liang. Discussion and analysis of seismic response of large-span main and auxiliary steel-truss structures[J]. China Earthquake Engineering Journal, 2019, 41(3):626-630. | |
| 5 | 胡长远, 唐和生, 薛松涛,等. 桁架结构可靠性优化设计的微分演化算法[J]. 江苏大学学报:自然科学版, 2013,34(2): 234-238, 243. |
| Hu Chang-yuan, Tang He-sheng, Xue Song-tao, et al. Differential evolution algorithm of reliability optimization for truss structure[J]. Journal of Jiangsu Univeristy(Natural Science Edition), 2013,34(2): 234-238, 243. | |
| 6 | 綦宝晖, 邬瑞锋, 蔡贤辉,等. 一种桁架结构损伤识别的柔度阵法[J]. 计算力学学报, 2001, 18(1): 42-47. |
| Qi Bao-hui, Wu Rui-feng, Cai Xian-hui, et al. A flexible matrix method for damage identification of truss structures[J]. Chinese Journal of Computational Mechanics, 2001, 18(1): 42-47. | |
| 7 | Nguyen K D, Chan T H T, Thambiratnam D P, et al. Damage identification in a complex truss structure using modal characteristics correlation method and sensitivity-weighted search space[J]. Structural Health Monitoring, 2019, 18(1):49-65. |
| 8 | 谢甫哲, 周广杰, 顾斌,等. 钢框架连续倒塌分析中全焊接刚性节点的组件模型[J]. 江苏大学学报:自然科学版, 2020,41(4):466-472. |
| Xie Fu-Zhe, Zhou Guang-jie, Gu Bin, et al. Component-based model of all-welded joint in progressive collapse analysis of steel frames[J]. Journal of Jiangsu Univeristy(Natural Science Edition),2020,41(4):466-472. | |
| 9 | 谭国金. 中小跨径梁式桥动力检测技术及损伤识别方法研究[D]. 长春:吉林大学交通学院, 2009. |
| Tan Guo-jin. Dynamic detection technique and damage identification method for beam bridges with small and medium span[D]. Changchun: College of Transportation, Jilin University, 2009. | |
| 10 | 谭国金, 姜霖, 吴春利, 等. 移动车辆作用下带有裂缝的多片梁式桥动力响应分析[J]. 吉林大学学报:工学版,2020, 50(6):2147-2158. |
| Tan Guo-jin, Jiang Lin, Wu Chun-li, et al. Dynamic response analysis of multiple beam bridge with cracks under moving vehicles[J]. Journal of Jilin University (Engineering and Technology Edition), 2020, 50(6):2147-2158. | |
| 11 | 申林, 李万恒, 赵尚传. 基于模态柔度参数的连续梁桥损伤识别方法[J]. 吉林大学学报:工学版, 2019, 49(1):66-72. |
| Shen Lin, Li Wan-heng, Zhao Shang-chuan. Damage identification of continuous beam bridge based on modal flexibility recognition[J]. Journal of Jilin University (Engineering and Technology Edition), 2019, 49(1):66-72. | |
| 12 | 王术新, 姜哲. 裂缝梁固有频率分析[J]. 江苏大学学报:自然科学版, 2003,24(4):30-33. |
| Wang Shu-xin, Jiang Zhe. Analysis on natural frequencies of cracked beams[J]. Journal of Jiangsu Univeristy(Natural Science Edition), 2003,24(4):30-33. | |
| 13 | Ostachowicz W, Kudela P, Krawczuk M, et al. Guided Waves in Wtructures for SHM: the Time-domain Spectral Element Method[M]. Chichester: John Wiley & Sons Ltd, 2011. |
| 14 | Liu X L, Jiang Z W. Design of a PZT patch for measuring longitudinal mode impedance in the assessment of truss structure damage[J]. Smart Materials & Structures, 2009, 18(12):No.125017. |
| 15 | 吴冠男, 裘群海, 王腾,等. 弹性波在螺栓搭接结构中传播行为的数值模拟[J]. 应用力学学报, 2018, 35(3):458-464, 682. |
| Wu Guan-nan, Qiu Qun-hai, Wang Teng, et al. Numerical simulation of elastic wave propagation in bolted lapped structures[J]. Chinese Journal of Applied Mechanics, 2018, 35(3):458-464, 682. | |
| 16 | Stawiarski A, Barski M, Pająk P. Fatigue crack detection and identification by the elastic wave propagation method[J]. Mechanical Systems & Signal Processing, 2017, 89:119-130. |
| 17 | 王腾. 基于谱单元的弹性波传播分析及损伤检测研究[D]. 西安:西北工业大学材料学院, 2016. |
| Wang Teng. Analysis of elastic wave propagation and damage detection based on spectral element[D]. Xi′an: Institute of Materials, Northwestern Polytechnical University, 2016. | |
| 18 | Lee U. Spectral Element Method in Structural Dynamics[M]. Singapore: John Wiley & Sons (Asia), 2009. |
| 19 | Krawczuk M, Palacz M, Ostachowicz W. The dynamic analysis of a cracked Timoshenko beam by the spectral element method[J]. Journal of Sound & Vibration, 2003, 264(5): 1139-1153. |
| 20 | 彭海阔, 孟光. 基于谱元法的梁结构中Lamb波传播特性研究[J]. 噪声与振动控制, 2009, 29(6):62-66. |
| Peng Hai-kuo, Meng Guang. Study on Lamb wave propagation in beam structure based on spectral element method[J]. Noise and Vibration Control, 2009, 29(6):62-66. | |
| 21 | Zhu X P, Rizzo P, Marzani A, et al. Ultrasonic guided waves for nondestructive evaluation/structural health monitoring of trusses[J]. Measurement Science & Technology, 2010, 21(4):No.045701. |
| 22 | Igawa H, Komatsu K, Yamaguchi I, et al. Wave propagation analysis of frame structures using the spectral element method[J]. Journal of Sound and Vibration, 2004, 277(4/5):1071-1081. |
| 23 | Yang Z B, Chen X F, Li X, et al. Wave motion analysis in arch structures via wavelet finite element method[J]. Journal of Sound & Vibration, 2014, 333(2):446-469. |
| 24 | Brancik L. Programs for fast numerical inversion of Laplace transforms in MATLAB language environment[C]∥Proceedings of the 7th Conference MATLAB'99, Czech Republic, Prague, 1999:27-39. |
| 25 | 胡海岩. 结构阻尼模型及系统时域动响应[J]. 应用力学学报, 1993, 9(1): 34-43, 136. |
| Hu Hai-yan. Structural damping model and system dynamic response in time domain[J]. Chinese Journal of Applied Mechanics, 1993, 9(1):34-43, 136. | |
| 26 | Doyle J F. Wave Propagation in Structures Spectral Analysis Using Fast Discrete Fourier Transforms[M]. New York: Springer, 1997. |
| [1] | 樊学平,杨光红,肖青凯,刘月飞. 大跨桥梁主梁失效概率分析的最优R-Vine Copula[J]. 吉林大学学报(工学版), 2021, 51(4): 1296-1305. |
| [2] | 于江,赵志浩,秦拥军. 基于声发射和分形的钢筋混凝土受剪梁损伤[J]. 吉林大学学报(工学版), 2021, 51(2): 620-630. |
| [3] | 熊二刚,徐涵,谭赐,王婧,丁若愚. 基于弹塑性应力场理论的钢筋混凝土梁受剪承载力[J]. 吉林大学学报(工学版), 2021, 51(1): 259-267. |
| [4] | 樊学平,屈广,刘月飞. 应用新数据同化算法的桥梁极值应力预测[J]. 吉林大学学报(工学版), 2020, 50(2): 572-580. |
| [5] | 杨德磊,童乐为. 支管受轴向受拉工况下CHS-CFSHS T型节点应力集中系数计算公式[J]. 吉林大学学报(工学版), 2019, 49(6): 1891-1899. |
| [6] | 戴岩, 聂少锋, 周天华. 带环梁的方钢管约束钢骨混凝土柱-钢梁节点滞回性能有限元分析[J]. 吉林大学学报(工学版), 2018, 48(5): 1426-1435. |
| [7] | 杨昕卉, 薛伟, 郭楠. 钢板增强胶合木梁的抗弯性能[J]. 吉林大学学报(工学版), 2017, 47(2): 468-477. |
| [8] | 王少杰, 徐赵东, 李舒, 王凯洋,Dyke Shirley J. 基于应变监测的连续梁支承差异沉降识别[J]. 吉林大学学报(工学版), 2016, 46(4): 1090-1096. |
| [9] | 宿晓萍,王清. 复合盐浸-冻融-干湿多因素作用下的混凝土腐蚀破坏[J]. 吉林大学学报(工学版), 2015, 45(1): 112-120. |
| [10] | 姜浩, 郭学东. 基于地震激励的混凝土桥梁模态参数识别[J]. 吉林大学学报(工学版), 2011, 41(增刊2): 185-188. |
|
||