液力缓速器全充液工况下本征正交基对流场重构精度的影响规律

doi:10.13229/j.cnki.jdxbgxb20180583

摘要/Abstract

摘要：

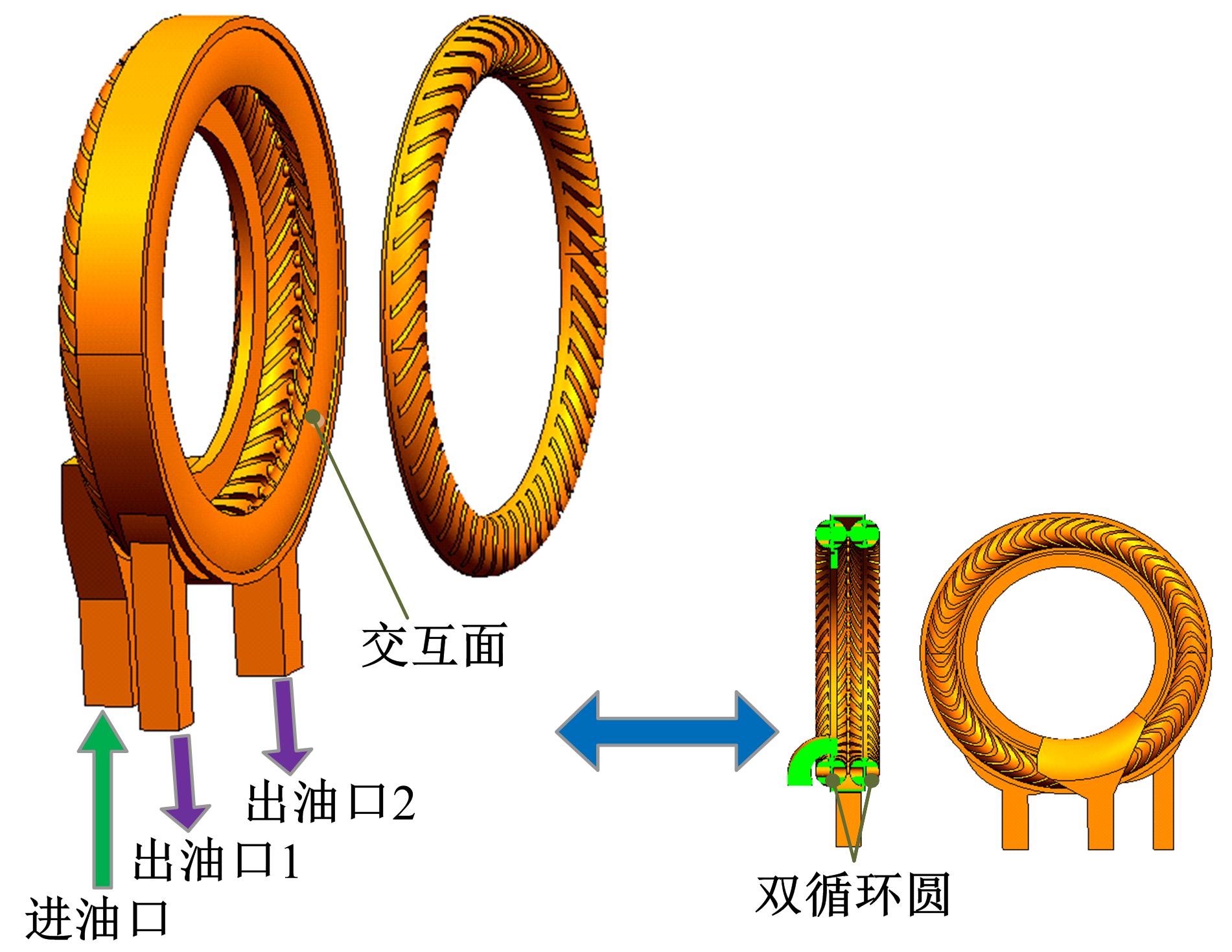
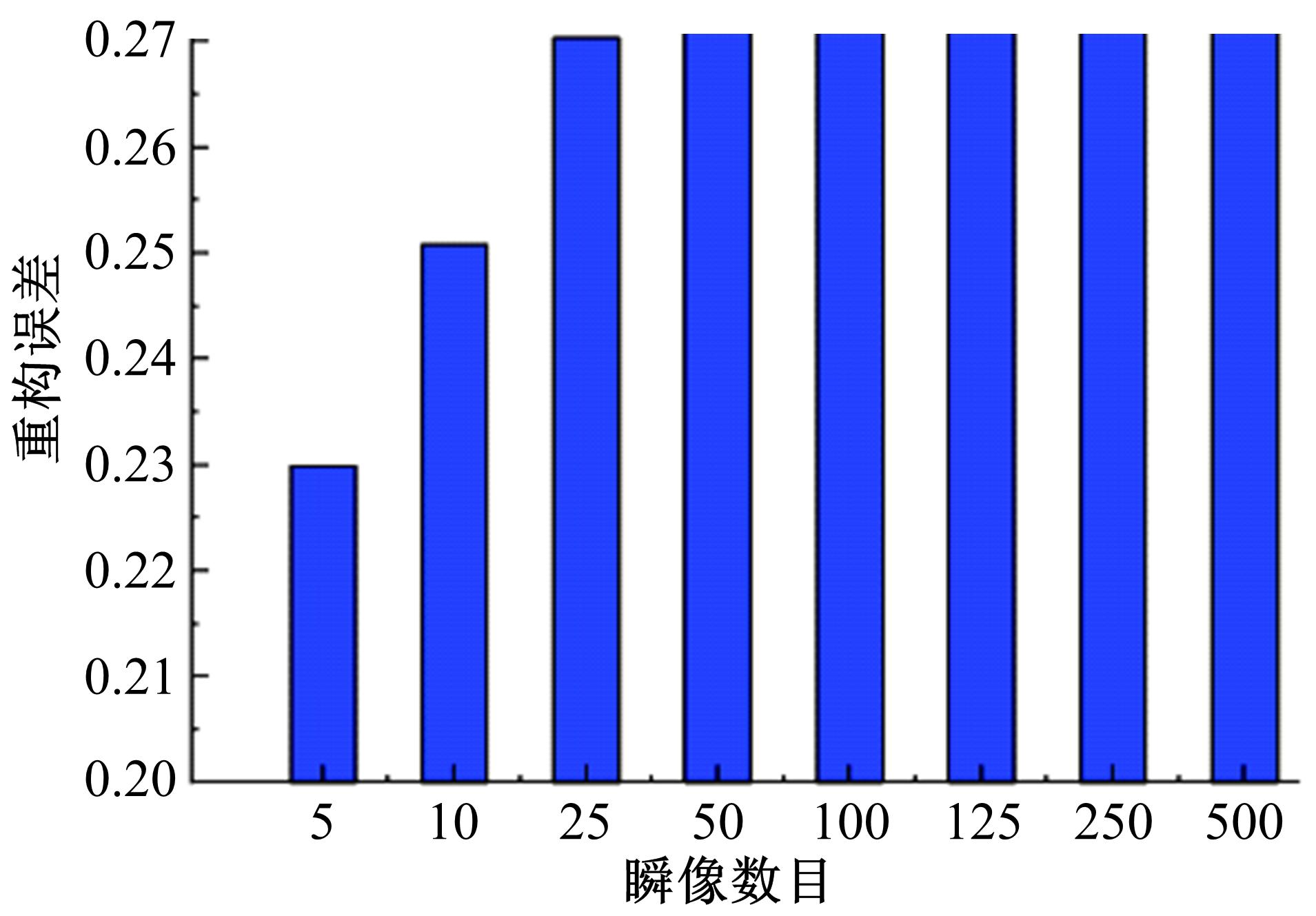
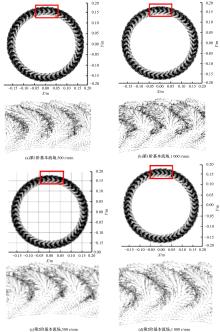
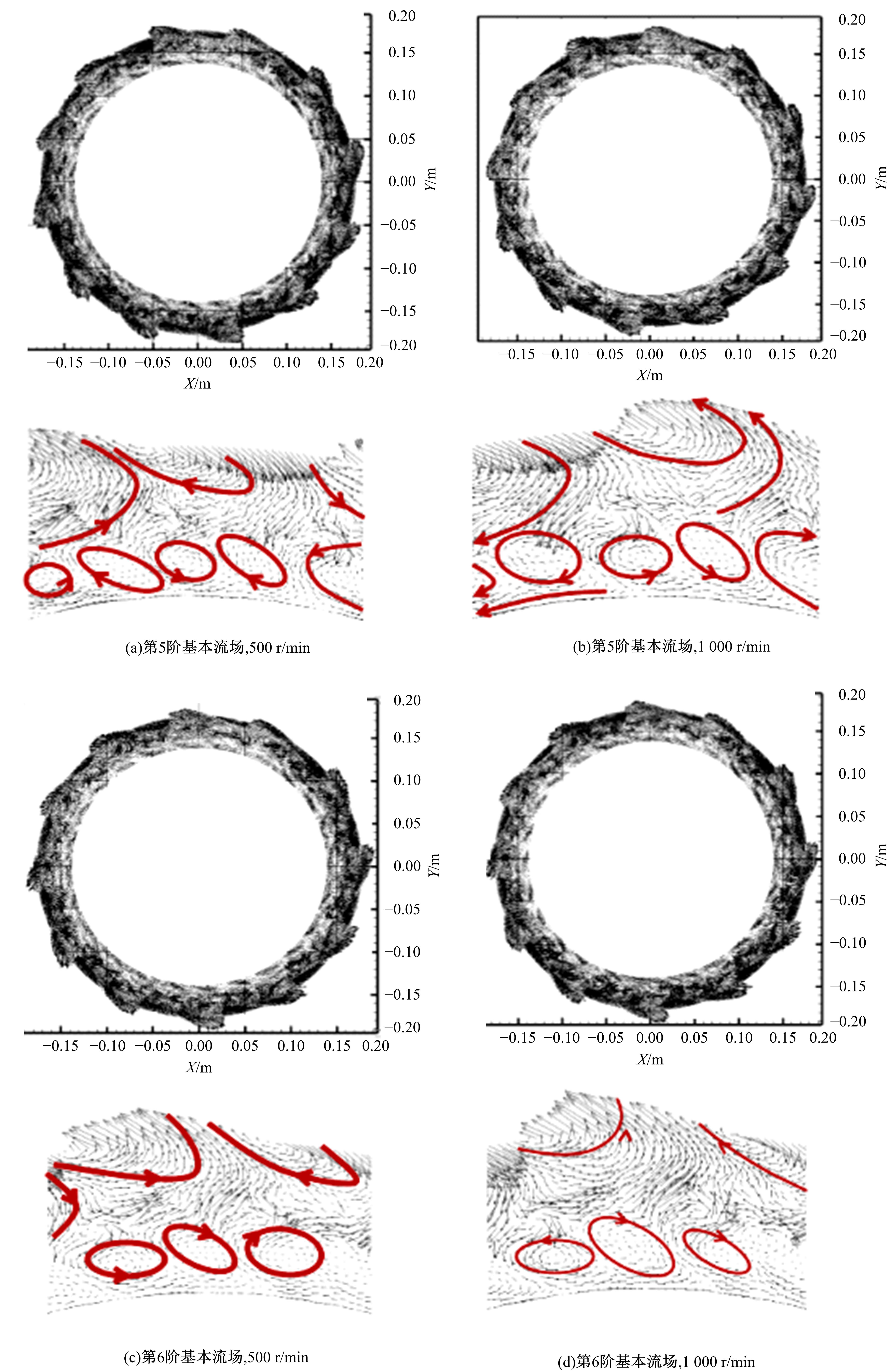
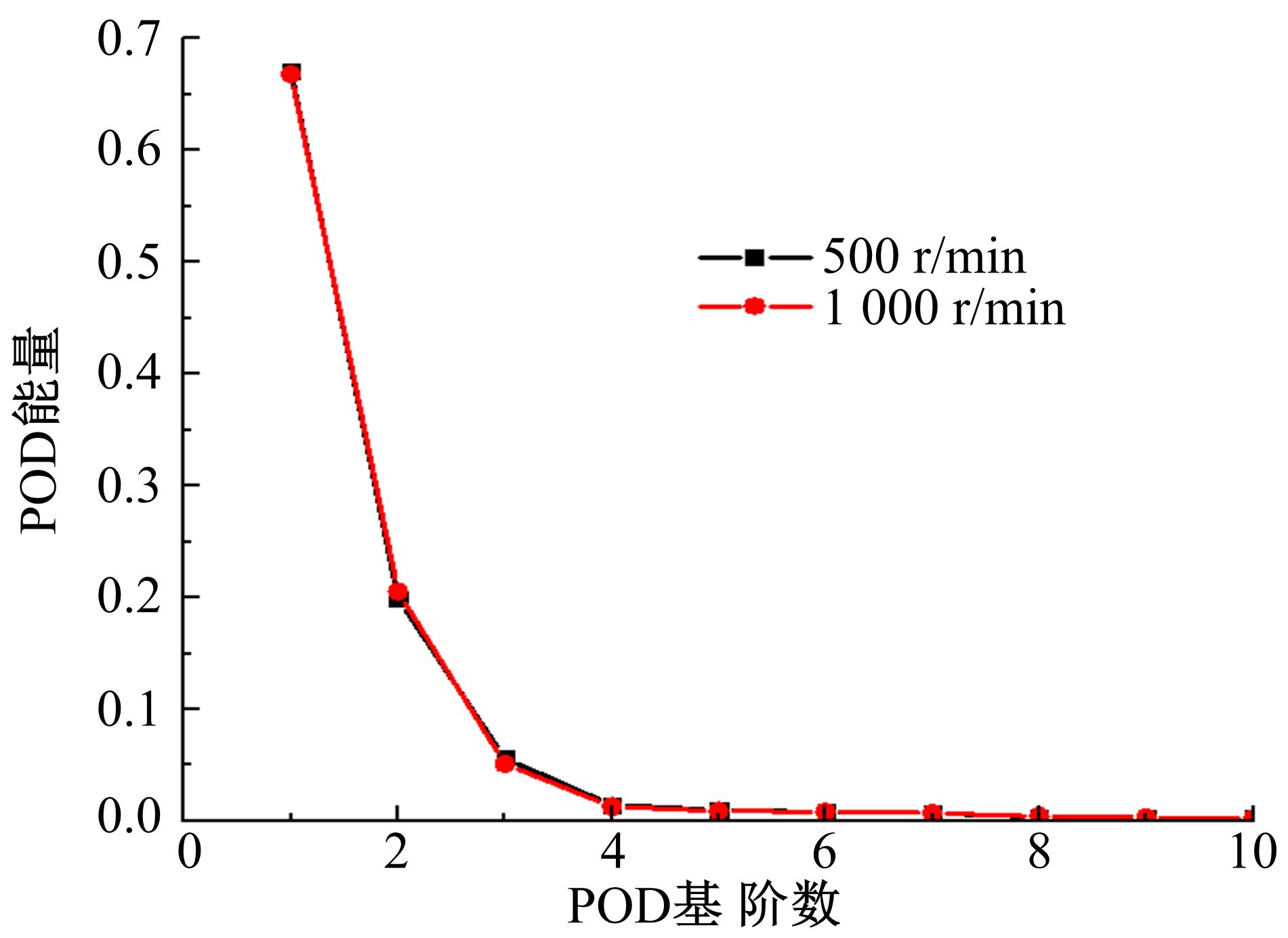
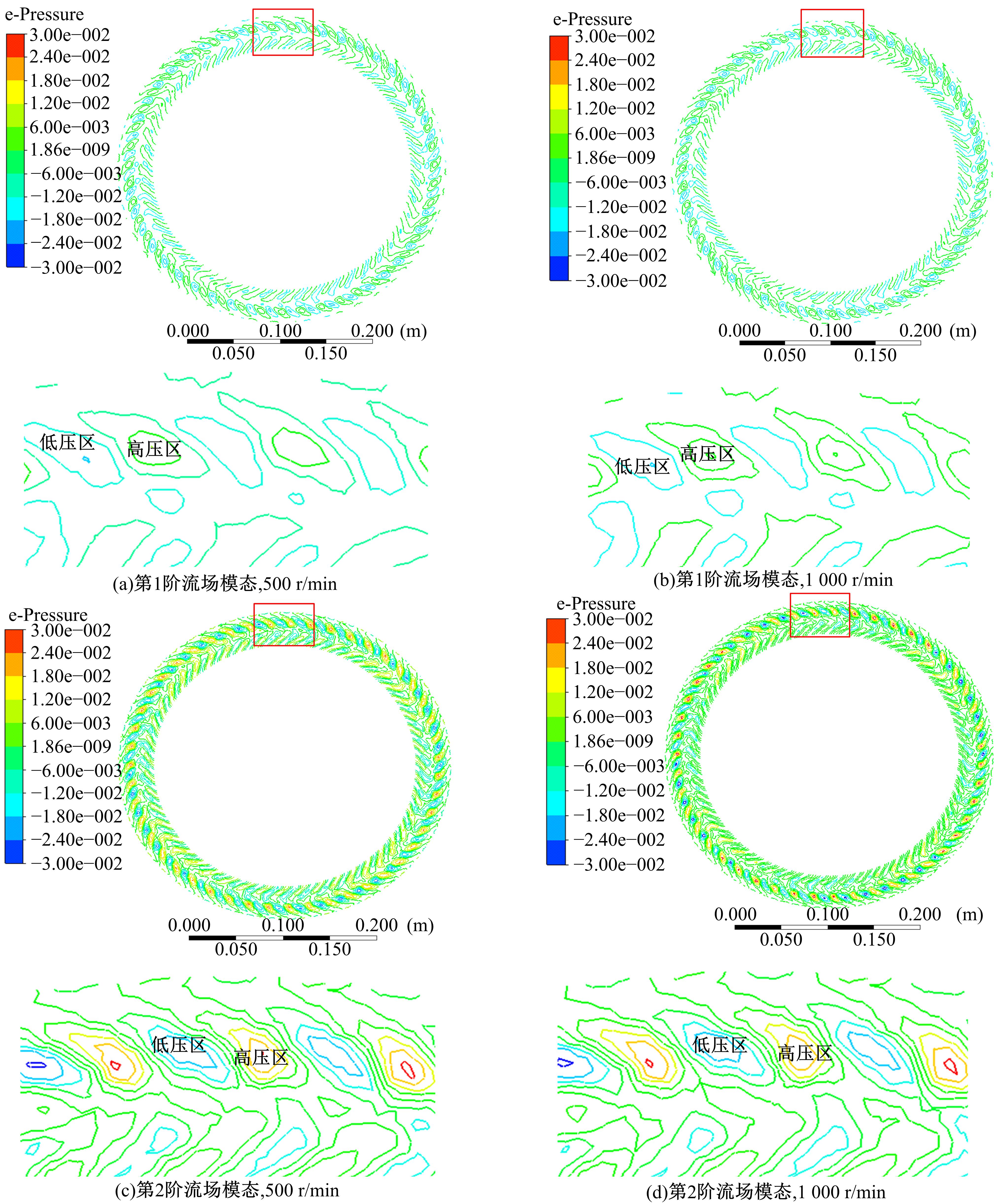
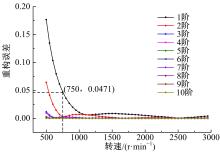
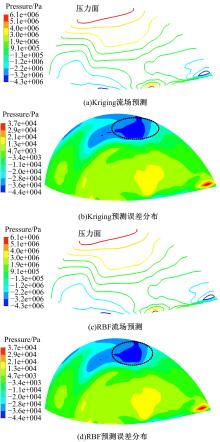
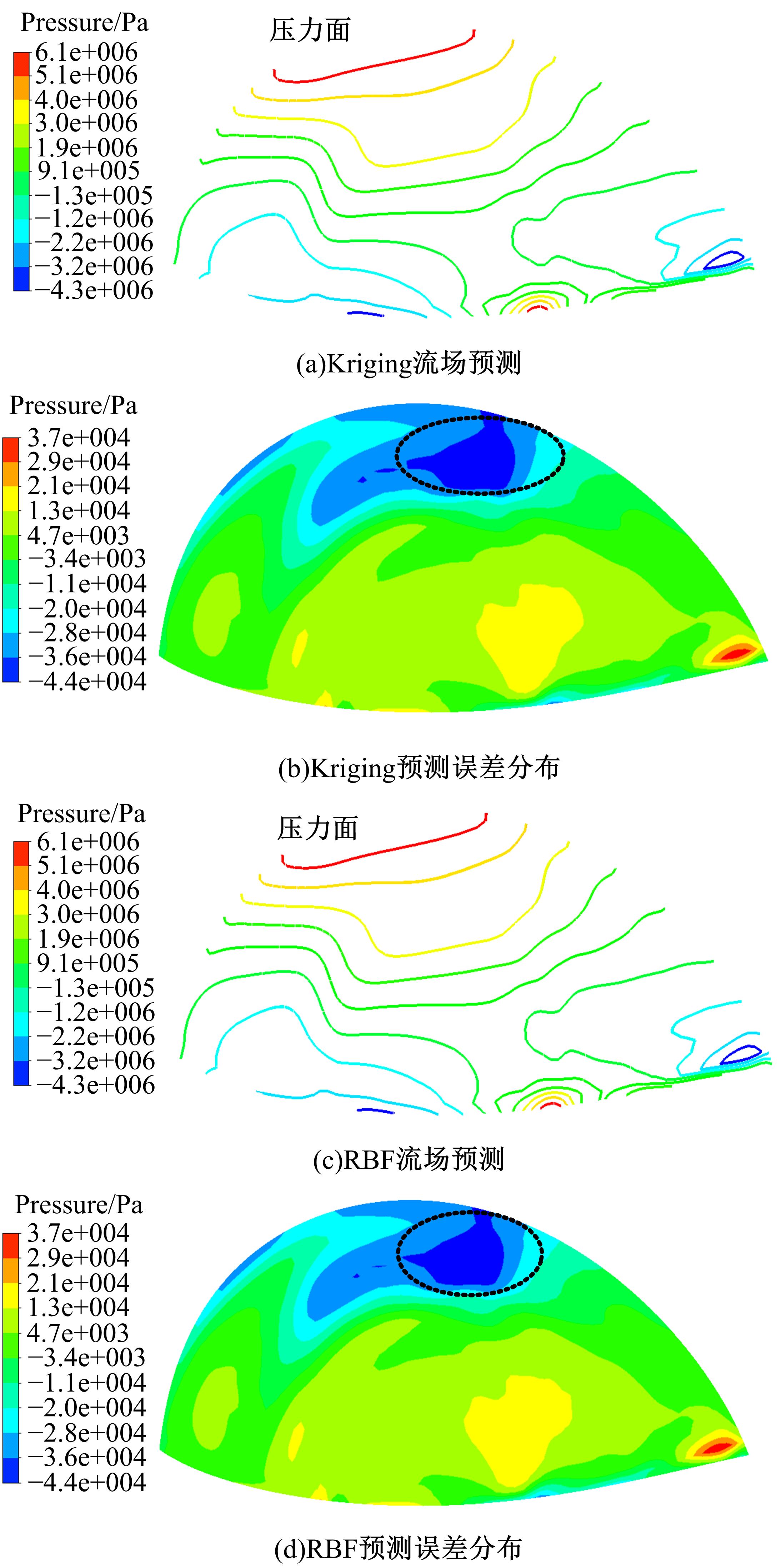
为揭示本征正交基对流场重构精度的影响规律，以全充液状态下液力缓速器定轮交互面上的流场为研究对象，分别获取速度和压力本征正交基。研究了影响本征正交基的瞬像和转速，并利用本征正交基和代理模型技术对流场进行预测。结果表明：瞬像集合的选择和采样频率对本征正交基的精确性影响较大，而液力缓速器动轮转速对速度场和压力场的本征正交基影响较小;基于本征正交基的流场预测具有一定精度。

关键词: 流体传动与控制, 液力缓速器, 本征正交基, 瞬像, 采样频率, 流场重构

Abstract:

In order to study the influence of Proper Orthogonal Decomposition (POD) basis functions on the accuracy of flow field reconstruction, the velocity and pressure proper orthogonal bases were obtained respectively for the flow field on the stator interaction surface of hydraulic retarder under full-filled condition. The influences of the instantaneous image factors and rotational speed factors on the proper orthogonal basis were studied, and the flow field is predicted by means of proper orthogonal basis and surrogate model technology. Results show that both the spiting of snapshots database and the frequency of sampling snapshots have significant effects on flow field reconstruction accuracy by POD basis functions while the features of POD basis functions are immune to the speed of the rotor, and the flow field reconstruction based on POD basis is of certain accuracy.

Key words: fluid transmission and control, hydrodynamic retarder, proper orthogonal decomposition basis function, snapshot, sampling frequency, flow field reconstruction

中图分类号:

TH137

魏巍,刘旭,韩雪永,闫清东. 液力缓速器全充液工况下本征正交基对流场重构精度的影响规律[J]. 吉林大学学报(工学版), 2019, 49(6): 1959-1968.

Wei WEI,Xu LIU,Xue-yong HAN,Qing-dong YAN. Flow field reconstruction accuracy influence of proper orthogonal decomposition basis functions in hydrodynamic retarderunder full⁃filled operating condition[J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(6): 1959-1968.

图/表 15

图1

图2

表1

图3

图4

表2

图5

图6

图7

图8

图9

图10

图11

图12

图13

参考文献

1	袁哲. 重型车液力缓速器热流耦合与散热系统研究[D].长春：吉林大学机械科学与工程学院, 2013.
1	YuanZhe. Study on heat-flow coupling and heat transfer system of hydrodynamic retarder of heavy vehicle[D]. Changchun: College of Mechanical Science and Engineering, Jilin University, 2013.
2	闫清东, 邹波, 魏巍, 等. 液力减速器充液过程瞬态特性三维数值模拟[J]. 农业机械学报, 2012, 43(1): 12-17.
2	YanQing-dong, ZouBo, WeiWei, et al. Numerical investigation on transient oil-filling process of hydraulic retarder[J]. Transactions of the Chinese Society for Agricultural Machinery, 2012, 43(1): 12-17.
3	卢秀泉, 褚亚旭, 才委, 等. 基于一维束流理论的液力减速器部分充液特性预测[J]. 吉林大学学报:工学版, 2011, 41(4): 988-992．
3	LuXiu-quan, ChuYa-xu, CaiWei, et al. Predicting method for partial-filling performance of hydrodynamic retarder based on one-dimensional flow beam theory[J]. Journal of Jilin University(Enginersity and Technology Edition), 2011, 41(4): 988-992.
4	LegresleyP A, AlonsoJ J. Investigation of non-linear projection for pod based reduced order models for aerodynamics[J]. AIAA paper, 2001: 1-15.
5	PlaF, HerreroH, VegaJ M. A flexible symmetry-preserving Galerkin/POD reduced order model applied to a convective instability problem[J]. Computers & Fluids, 2015, 119: 162-175.
6	LumleyJ L. The Structure of Inhomogeneous Turbulence[C]∥Atmospheric Turbulence and Ratio Wave Propagation, Moscow, Nauka, 1967:166-178.
7	SirovichL. Turbulence and the dynamics of coherent structures. Part I: Coherent structures[J]. Quarterly of Applied Mathematics, 1987,45(3): 561-571.
8	SinglerJ R, BattenB A. Balanced POD for linear PDE robust control computations[J]. Computational Optimization and Applications, 2012, 53(1): 227-248.
9	PodvinB. A proper-orthogonal-decomposition–based model for the wall layer of a turbulent channel flow[J]. Physics of Fluids, 2009, 21(1): 015111.
10	WangZ, AkhtarI, BorggaardJ, et al. Proper orthogonal decomposition closure models for turbulent flows: a numerical comparison[J]. Computer Methods in Applied Mechanics & Engineering, 2012, 237: 10-26.
11	TerragniF, VegaJ M. Construction of bifurcation diagrams using POD on the fly[J]. SIAM Journal on Applied Dynamical Systems, 2014, 13(1): 339-365.
12	HolmesP, LumleyJ, BerkoozG. Turbulence, Coherent Structures, Dynamical Systems and Symmetry[M]. Cambridge： Cambridge University Press, 1996.
13	CizmasP G A, RichardsonB R, BrennerT A, et al. Acceleration techniques for reduced-order models based on proper orthogonal decomposition[J]. Journal of Computational Physics, 2008, 227(16): 7791-7812.
14	康伟, 代向艳, 刘凝. 低速翼型绕流的多模态耦合与流动稳定性研究[J]. 西北工业大学学报, 2015, 33(3): 382-387.
14	KangWei, DaiXiang-yan, LiuNing. Multi-modal interaction and flow instability of flow around an airfoil at low reynolds number[J]. Journal of Northwestern Polytechnical University, 2015, 33(3): 382-387.

相关文章 15

[1]	刘俊龙,孙冬野,刘小军,尤勇. 基于耦合特性的机电控制无级变速器速比控制[J]. 吉林大学学报(工学版), 2019, 49(6): 1951-1958.
[2]	卢秀泉,胡春玉,柴亚龙,马文星,张剑楠. 大功率液力偶合器调速工况瞬态流场特性[J]. 吉林大学学报(工学版), 2019, 49(5): 1539-1546.
[3]	王晓晶,刘美珍,陈帅,李嵩. 仿真转台用连续回转电液伺服马达预测滑模控制[J]. 吉林大学学报(工学版), 2019, 49(5): 1547-1557.
[4]	闻德生,隋广东,田山恒,王少朋,冯佩坤,刘小雪. 内外啮合齿轮马达泄漏与容积效率分析及试验[J]. 吉林大学学报(工学版), 2019, 49(4): 1186-1193.
[5]	刘建芳, 王记波, 刘国君, 李新波, 梁实海, 杨志刚. 基于PMMA内嵌三维流道的压电驱动微混合器[J]. 吉林大学学报(工学版), 2018, 48(5): 1500-1507.
[6]	姜继海, 葛泽华, 杨晨, 梁海健. 基于微分器的直驱电液伺服系统离散滑模控制[J]. 吉林大学学报(工学版), 2018, 48(5): 1492-1499.
[7]	刘祥勇, 李万莉. 包含蓄能器的电液比例控制模型[J]. 吉林大学学报(工学版), 2018, 48(4): 1072-1084.
[8]	刘国君, 马祥, 杨志刚, 王聪慧, 吴越, 王腾飞. 集成式三相流脉动微混合芯片[J]. 吉林大学学报(工学版), 2018, 48(4): 1063-1071.
[9]	王佳怡, 刘昕晖, 王昕, 齐海波, 孙晓宇, 王丽. 数字二次元件变量冲击机理及其抑制[J]. 吉林大学学报(工学版), 2017, 47(6): 1775-1781.
[10]	何仁, 陈珊珊. 基于液力缓速器换挡控制的半挂汽车列车制动稳定性[J]. 吉林大学学报(工学版), 2017, 47(6): 1677-1687.
[11]	闻德生, 王京, 高俊峰, 周聪. 双定子单作用叶片泵闭死容腔的压力特性[J]. 吉林大学学报(工学版), 2017, 47(4): 1094-1101.
[12]	刘国君, 张炎炎, 杨旭豪, 李新波, 刘建芳, 杨志刚. 声表面波技术在金纳米粒子可控制备中的应用[J]. 吉林大学学报(工学版), 2017, 47(4): 1102-1108.
[13]	王丽, 刘昕晖, 王昕, 陈晋市, 梁燚杰. 装载机数字液压传动系统换挡策略[J]. 吉林大学学报(工学版), 2017, 47(3): 819-826.
[14]	李慎龙, 刘树成, 邢庆坤, 张静, 赖宇阳. 基于LBM-LES模拟的离合器摩擦副流致运动效应[J]. 吉林大学学报(工学版), 2017, 47(2): 490-497.
[15]	闻德生, 陈帆, 甄新帅, 周聪, 王京, 商旭东. 双定子泵和马达在压力控制回路中的应用[J]. 吉林大学学报(工学版), 2017, 47(2): 504-509.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

转速/(r·min^-1)	周期/s	总时间步数	时间步长/(10^-4 s)
500	0.12	800	2.4
1 000	0.24	800	1.2

瞬像数目	50	100	125	250	500
时间间隔/ms	1.2	0.6	0.48	0.24	0.12
采样频率/Hz	83.3	1 666.66	2 083.3	4 166.6	8 333.3