钢-混凝土组合梁的固有频率及其振型

doi:10.13229/j.cnki.jdxbgxb20181007

摘要/Abstract

摘要：

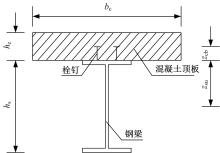
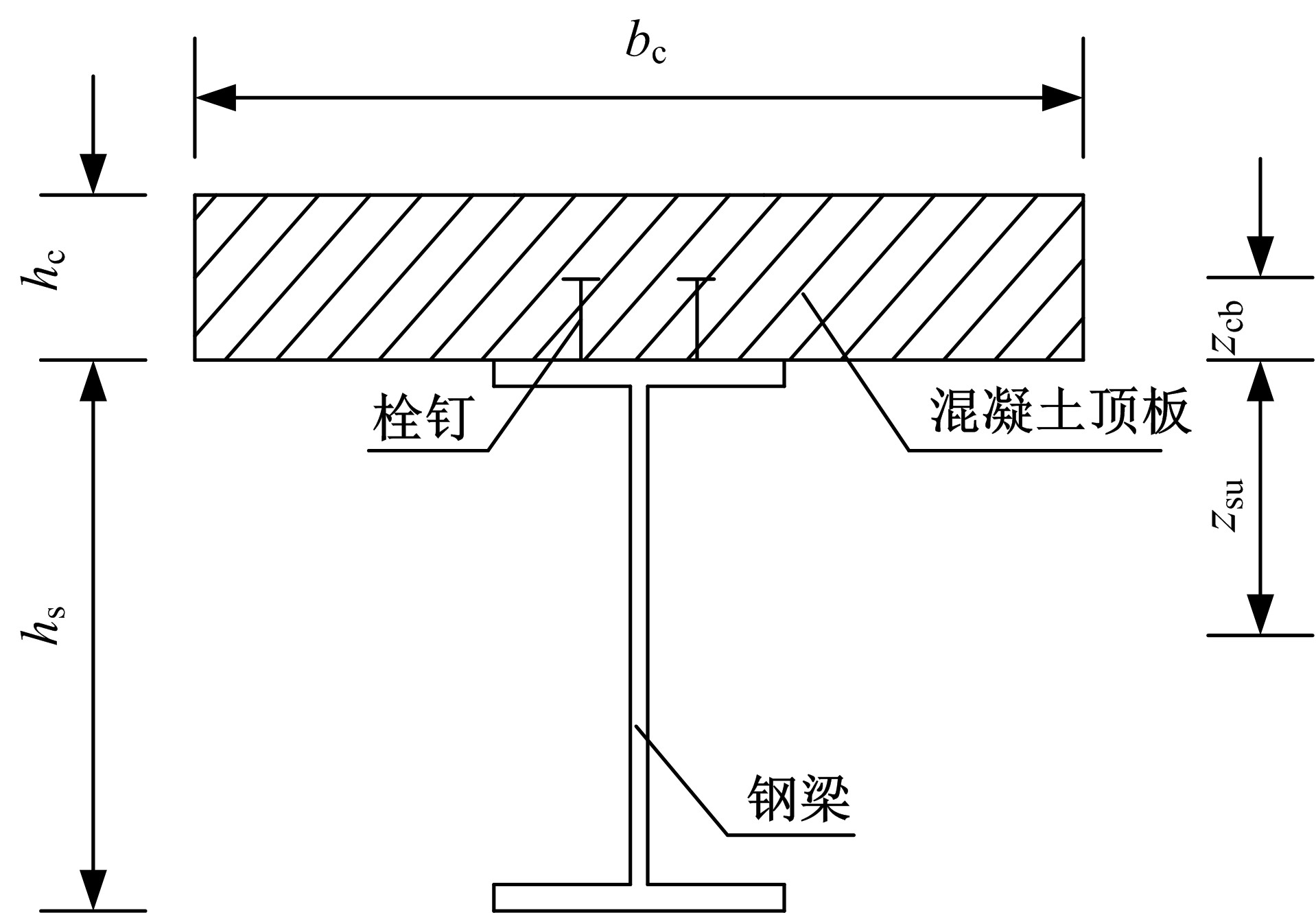
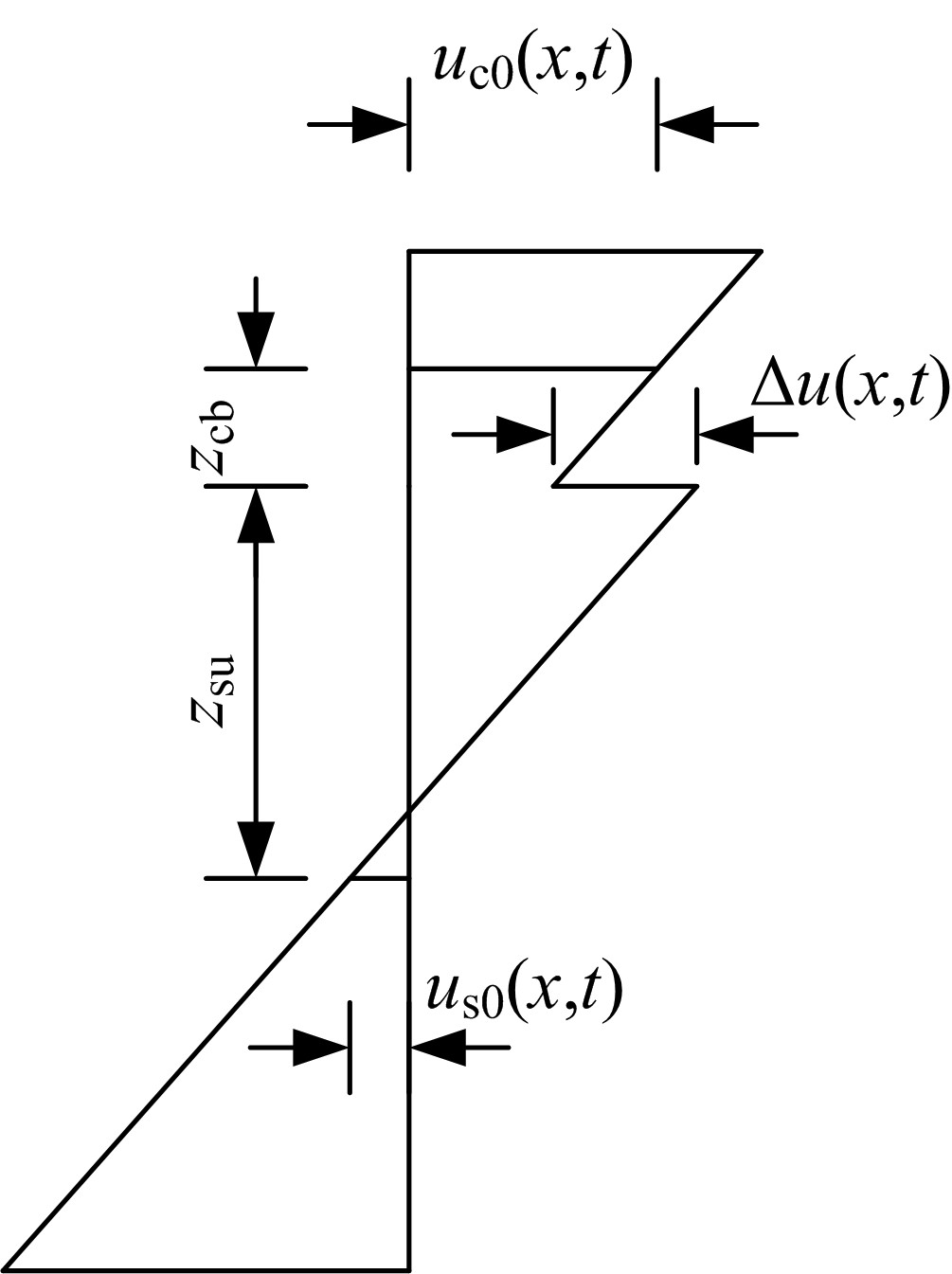
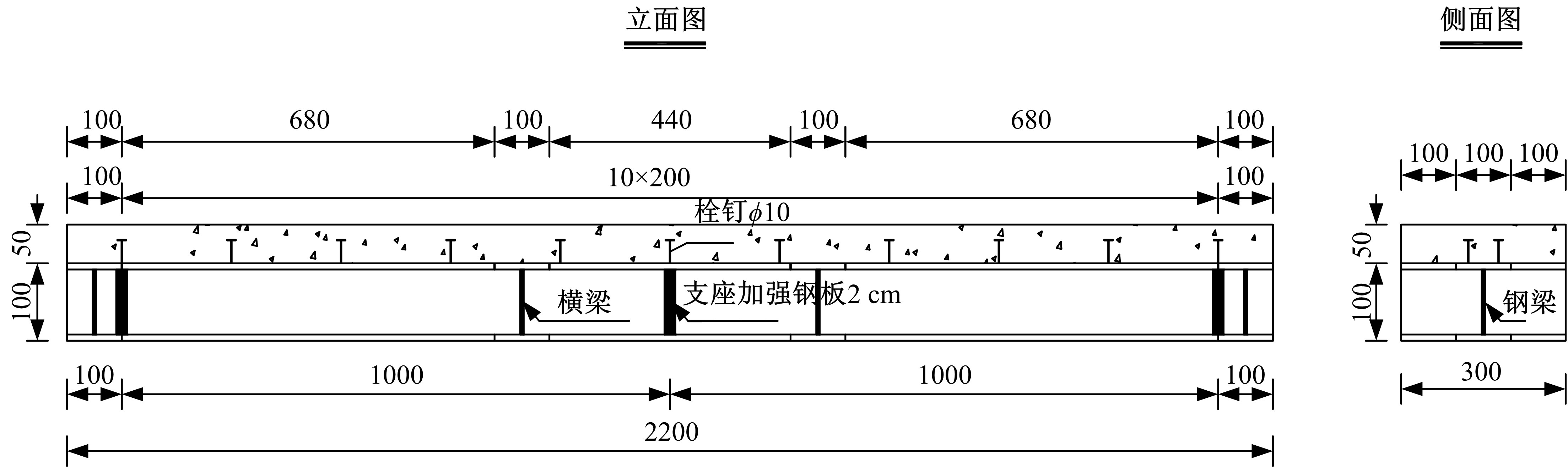
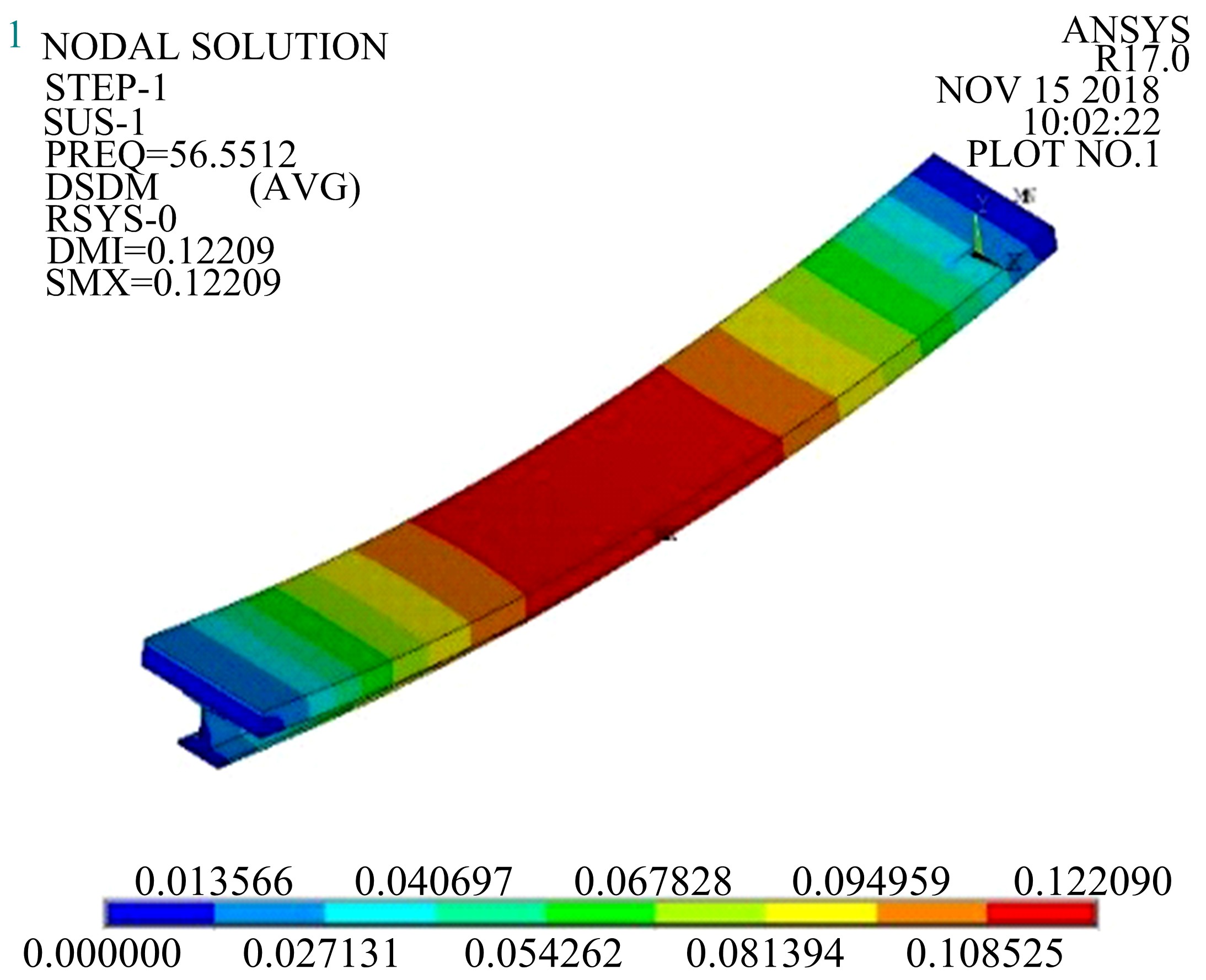
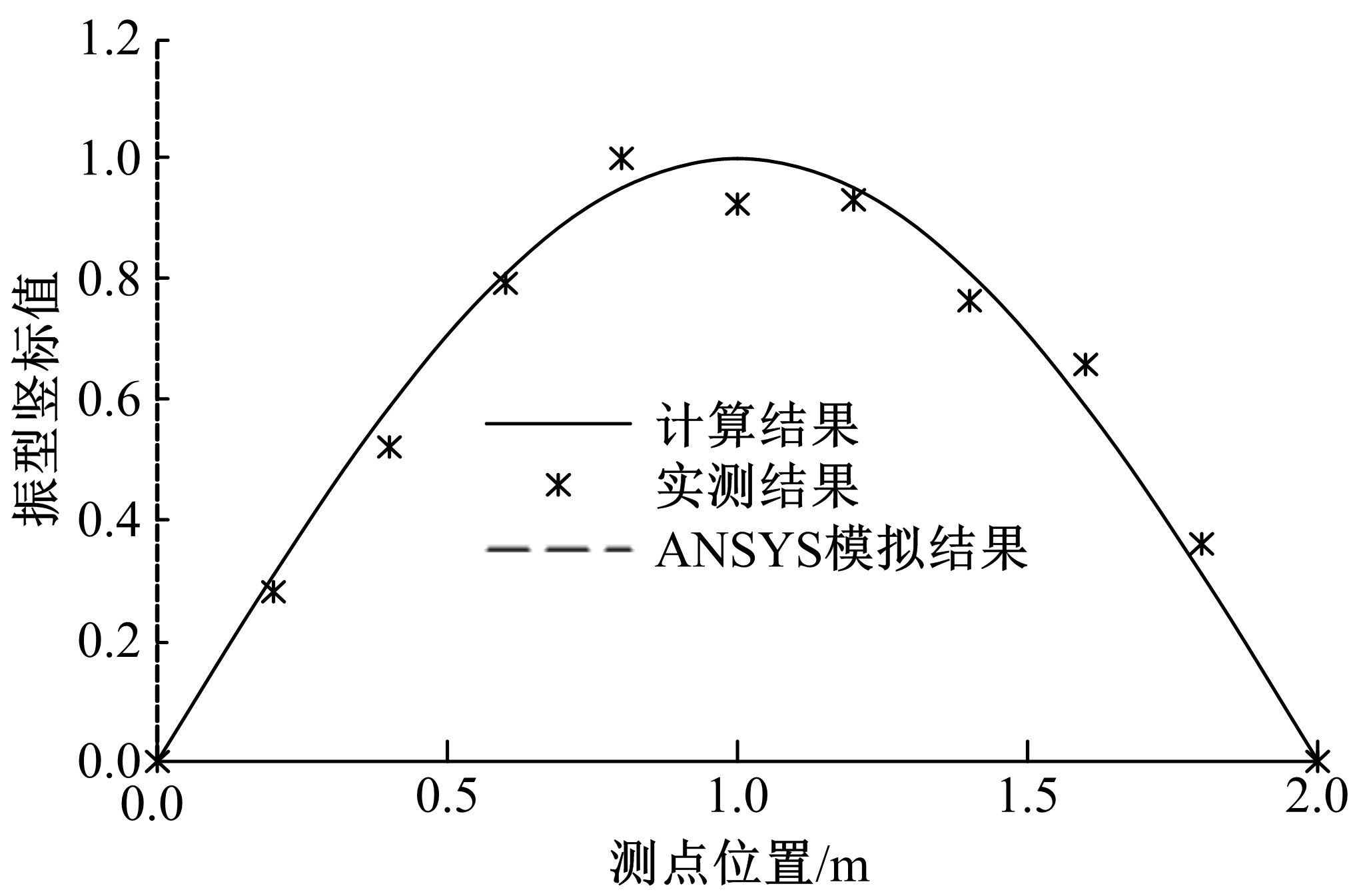
为准确分析考虑剪切滑移效应的钢-混凝土组合梁频率及其振型，根据经典动力学的基本思想和粘结滑移理论，提出了钢-混凝土组合梁考虑剪切滑移效应的位移函数，基于能量法推导了考虑剪切滑移效应的组合梁自由振动方程并得出固有频率及其振型，解决了考虑钢-混凝土组合梁交界面剪切滑移的难题。通过室内试验对试验梁的频率及其振型进行测试，并基于ANSYS对钢-混凝土组合梁进行了仿真模拟分析，将试验梁的测量值、ANSYS仿真模拟分析值与理论解进行对比，结果表明：考虑剪切滑移效应求解钢-混凝土组合梁频率和振型的解析解是正确的，在实际组合梁工程中考虑剪切滑移效应十分必要。

关键词: 公路运输, 桥梁工程, 组合梁, 自由振动, 剪切滑移效应

Abstract:

In order to accurately analyze the frequency and mode shape of steel-concrete composite beam considering the shear-slip effect, the displacement function of the steel-concrete composite beam is proposed according to the basic idea of classical dynamics and the bond slip theory. Based on the energy method, the free vibration equation of composite beam considering shear-slip effect is derived and the natural frequency and its mode shape are obtained, which solves the problem of considering shear-slip at the interface of steel-concrete composite beam. The frequency and mode shape of the testing beam are measured by laboratory test, and the simulation analysis of steel-concrete composite beams is carried out based on ANSYS. The measured value and simulation value are compared with the theoretical solution. The results show that the theoretical solution of the frequency and mode shape of the steel-concrete composite beam is correct and reasonable considering the shear-slip effect. It is very necessary to take the shear-slip effect into consideration in the practical composite beam engineering.

Key words: road transport, bridge engineering, composite beam, free vibration, shear slip effect

中图分类号:

U441

张云龙,郭阳阳,王静,梁东. 钢-混凝土组合梁的固有频率及其振型[J]. 吉林大学学报(工学版), 2020, 50(2): 581-588.

Yun-long ZHANG,Yang-yang GUO,Jing WANG,Dong LIANG. Natural frequency and mode of vibration of steel⁃concrete composite beam[J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 581-588.

图/表 9

图1

图2

图3

图4

图5

图6

表1

图7

图8

参考文献 19

1	Mackay H M, Gillespie P, Leluau C. Reporton the strength of steel I-beams haunched with concrete[J]. Engineering Journal, 1923, 6(8): 365-369.
2	Viest I M. Investigation of stud shear connectors for composite concrete and steel T-beams[J]. Journal of the American Concrete Insti-tute, 1956, 27(8): 875-891.
3	聂建国, 樊健生. 《钢结构设计规范》(GB50017—2002)钢-混凝土组合梁修订内容介绍[J]. 建筑结构学报, 2003,24(1): 34-39.
	Nie Jian-guo, Fan Jian-sheng. Introduction of the revised contents of composite steel-concrete beams in China Design Code of Steel Structure (GB50017—2002)[J]. Journal of Building Structures, 2003,24(1): 34-39.
4	聂建国, 唐亮, 魏茂林. 钢-混凝土组合梁扭转的组合作用分析[J]. 工业建筑, 2008, 38(3): 1-4.
	Nie Jian-guo, Tang Liang, Wei Mao-lin.Analysis of composite actions in steel-concrete composite beams subjected to torsion[J]. Industrial Construction, 2008, 38(3): 1-4.
5	聂建国, 陶慕轩, 吴丽丽, 等. 钢-混凝土组合结构桥梁研究新进展[J]. 土木工程学报, 2012, 45(6): 110-122.
	Nie Jian-guo, Tao Mu-xuan, Wu Li-li, et al. Advances of research on steel-concrete composite bridges[J]. China Civil Engineering Journal, 2012, 45(6): 110-122.
6	Shahdin A, Morlier J, Gourinat Y. Significance of low energy impact damage on modal parameters of composite beams by design of experiments[J]. Journal of Physics: Conference Series, 2009, 181(1): 1-8.
7	Hios J D, Fassois S D. Stochastic vector identification and uncertain modal parameter estimation for a smart composite beam[J]. Advances in Science & Technology, 2008, 56: 453-458.
8	Khan I A, Parhi D R. Fault detection of composite beam by using the modal parameters and RBFNN technique[J]. Journal of Mechanical Science & Technology, 2015, 29(4): 1637-1648.
9	Singh K V, Li G, Pang S S. Free vibration and physical parameter identification of non-uniform composite beams[J]. Composite Structures, 2006, 74(1): 37-50.
10	Yoo H H, Lee S H, Shin S H. Flapwise bending vibration analysis of rotating multi-layered composite beams[J]. Journal of Sound & Vibration, 2005, 286(4/5): 745-761.
11	Huang C W, Su Y H. Dynamic characteristics of partial composite beams[J]. International Journal of Structural Stability & Dynamics, 2008, 8(4): 665-685.
12	张云龙, 刘占莹, 吴春利, 等. 钢-混凝土组合梁静动力响应[J]. 吉林大学学报: 工学版, 2017, 47(3): 789-795.
	Zhang Yun-long, Liu Zhan-ying, Wu Chun-li, et al. Static and dynamic responses of steel-concrete composite beams[J]. Journal of Jilin University (Engineering and Technology Edition), 2017, 47(3): 789-795.
13	侯忠明, 夏禾, 王元清, 等. 钢-混凝土组合梁动力折减系数研究[J]. 振动与冲击, 2015, 34(4): 74-81.
	Hou Zhong-ming, Xia He, Wang Yuan-qing, et al. Dynamic reduction coefficients for a steel-concrete composite beam[J]. Journal of Vibration and Shock, 2015, 34(4): 74-81.
14	侯忠明, 夏禾, 张彦玲. 钢-混凝土简支结合梁基本动力特性的解析解[J]. 铁道学报, 2014, 36(3): 100-105.
	Hou Zhong-ming, Xia He, Zhang Yan-ling. Analytical solution to dynamic characteristics of simply-supported steel-concrete composite beams[J]. Journal of the China Railway Society, 2014, 36(3): 100-105.
15	侯忠明, 夏禾, 张彦玲. 栓钉连接件抗剪刚度对钢—混凝土结合梁自振特性影响研究[J]. 中国铁道科学, 2012, 33(6): 24-29.
	Hou Zhong-ming, Xia He, Zhang Yan-ling. The influence of the shear stiffness of stud connectors on the natural vibration characteristics of steel-connectors on the natural vibration characteristics of steel-concrete composite beam[J]. China Railway Science, 2012, 33(6): 24-29.
16	申志强, 夏军, 吴克刚, 等. 楔形变截面钢-混凝土组合梁弯曲和自由振动的求积元分析[J]. 国防科技大学学报, 2018, 40(1): 42-48.
	Shen Zhi-qiang, Xia Jun, Wu Ke-gang, et al. Flexural and free vibrational analysis of tapered partially steel-concrete composite beams using the weak form quadrature element method[J]. Journal of National University of Defense Technology, 2018, 40(1): 42-48.
17	刘寒冰, 马辉, 刘天明, 等. 竖向集中荷载作用下钢-混凝土组合梁的解析解[J]. 中国公路学报, 2010, 23(4): 39-44.
	Liu Han-bing, Ma Hui, Liu Tian-ming, et al. Analytical solution of steel-concrete composite beam under vertical loads[J]. China Journal of Highway and Transport, 2010, 23(4): 39-44.
18	郭学东, 马立军, 张云龙. 集中力作用下考虑剪切滑移效应的双层结合面组合梁解析解[J]. 吉林大学学报: 工学版, 2016, 46(2): 432-438.
	Guo Xue-dong, Ma Li-jun, Zhang Yun-long. Analytical solution of the double joint layer composite beams with shear-slipe under vertical concentrated load[J]. Journal of Jilin University (Engineering and Technology Edition), 2016, 46(2): 432-438.
19	陈玉骥, 罗旗帜. 钢-混凝土组合梁考虑剪力滞和滑移的一阶自振频率[J]. 烟台大学学报: 自然科学与工程版, 2015, 28(1): 49-53, 69.
	Chen Yu-ji, Luo Qi-zhi. First order natural vibration frequency for steel-concrete composite beams considering influence of shear lags and slips[J]. Journal of Yantai University (Natural Science and Engineering Edition), 2015, 28(1): 49-53, 69.

相关文章 15

[1]	王伯昕,杨海涛,王清,高欣,陈小旭. 基于补充改进集合经验模态分析法⁃多尺度排列熵分析桥梁振动信号优化滤波方法[J]. 吉林大学学报(工学版), 2020, 50(1): 216-226.
[2]	张淼,钱永久,张方,朱守芹. 基于增大截面法的混凝土加固石拱桥空间受力性能试验分析[J]. 吉林大学学报(工学版), 2020, 50(1): 210-215.
[3]	贾毅,赵人达,王永宝,李福海. 多跨长联连续梁桥粘滞阻尼器参数敏感性分析[J]. 吉林大学学报(工学版), 2019, 49(6): 1871-1883.
[4]	钟春玲,梁东,张云龙,王静. 体外预应力加固简支梁自振频率计算[J]. 吉林大学学报(工学版), 2019, 49(6): 1884-1890.
[5]	白伦华,沈锐利,张兴标,王路. 自锚式悬索桥的面内稳定性[J]. 吉林大学学报(工学版), 2019, 49(5): 1500-1508.
[6]	赵金钢,张明,占玉林,谢明志. 基于塑性应变能密度的钢筋混凝土墩柱损伤准则[J]. 吉林大学学报(工学版), 2019, 49(4): 1124-1133.
[7]	万世成,黄侨,关健,郭赵元. 预应力碳纤维板加固钢⁃混凝土组合连续梁负弯矩区试验[J]. 吉林大学学报(工学版), 2019, 49(4): 1114-1123.
[8]	李万恒,申林,王少鹏,赵尚传. 基于多阶段分区域动力测试的桥梁结构损伤评估[J]. 吉林大学学报(工学版), 2019, 49(3): 773-780.
[9]	尼颖升,孙启鑫,马晔,徐栋,刘超. 基于空间网格分析的多箱室波形钢腹板组合梁腹板剪力分配[J]. 吉林大学学报(工学版), 2018, 48(6): 1735-1746.
[10]	惠迎新,毛明杰,刘海峰,张尚荣. 跨断层桥梁结构地震响应影响[J]. 吉林大学学报(工学版), 2018, 48(6): 1725-1734.
[11]	郑一峰, 赵群, 暴伟, 李壮, 于笑非. 大跨径刚构连续梁桥悬臂施工阶段抗风性能[J]. 吉林大学学报(工学版), 2018, 48(2): 466-472.
[12]	宫亚峰, 何钰龙, 谭国金, 申杨凡. 三跨独柱连续曲线梁桥抗倾覆稳定性分析[J]. 吉林大学学报(工学版), 2018, 48(1): 133-140.
[13]	尼颖升, 孙启鑫, 马晔, 徐栋. 基于拉应力域的波形钢腹板组合梁承载力配筋计算[J]. 吉林大学学报(工学版), 2018, 48(1): 148-158.
[14]	魏志刚, 刘寒冰, 时成林, 宫亚峰. 考虑桥面铺装作用的简支梁桥横向分布系数计算[J]. 吉林大学学报(工学版), 2018, 48(1): 105-112.
[15]	魏志刚, 时成林, 刘寒冰, 张云龙. 车辆作用下钢-混凝土组合简支梁动力特性[J]. 吉林大学学报(工学版), 2017, 47(6): 1744-1752.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

项目	固有频率/Hz	误差/%
理论解	55.00	2.00
ANSYS解	56.55	0.76
实测值	56.12	—