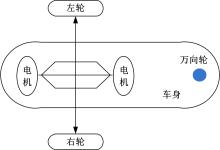
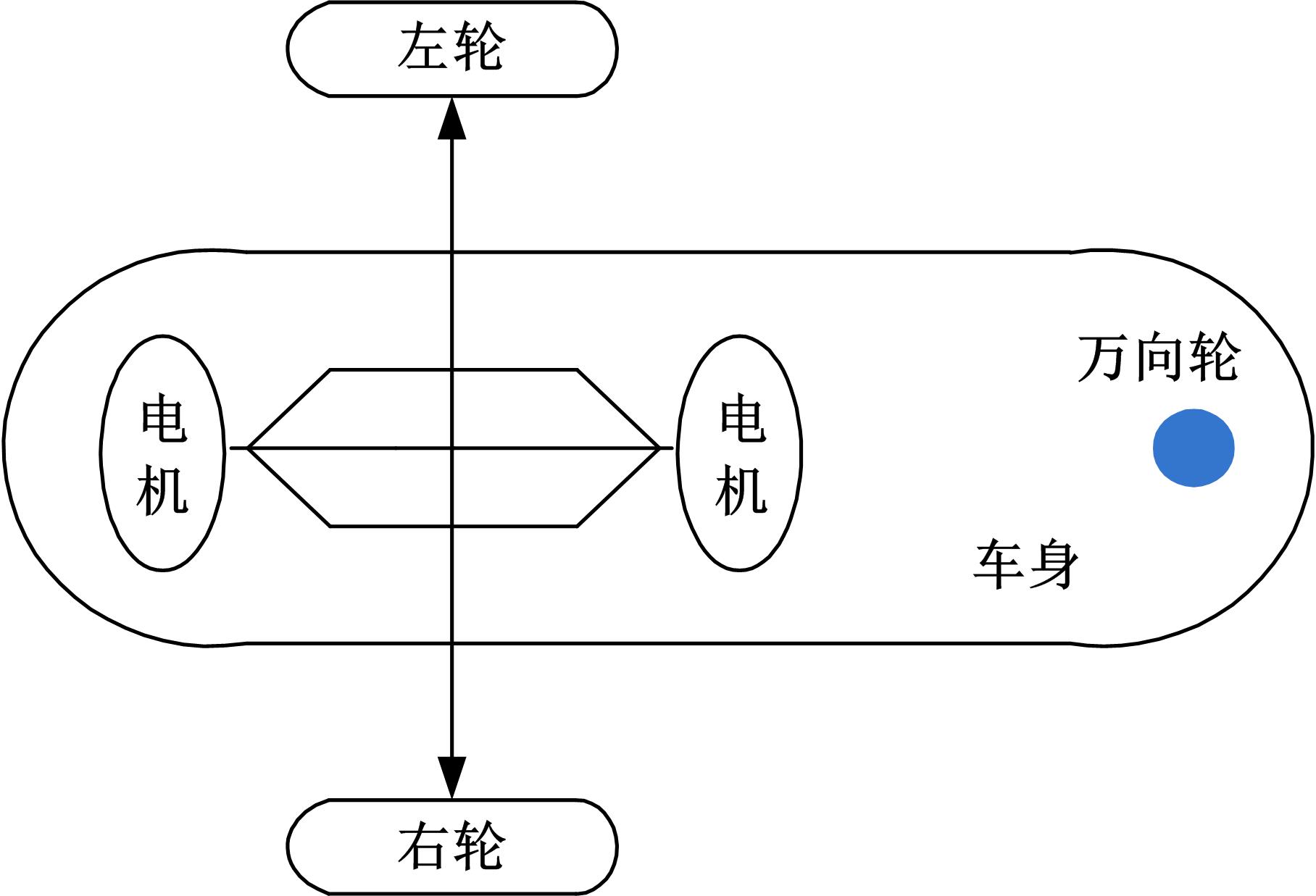
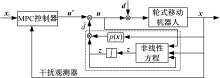
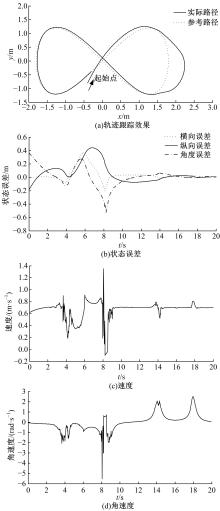
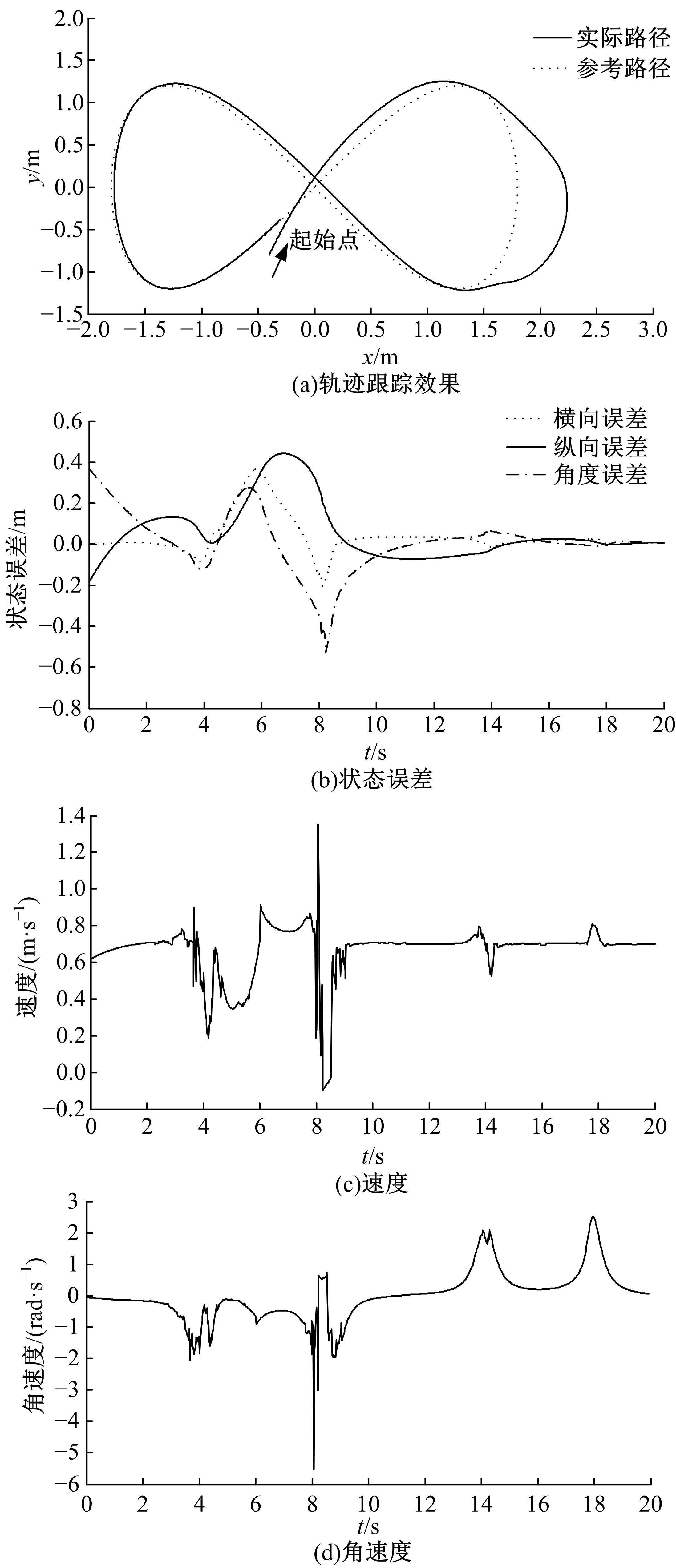
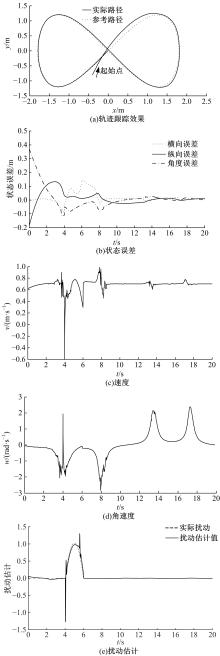
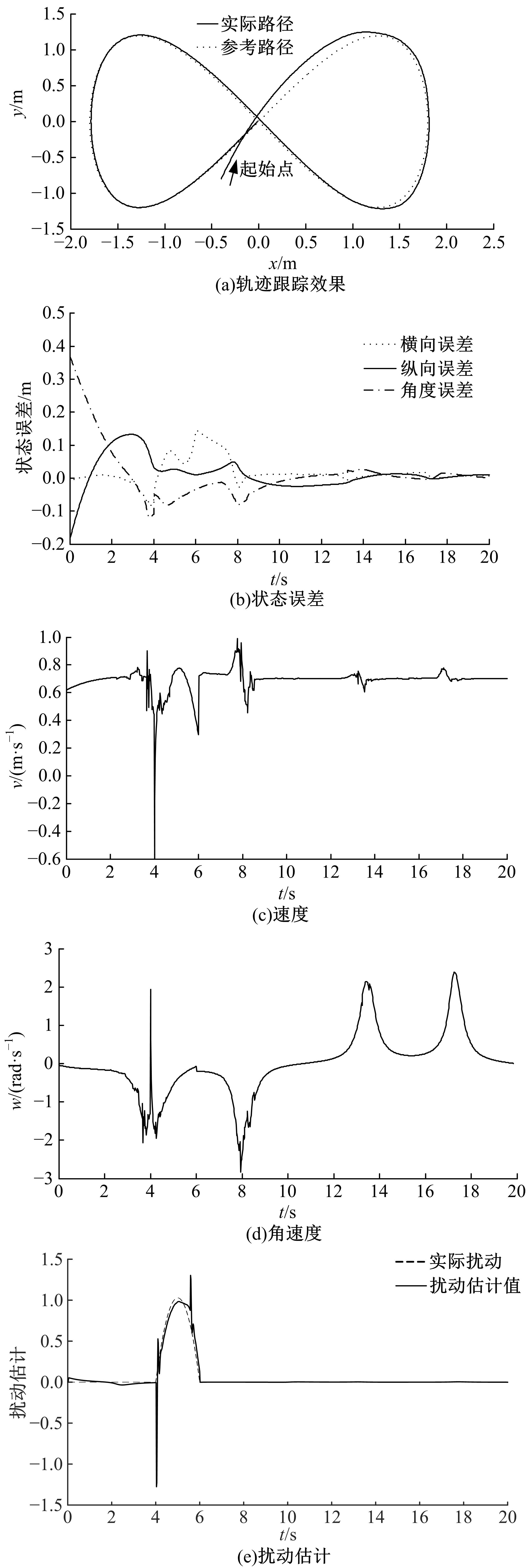
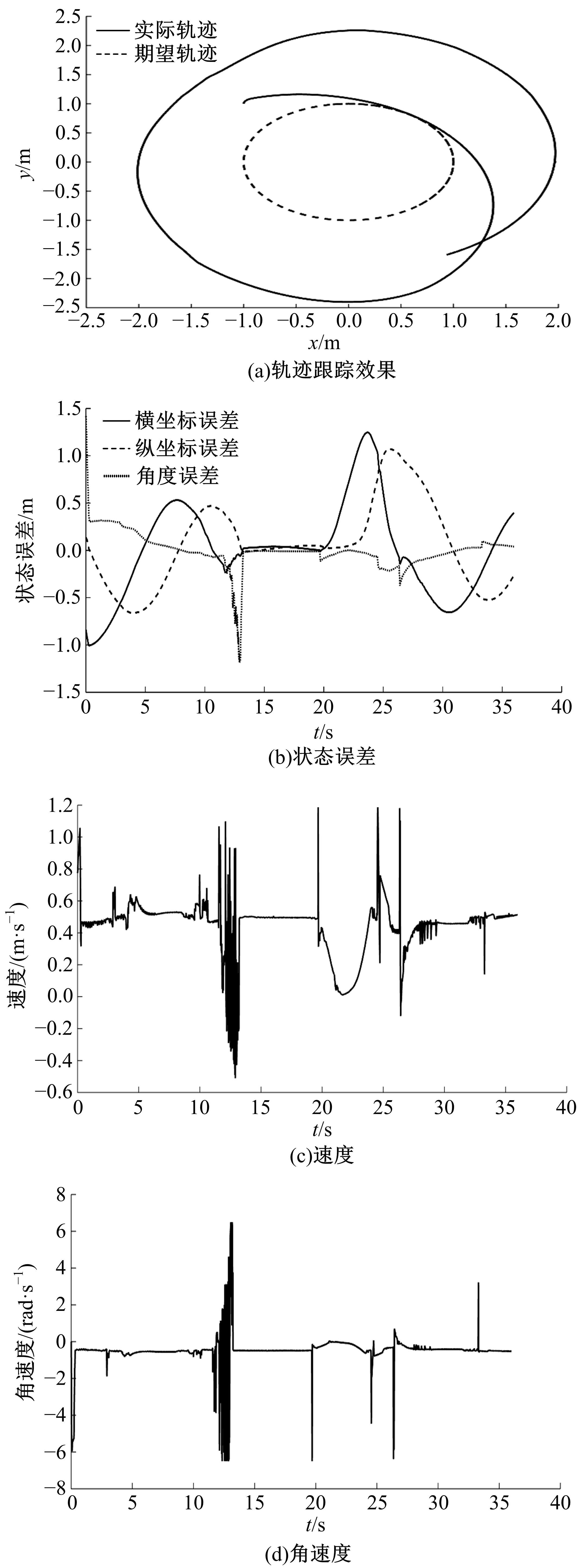
Journal of Jilin University(Engineering and Technology Edition) ›› 2021, Vol. 51 ›› Issue (3): 1097-1105.doi: 10.13229/j.cnki.jdxbgxb20200065
Disturbance observer based moving horizon control for path following problems of wheeled mobile robots
Shu-you YU1,2( ),Huan CHANG2,Ling-yu MENG2,Yang GUO2,Ting QU1(
),Huan CHANG2,Ling-yu MENG2,Yang GUO2,Ting QU1( )
)
- 1.State Key Laboratory of Automotive Simulation and Control,Jilin University,Changchun 130022,China
2.College of Communication Engineering,Jilin University,Changchun 130022,China
CLC Number:
- TP273
| 1 | Brockett R. Asymptotic stability and feedback stabilization[J]. Differential Geometry Control Theory, 1983, 27(3): 181-191. |
| 2 | Kanayama Y, Kimura Y, Miyazaki F, et al. A stable tracking control method for an autonomous mobile robot[C]∥IEEE International Conference on Robotics & Automation, Cincinnati, USA, 1990: 384-389. |
| 3 | Luca A D, Benedetto M D D. Control of non-holonomic systems via dynamic compensation[J]. Kybernetika Praha, 1993, 29(6): 593-608. |
| 4 | D' Andrea-Novel B, Campion G, Bastin G. Control of nonholonomic wheeled mobile robots by state feedback linearization[J]. International Journal of Robotics Research, 1995, 14(6): 543-559. |
| 5 | Samson C, Ait-Abderrahim K. Feedback control of a nonholonomic wheeled cart in Cartesian sp- ace[C]∥IEEE International Conference on Robotics & Automation, California, USA, 1991:1136-1141. |
| 6 | Fierro R, Lewis F L. Control of a nonholonomic mobile robot: backstepping kinematics into dynamics[C]∥Proceedings of 34th IEEE Conference on Decision and Control, New Orleans, LA, 1995: 3805-3810. |
| 7 | Indiveri G. Kinematic time-invariant control of a 2-D nonholonomic vehicle[C]∥Proceedings of 38th IEEE Conference on Decision and Control, Phoenix, USA, 1999: 2112-2117. |
| 8 | Jiang Z P, Nijmeijer H. Tracking control of mobile robots: a case study in backstepping[J]. Automatica, 1997, 33(7): 1393-1399. |
| 9 | Bloch A, Drakunov S. Tracking in nonholonomic dynamic systems via sliding modes[C]∥IEEE Conference on Decision & Control, New Orleans, USA, 1995: 2103-2106. |
| 10 | 朱亮, 姜长生, 张春雨. 基于径向基神经网络干扰观测器的空天飞行器自适应轨迹线性化控制[J]. 航空学报,2007,28(3):673-677. |
| Zhu Liang,Jiang Chang-sheng,Zhang Chun-yu. Adaptive trajectory linearization control for aerospace vehicle based on RBFNN disturbance observer[J]. Acta Aeronautica et Astronautica Sinica, 2007, 28(3): 673-677. | |
| 11 | 于靖,陈谋,姜长生. 基于观测器的非线性不确定系统自适应滑模控制[J]. 控制理论与应用,2014,31(8):993-999. |
| Yu Jing,Chen Mou,Jiang Chang-sheng. Adaptive sliding mode control for nonlinear uncertain systems based on disturbance observer[J]. Control Theory & Applications, 2014, 31(8):993-999. | |
| 12 | 王晓飞,邹早建,李铁山,等. 欠驱动船舶鲁棒路径跟踪控制器设计[J].航海工程, 2009, 38(5):16-18. |
| Wang Xiao-fei,Zou Zao-jian,Li Tie-shan,et al. Robust path following controller design of under-actuated ships[J]. Ship & Ocean Engineering, 2009,38(5):16-18. | |
| 13 | Chen Wen-hua, Yang Jun, Guo Lei, et al. Disturbance-observer-based control and related methos—an overview[J]. IEEE Transactions on Industrial Electronics, 2016, 63(2): 1083-1095. |
| 14 | Liu Cun-jia, Chen Wen-hua, Andrews J. Trajectory tracking of small helicopters using explicit nonlinear MPC and DOBC[J]. IFAC Proceedings Volumes, 2011,44(1):1498-1503. |
| 15 | 许坤,陈谋. 基于干扰观测器的移动机器人轨迹跟踪控制[J]. 应用科学学报,2016,34(2):177-189. |
| Xu Kun,Chen Mou. Control of trajectory tracking of mobile robots based on disturbance observer[J]. Journal of Applied Sciences, 2016,34(2):177-189. | |
| 16 | Yu S, Li X, Chen H, et al. Nonlinear model predictive control for path following problem[J]. International Journal of Robust & Nonlinear Control, 2015, 25(8):1168-1182. |
| 17 | Faulwasser T. Optimization-based Solutions to Constrained Trajectory-tracking and Path Following Problems[M]. Germany: Aachen, Shaker Verlag, 2013. |
| 18 | Liu Y, Yu S, Gao B, et al. Receding horizon following control of wheeled mobile robots: a case study[C]∥IEEE International Conference on Mechatronics & Automation, Beijing, China, 2015: 2571-2576. |
| 19 | 刘洋. 基于模型预测控制的移动机器人路径跟踪控制[D]. 长春:吉林大学通信工程学院,2016. |
| Liu Yang. Path following control of wheeled mobile robots based on model predictive control[D]. Chang-chun:College of Communication Engineering, Jilin University, 2016. | |
| 20 | Chen Wen-hua. Disturbance observer based control for nonlinear system[J]. IEEE/ASME Transaction on Mechatronics, 2004,9(4):706-710. |
| 21 | Yu S Y, Guo Y, Meng L Y, et al. MPC for path following problems of wheeled mobile robots[J]. IFAC, 2018,51(20):247-252. |
| 22 | 胡准庆,房海容,彭俊斌,等. 机器人奇异性分析[J]. 机器人技术与应用,2001(6):32-35. |
| Hu Huai-qing,Fang Hai-rong,Peng Jun-bin,et al. Robot singularity analysis[J]. Robot Technique and Application, 2001(6):32-35. | |
| 23 | 赵韩, 尹晓红, 吴焱明. 非完整约束AGV轨迹跟踪的非线性预测控制[J]. 中国机械工程, 2011,22(6):681-686. |
| Zhao Han,Yin Xiao-hong,Wu Yan-ming. Nonlinear model pridictive control of trajectory tracking for nonholonomic AGV[J]. China Mechanical Engineering, 2011,22(6):681-686. | |
| 24 | Khalil H K. Nonlinear Systems[M]. 3rd ed. Upper Saddle River: Prentice Hall, 2002. |
| [1] | Jia-xu ZHANG,Xin-zhi WANG,Jian ZHAO,Zheng-tang SHI. Path planning and discrete sliding mode tracking control for high⁃speed lane changing collision avoidance of vehicle [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(3): 1081-1090. |
| [2] | Ai-guo WU,Jun-qing HAN,Na DONG. Adaptive sliding mode control based on ultra⁃local model for robotic manipulator [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(5): 1905-1912. |
| [3] | Chao JIA,Hong-ze XU,Long-sheng WANG. Nonlinear model predictive control for automatic train operation based on multi⁃point model [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(5): 1913-1922. |
| [4] | Wei WANG,Jian-ting ZHAO,Kuan-rong HU,Yong-cang GUO. Trajectory tracking of robotic manipulators based on fast nonsingular terminal sliding mode [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 464-471. |
| [5] | Fu LIU,Yi AN,Bo DONG,Yuan-chun LI. Decentralized energy guaranteed cost decentralized optimal control of reconfigurable robots based on ADP [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(1): 342-350. |
| [6] | Xing-tian QU,Xue-xu WANG,Hui-chao SUN,Kun ZHANG,Long-wei YAN,Hong-yi WANG. Fuzzy self⁃adaptive PID control for fused deposition modeling 3D printer heating system [J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(1): 77-83. |
| [7] | Miao-miao MA,Jun-jun PAN,Xiang-jie LIU. Model predictive load frequency control of microgrid with electrical vehicles [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(5): 1644-1652. |
| [8] | Shu⁃you YU,Lei TAN,Wu⁃yang WANG,Hong CHEN. Control of active four wheel steering vehicle based ontriple⁃step method [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(3): 934-942. |
| [9] | Hai⁃ying WEN,Xiang REN,Wei⁃liang XU,Ming CONG,Wen⁃long QIN,Shu⁃hai HU. Bionic design and experimental test of temporomandibular joint for masticatory robot [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(3): 943-952. |
| [10] | GU Wan-li,WANG Ping,HU Yun-feng,CAI Shuo,CHEN Hong. Nonlinear controller design of wheeled mobile robot with H∞ performance [J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(6): 1811-1819. |
| [11] | LI Zhan-dong,TAO Jian-guo,LUO Yang,SUN Hao,DING Liang,DENG Zong-quan. Design of thrust attachment underwater robot system in nuclear power station pool [J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(6): 1820-1826. |
| [12] | WANG De-jun, WEI Wei-li, BAO Ya-xin. Actuator fault diagnosis of ESC system considering crosswind interference [J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(5): 1548-1555. |
| [13] | YAN Dong-mei, ZHONG Hui, REN Li-li, WANG Ruo-lin, LI Hong-mei. Stability analysis of linear systems with interval time-varying delay [J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(5): 1556-1562. |
| [14] | TIAN Yan-tao, ZHANG Yu, WANG Xiao-yu, CHEN Hua. Estimation of side-slip angle of electric vehicle based on square-root unscented Kalman filter algorithm [J]. 吉林大学学报(工学版), 2018, 48(3): 845-852. |
| [15] | ZHANG Shi-tao, ZHANG Bao, LI Xian-tao, WANG Zheng-xi, TIAN Da-peng. Enhancing performance of FSM based on zero phase error tracking control [J]. 吉林大学学报(工学版), 2018, 48(3): 853-858. |
|
||