基于位置跟踪的机械臂多电机新型滑模控制策略

doi:10.13229/j.cnki.jdxbgxb.20220742

摘要/Abstract

摘要：

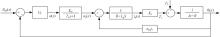
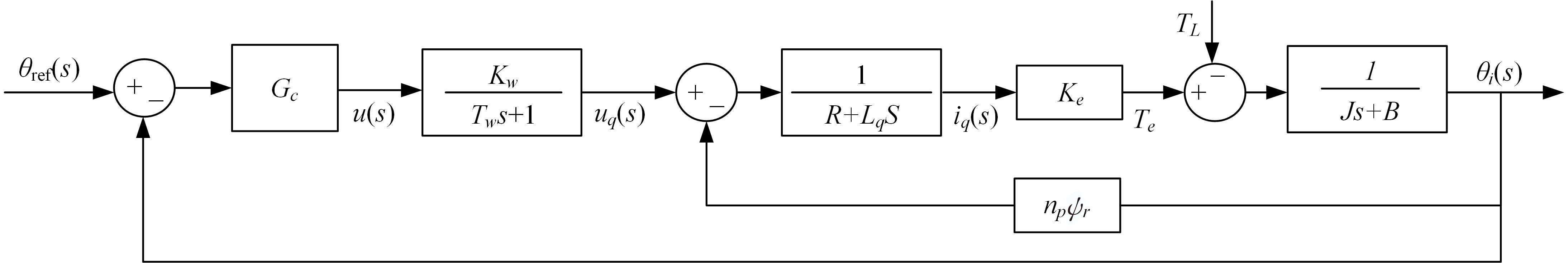
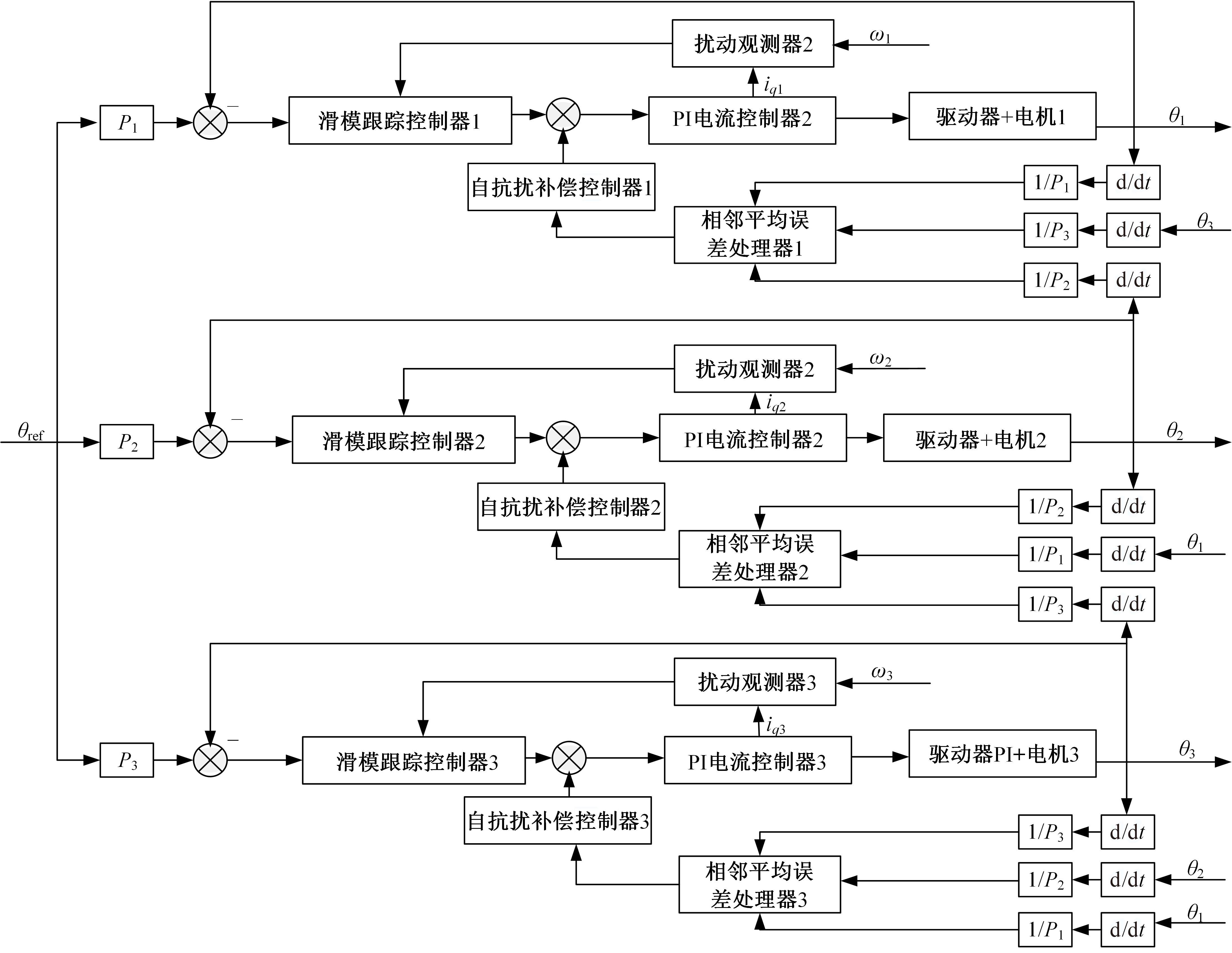
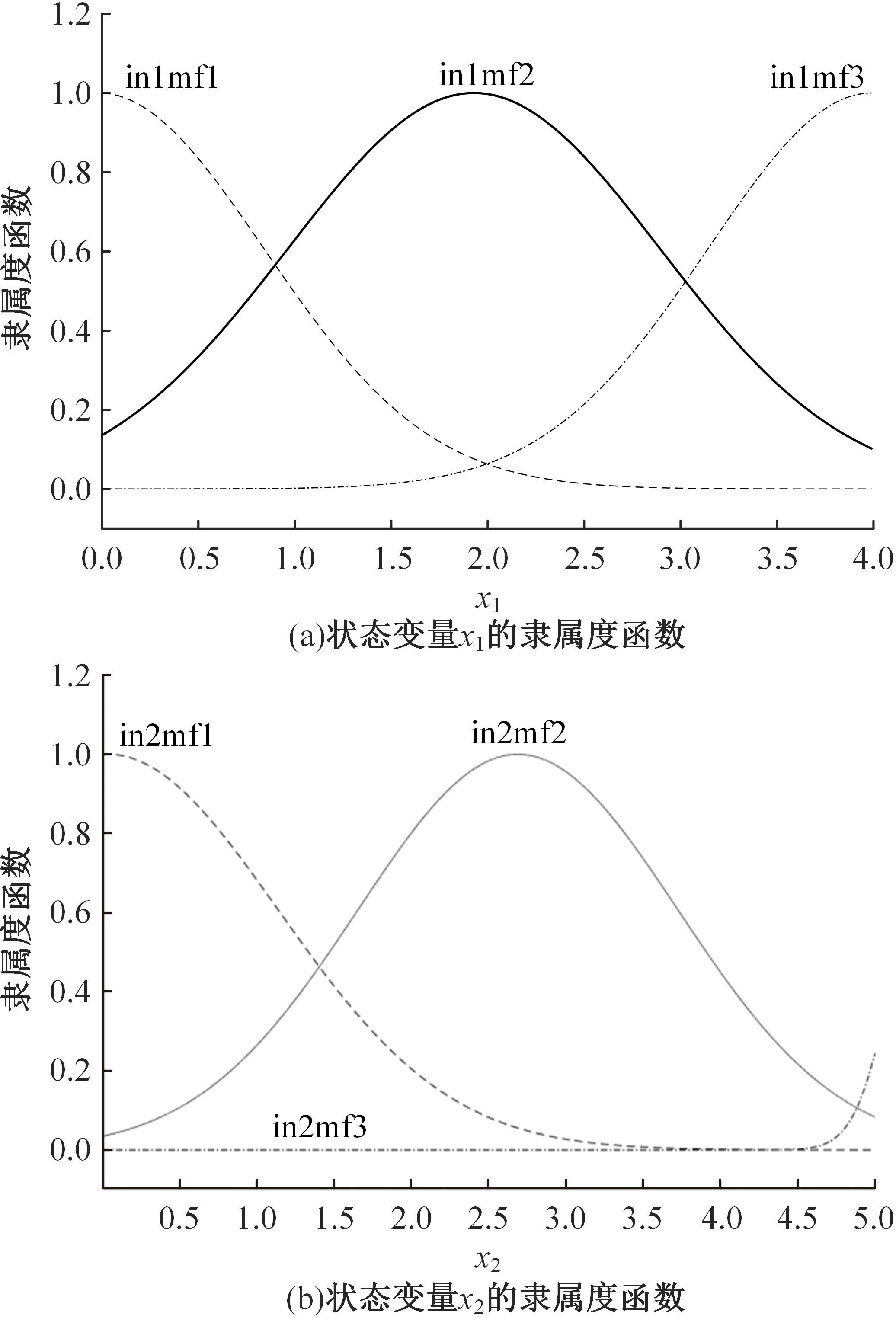
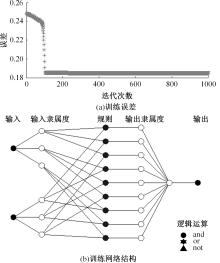
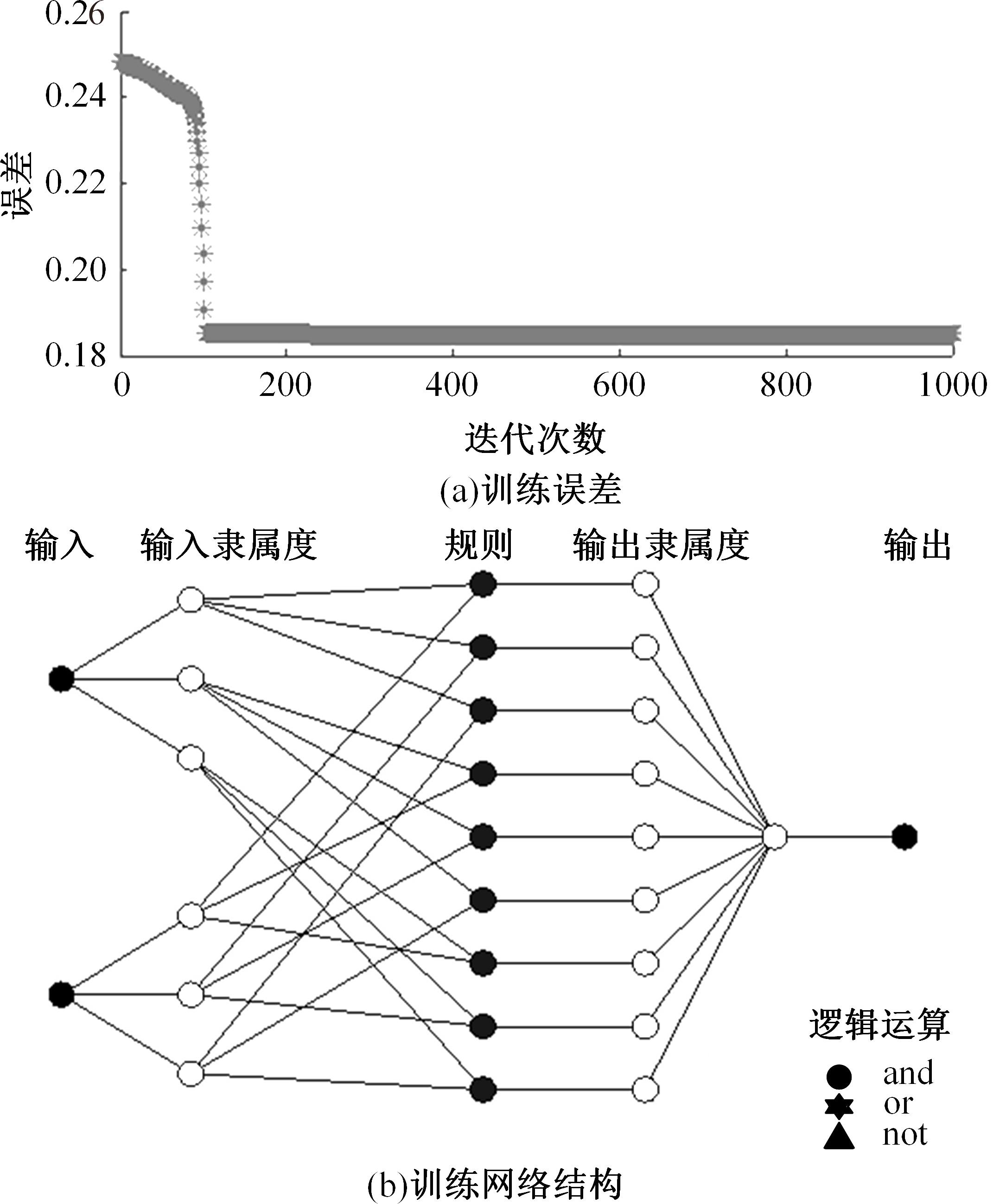
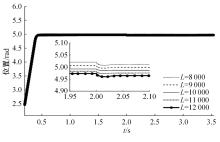
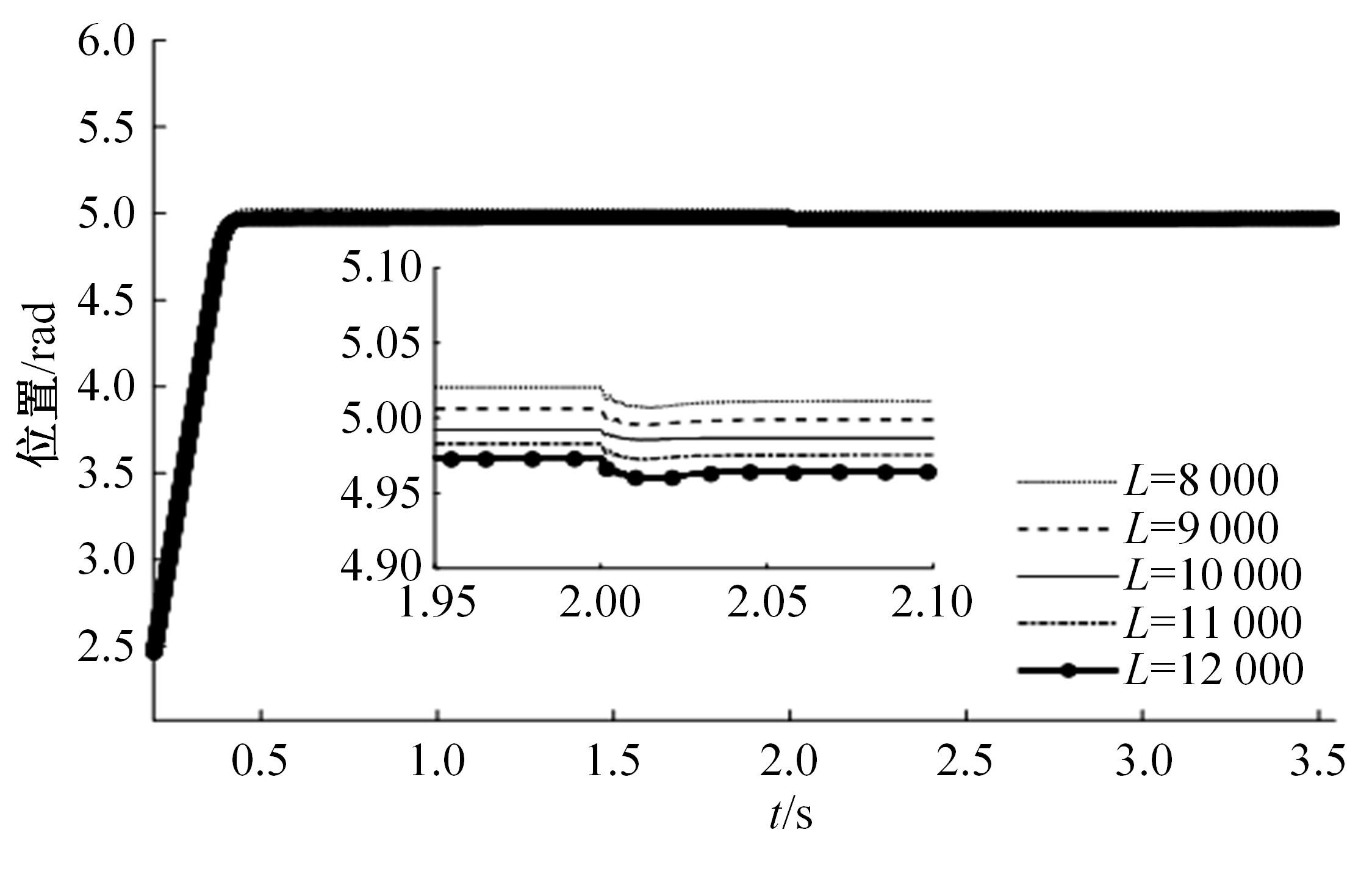
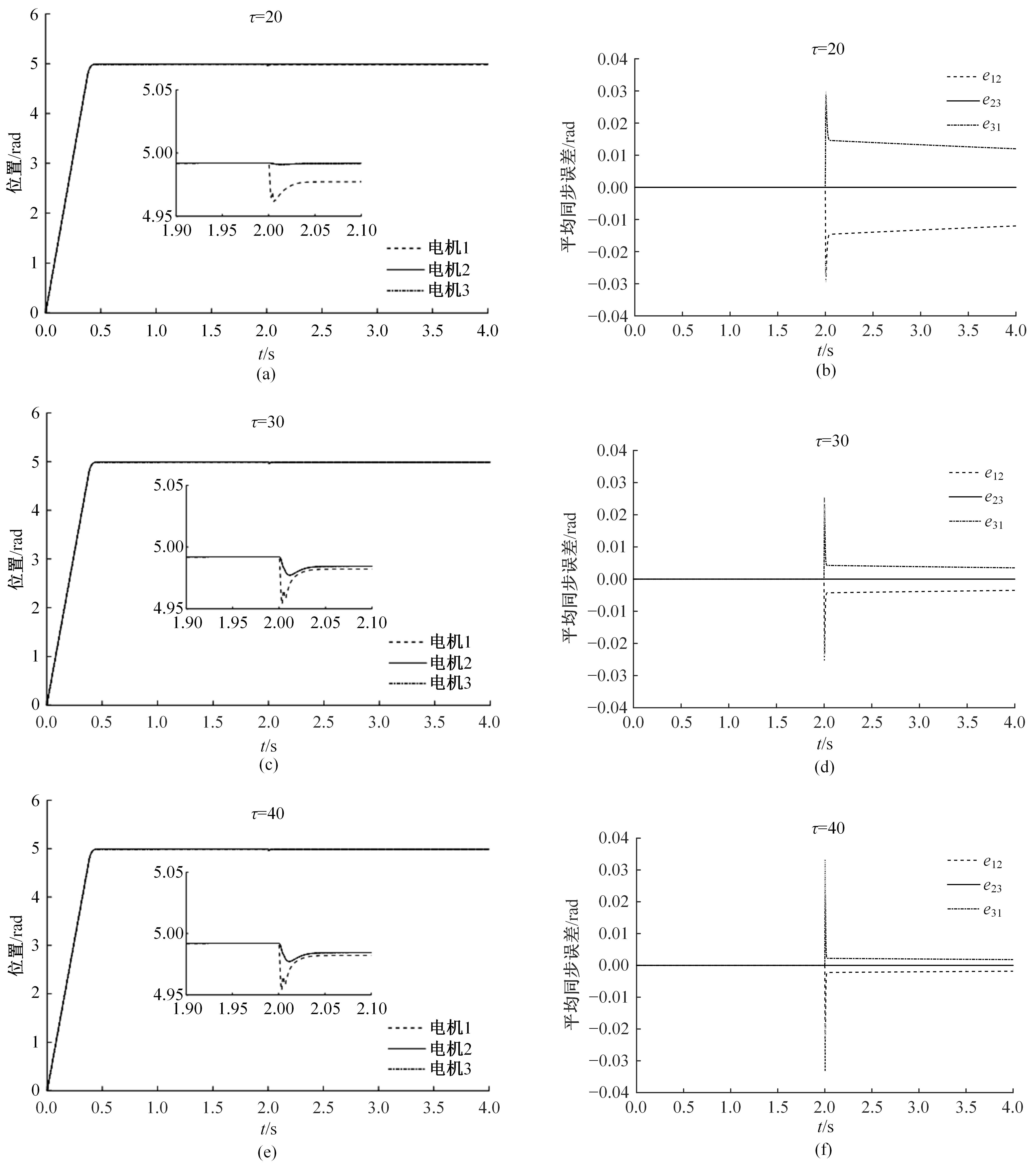
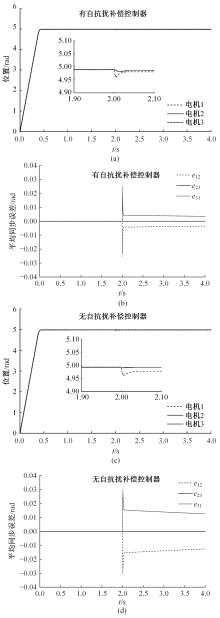
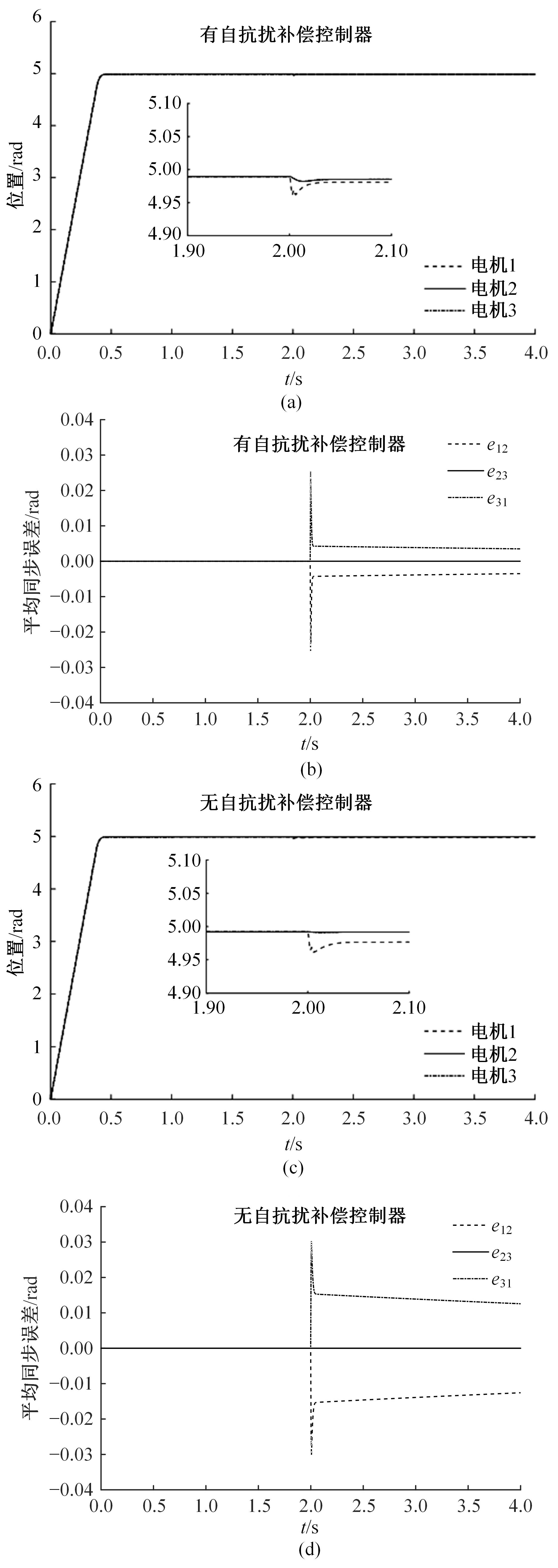
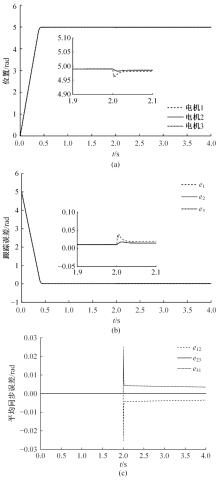
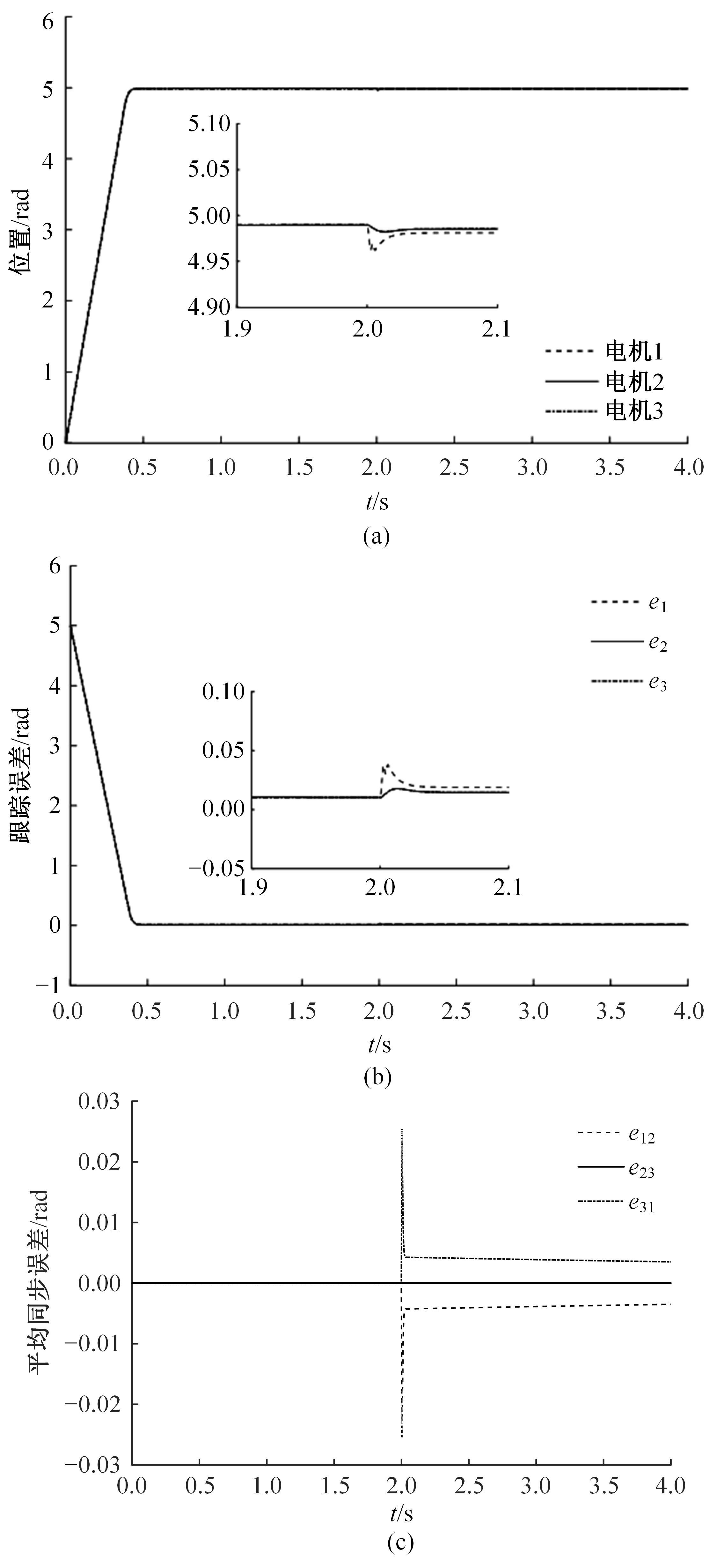
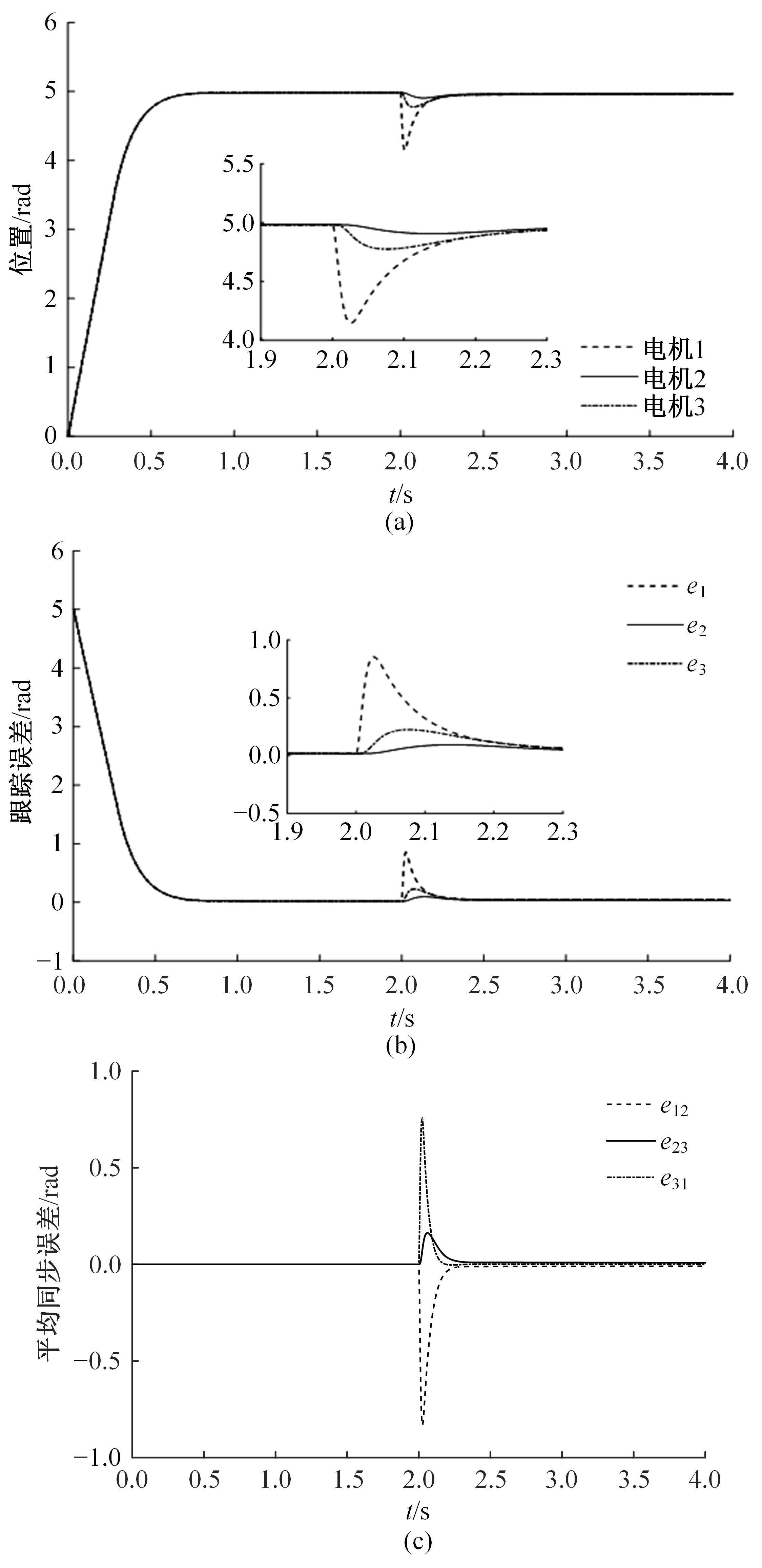
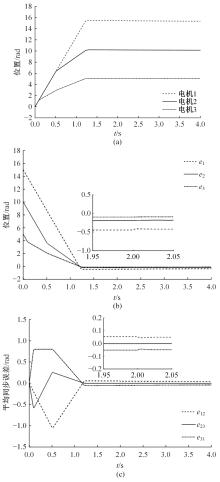
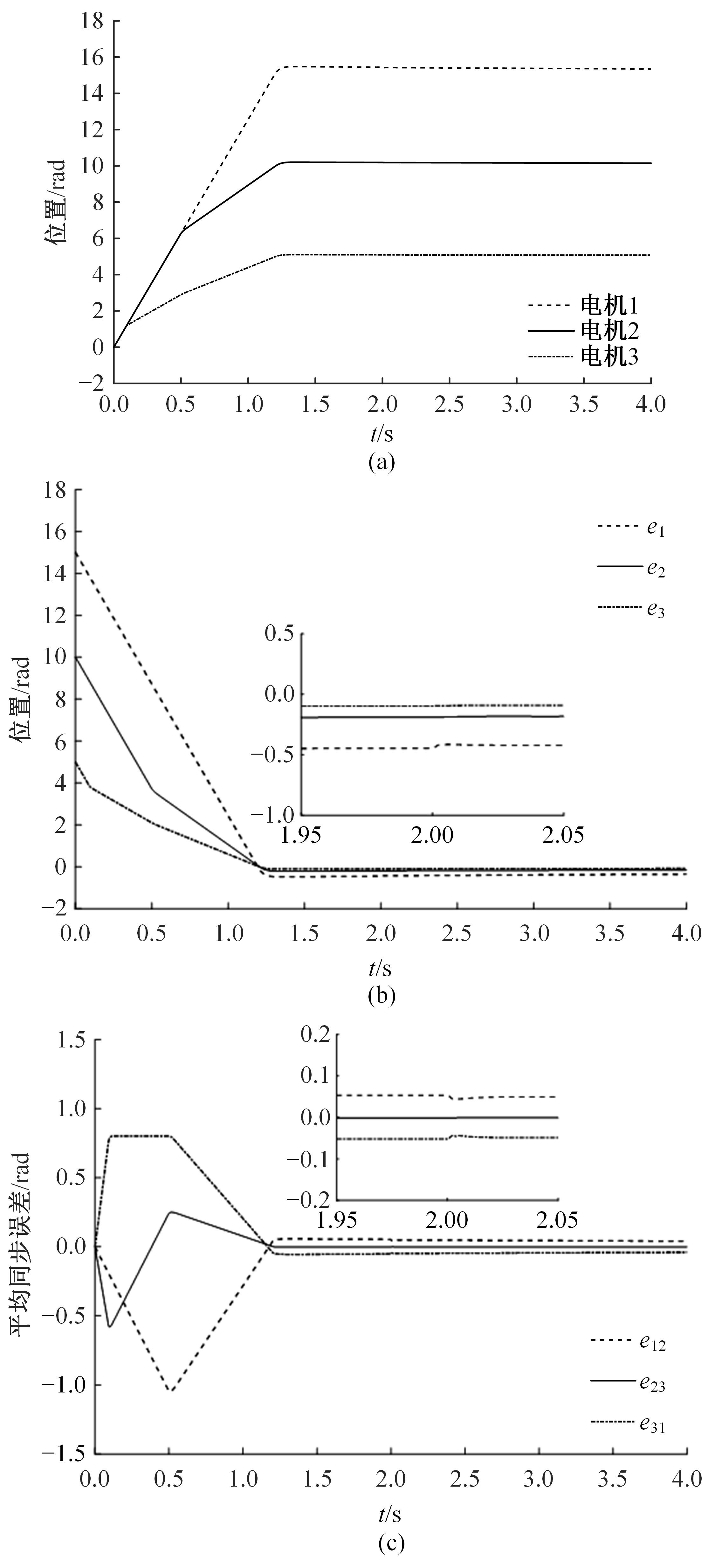
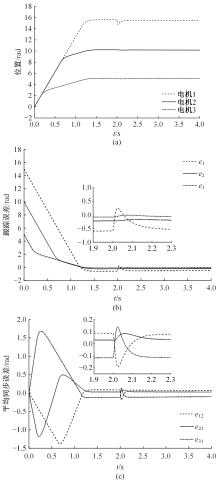
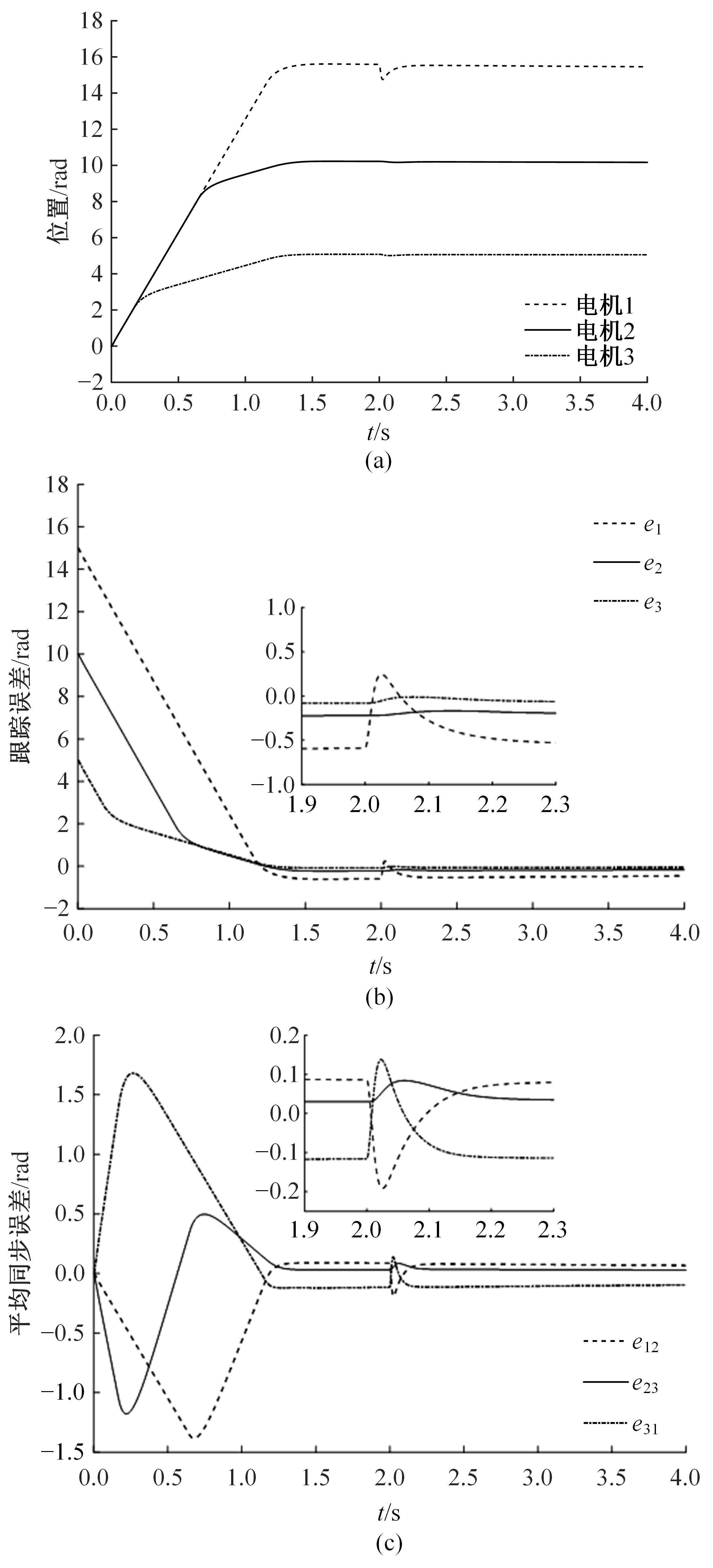
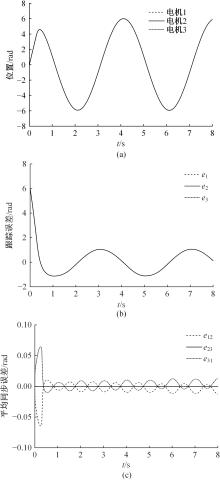
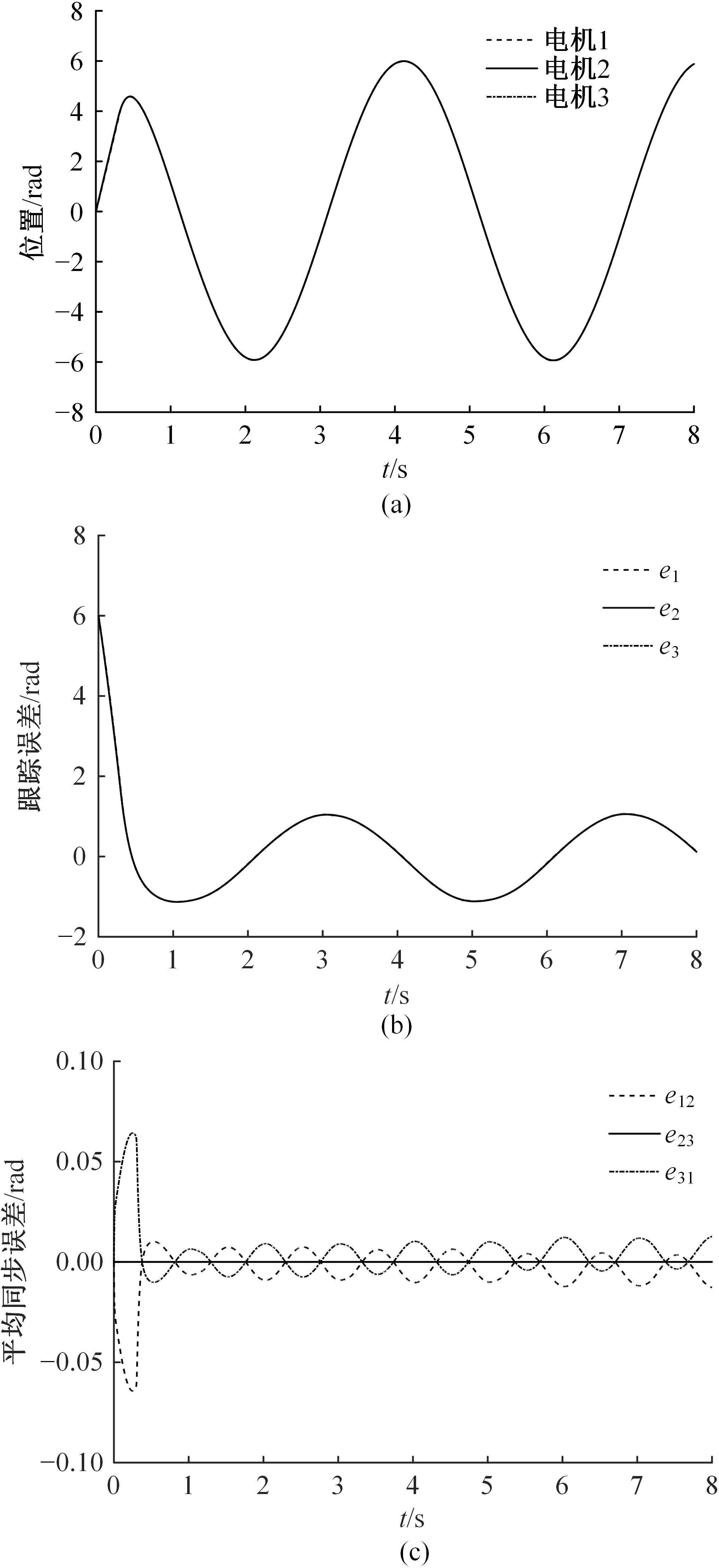
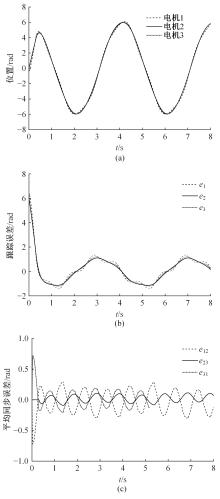
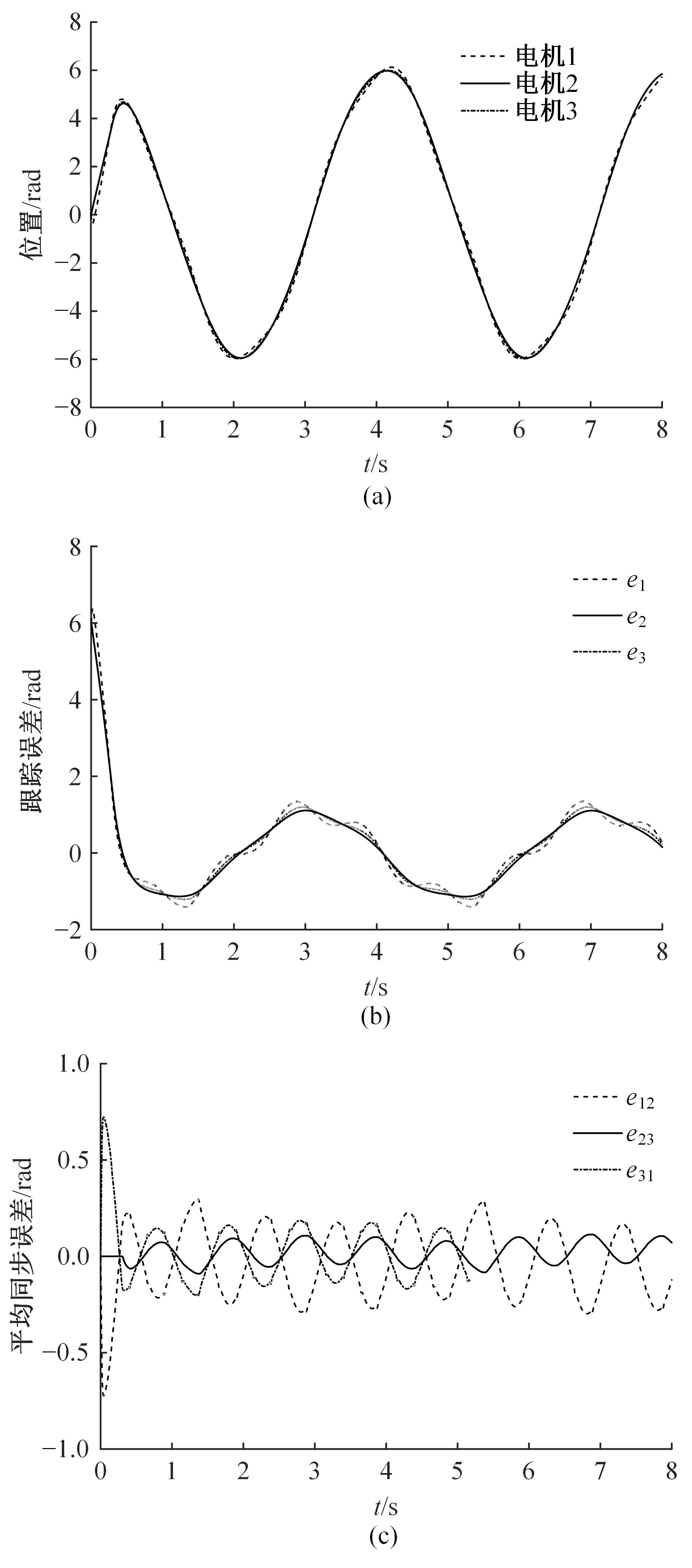
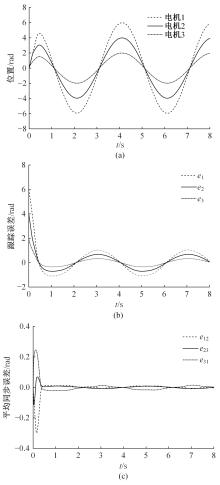
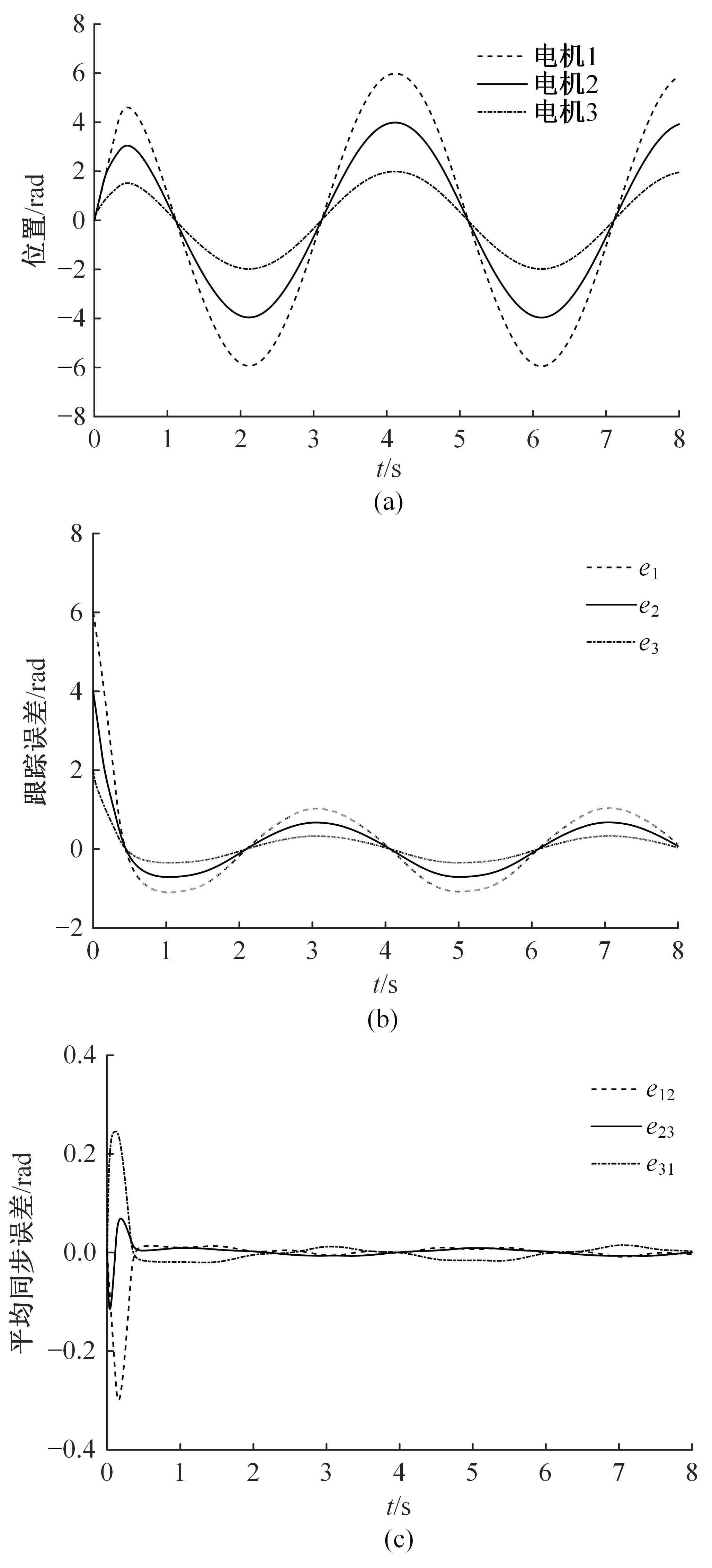
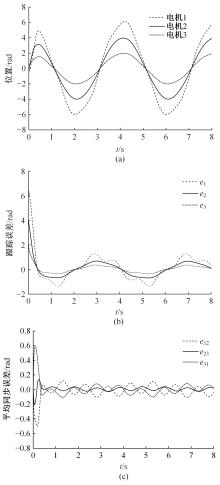
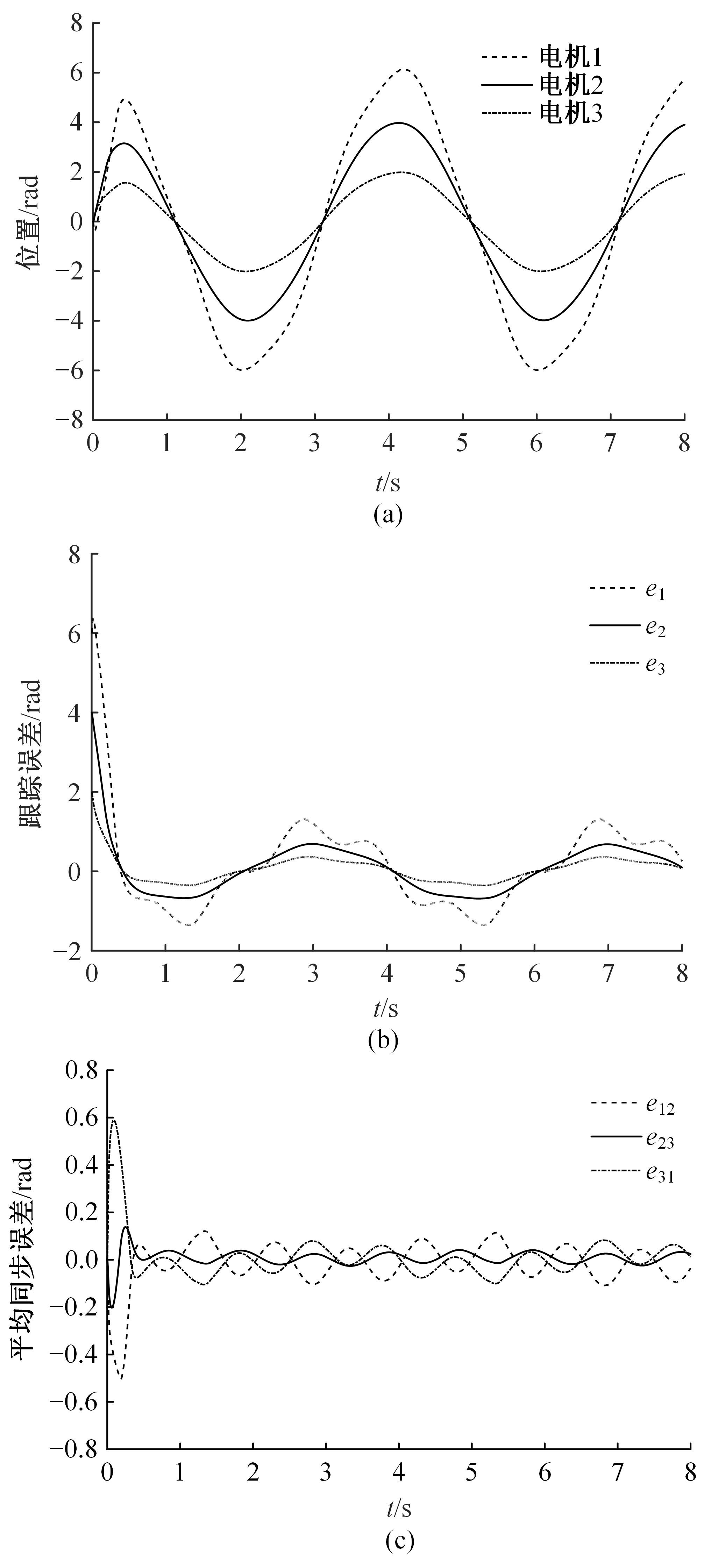
为了解决多电机驱动的机械臂系统在复杂环境运行时，其关节位置容易受到负载等外界干扰，导致位置的跟踪误差及同步误差较大，造成系统工作性能下降等问题，提出了一种新型多电机环形耦合控制策略（NRCC）。NRCC中设置了同步比例系数保证多电机协调运行；设计了自抗扰补偿控制器和相邻平均误差处理器，自抗扰补偿控制器通过相邻平均误差信号对电机位置控制信号进行二次补偿，减小了多电机间的同步误差；同时，提出了一种自适应神经模糊推理系统（ANFIS）优化的新型指数趋近率滑模跟踪控制器（ANFIS-SMC）和扰动观测器保证电机的位置跟踪性能。仿真结果表明：该控制策略有效地减小了多电机间的同步误差，保证了电机的高精度跟踪性能。

关键词: 控制理论与控制工程, 机械臂, 多电机, 环形耦合控制, 自适应神经模糊推理系统, 滑模跟踪控制器

Abstract:

When the multi-motors driven robotic arm system operates in a complex environment， its joint position is easily disturbed by external interference such as load， resulting in large tracking error and synchronization error， which causes system performance degradation. To solve this problem， a new multi-motors ring coupling control （NRCC） strategy is proposed. The synchronous proportional coefficient is set in NRCC to ensure the coordinated operation of multiple motors. The active disturbance rejection compensation controller （ADRCC） and the adjacent mean error processor are designed. The ADRCC compensates the position control signal of the motor twice through the adjacent mean error signal， which reduces the synchronization error between multi-motors. Meanwhile， a novel adaptive neuro-fuzzy inference system （ANFIS） optimizes exponential reaching rate sliding mode tracking controller （ANFIS-SMC） and disturbance observer are proposed to ensure the position tracking performance of motors. The simulation results show that the proposed control strategy effectively reduces the synchronization error between multiple motors and ensures the high-precision tracking performance of the motors.

Key words: control theory and control engineering, robotic arm, multi-motors, ring coupling control, adaptive neuro-fuzzy inference system, sliding mode tracking controller

中图分类号:

TP273

王宏志,王婷婷,兰淼淼,徐硕. 基于位置跟踪的机械臂多电机新型滑模控制策略[J]. 吉林大学学报(工学版), 2024, 54(5): 1443-1458.

Hong-zhi WANG,Ting-ting WANG,Miao-miao LAN,Shuo XU. A novel sliding mode control strategy of multi-motor for robot arm based on position tracking[J]. Journal of Jilin University(Engineering and Technology Edition), 2024, 54(5): 1443-1458.

图/表 19

图1

图2

图3

图4

图5

图6

表1

多电机同步控制策略主要相关参数"

策略	参数名称	数值
SMC+TRCC	滑模跟踪控制器	$ε = 2 ? 000$ ， $k = 1 ? 600$ ， $c = 0.1$
ANFIS-SMC+NRCC	滑模跟踪控制器	$ε 0 = 19 ? 000$ ， $μ = 0.03$ ， $k = 10 ? 000$ ， $δ = 0.1$ ， $c = 0.1$
	扰动观测器增益	$L = 10 ? 000$
	自抗扰补偿控制器	$γ = 300$ ， $β 01 = 10 ? 000$ ， $β 02 = 5 ? 000$ ， $b 0 = 1 ? 400$ ， $τ 0 = 30$

表1

图7

图8

表2

电机参数"

参数	值
定子电阻 $R$ /Ω	2.875
电感（ $L d / L q$ ）/H	0.008 5
磁链 $ψ$ /V-s	0.175
转动惯量 $J$ /（kg $?$ m²）	0.000 8
摩擦系数 $B$ /（（N $?$ m $?$ s）/rad）	0.001
极对数 $P$	4
逆变器增益 $K w$	500
逆变器时间常数 $T w$ /s	5×10^-6

表2

图9

图10

图11

图12

图13

图14

图15

图16

图17

参考文献 26

1	Aljalal M, Ibrahim S, Djemal R, et al. Comprehensive review on brain-controlled mobile robots and robotic arms based on electroencephalography signals[J]. Intelligent Service Robotics, 2020, 13(4): 539-563.
2	Grushko S, Vysock A, Oádal P, et al. Improved mutual understanding for human-robot collaboration: combining human-aware motion planning with haptic feedback devices for communicating panned trajectory[J]. Sensors, 2021, 21(11): No.21113673.
3	刘克平, 秦悦, 杨宏韬, 等. 多轴工业机器人非线性环形耦合补偿同步控制[J]. 机械科学与技术, 2018, 37(6): 910-914.
	Liu Ke-ping, Qin Yue, Yang Hong-tao, et al. Nonlinear ring coupling compensation synchronization control for multi-axis industrial robot[J]. Mechanical Science and Technology for Aerospace Engineering, 2018, 37(6): 910-914.
4	Cordeiro A, Manuel J, Pires V F. Performance of synchronized master-slave closed-loop control of AC electric drives using real time motion over ethernet (RTMoE)[J]. Mechatronics, 2020, 69(4): No.102400.
5	杨小庆, 赵振华. 多电机机器人模糊PID控制仿真研究[J]. 中国工程机械学报, 2020, 18(3): 248-252.
	Yang Xiao-qing, Zhao Zhen-hua. Simulation of fuzzy-PID control for multi-motor robot[J]. Chinese Journal of Construction Machinery, 2020, 18(3): 248-252.
6	Li Z, Zhang Q, An J, et al. Cross-coupling control method of the two-axis linear motor based on second-order terminal sliding mode[J]. Journal of Mechanical Science and Technology, 2022, 36(3): 1485-1495.
7	赵宏英, 曾彦, 廖丽. 基于改进交叉耦合的多永磁同步电机速度同步控制[J]. 机床与液压, 2021, 49(22): 44-51.
	Zhao Hong-ying, Zeng Yan, Liao Li. Speed synchronous control of multiple permanent magnet synchronous motors based on an improved cross-coupling structure[J]. Machine Tool & Hydraulics, 2021, 49(22): 44-51.
8	Deng Z, Shang J, Nian X. Synchronization controller design of two coupling permanent magnet synchronous motors system with nonlinear constraints[J]. ISA Transac Tions, 2015, 59: 243-255.
9	Sun D, Mills J K. Adaptive synchronized control for coordination of multirobot assembly tasks[J]. IEEE Transactions on Robotics and Automation, 2002, 18(4): 498-510.
10	刘玉强, 徐为民, 张梦杰, 等. 关于多电机位置同步优化控制仿真研究[J]. 计算机仿真, 2016, 33(11): 369-374.
	Liu Yu-qiang, Xu Wei-ming, Zhang Meng-jie, et al. Simulation research on optimal control of multi motor position synchronization[J]. Computer Simulation, 2016, 33(11): 369-374.
11	Perez-Pinal F J, Calderon G, Araujo-Vargas I. Relative coupling strategy[C]∥IEEE International Electric Machines and Drives Conference, Madison, USA, 2003: 1162-1166.
12	Zhang C, Xiao M, He J. Multimotor improved relative coupling cooperative control based on sliding-mode controller[J]. Mathematical Problems in Engineering, 2020, 2020(23): 1-10.
13	Shi T, Hao L, Qiang G, et al. An improved relative coupling control structure for multi-motor speed synchronous driving system[J]. IET Electric Power Applications, 2016, 10(6): 451-457.
14	Lorenz R D, Schmidt P B. Synchronized motion control for process automation[C]∥IEEE Conference Record of the Industry Applications Society Annual Meeting, San Diego, USA, 1989: 1693-1698.
15	Payette K. The virtual shaft control algorithm for synchronized motion control[C]∥IEEE Proceedings of the 1998 American Control Conference, Philadelphia, USA, 1998: 3008-3012.
16	Zhang C, Xiao M, He J, et al. Collaborative control of multimotor systems for fixed-time optimisation based on virtual main-axis speed compensation structure[J]. Complexity, 2021, 2021:1-15.
17	鲁文儒. 多关节机械臂同步控制策略研究[D]. 兰州: 兰州交通大学自动化与电气工程学院, 2021.
	Lu Wen-ru. Research on synchronous control strategy of multi-joint manipulator[D]. Lanzhou: School of Automation and Electrical Engineering of Lanzhou Jiaotong University, 2021.
18	Liu R, Sun J Z, Luo Y Q, et al. Research on multi-motor synchronization control based on the ring coupling strategy for cutter head driving system of shield machines[J]. Applied Mechanics & Materials, 2011, 52: 65-72.
19	Wu Y, Cheng Y, Wang Y. Research on a multi-motor coordinated control strategy based on fuzzy ring network control[J]. IEEE Access, 2020, 8: 39375-39388.
20	Sun D. Position synchronization of multiple motion axes with adaptive coupling control[J]. Automatica, 2003, 39(6): 997-1005.
21	丁威, 杜钦君, 宋传明, 等. 均值耦合多电机滑模速度同步控制[J]. 西安交通大学学报, 2022, 56(2): 159-170.
	Ding Wei, Du Qin-jun, Song Chuan-ming, et al. A synchronous control method for mean-coupled sliding mode speed of multi-motor [J]. Journal of Xi 'an Jiaotong University, 2022, 56(2): 159-170.
22	张润梅, 罗谷安, 袁彬, 等. 多关节机械臂干扰观测器的自适应滑模控制[J]. 机械科学与技术, 2021, 40(10): 1595-1602.
	Zhang Run-mei, Luo Gu-an, Yuan Bin, et al. Adaptive sliding mode control of disturbance observer for multi joint manipulator[J]. Mechanical Science and Technology for Aerospace Engineering, 2021, 40(10): 1595-1602.
23	贺志浩, 于海生. 基于自抗扰与观测器的环形耦合多电机协调滑模控制[J]. 微电机, 2021, 54(4): 48-55.
	He Zhi-hao, Yu Hai-sheng. Coordinated sliding mode control of ring-coupled multi-motor based on active disturbance rejection and observer[J]. Micromotors, 2021, 54(4): 48-55.
24	周挺, 徐宇工, 吴斌. 球形机器人的自适应分数阶PI^λD^μ滑模速度控制方法[J]. 吉林大学学报: 工学版, 2021, 51(2): 728-737.
	Zhou Ting, Xu Yu-gong, Wu Bin. Adaptive fractional PI^λD^μsliding mode control method for speed control of spherical robot[J]. Journal of Jilin University (Engineering and Technology Edition), 2021, 51(2): 728-737.
25	Vanchinathan K, Selvaganesan N. Adaptive fractional order PID controller tuning for brushless DC motor using artificial bee colony algorithm[J]. Results in Control and Optimization, 2021, 4(4): No.100032.
26	吴伟, 曾庆军, 王阳, 等. 水下机器人多电机协同模糊滑模控制研究[J]. 中国测试, 2021, 47(11): 101-106.
	Wu Wei, Zeng Qing-jun, Wang Yang, et al. Research on fuzzy sliding mode control of multi motor cooperative underwater robot[J]. China Measurement & Test, 2021, 47(11): 101-106.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

[1]	赵军,赵子亮,朱庆林,郭斌. 不依赖观测器的不确定性系统输出反馈鲁棒控制[J]. 吉林大学学报(工学版), 2024, 54(3): 828-835.
[2]	齐国元,陈浩. 基于观测器的四旋翼控制-抗扰-避障一体化[J]. 吉林大学学报(工学版), 2023, 53(3): 810-822.
[3]	陈珑茏,冯天宇,吕宗阳,吴玉虎. 共轴倾转旋翼无人机有限时间滑模姿态控制[J]. 吉林大学学报(工学版), 2023, 53(3): 883-890.
[4]	齐国元,李阔,王琨. 基于补偿函数观测器的四旋翼无人机姿态受限控制[J]. 吉林大学学报(工学版), 2023, 53(3): 853-862.
[5]	郭洪艳,于文雅,刘俊,戴启坤. 复杂场景智能车辆车道与速度一体化滚动优化决策[J]. 吉林大学学报(工学版), 2023, 53(3): 693-703.
[6]	王德军,张凯然,徐鹏,顾添骠,于文雅. 基于车辆执行驱动能力的复杂路况速度规划及控制[J]. 吉林大学学报(工学版), 2023, 53(3): 643-652.
[7]	徐卓君,王耀祥,黄兴,彭程. 多无人机地面移动目标搜寻和定位[J]. 吉林大学学报(工学版), 2023, 53(3): 832-840.
[8]	何德峰,周丹,罗捷. 跟随式车辆队列高效协同弦稳定预测控制[J]. 吉林大学学报(工学版), 2023, 53(3): 726-734.
[9]	张铮,朱齐丹,吕晓龙,樊星. 冗余机械臂运动学逆解的求解优化方法[J]. 吉林大学学报(工学版), 2023, 53(12): 3379-3387.
[10]	马彦,郭则宣. 基于改进BPNN⁃MPF算法的锂离子电池SoE估计[J]. 吉林大学学报(工学版), 2023, 53(1): 263-272.
[11]	王宏志,王婷婷,胡黄水,鲁晓帆. 基于Q学习优化BP神经网络的BLDCM转速PID控制[J]. 吉林大学学报(工学版), 2021, 51(6): 2280-2286.
[12]	冯建鑫,王强,王雅雷,胥彪. 基于改进量子遗传算法的超声电机模糊PID控制[J]. 吉林大学学报(工学版), 2021, 51(6): 1990-1996.
[13]	吴爱国,韩俊庆,董娜. 基于极局部模型的机械臂自适应滑模控制[J]. 吉林大学学报(工学版), 2020, 50(5): 1905-1912.
[14]	马彦,黄健飞,赵海艳. 基于车间通信的车辆编队控制方法设计[J]. 吉林大学学报(工学版), 2020, 50(2): 711-718.
[15]	王伟,赵健廷,胡宽荣,郭永仓. 基于快速非奇异终端滑模的机械臂轨迹跟踪方法[J]. 吉林大学学报(工学版), 2020, 50(2): 464-471.