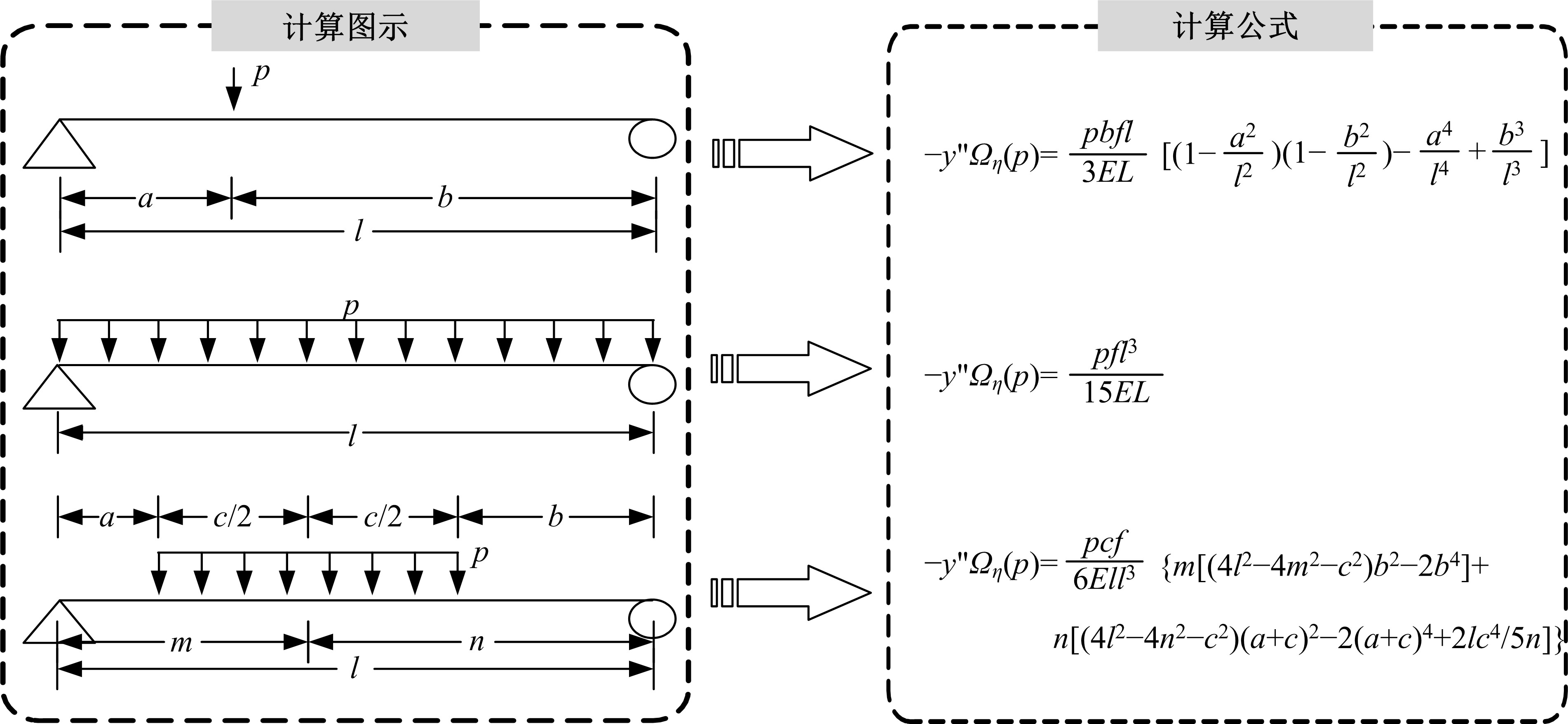
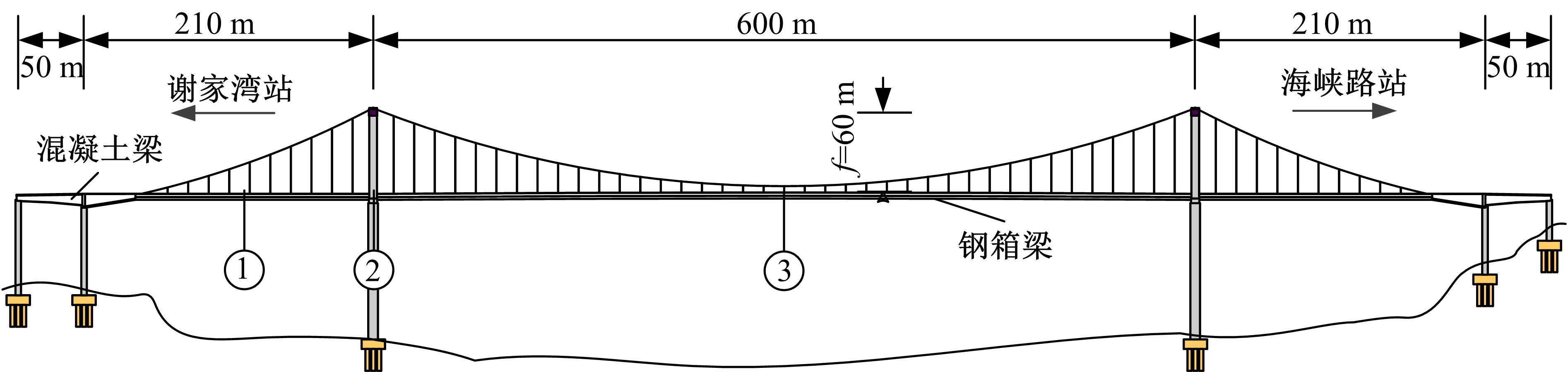
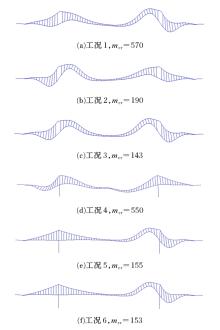
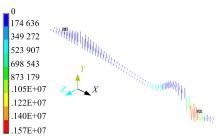
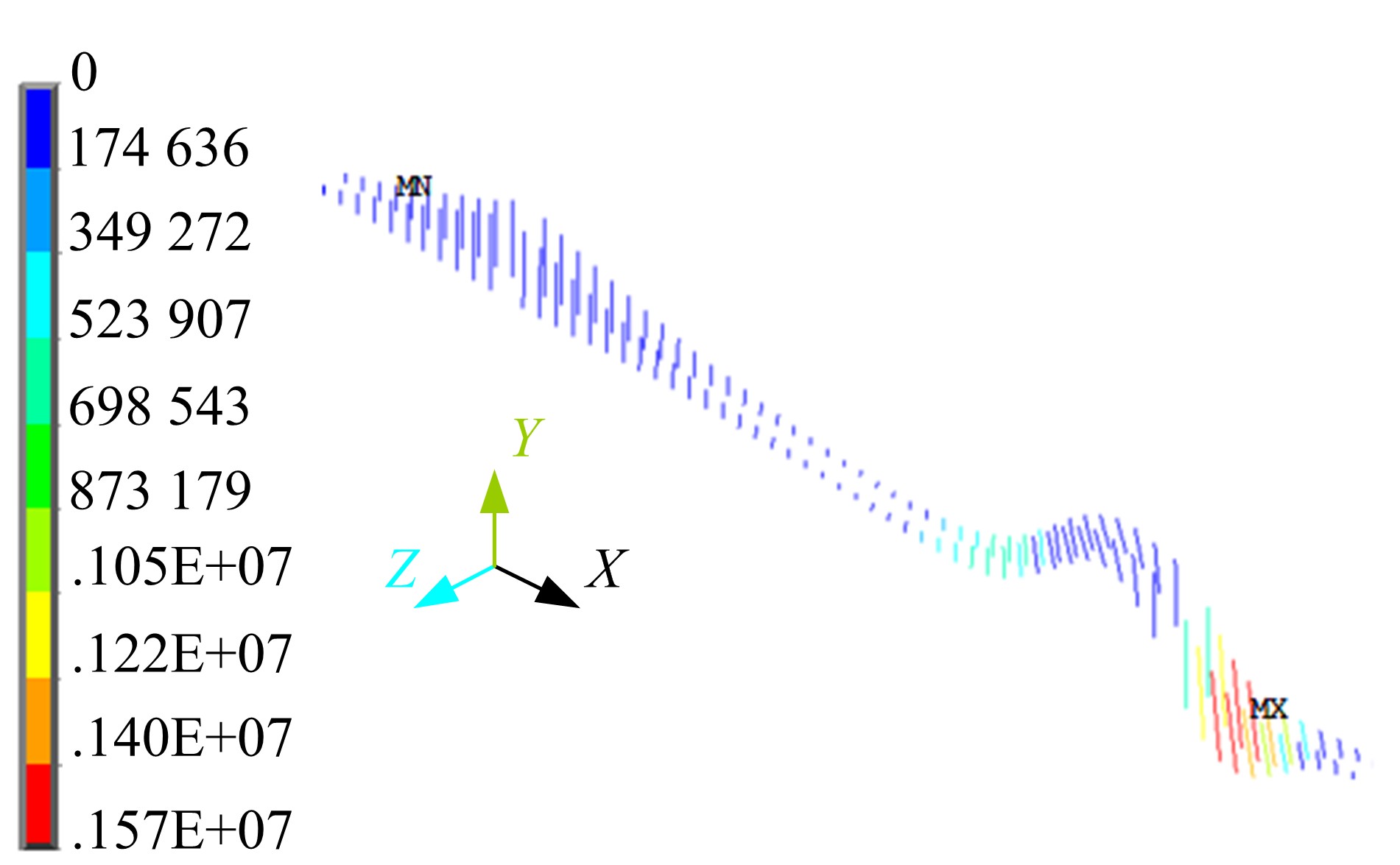
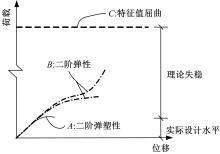
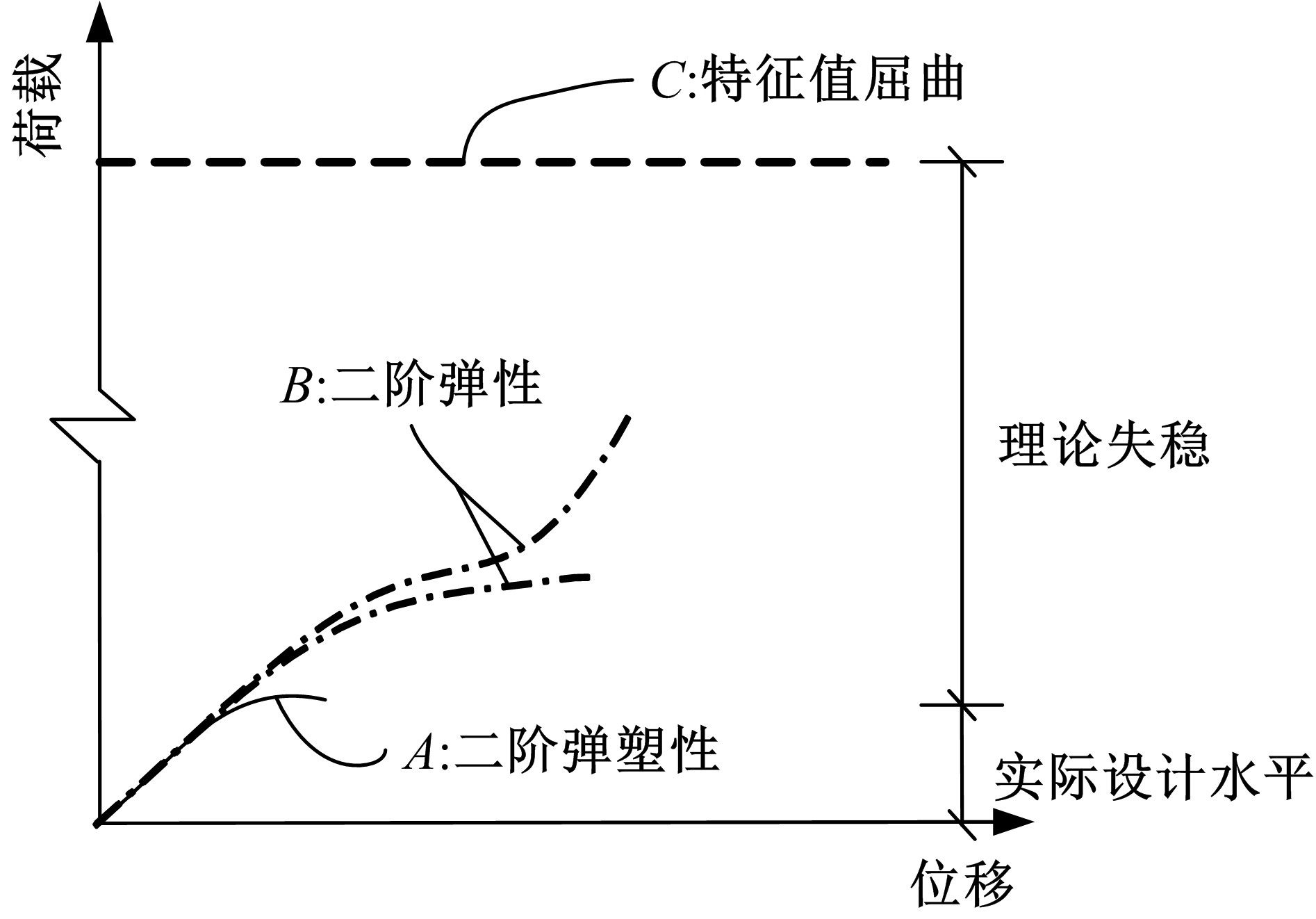
Journal of Jilin University(Engineering and Technology Edition) ›› 2019, Vol. 49 ›› Issue (5): 1500-1508.doi: 10.13229/j.cnki.jdxbgxb20180178
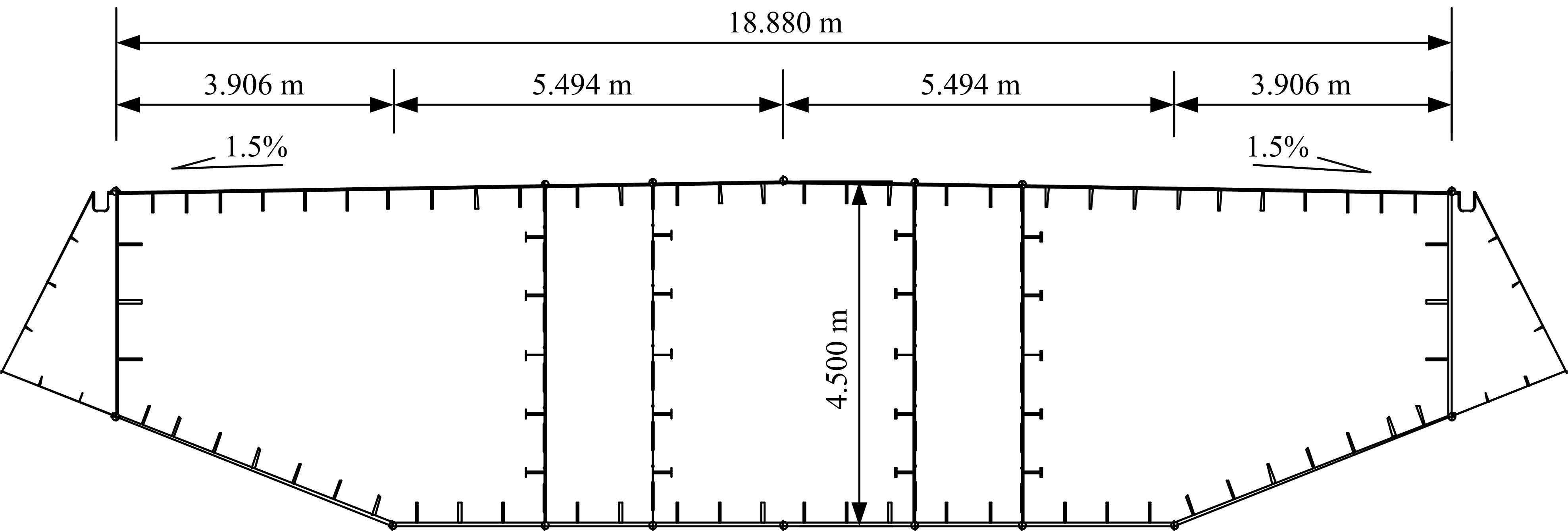
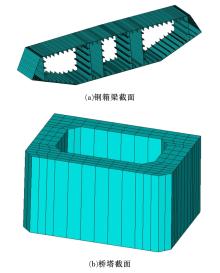
In-plane stability of self-anchored suspension bridge
Lun-hua BAI( ),Rui-li SHEN(
),Rui-li SHEN( ),Xing-biao ZHANG,Lu WANG
),Xing-biao ZHANG,Lu WANG
- Department of Bridge Engineering, Southwest Jiaotong Unviersity, Chengdu 610031, China
CLC Number:
- U448.25
| 1 | Nie Jian-guo , Zhou Meng , Wang Yu-hang , et al . Cable anchorage system modeling methods for self-anchored suspension bridges with steel box girders[J]. Journal of Bridge Engineering, 2014, 19(2): 172-185. |
| 2 | 王邵锐, 周志祥, 高燕梅, 等 . 考虑缆-梁联合作用的自锚式悬索桥恒载状态计算方法研究[J]. 土木工程学报, 2015, 48(8): 70-76. |
| Wang Shao-rui , Zhou Zhi-xiang , Gao Yan-mei , et al . Study on the calculation method of the dead load state for the self-anchored suspension bridge considering the joint action of cable-stiffening girder[J]. China Civil Engineering Journal, 2015, 48(8): 70-76. | |
| 3 | 李建慧, 李爱群 . 自锚式悬索桥静力随机分析与可靠度评估[J]. 中国公路学报, 2012, 25(6): 74-79. |
| Li Jian-hui , Li Ai-qun . Stochastic analysis of static charateristics and reliability assessment for self-anchored suspension bridge[J]. China Journal of Highway and Transport, 2012, 25(6): 74-79. | |
| 4 | 王保群, 张强勇, 张凯, 等 . 自锚式斜拉-悬吊协作体系桥梁动力性能[J]. 吉林大学学报: 工学版, 2009, 39(3): 686-690. |
| Wang Bao-qun , Zhang Qiang-yong , Zhang Kai , et al . Dynamic characteristics for self-anchored cable-stayed suspension bridges[J]. Journal of Jilin University (Engineering and Technology Edition), 2009, 39(3): 686-690. | |
| 5 | 沈锐利, 齐东春, 唐茂林 . 杭州江东大桥静力特性全桥模型试验研究[J]. 土木工程学报, 2011, 44(1): 74-80. |
| Shen Rui-li , Qi Dong-chun , Tang Mao-lin . Model test study of the static property of the Jiangdong Bridge in Hangzhou[J]. China Civil Engineering Journal, 2011, 44(1): 74-80. | |
| 6 | 胡建华, 沈锐利, 张贵明, 等 . 佛山平胜大桥全桥模型试验研究[J]. 土木工程学报, 2007, 40(5): 17-25. |
| Hu Jian-hua , Shen Rui-li , Zhang Gui-ming , et al . Total bridge model study of the Pingsheng Bridge in Foshan[J]. China Civil Engineering Journal, 2007, 40(5): 17-25. | |
| 7 | 王桢, 吴海军, 周志祥, 等 . 大跨径自锚式悬索桥吊索索力相邻影响分析[J]. 土木工程学报, 2016, 49(6): 51-60. |
| Wang Zhen , Wu Hai-jun , Zhou Zhi-xiang , et al . Analysis on near influence of cable force in large-span self-anchored suspension bridges[J]. China Civil Engineering Journal, 2016, 49(6): 51-60. | |
| 8 | 胡建华, 王连华, 沈锐利, 等 . 大跨度自锚式悬索桥稳定性研究[J]. 湖南大学学报: 自然科学版, 2008, 35(7): 12-15. |
| Hu Jian-Hua , Wang Lian-hua , Shen Rui-li , et al . Research on the stability of long span self-anchored suspension bridges[J]. Journal of Hunan University (Natural Sciences), 2008, 35(7): 12-15. | |
| 9 | Jung M R , Shin S U , Attard M M , et al . Deflection theory for self-anchored suspension bridges under live load[J]. Journal of Bridge Engineering, 2014, 20(7): 1-19. |
| 10 | Jung M R , Jang M J , Attard M M , et al . Elastic stability behavior of self-anchored suspension bridges by the deflection theory[J]. International Journal of Structural Stability & Dynamics, 2017, 17(4): 1-23. |
| 11 | 李立峰, 程翔云 . 代换梁法在自锚式悬索桥上的推广应用[J]. 工程力学, 2008, 25(8): 212-217. |
| Li Li-feng , Cheng Xiang-yun . Extensional application of substitutional beam method to self-anchored suspension bridge[J]. Engineering Mechanics, 2008, 25(8): 212-217. | |
| 12 | 赵维贺 . 新型自锚式悬索桥的稳定性及极限承载力分析[D]. 大连: 大连理工大学土木工程学院, 2007. |
| Zhao Wei-he . The buckle and ultimate bearing capacity analysis for a new style self-anchored suspension bridge[D]. Dalian: School of Civil Engieering, Dalian University of Technology, 2007. | |
| 13 | 铁道第三勘察设计院 . 铁路桥涵设计基本规范[M]. 2版. 北京: 中国铁道出版社, 2005. |
| 14 | 重庆交通科研设计院 . 公路斜拉桥设计细则(JTG/T D65-01-2007)[M]. 北京: 人民交通出版社, 2007. |
| 15 | 沈锐利, 成新, 白伦华, 等 . 自锚式悬索桥极限承载力及安全性评价方法研究[J]. 铁道学报, 2017, 39(11): 89-96. |
| Shen Rui-li , Cheng Xin , Bai Lun-hua , et al . Study on static ultimate bearing capacity and safety evaluation method of self-anchored suspension bridges[J]. Journal of the China Railway Society, 2017, 39(11): 89-96. |
| [1] | Shi⁃cheng WAN,Qiao HUANG,Jian GUAN,Zhao⁃yuan GUO. Strengthening of continuous steel⁃concrete composite beams in negative moment region using prestressed carbon fiber⁃reinforced polymer plates [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(4): 1114-1123. |
| [2] | Jin⁃gang ZHAO,Ming ZHANG,Yu⁃lin ZHAN,Ming⁃zhi XIE. Damage criterion of reinforced concrete pier based on plastic strain energy density [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(4): 1124-1133. |
| [3] | Wan⁃heng LI,Lin SHEN,Shao⁃peng WANG,Shang⁃chuan ZHAO. Damage assessment of bridge construction based onmulti⁃stage subregion mobile test [J]. Journal of Jilin University(Engineering and Technology Edition), 2019, 49(3): 773-780. |
| [4] | HUI Ying-xin,MAO Ming-jie,LIU Hai-feng,ZHANG Shang-rong. Influence of structural seismic response of bridges crossing active fault [J]. Journal of Jilin University(Engineering and Technology Edition), 2018, 48(6): 1725-1734. |
| [5] | ZHENG Yi-feng, ZHAO Qun, BAO Wei, LI Zhuang, YU Xiao-fei. Wind resistance performance of long-span continuous rigid-frame bridge in cantilever construction stage [J]. 吉林大学学报(工学版), 2018, 48(2): 466-472. |
| [6] | GONG Ya-feng, HE Yu-long, TAN Guo-jin, SHEN Yang-fan. Anti-overturning stability analysis for three-span continuous curved girder bridge with single column pier [J]. 吉林大学学报(工学版), 2018, 48(1): 133-140. |
| [7] | WEI Zhi-gang, LIU Han-bing, SHI Cheng-lin, GONG Ya-feng. Calculation of transverse distribution coefficient of simply supported beam bridge with effect of bridge deck pavement [J]. 吉林大学学报(工学版), 2018, 48(1): 105-112. |
| [8] | WEI Zhi-gang, SHI Cheng-lin, LIU Han-bing, ZHANG Yun-long. Dynamic characteristics of steel-concrete composite simply supported beam under vehicles [J]. 吉林大学学报(工学版), 2017, 47(6): 1744-1752. |
| [9] | ZHANG Yun-long, LIU Zhan-ying, WU Chun-li, WANG Jing. Static and dynamic responses of steel-concrete composite beams [J]. 吉林大学学报(工学版), 2017, 47(3): 789-795. |
| [10] | LIU Han-bing, SHI Cheng-lin, TAN Guo-jin. Finite element solution of composite beam with effect of shear slip [J]. 吉林大学学报(工学版), 2016, 46(3): 792-797. |
| [11] | TAN Guo-jin, LIU Zi-yu, WEI Hai-bin, WANG Long-lin. Calculation of natural frequency of simply supported beam bridge reinforced with eccentric prestressed tendons [J]. 吉林大学学报(工学版), 2016, 46(3): 798-803. |
| [12] | CAO Shan-shan, LEI Jun-qing. Fatigue life prediction of steel structure considering interval uncertainty [J]. 吉林大学学报(工学版), 2016, 46(3): 804-810. |
| [13] | XIAO Yun, LEI Jun-qing, ZHANG Kun, LI Zhong-san. Fatigue stiffness degradation of prestressed concrete beam under multilevel amplitude cycle loading [J]. 吉林大学学报(工学版), 2013, 43(03): 665-670. |
| [14] | JIAO Chang-ke, LI Ai-qun, WU Xiao-ping. Double-deck long-span cable-stayed bridge seismic response to multi-support excitation [J]. , 2012, 42(04): 910-917. |
| [15] | LIU Han-bing, ZHENG Ji-guang, ZOU Pin-de. Calculation on bearing capacity of eccentric compression reinforced concrete short column with circular combined-section [J]. 吉林大学学报(工学版), 2011, 41(增刊2): 159-163. |
|
||