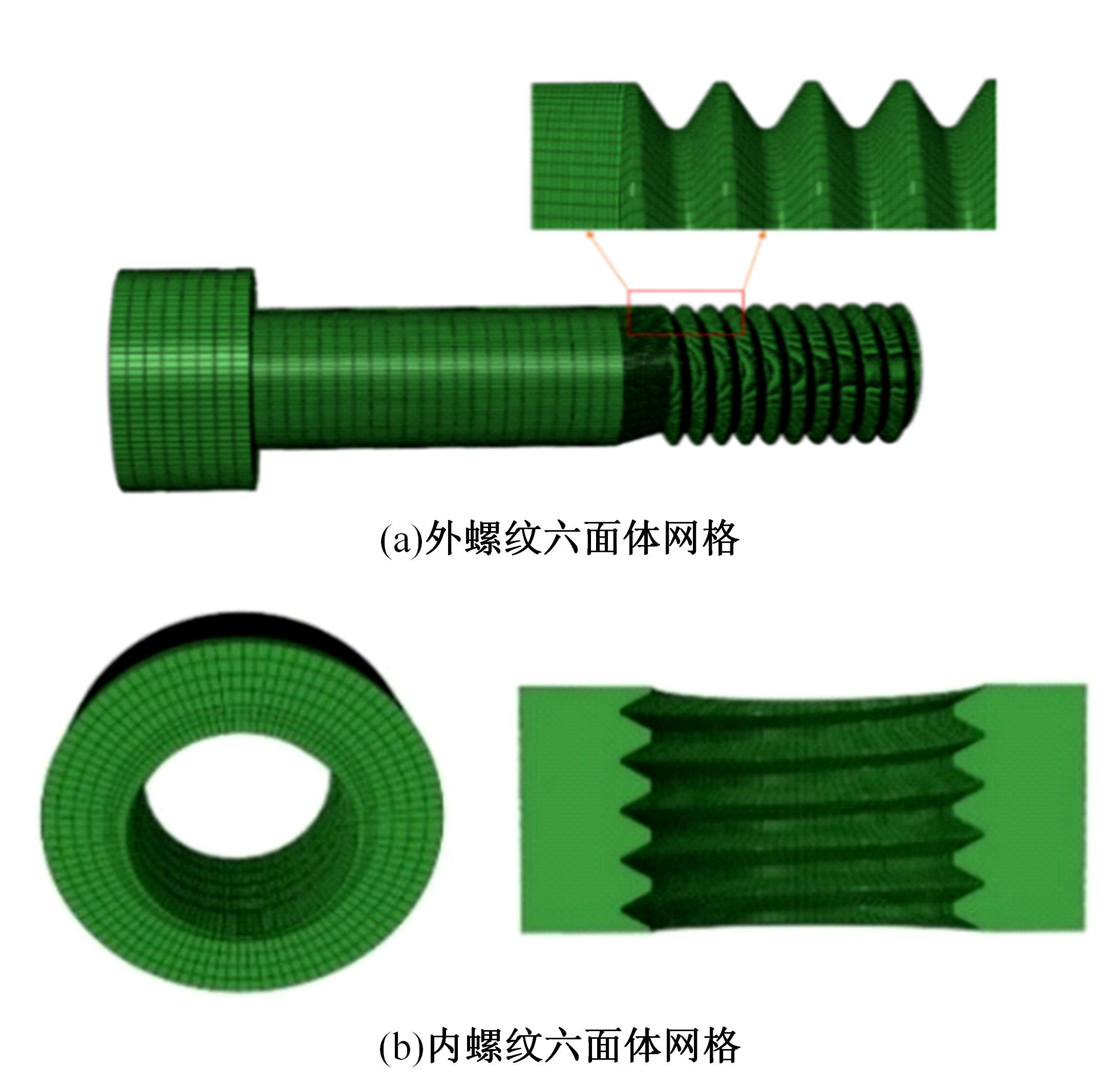
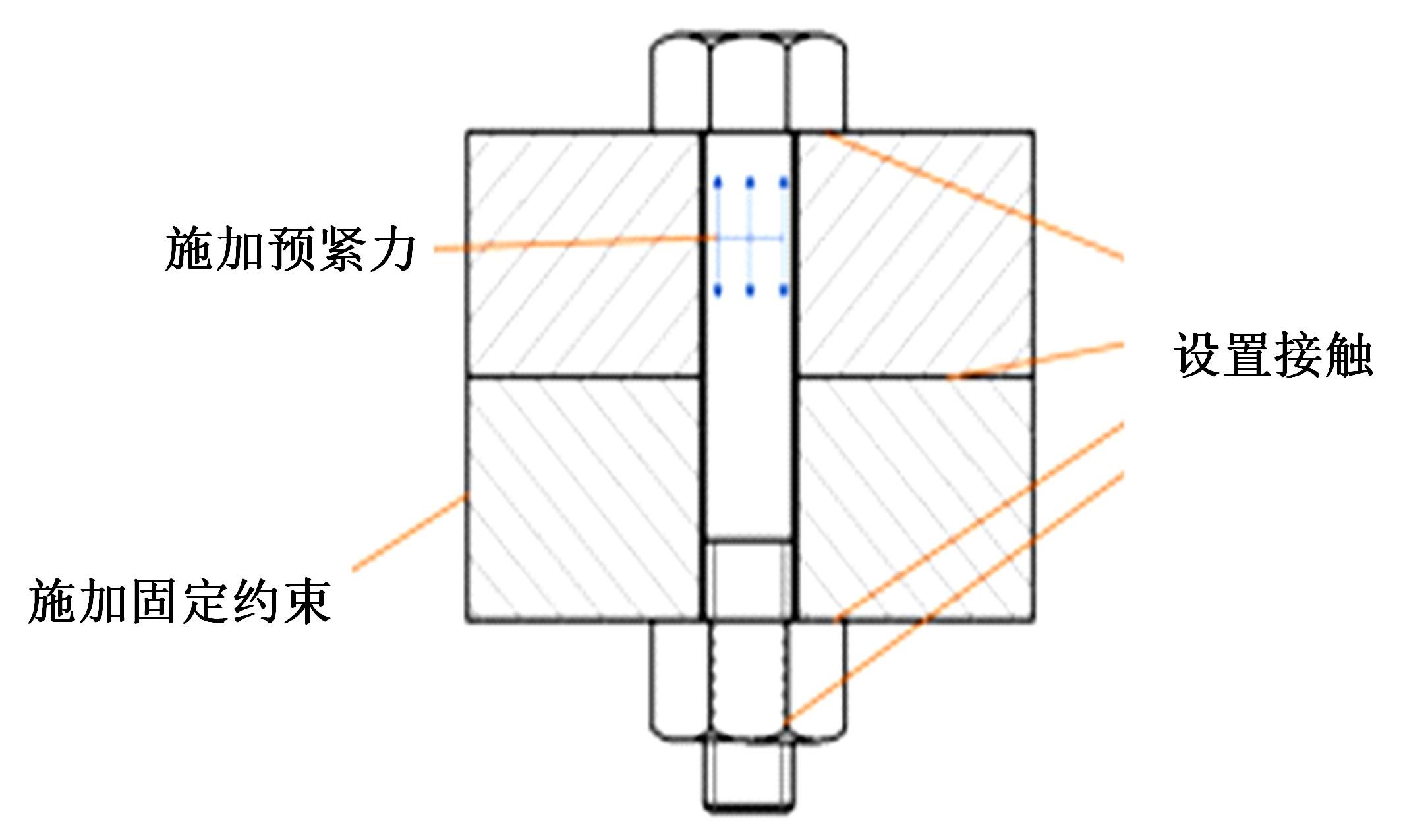
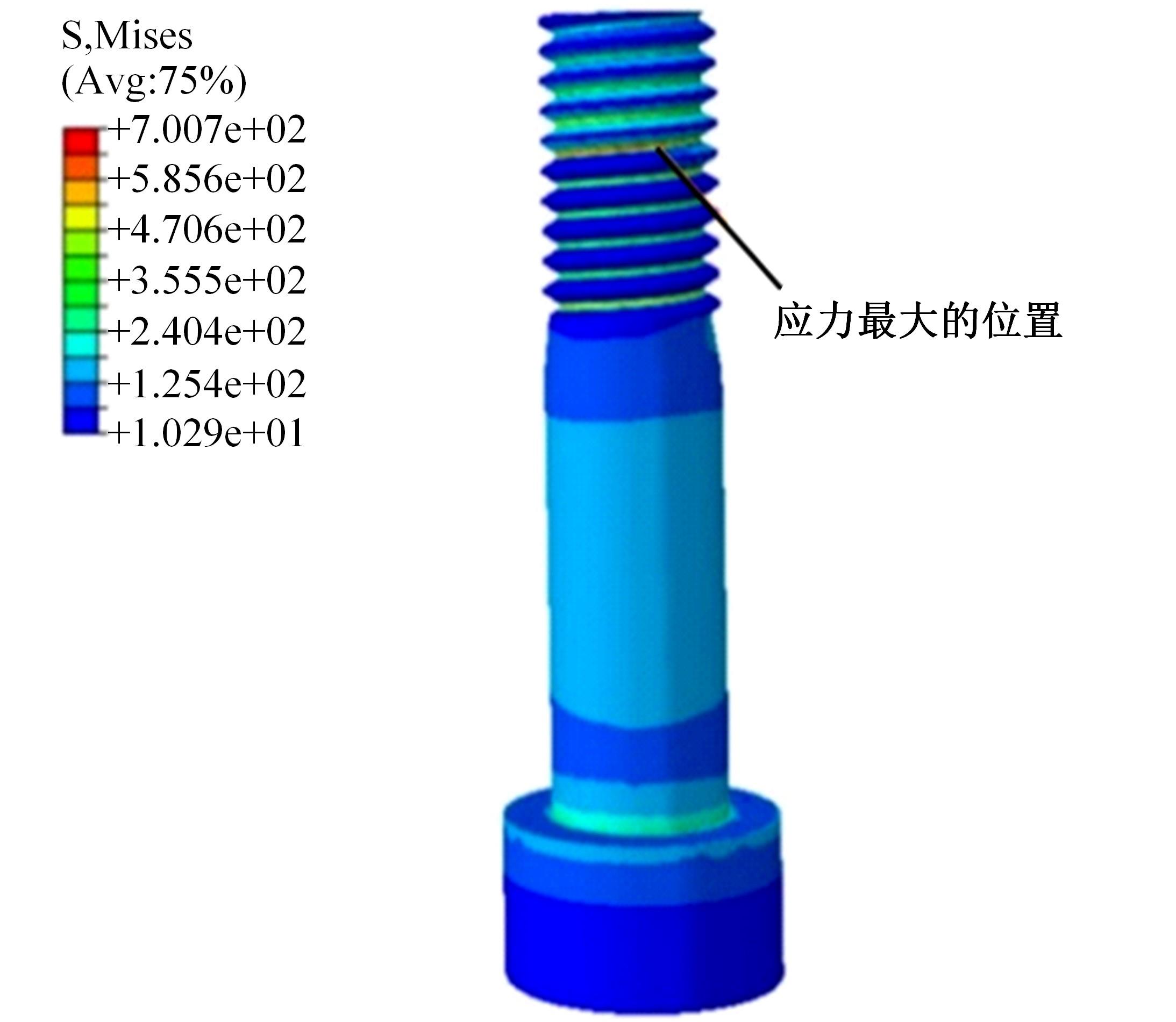
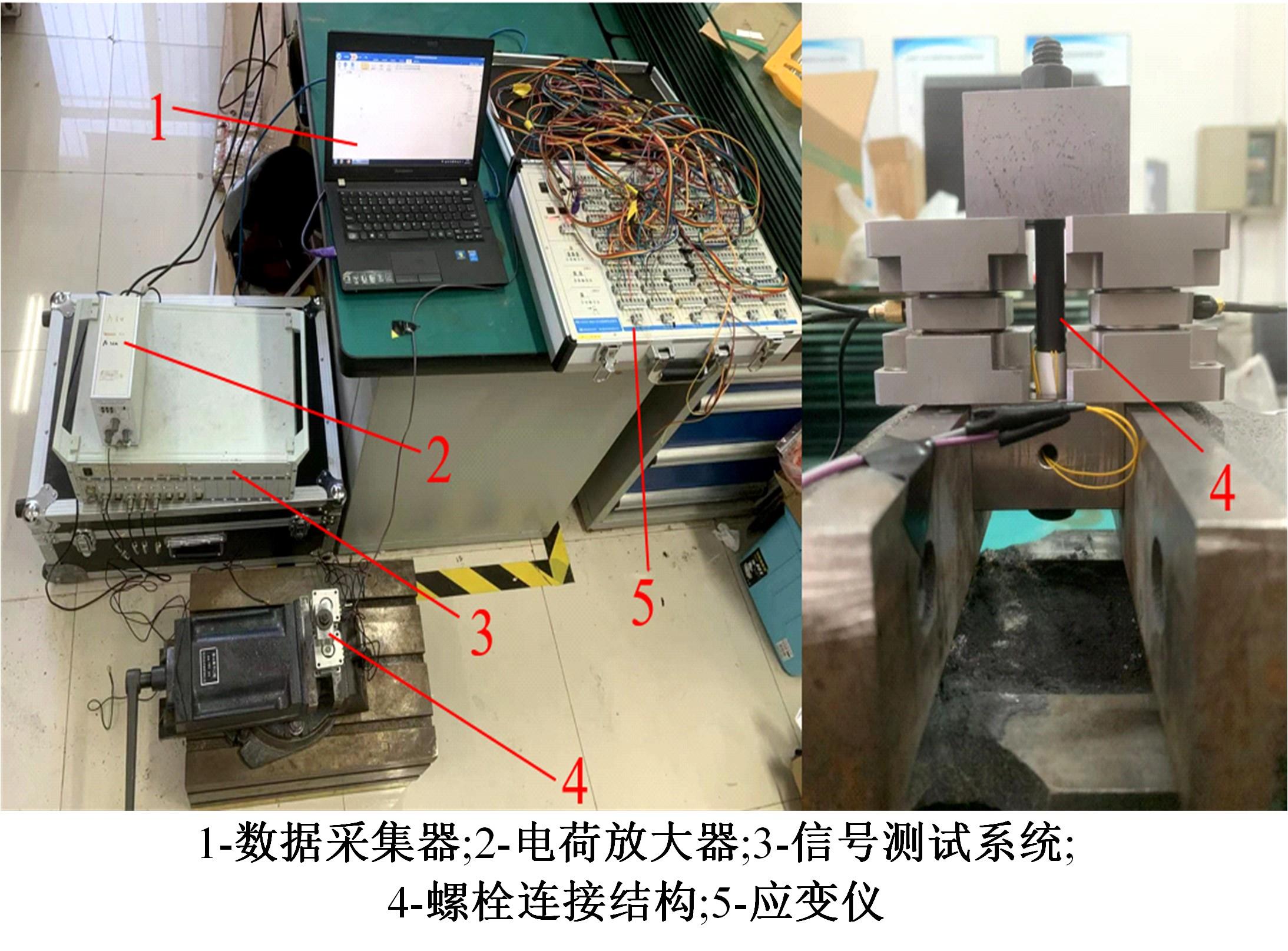
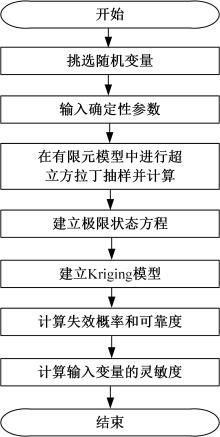
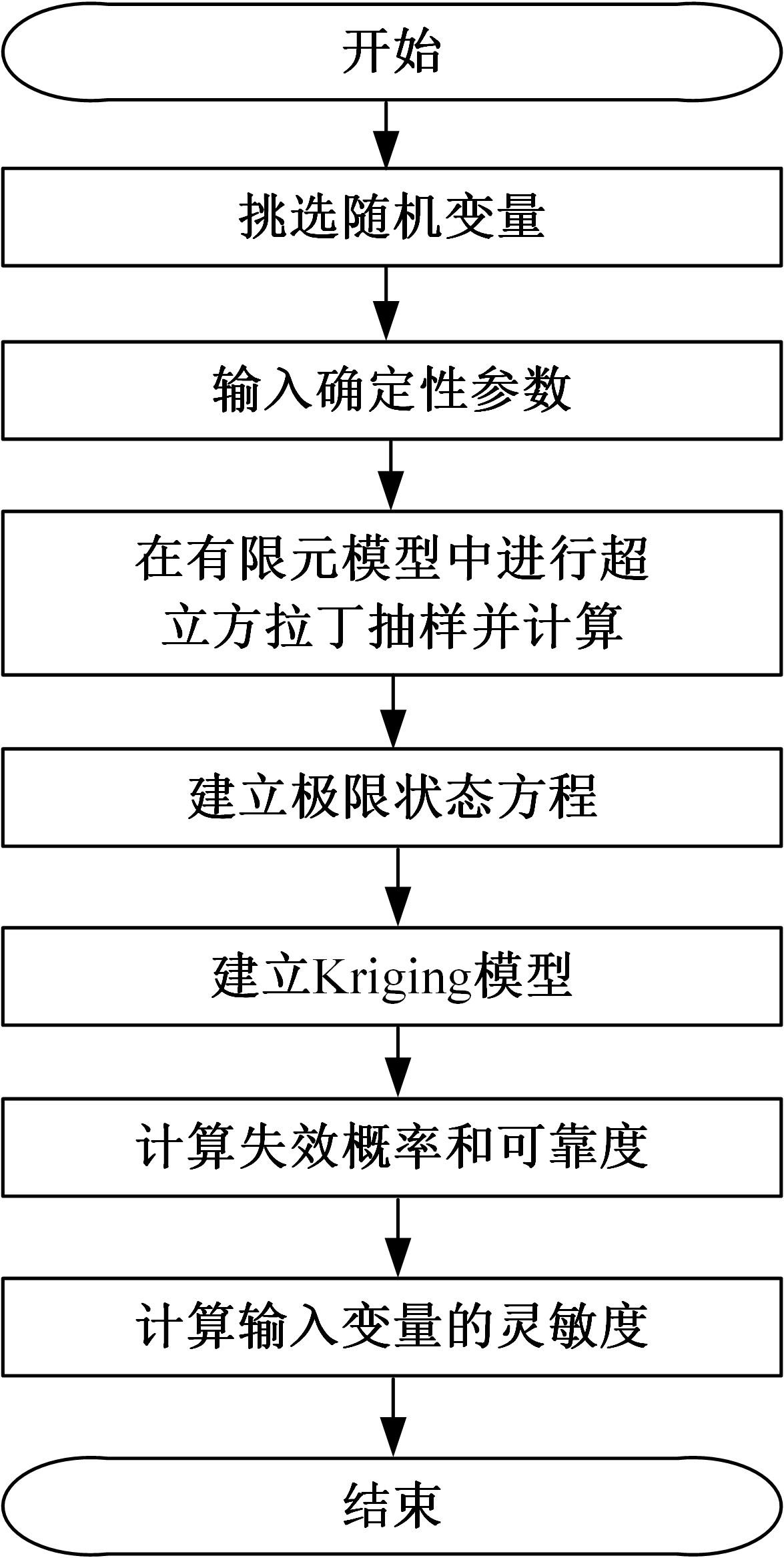
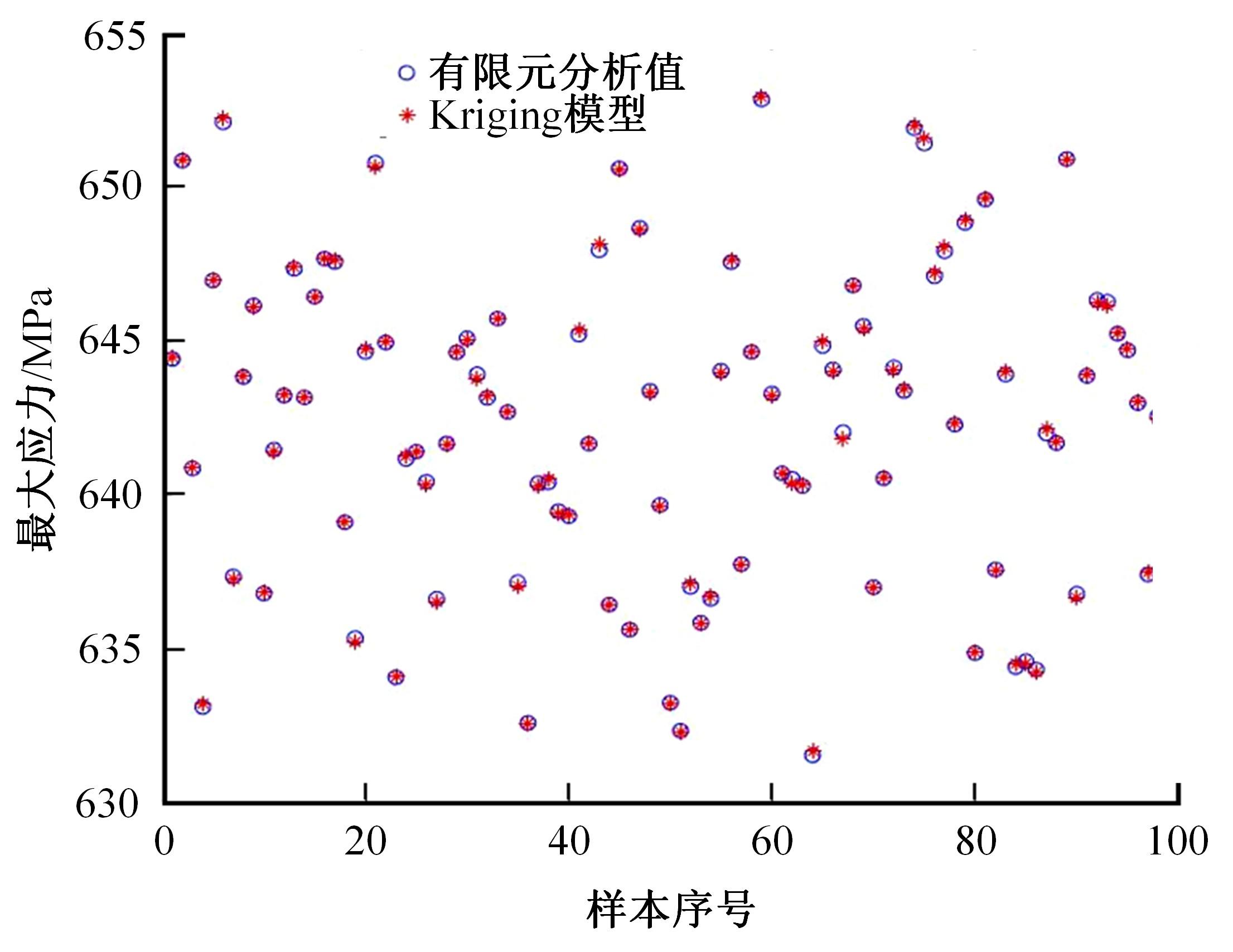
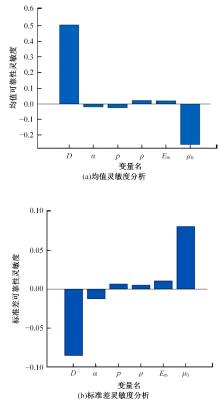
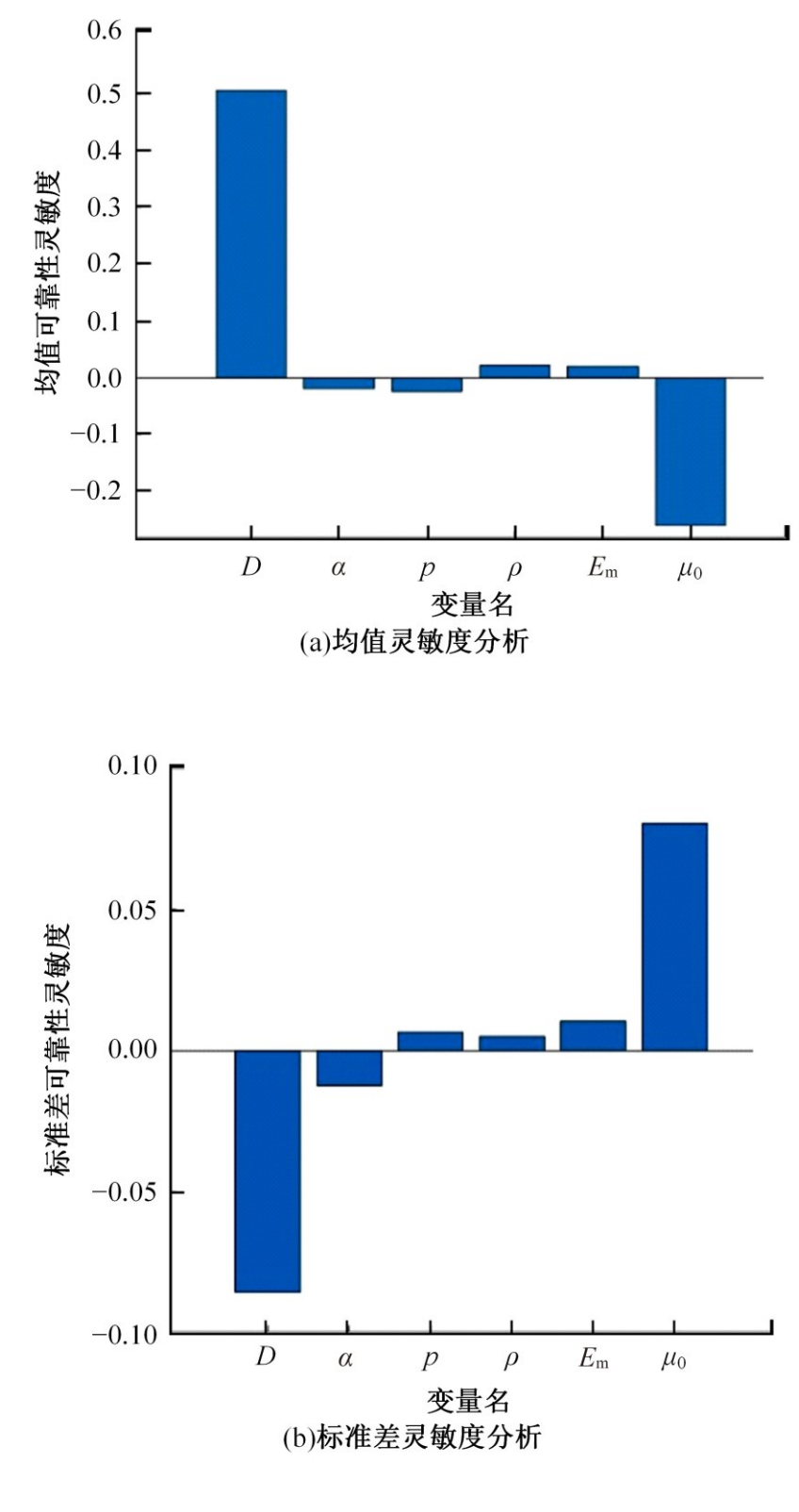
Journal of Jilin University(Engineering and Technology Edition) ›› 2023, Vol. 53 ›› Issue (8): 2219-2226.doi: 10.13229/j.cnki.jdxbgxb.20211164

Previous Articles    

Reliability sensitivity analysis of bolt pre-tightening connection

Xian-zhen HUANG1,2(),Kai-bo SUN1,Xiao-gang LUAN1,Bing HU1   

  1. 1.School of Mechanical Engineering and Automation，Northeastern University，Shenyang 110819，China
    2.Key Laboratory of Vibration and Control of Aero-Propulsion Systems Ministry of Education of China，Northeastern University，Shenyang 110819，China
  • Received:2021-11-08 Online:2023-08-01 Published:2023-08-21

RICH HTML

 8   

PDF (PC)

611

Abstract:

In bolt connection， excessive pre-tightening force will make bolts fail in case of accidental overload. Traditional method of bolt pre-tightening analysis considers that the parameters are determined， but the parameters are random in actual working conditions， which causes larger analysis errors. To solve this problem， considering the influence of random factors， a finite element analysis model of bolt pre-tightening failure behavior is proposed in this paper， the reliability analysis is carried out by judging whether the stress value at the thread of bolt exceeds the allowable stress value， and the reliability sensitivity analysis is calculated to evaluate the influence of various parameters on the failure phenomenon in bolt connection. The results show that the change of bolt diameter has the greatest influence on the phenomenon of bolt static strength failure， and the Poisson's ratio of material has the second influence on it. The reliability of bolt connection will increase with the increase of bolt tooth angle， screw pitch and Poisson's ratio of material， and decrease with the increase of bolt diameter， material density and elastic modulus.

Key words: machanical design, bolt connection, statics, reliability, sensitivity

CLC Number: 

  • TH131.3

Fig.1

Mesh generation of hexahedron connected by bolts"

Fig.2

Schematic diagram of bolt connection Pic title"

Table 1

Structure and material parameters of bolts and nuts"

参数螺栓螺母
公称直径/mm1010
牙型角/（°）6060
螺距/mm1.51.5
弹性模量/GPa210210
泊松比0.30.3
密度/（kg·m-2）78507850

Fig.3

Static analysis of bolt pre-tightening"

Fig.4

Experimental device"

Table 2

Experimental and simulated strain values"

夹紧力/N仿真值组别平均值
1234
503.182.873.353.833.233.32
1006.346.235.276.716.486.17
1509.5210.549.5810.069.8910.02
20012.5913.4111.5012.4512.9312.57
25015.8414.8515.3316.2916.7715.81
30018.9919.6418.2018.6817.7218.56
35022.1422.9923.4720.1222.0422.16
40025.2424.9126.3524.4323.9524.91
45028.5529.2228.7426.8325.3927.55
50031.5230.1831.1432.5734.4932.10

Table 3

Experimental and simulated stress values"

夹紧力/N有限元应力值/MPa各项夹紧力下对应的实验应力值/MPa
500.6340.664
1001.2551.234
1501.9042.004
2002.5232.514
2503.1713.162
3003.7973.712
3504.4194.432
4005.0664.982
4505.5935.510
5006.3526.420

Fig.5

Kriging reliability analysis flow chart"

Table 4

Table of bearing parameters random variables"

螺栓参数数值分布变异系数
螺栓大径D/mm10正态分布0.002
螺栓的牙型角α/（°）60正态分布0.005
螺栓的螺距p/mm1.5正态分布0.005
弹性模量Em/GPa210正态分布0.05
密度ρ/（kg·m-3）7850正态分布0.05
泊松比μ00.3正态分布0.05

Fig.6

Comparison between function values and finite element calculation values"

Fig.7

Sensitivity analysis"

1 Lin Q, Zhao Y, Sun Q, et al. Reliability evaluation method of anti-loosening performance of bolted joints[J]. Mechanical Systems and Signal Processing, 2022, 162(5): 108067.
2 Bozca M, Knt U. Reliability analysis of the mechanical properties of 30MnB4 high strength steel bolts under static loading conditions[J]. Ecological Restoration, 2020, 38(2): 495-512.
3 Öztekin E. Investigation of reliabilities of bolt distances for bolted structural steel connections by Monte Carlo simulation method[J]. Pamukkale University Journal of Engineering Sciences, 2015, 21(6): 213-223.
4 山本晃. 螺纹联接的理论与计算[M]. 1版. 上海: 上海科学技术文献出版社, 1984.
5 Sopwith D G. The distribution of load in screw threads[J]. Proceedings of the Institution of Mechanical Engineers, 2006, 159: 373-383.
6 Dragoni E. Effect of thread pitch and frictional coefficient on the stress concentration in metric nut-bolt connections[J]. Journal of Offshore Mechanics & Arctic Engineering, 1994, 116(1):21-27.
7 Zhao H. Stress concentration factors within bolt-nut connectors under elasto-plastic deformation[J]. International Journal of Fatigue, 1998, 20(9): 651-659.
8 Yokoyama T, Olsson M, Izumi S, et al. Investigation into the self-loosening behavior of bolted joint subjected to rotational loading[J]. Engineering Failure Analysis, 2012, 23(2): 35-43.
9 Dinger G, Friedrich C. Avoiding self-loosening failure of bolted joints with numerical assessment of local contact state[J]. Engineering Failure Analysis, 2011, 18(8): 2188-2200.
10 Fukuoka T, Nomura M. Proposition of helical thread modeling with accurate geometry and finite element analysis[J]. Journal of Pressure Vessel Technology, 2008, 130(1): 135-140.
11 胡大勇. 参数化三维螺纹实体建模技术及仿真研究[D]. 大连: 大连交通大学机械工程学院, 2005.
Hu Da-yong. Parametric 3D thread solid modeling technology and simulation research[D]. Dalian: School of Mechanical Engineering, Dalian Jiaotong University, 2005.
12 侯世远. 螺纹联接松动机理研究[D]. 北京: 北京理工大学机械与车辆学院, 2015.
Hou Shi-yuan. Research on the mechanism of thread connection looseness[D]. Beijing: School of Machinery and Vehicle, Beijing University of Technology, 2015.
13 陆秉权, 王海龙, 周小飞, 等. 应用有限元技术计算螺栓联接的方法研究[J]. 黑龙江电力, 2004, 26(2): 100-102.
Lu Bing-quan, Wang Hai-long, Zhou Xiao-fei, et al. Research on the method of calculating bolted connections using finite element technology[J]. Heilongjiang Electric Power, 2004, 26 (2): 100-102.
14 李军, 杨洁明, 高俊云. 大型风力机组塔架螺栓连接应力分析[J]. 钢结构, 2011, 26(7): 22-25.
Li Jun, Yang Jie-ming, Gao Jun-yun. Stress analysis of bolt connections in large wind turbine tower frames[J]. Steel Structures, 2011, 26(7): 22-25.
15 邱继伟, 罗海胜. 基于混合不确定性的螺旋锥齿轮结构可靠性分析[J]. 吉林大学学报: 工学版, 2022, 52(2): 466-473.
Qiu Ji-wei, Luo Hai-sheng. Reliability analysis of spiral bevel gear structure based on mixed uncertainty [J]. Journal of Jilin University (Engineering and Techndogy Edition), 2022, 52(2): 466-473.
16 Jamia N, Jalali H, Taghipour J, et al. An equivalent model of a nonlinear bolted flange joint[J]. Mechanical Systems and Signal Processing, 2021, 153(25/26): 107507.
17 Izumi S, Yokoyama T, Kimura M, et al. Loosening-resistance evaluation of double-nut tightening method and spring washer by three-dimensional finite element analysis[J]. Engineering Failure Analysis, 2009, 16(5): 1510-1519.
18 Zou Q, Sun T S, Nassar S A, et al. Effect of lubrication on friction and torque-tension relationship in threaded fasteners[J]. Tribology Transactions, 2007, 50(1): 127-136.
19 李成, 朱红红, 铁瑛,等. 单搭胶/螺栓混合连接结构的应力分布与载荷分配[J]. 吉林大学学报: 工学版, 2013, 43(4): 933-938.
Li Cheng, Zhu Hong-hong, Ying Tie, et al. Stress distribution and load distribution of single adhesive/bolt hybrid connection structure[J]. Journal of Jilin University(Engineering and Technology Edition), 2013, 43(4): 933-938.
20 Liu J H, Ouyang H J, Peng J F, et al. Experimental and numerical studies of bolted joints subjected to axial excitation[J]. Wear, 2016, 346(1): 66-77.
21 Yang X, Nassar S A, Wu Z, et al. Nonlinear Behavior of preloaded bolted joints under a cyclic separating load[J]. Journal of Pressure Vessel Technology, 2012, 134(1): 011206.
22 闫明, 张义民, 孙志礼, 等. 机械零件相关失效可靠度及灵敏度计算的Monte Carlo方法[J]. 东北大学学报: 自然科学版, 2011, 32(6): 834-837.
Yan Ming, Zhang Yi-min, Sun Zhi-li, et al. The Monte Carlo method for calculating the reliability and sensitivity of mechanical component related failures[J]. Journal of Northeast University(Natural Science Edition), 2011, 32(6): 834-837.
23 Jiang C, Qiu H, Gao L, et al. Real-time estimation error-guided active learning Kriging method for time-dependent reliability analysis[J]. Applied Mathematical Modelling, 2020, 77(1): 82-98.
[1] Zhi-qiang HAN,Gang XIE,Yong-jun ZHOU,Shi-zhong LIU,Min-jie JIN. Numerical analysis method of vehicle⁃bridge coupling vibration of curved bridge [J]. Journal of Jilin University(Engineering and Technology Edition), 2023, 53(2): 515-522.
[2] Xu CHEN,Chao-fei CAO,Jing SHANG,Ming-xing HUANG,Chang-fa AI,Dong-ya Ren. Evaluation of influence of gradation segregation on pavement moisture damage under action of dynamic and static water environment [J]. Journal of Jilin University(Engineering and Technology Edition), 2023, 53(1): 210-219.
[3] Zi-rong YANG,Yan LI,Xue-feng JI,Fang LIU,Dong HAO. Sensitivity analysis of operating parameters for proton exchange membrane fuel cells [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(9): 1971-1981.
[4] Xin-tian LIU,Mu-zhou MA,Jia-long HE. Reliability prediction method of machining precision of machine tool parts based on fuzzy coupling [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 377-383.
[5] Hong-xun ZHAO,Zhao-jun YANG,Chuan-hai CHEN,Hai-long TIAN,Li-ping WANG. Optimal design of acceleration test of motorized spindle of numerical control Machine Tool considering parameter weight [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 409-416.
[6] Chuan-hai CHEN,Cheng-gong WANG,Zhao-jun YANG,Zhi-feng LIU,Hai-long TIAN. Research status and development trend analysis of reliability modeling of CNC machine tools [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 253-266.
[7] Lin SONG,Li-ping WANG,Jun WU,Li-wen GUAN,Zhi-gui LIU. Reliability analysis based on cyber⁃physical system and digital twin [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 439-449.
[8] Ji-wei QIU,Hai-sheng LUO. Structure reliability analysis of spiral bevel gear based on hybrid uncertainties [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 466-473.
[9] Li-ping WANG,Bin ZHU,Jun WU,Zi-han TAO. Fault analysis of circular tool magazine based on Bayesian network [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 280-287.
[10] Zi-ling ZHANG,Xiong HU,Yin QI,Wei WANG,Zhi-qiang TAO,Zhi-feng LIU. An approach for error allocation of machine tool based on vector projection response surface method [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 384-391.
[11] Lu LIU,Hua-xi ZHOU,Chuan-hai CHEN,Meng-hui GONG,Hu-tian FENG,Chang-guang ZHOU. Reliability design model of ball screws based on competition failure [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(2): 458-465.
[12] Xue-ping FAN,Guang-hong YANG,Zhi-peng SHANG,Xiao-xiong ZHAO,Qing-kai XIAO,Yue-fei LIU. Dynamic reliability fusion prediction of long-span bridge girder considering structural serviceability [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(1): 144-153.
[13] Yu-xuan WEI,Ming ZHANG,Jia LIU,Shuo LIU,Ming-yu LU,Hong-yu WANG. Buckling performance of variable stiffness composite cylindrical shells based on mode imperfections [J]. Journal of Jilin University(Engineering and Technology Edition), 2022, 52(1): 91-100.
[14] Zun-ling DU,Yi-min ZHANG. Reliability analysis of piston pump under random flow rate [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(6): 1967-1974.
[15] Guo-fa LI,Ze-quan CHEN,Jia-long HE. New adaptive sampling strategy for structural reliability analysis [J]. Journal of Jilin University(Engineering and Technology Edition), 2021, 51(6): 1975-1981.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
Copyright © Journal of Jilin University(Engineering and Technology Edition), All Rights Reserved.
Tel: +86-431-85095297　Fax: +86-431-85094074　E-mail: xbgxb@jlu.edu.cn
Powered by Beijing Magtech Co. Ltd