吉林大学学报(工学版) ›› 2021, Vol. 51 ›› Issue (4): 1222-1229.doi: 10.13229/j.cnki.jdxbgxb20200321

• 车辆工程·机械工程 • 上一篇    

随机振动环境下液压直管道设计方法

李卫1,2(),张怀亮1,2(),瞿维1,2   

  1. 1.中南大学 高性能复杂制造国家重点实验室，长沙 410083
    2.中南大学 机电工程学院，长沙 410083
  • 收稿日期:2020-04-19 出版日期:2021-07-01 发布日期:2021-07-14
  • 通讯作者: 张怀亮 E-mail:liwei16121@163.com;zhl2001@csu.edu.cn
  • 作者简介:李卫（1993-），男，博士研究生.研究方向：液压系统动力学.E-mail：liwei16121@163.com
  • 基金资助:
    国家重点基础研究发展计划项目(2013CB035400);湖南省自然科学基金项目(2020JJ4736);中南大学中央高校基本科研业务费专项资金项目(2021zzts0142)

Design method of hydraulic straight pipe under random vibration

Wei LI1,2(),Huai-liang ZHANG1,2(),Wei QU1,2   

  1. 1.State Key Laboratory of High Performance and Complex Manufacturing，Central South University，Changsha 410083，China
    2.College of Mechanical and Electrical Engineering，Central South University，Changsha 410083，China
  • Received:2020-04-19 Online:2021-07-01 Published:2021-07-14
  • Contact: Huai-liang ZHANG E-mail:liwei16121@163.com;zhl2001@csu.edu.cn

RICH HTML

 4   

PDF (PC)

86

摘要：

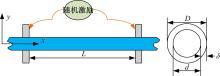
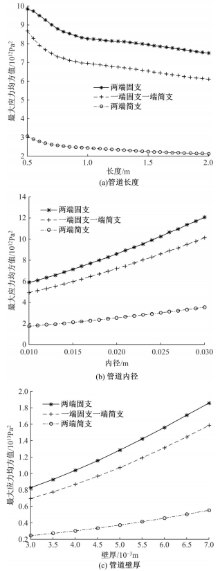
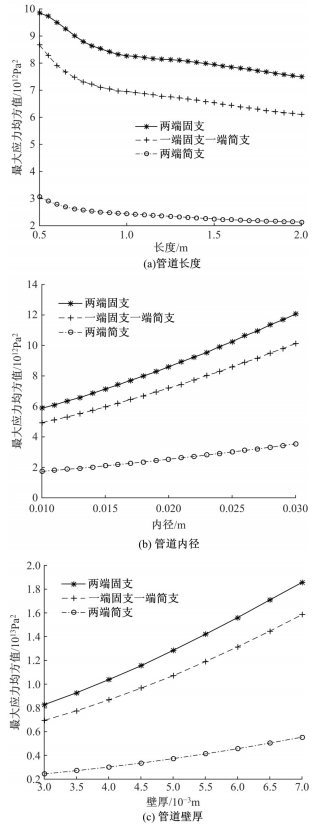
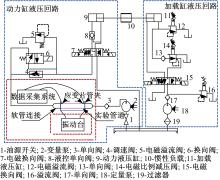
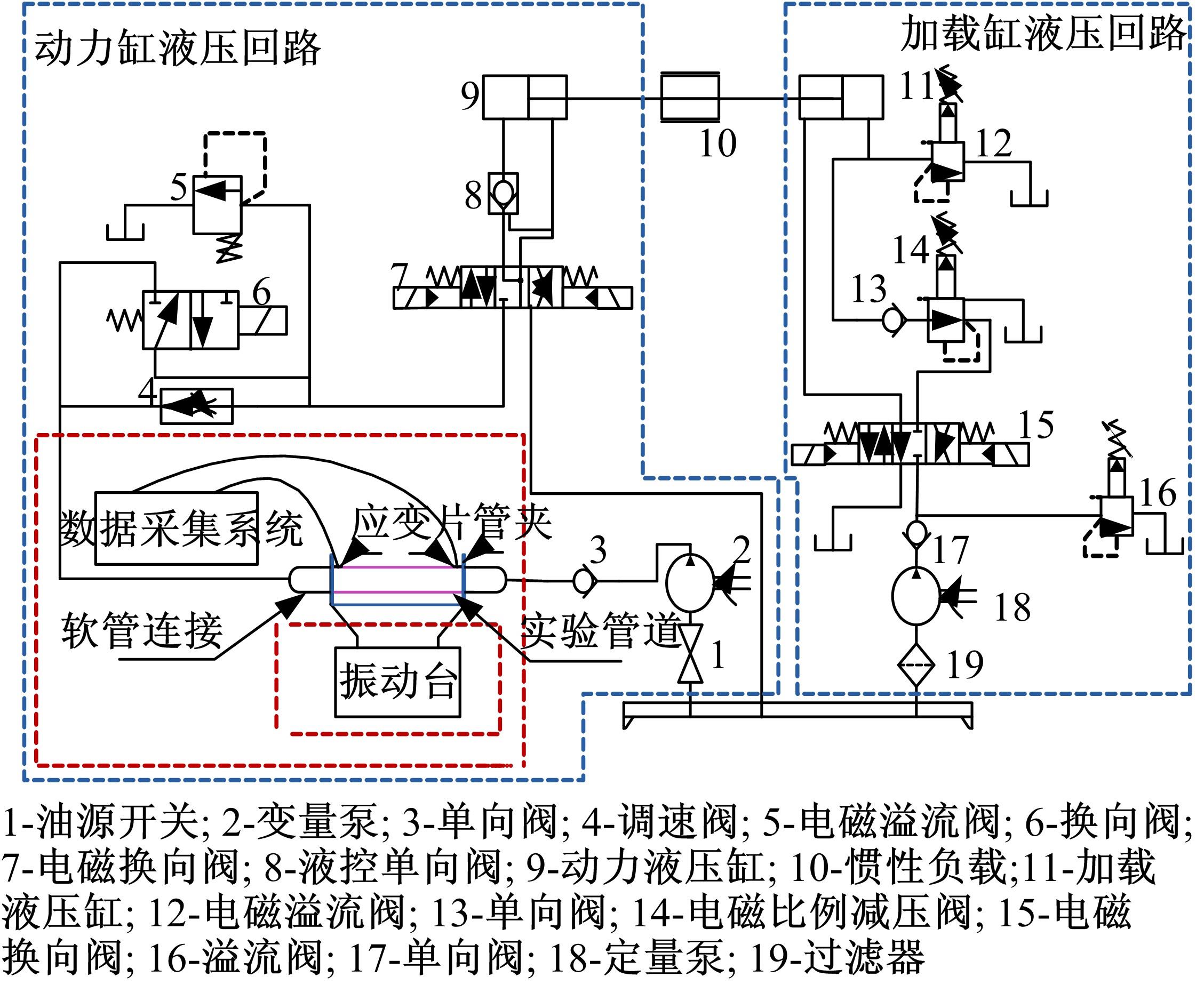
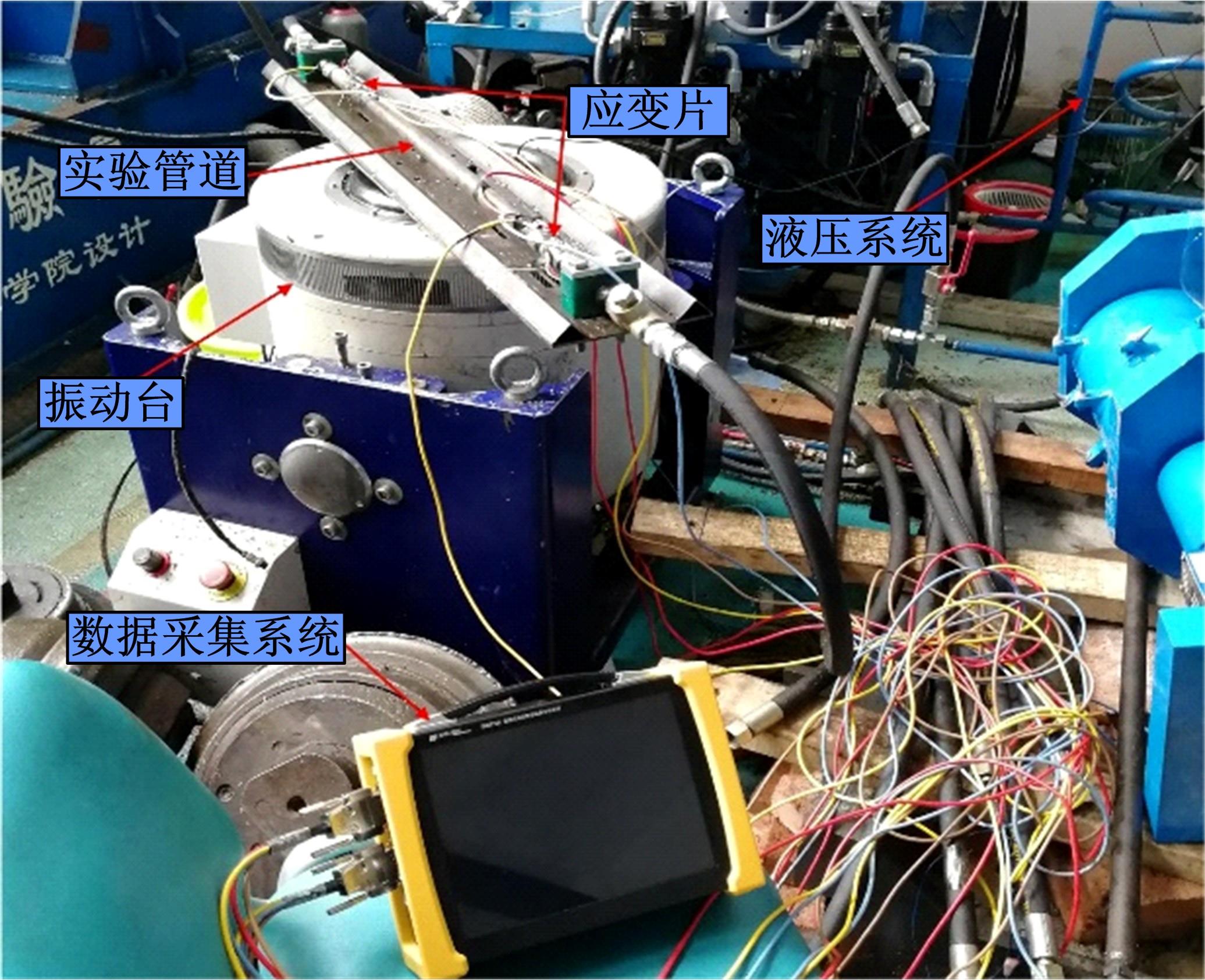
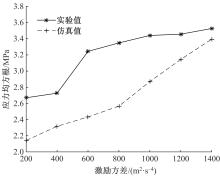
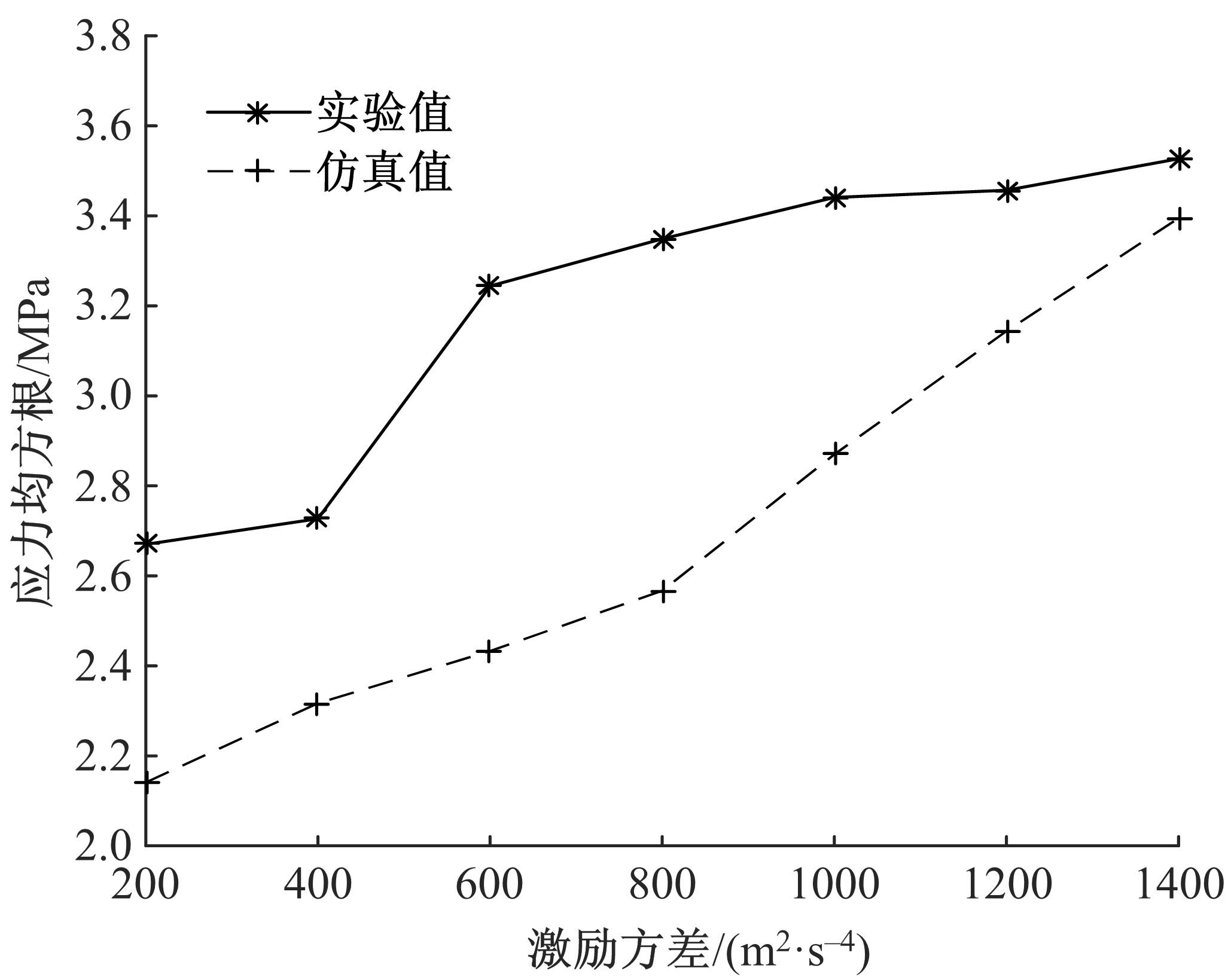
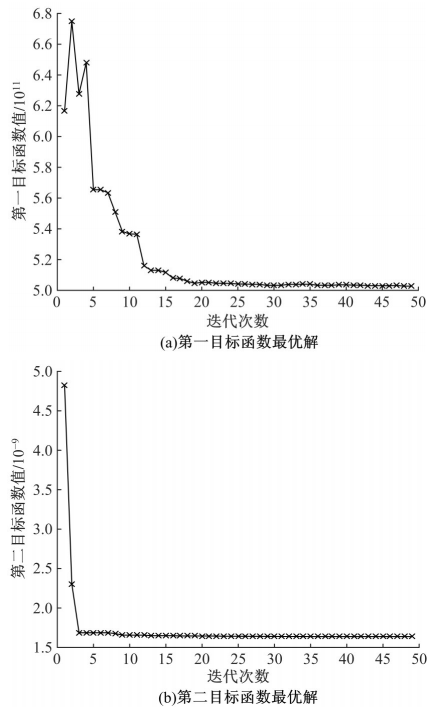
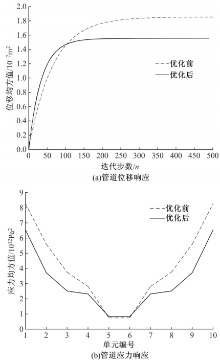
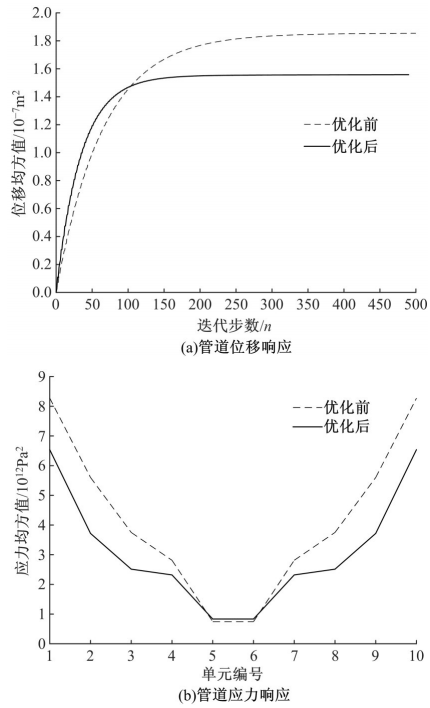
为了减小硬岩掘进机（TBM）工作过程中产生的随机振动对其液压系统中的液压直管道位移和应力的影响，建立了随机振动环境下液压直管道设计模型，并采用实验验证了模型的正确性。分析了管道结构参数对液压直管道应力响应和位移响应的影响规律，发现液压直管道应力响应和位移响应随管道内径和管道长度的变化趋势是相反的，而随着管道壁厚的增大，管道最大位移响应和最大应力响应均增大。采用基于遗传算法的多目标优化算法对随机振动环境下两端固支液压直管道的结构参数进行优化设计，对比分析了设计前后管道的应力响应和位移响应，结果表明，设计后的管道最大位移均方值降低了约16.21%，最大应力均方值降低了21.04%。研究结果可为随机振动环境中液压管道的抗振设计与选型提供理论依据。

关键词: 液压直管道, 流固耦合, 随机振动, 优化设计

Abstract:

For reducing the impact of random vibration generated by Tunnel Boring Machine (TBM) on the displacement and stress of hydraulic straight pipe in its hydraulic system, a design model of hydraulic straight pipe under random vibration environment was established, and the correctness of the model was verified by experiments. The influences of pipeline structural parameters on the stress response and displacement response of hydraulic straight pipeline were analyzed. It is found that the stress response and displacement response of hydraulic straight pipe change with pipe diameter and pipe length in the opposite direction. The multi-objective optimization algorithm based on genetic algorithm was adopted to optimize the structural parameters of the fixed-supported hydraulic straight pipeline at both ends under the random vibration environment. The stress response and displacement response of the pipeline before and after the design were compared and analyzed. The results show that the mean square value of the maximum displacement of the pipeline after the design was reduced by about 16.21% and the mean square value of the maximum stress was reduced by 21.04%. The research results could provide a theoretical basis for the design and selection of vibration resistance of hydraulic pipeline in random vibration environment.

Key words: hydraulic straight pipe, fluid-solid interaction, random vibration, optimization design

中图分类号: 

  • TH137.5

图1

随机振动下液压直管道物理模型"

表1

管道分析主要参数"

参数名称数值参数名称数值
管道内径d/m0.019泊松比μ0.3
管壁厚度δ/m0.003管道外径D/m0.025

流体平均流速v

/（m·s-1）

5流体平均压力p/Pa2×107
管道支撑间距L/m1

管道密度ρp

/（kg·m-3）

7850

管道弹性模量

E/Pa

2.01×1011

流体密度ρf

/（kg·m-3）3

890

图2

管道参数对管道最大位移响应的影响"

图3

管道参数对管道最大应力响应的影响"

图4

实验系统液压原理图"

图5

实验系统实物图"

图6

应力响应实验值与仿真值对比"

图7

最优解的变化"

图8

优化前、后管道响应对比"

1 刘森,张怀亮,彭欢. 基础振动诱发的流体-管道轴向耦合振动特性[J]. 北京航空航天大学学报, 2016,42(3):610-618.
Liu Sen, Zhang Huai-liang, Peng Huan. Fluid-pipeline coupling vibration characteristics induced by foundation vibration[J]. Journal of Beijing University of Aeronautics and Astronautics, 2016, 42(3):610-618.
2 瞿维,张怀亮,宁海辉,等. TBM液压弯管动态特性分析[J]. 机械工程学报, 2018,54(1):82-89.
Qu Wei, Zhang Huai-liang, Ning Hai-hui, et al. Dynamic characteristics of the hydraulic bend pipe on the TBM[J]. Journal of Mechanical Engineering, 2018,54(1):82-89.
3 Khudayarov B A, Turaev F J. Mathematical simulation of nonlinear oscillations of viscoelastic pipelines conveying fluid[J]. Applied Mathematical Modelling,2019,66:662-679.
4 Dai H L, Abdelkefi A, Wang L. Modeling and nonlinear dynamics of fluid-conveying risers under hybrid excitations[J]. International Journal of Engineering Science, 2014, 81:1-14.
5 Heshmati M, Amini Y, Daneshmand F. Vibration and instability analysis of closed-cell poroelastic pipes conveying fluid[J]. European Journal of Mechanics A—Solids, 2019, 73:356-365.
6 Naji Abhari M, Ghodsian M, Ghodsian M,et al. Experimental and numerical simulation of flow in a 90° bend[J]. Flow Measurement and Instrumentation, 2010,21(3):292-298.
7 Tan X, Mao X Y, Ding H, et al. Vibration around non-trivial equilibrium of a supercritical Timoshenko pipe conveying fluid[J]. Journal of Sound and Vibration, 2018, 428:104-118.
8 Maalawi K Y, Abouel-Fotouh A M, El Bayoumi M, et al. Design of composite pipes conveying fluid for improved stability characteristics[J]. International Journal of Applied Engineering Research, 2016,11(12):7633-7639.
9 Borglund D. On the optimal design of pipes conveying fluid[J]. Journal of Fluids & Structures, 1998, 12(3):353-365.
10 Jung B S, Karney B. Fluid transients and pipeline optimization using GA and PSO: the diameter connection[J]. Urban Water Journal, 2004, 1(2):167-176.
11 Hiramoto Kazuhiko, Doki Hitoshi. Simultaneous optimal design of structural and control systems for cantilevered pipes conveying fluid[J]. Journal of Sound and Vibration, 2004,274(3-5):685-699.
12 Wang Fa-cheng. Effective design of submarine pipe-in-pipe using finite element analysis[J]. Ocean Engineering, 2018, 153:23-32.
13 Qu W, Zhang H, Li W, et al. Influence of support stiffness on dynamic characteristics of the hydraulic pipe subjected to basic vibration[J]. Shock and Vibration, 2018, 2018:1-8.
14 Lin Y H, Tsai Y K. Nonlinear vibrations of Timoshenko pipes conveying fluid[J]. International Journal of Solids & Structures, 1997, 34(23):2945-2956.
15 Zhai H B, Wu Z Y, Liu Y S, et al. Dynamic response of pipeline conveying fluid to random excitation[J]. Nuclear Engineering and Design, 2011,241(8):2744-2749.
16 谭东耀.随机振动离散分析方法[J].力学学报,1992,24(4):473-479.
Tan Dong-yao. Random vibration discrete analysis method[J]. Chinese Journal of Theoretical and Applied Mechanics, 1992,24(4):473-479.
[1] 叶辉,刘畅,闫康康. 纤维增强复合材料在汽车覆盖件中的应用[J]. 吉林大学学报(工学版), 2020, 50(2): 417-425.
[2] 胡兴军,惠政,郭鹏,张扬辉,张靖龙,王靖宇,刘飞. 基于流固耦合的汽车气动特性[J]. 吉林大学学报(工学版), 2019, 49(5): 1414-1419.
[3] 郭昊添,徐涛,梁逍,于征磊,刘欢,马龙. 仿鲨鳃扰流结构的过渡段换热表面优化设计[J]. 吉林大学学报(工学版), 2018, 48(6): 1793-1798.
[4] 王扬, 王晓梅, 陈泽仁, 于建群. 基于离散元法的玉米籽粒建模[J]. 吉林大学学报(工学版), 2018, 48(5): 1537-1547.
[5] 于繁华, 刘仁云, 张义民, 张晓丽, 孙秋成. 机械零部件动态可靠性稳健优化设计的群智能算法[J]. 吉林大学学报(工学版), 2017, 47(6): 1903-1908.
[6] 王靖宇, 于旭涛, 胡兴军, 郭鹏, 辛俐, 郭峰, 张扬辉. 汽车外后视镜流致振特性及其流动机理[J]. 吉林大学学报(工学版), 2017, 47(6): 1669-1676.
[7] 江海, 李敏姣, 顾守东, 张莎莎, 张凯, 焦晓阳, 刘建芳. 谐振管对驻波悬浮特性的理论分析及实验[J]. 吉林大学学报(工学版), 2017, 47(1): 151-156.
[8] 袁哲, 徐东, 刘春宝, 李雪松, 李世超. 基于热流固耦合过程的液力缓速器叶片强度分析[J]. 吉林大学学报(工学版), 2016, 46(5): 1506-1512.
[9] 于繁华, 刘仁云, 张义民, 孙秋成, 张晓丽. 机械结构动态可靠性设计的智能计算方法[J]. 吉林大学学报(工学版), 2016, 46(4): 1269-1275.
[10] 齐龙, 梁仲维, 蒋郁, 马旭, 武涛, 芦玉龙, 赵柳霖. 轻型水田除草机的设计及试验[J]. 吉林大学学报(工学版), 2016, 46(3): 1004-1012.
[11] 杨一洋, 许男, 郭孔辉, 陈平. 轮胎滑水机理在钢带式高速轮胎试验台上的应用[J]. 吉林大学学报(工学版), 2016, 46(1): 1-7.
[12] 陈书明, 彭登志, 王登峰, 梁杰. 车内低频噪声声固耦合及试验优化设计[J]. 吉林大学学报(工学版), 2014, 44(6): 1550-1556.
[13] 武彬, 李骏, 李康, 侯福建, 蒋文虎. 发动机低摩擦优化设计及试验[J]. 吉林大学学报(工学版), 2014, 44(01): 81-85.
[14] 谭越, 马文星, 卢秀泉. 基于流固耦合的冲焊型液力变矩器焊接强度分析[J]. 吉林大学学报(工学版), 2013, 43(04): 928-932.
[15] 焦晓阳, 刘建芳, 刘晓论, 孙旭光. 超声驻波悬浮能力特性[J]. 吉林大学学报(工学版), 2013, 43(02): 340-345.
Viewed
Full text


Abstract

Cited
  Shared   
  Discussed   
No Suggested Reading articles found!
版权所有 © 《吉林大学学报(工学版)》编辑部
地址：长春市人民大街5988号　电话：+86-431-85095297　传真：+86-431-85094074　E-mail: xbgxb@jlu.edu.cn
本系统由北京玛格泰克科技发展有限公司设计开发