考虑各向异性的铝合金型材辊弯断裂预测

doi:10.13229/j.cnki.jdxbgxb.20220084

摘要/Abstract

摘要：

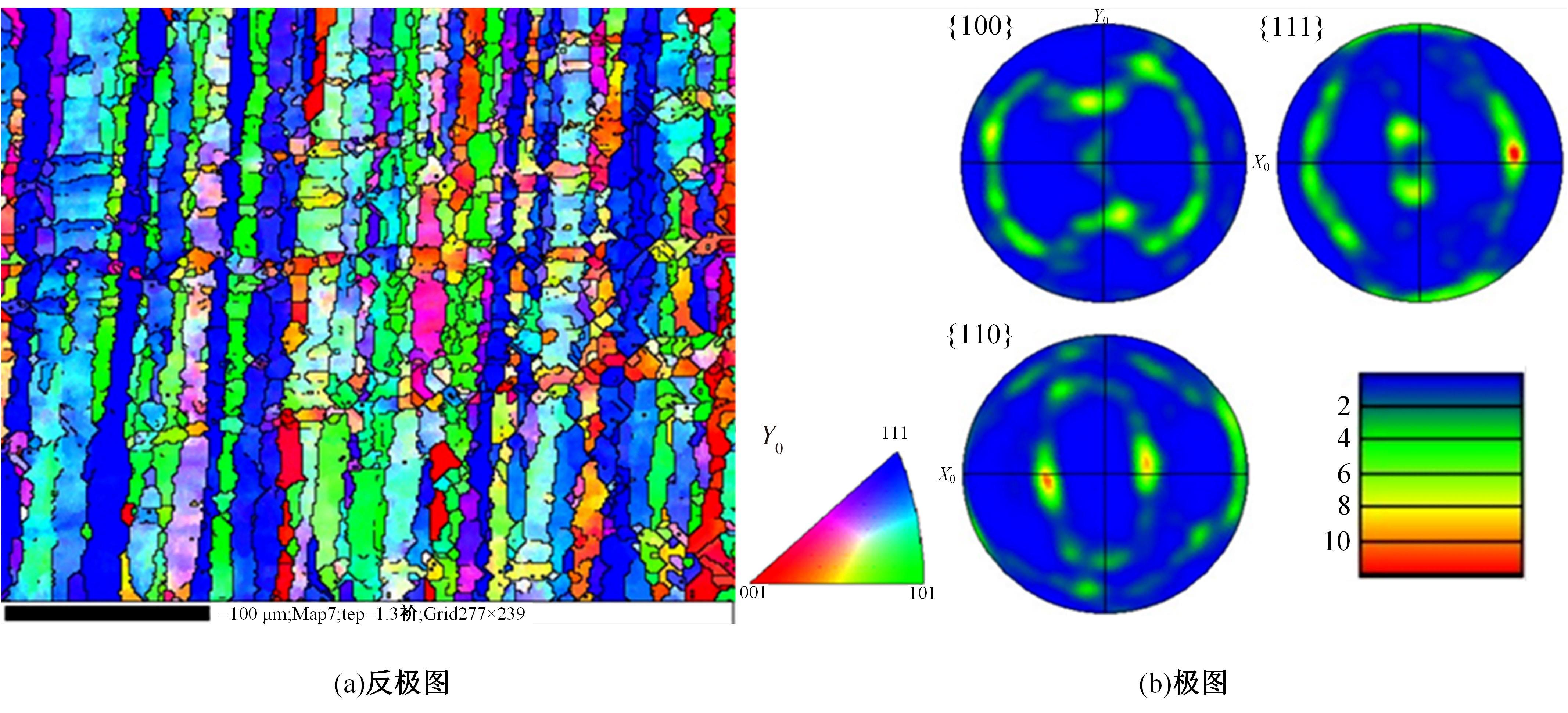
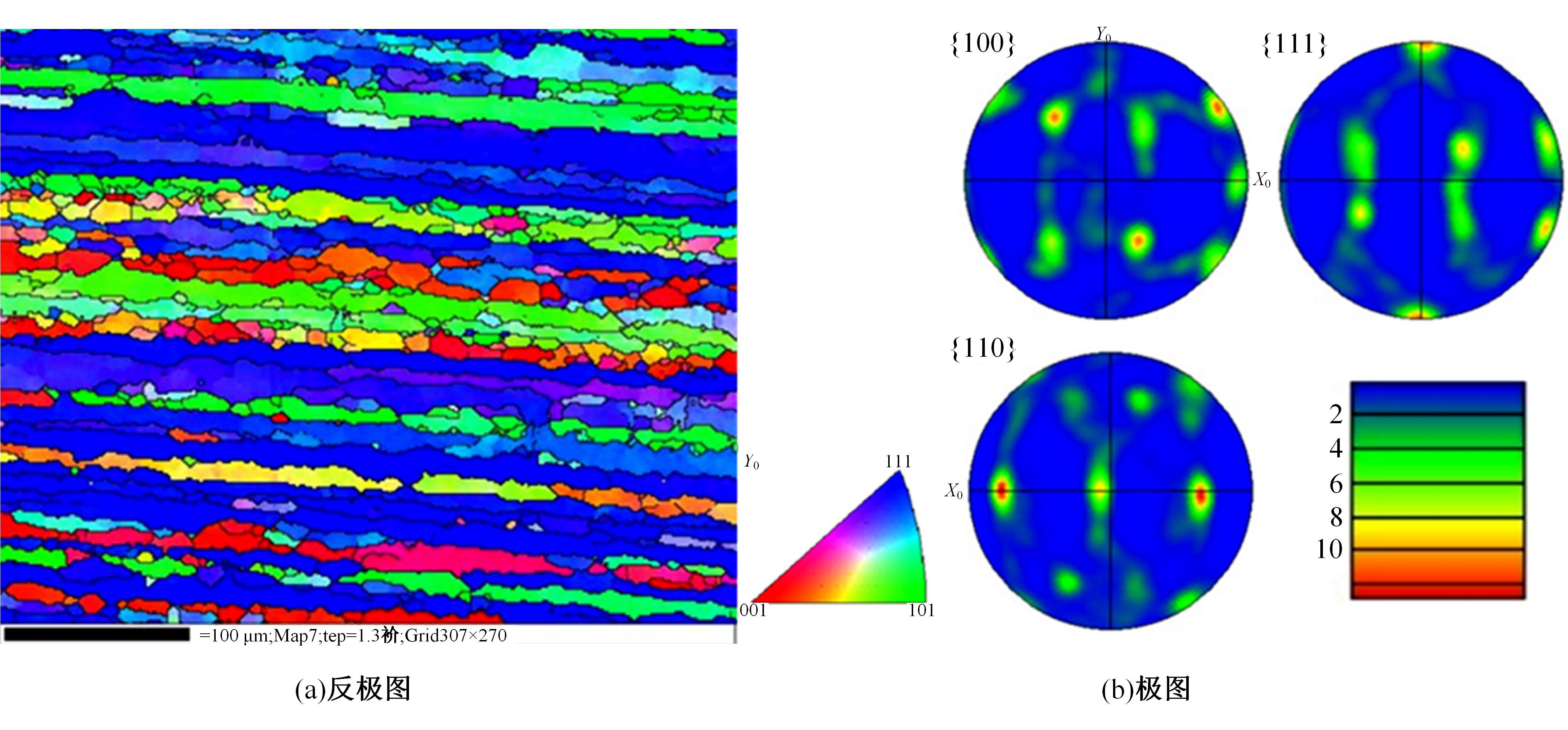
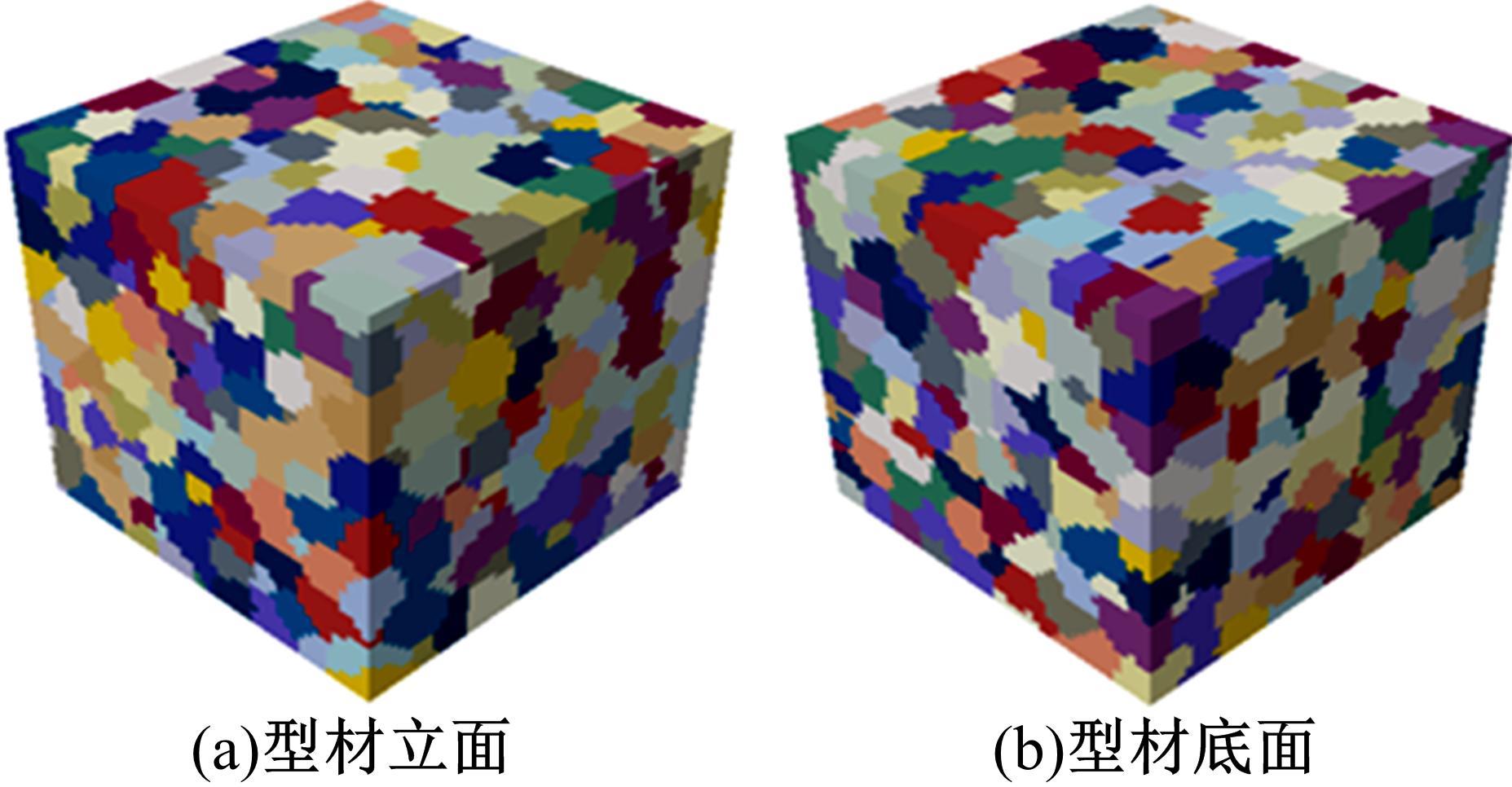
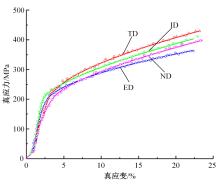
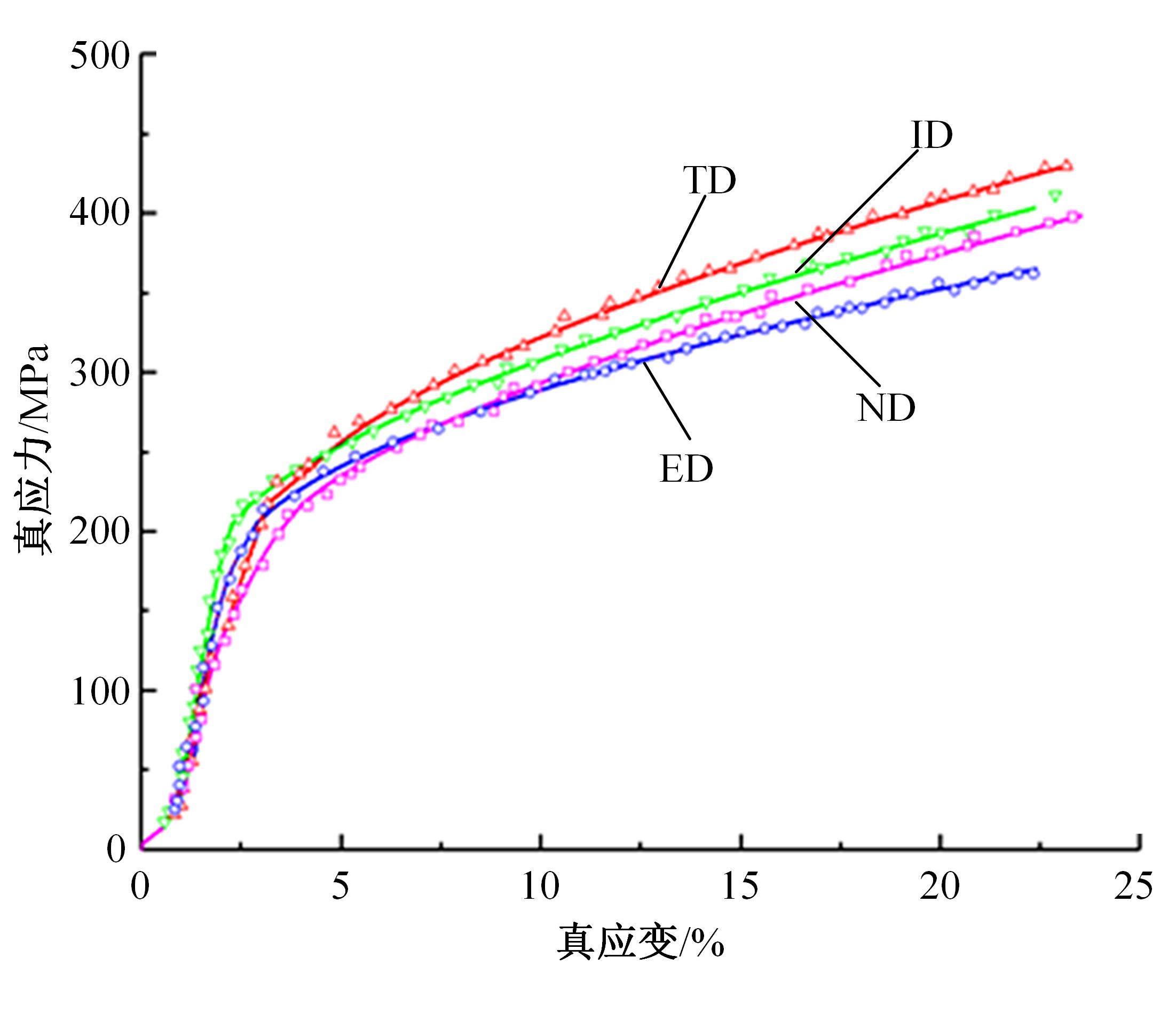
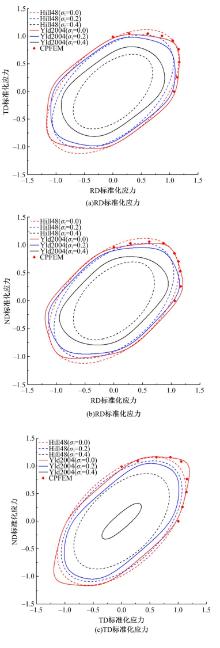
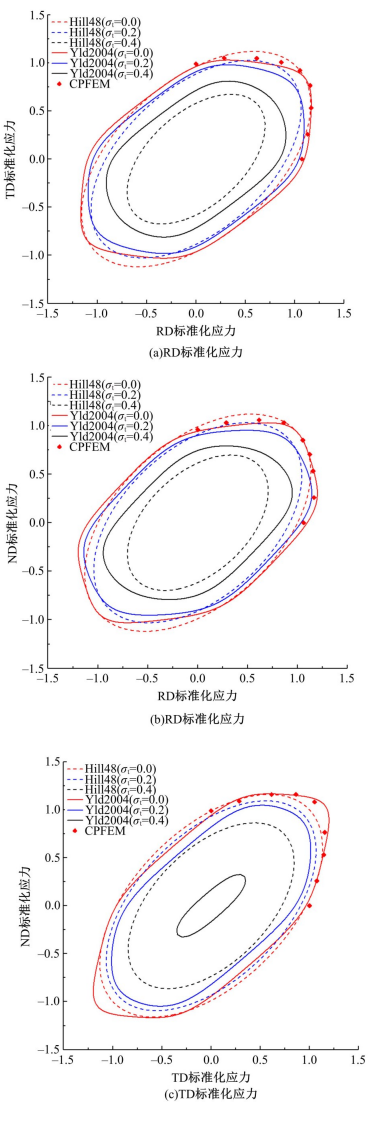
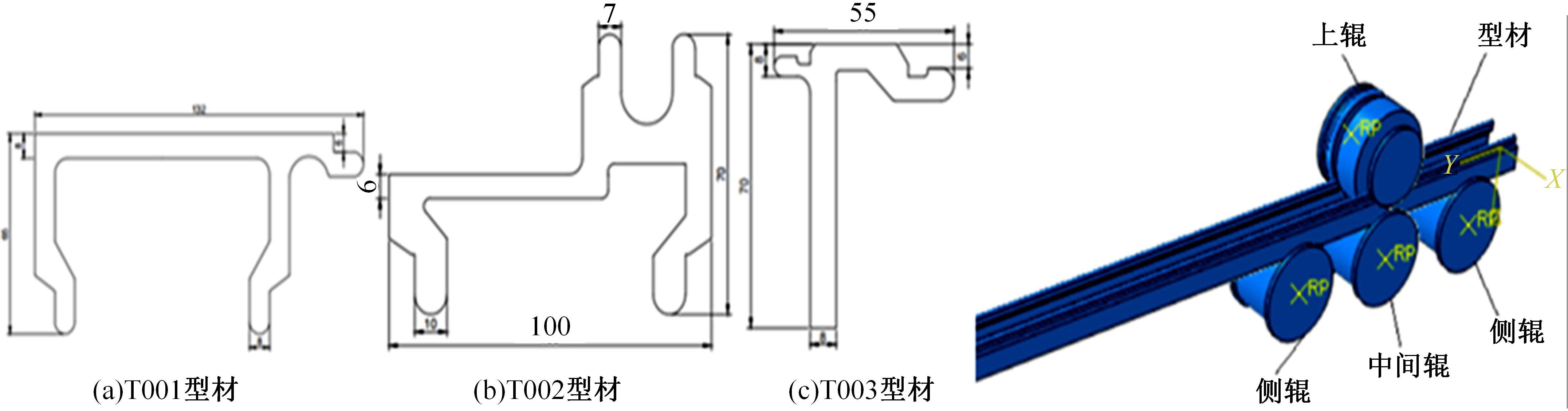
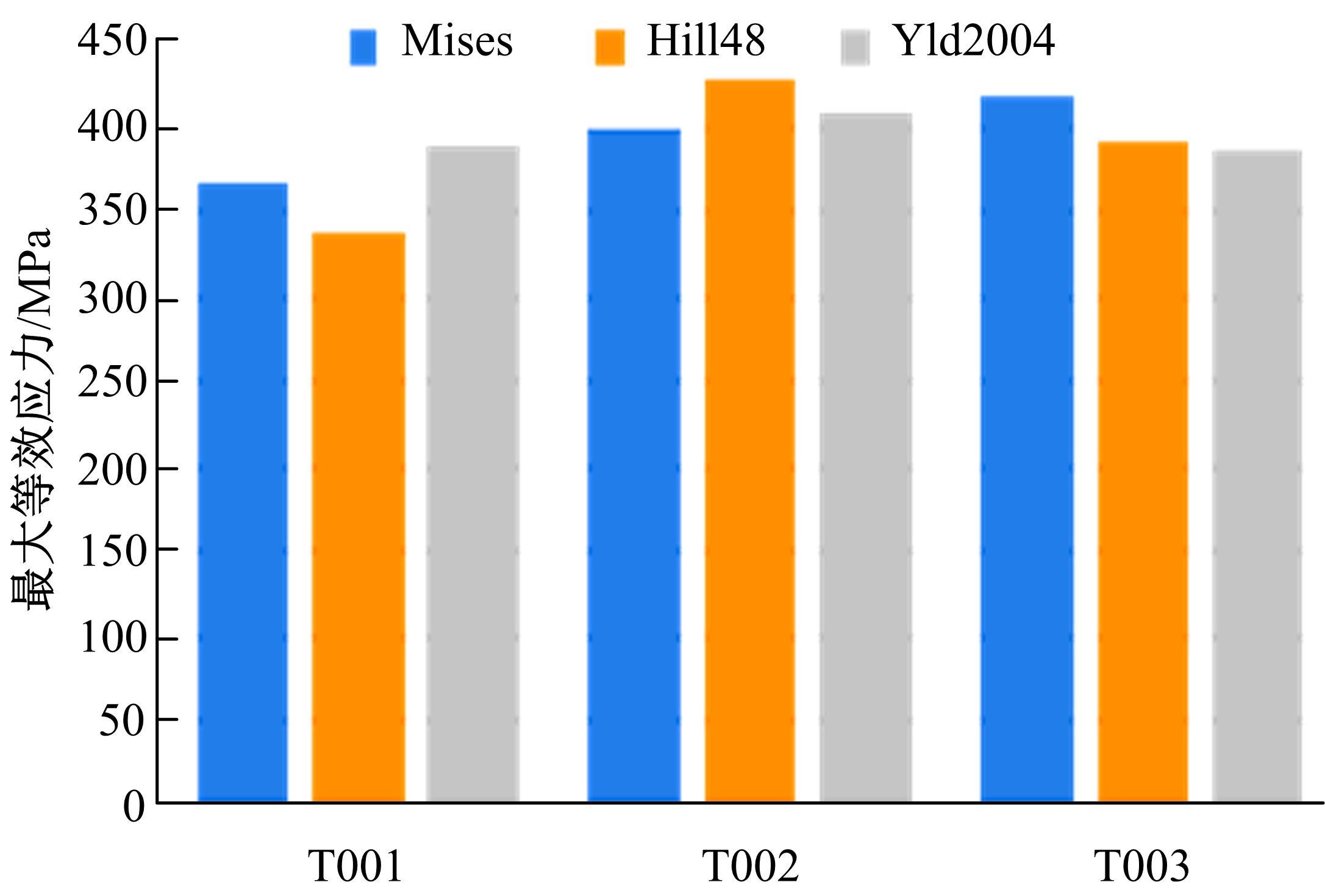
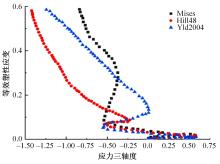
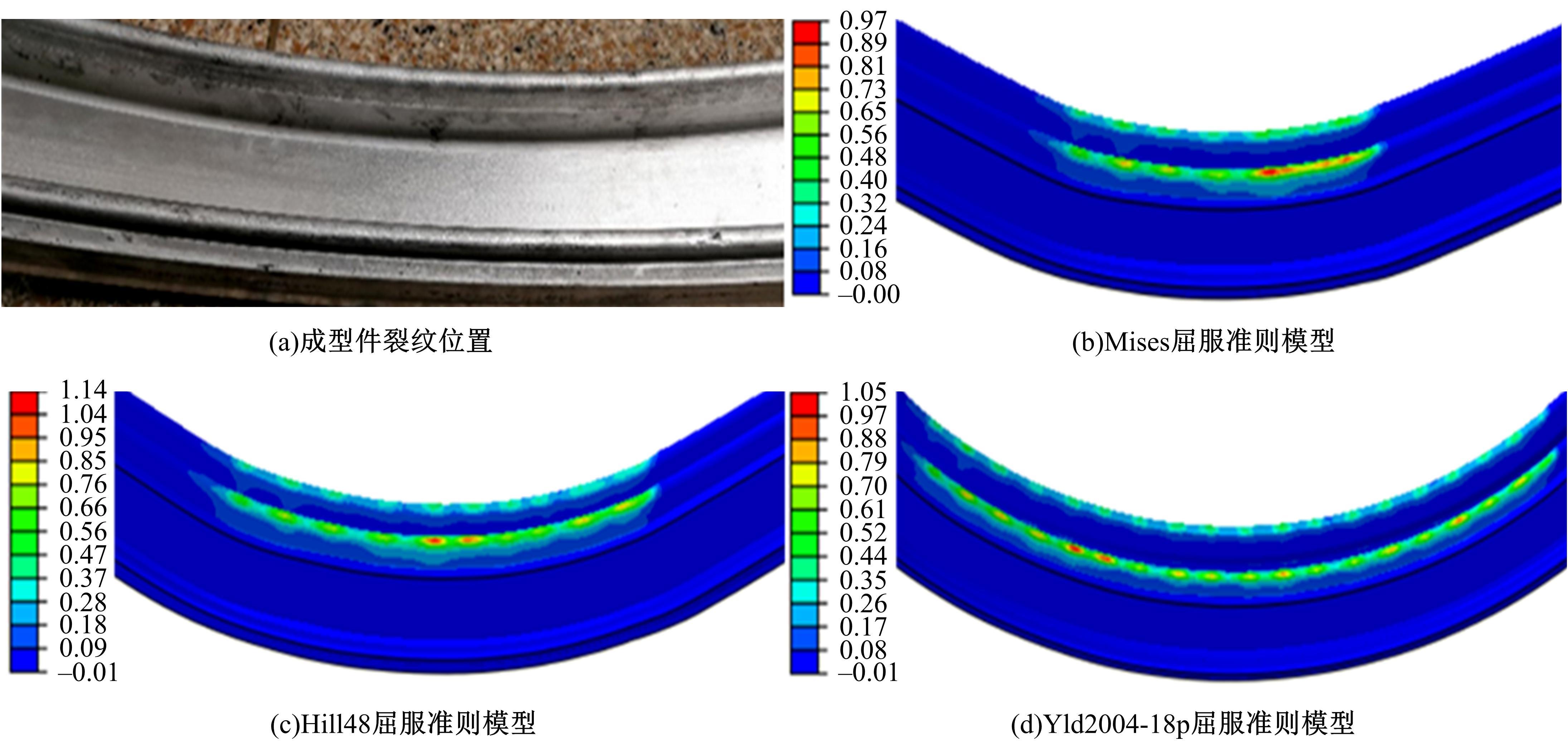
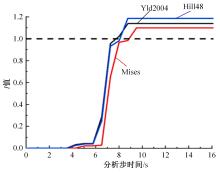
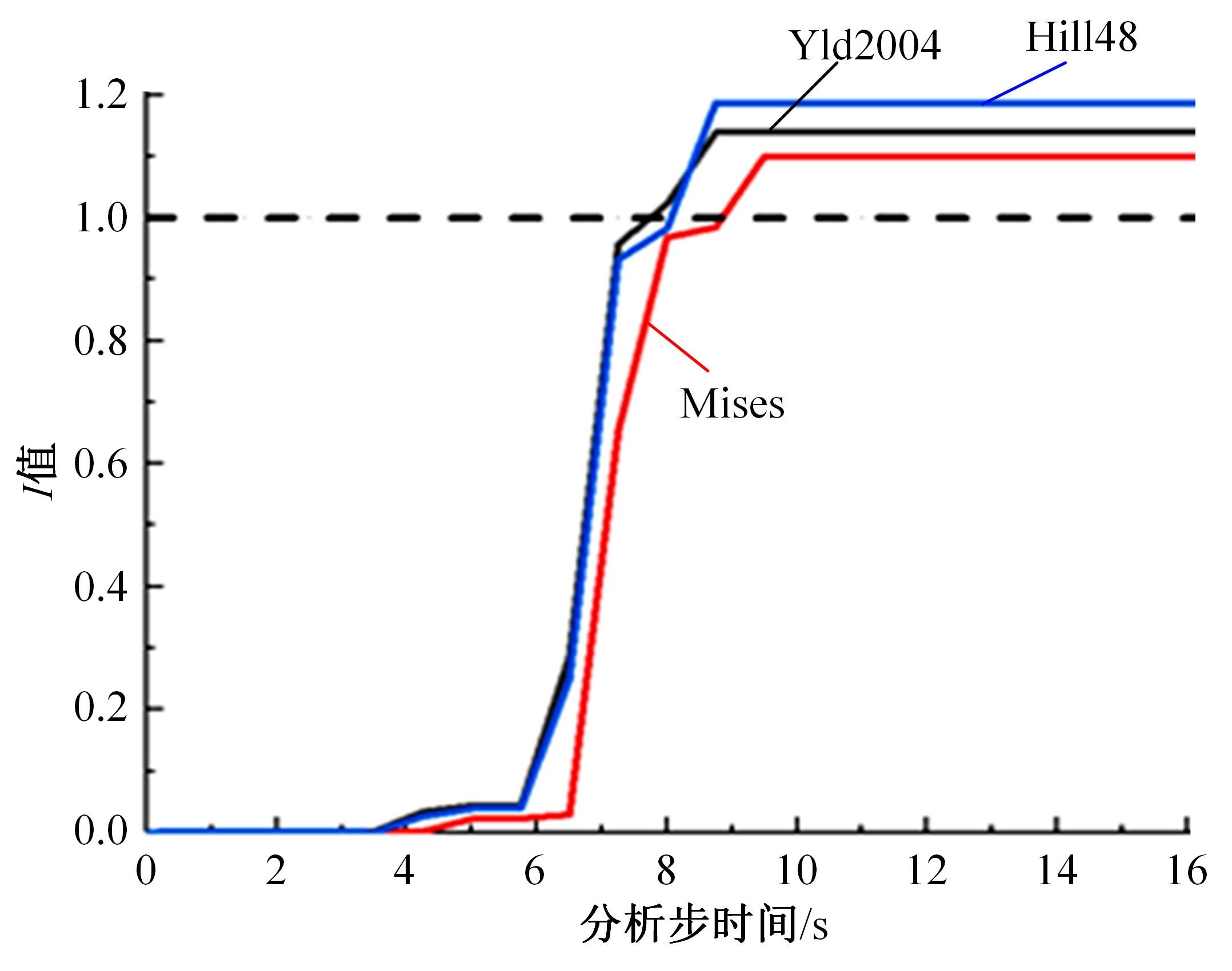
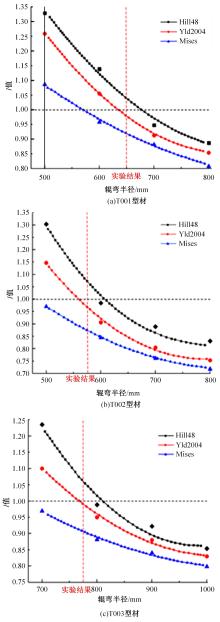
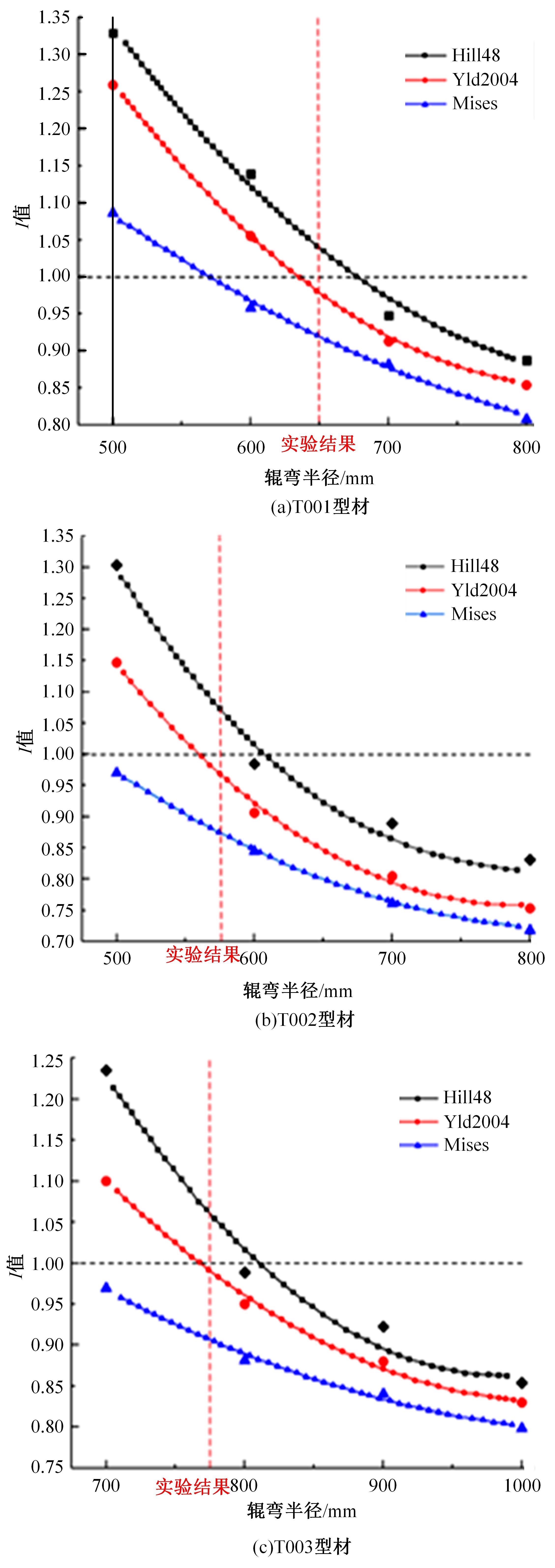
以6005A铝合金型材为例，提出了一种考虑各向异性的铝合金型材辊弯断裂预测方法，分别采用von Mises、Hill48和Yld2004-18p屈服准则描述铝合金的各向异性，通过实验和晶体有限元模拟确定了模型参数。模拟结果与辊弯实验结果对比表明：采用Mises屈服准则的预测误差大于15%；采用Hill48屈服准则的预测误差小于5%；采用Yld2004-18p屈服准则的预测误差小于3%。说明使用各向异性屈服准则与连续损伤准则耦合的有限元模型，比各向同性模型能更准确地预测铝合金型材辊弯成形中的断裂行为。

关键词: 材料合成与加工工艺, 型材辊弯, 各向异性, 断裂准则, 晶体塑性

Abstract:

An anisotropic roll bending fracture prediction method was proposed for 6005A aluminum alloy profile. Von Mises， Hill48 and Yld2004-18p yield criteria were used to describe the anisotropy of aluminum alloy respectively. Model parameters were determined by experiments and crystal finite element simulation. The results of simulation and roll bending test show that the predicted error of Mises yield criterion is more than 15%， Hill48 yield criterion is less than 5% and Yld2004-18p yield criterion is less than 3%. It shows that the finite element model coupled with the anisotropic yield criterion and the continuous damage criterion can predict the fracture behavior of aluminum alloy profiles in roll bending more accurately than the isotropic model.

Key words: material synthesis and processing technology, profiles roll bending, anisotropy, fracture criterion, crystal plasticity

中图分类号:

TG386

刘纯国,李紫桐,张学广,李明. 考虑各向异性的铝合金型材辊弯断裂预测[J]. 吉林大学学报(工学版), 2023, 53(12): 3388-3396.

Chun-guo LIU,Zi-tong LI,Xue-guang ZHANG,Ming LI. Prediction of fracture of aluminum alloy profiles in roll bending considering anisotropy[J]. Journal of Jilin University(Engineering and Technology Edition), 2023, 53(12): 3388-3396.

图/表 13

图1

图2

图3

图4

图5

表1

Yld2004-18p屈服准则参数"

参数	取值	参数	取值	参数	取值
c $12'$	1.0223	c $44'$	0.9314	$c 23 ″$	0.9932
c $13'$	0.8537	c $55'$	1.0223	$c 31 ″$	0.9314
c $21'$	0.8722	c $66'$	0.8537	$c 32 ″$	0.9329
c $23'$	1.1434	$c 12 ″$	0.8721	$c 44 ″$	1.0038
c $31'$	1.0038	$c 13 ″$	1.1434	$c 55 ″$	0.8998
c $32'$	0.9932	$c 21 ″$	1.0038	$c 66 ″$	1.4962

表1

图6

图7

图8

图9

图10

图11

表2

参考文献 16

1	Shi L, Wen J B, Ren C. The prediction of microstructure evolution of 6005A aluminum alloy in a P-ECAP extrusion study[J]. Journal of Materials Engineering and Performance, 2018, 27(5): 2566-2575.
2	韩飞, 刘继英, 艾正青, 等. 辊弯成型技术理论及应用研究现状[J]. 塑性工程学报, 2010, 17(5): 53-60.
	Han Fei, Liu Ji-ying, Ai Zheng-qing, et al. Research status of roll forming technology theory and application[J]. Chinese Journal of Plastic Engineering, 2010, 17(5): 53-60.
3	Yan Y, Wang H B, Li Q. The inverse parameter identification of Hill 48 yield criterion and its verification in press bending and roll forming process simulations[J]. Journal of Manufacturing Processes, 2015, 20(1): 46-53.
4	Li S, Engler O, Houtte P V. Plastic anisotropy and texture evolution during tensile testing of extruded aluminium profiles[J]. Modelling and Simulation in Materials Science and Engineering, 2005, 13(5): 783-795.
5	Lian J, Shen F, Jia X, et al. An evolving non-associated Hill48 plasticity model accounting for anisotropic hardening and r-value evolution and its application to forming limit prediction[J]. International Journal of Solids and Structures, 2018, 151: 20-44.
6	Basak S, Panda S K, Lee M-G. Formability and fracture in deep drawing sheet metals: Extended studies for pre-strained anisotropic thin sheets[J]. International Journal of Mechanical Sciences, 2020, 170: No. 105346.
7	Achani D, Hopperstad O S, Lademo O G. Influence of advanced yield criteria on predictions of plastic anisotropy for aluminium alloy sheets[J]. International Journal of Material Forming, 2009, 2(Sup.1): 487-490.
8	Dick R E, Yoon J W. Plastic anisotropy and failure in thin metal: Material characterization and fracture prediction with an advanced constitutive model and polar EPS (effective plastic strain) fracture diagram for AA 3014-H19[J]. International Journal of Solids and Structures, 2018, 151: 195-213.
9	Lou Y, Yoon J W. Alternative approach to model ductile fracture by incorporating anisotropic yield function[J]. International Journal of Solids and Structures, 2019, 164: 12-24.
10	Grytten F, Holmedal B, Hopperstad O S, et al. Evaluation of identification methods for YLD2004-18p[J]. International Journal of Plasticity, 2008, 24(12): 2248-2277.
11	Bate P, An Y. Plastic anisotropy in AA5005 Al-1Mg: predictions using crystal plasticity finite element analysis[J]. Scripta Materialia, 2004, 51(10): 973-977.
12	Wei P, Lu C, Liu H, et al. Study of anisotropic plastic behavior in high pressure torsion of aluminum single crystal by crystal plasticity finite element method[J]. Crystals, 2017, 7(12): 362-372.
13	黄建科. 金属成形过程的细观损伤力学模型及韧性断裂准则研究[D]. 上海:上海交通大学材料科学与工程学院, 2009.
	Huang Jian-ke. Research on meso-scale damage mechanics model and ductile fracture criterion of metal forming process[D]. Shanghai: School of Materials Science and Engineering, Shanghai Jiaotong University, 2009.
14	高付海, 桂良进, 范子杰. 基于韧性准则的金属板料冲压成形断裂模拟[J]. 工程力学, 2010, 27(2): 204-208.
	Gao Fu-hai, Gui Liang-jin, Fan Zi-jie. Fracture simulation of sheet metal stamping based on toughness criterion[J]. Engineering Mechanics, 2010, 27(2): 204-208.
15	Rice J R, Tracey D M. On the ductile enlargement of voids in triaxial stress fields[J]. Journal of the Mechanics and Physics of Solids, 1969, 17(3): 201-217.
16	Liu C, Li M, Yue T. Thick anisotropy analysis for AA7B04 aluminum plate using CPFEM and its application for springback prediction in multi-point bending[J]. The International Journal of Advanced Manufacturing Technology, 2021, 115(4): 1139-1153.

相关文章 15

[1]	朱先勇,谢良稳,樊跃香,姜城,孙炜佳,王鹏,肖雄. 搅拌摩擦加工参数对镁合金表面改性层的影响[J]. 吉林大学学报(工学版), 2023, 53(8): 2263-2271.
[2]	张恒,詹志刚,陈奔,隋邦杰,潘牧. 气体扩散层各向异性传输特性的孔尺度模拟[J]. 吉林大学学报(工学版), 2022, 52(9): 2055-2062.
[3]	姬会爽,彭艳,刘洋,杨彦博,高鲲,尔立民. SCR生产线结晶腔铜液凝固行为及质量控制[J]. 吉林大学学报(工学版), 2021, 51(6): 2021-2030.
[4]	向红亮,陈盛涛,邓丽萍,张伟,詹土生. 微合金化2205双相不锈钢组织及性能[J]. 吉林大学学报(工学版), 2020, 50(5): 1645-1652.
[5]	谷晓燕,隋成龙,狄星,孟政宇,朱开轩,楚长春. 焊接能量对铜/钛超声波焊接接头性能的影响[J]. 吉林大学学报(工学版), 2020, 50(5): 1669-1676.
[6]	李小雨,许男,仇韬,郭孔辉. 各向异性刚度对轮胎力学特性及车辆操纵性的影响[J]. 吉林大学学报(工学版), 2020, 50(2): 389-398.
[7]	蔡中义,孟凡响,陈庆敏,赵轩. 复杂钩舌锻件近净成形的预锻形状优化设计[J]. 吉林大学学报(工学版), 2020, 50(1): 84-90.
[8]	庄蔚敏,施宏达,解东旋,杨冠男. 钢铝异质无铆钉粘铆复合连接胶层厚度分布[J]. 吉林大学学报(工学版), 2020, 50(1): 100-106.
[9]	关庆丰,姚欣雯,杨洋,张凌燕,刘迪,李晨,吕鹏. 强流脉冲电子束作用下TC4钛合金表面Cr合金层制备及性能[J]. 吉林大学学报(工学版), 2019, 49(6): 2002-2009.
[10]	石舟,寇淑清. 36MnVS4裂解连杆性能分析及轻量化设计[J]. 吉林大学学报(工学版), 2019, 49(6): 1992-2001.
[11]	依卓,付文智,李明哲. 双层剖分式超高压模具数值模拟及实验[J]. 吉林大学学报(工学版), 2019, 49(5): 1593-1599.
[12]	谷晓燕,刘东锋,刘婧,孙大千,马会峰. 焊接能量对Cu/Al超声波焊接接头组织与性能的影响[J]. 吉林大学学报(工学版), 2019, 49(5): 1600-1607.
[13]	李欣,孙延朋,王丹,陈军绪,谷诤巍,徐虹. 汽车前地板成形有限元数值模拟[J]. 吉林大学学报(工学版), 2019, 49(5): 1608-1614.
[14]	李欣,王丹,陈军绪,孙延朋,谷诤巍,徐虹. 手刹固定板冲压成形数值模拟[J]. 吉林大学学报(工学版), 2019, 49(4): 1258-1265.
[15]	刘文权,盈亮,荣海,胡平. 基于损伤修正M⁃K模型的高强度钢成形极限预测[J]. 吉林大学学报(工学版), 2019, 49(4): 1266-1271.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed