吉林大学学报(工学版) ›› 2023, Vol. 53 ›› Issue (2): 364-375.doi: 10.13229/j.cnki.jdxbgxb20220644
一类多刚性限幅振动系统的动态稳定性分析
- 1.兰州交通大学 机电工程学院,兰州 730070
2.甘肃省轨道交通装备系统动力学与可靠性重点实验室,兰州 730070
Dynamic stability analysis of a amplitude⁃limited vibration system with multiple rigid constraints
Yan YANG1,2( ),Yu-qing SHI1,2,Xiao-rong ZHANG2,Guan-wei LUO2
),Yu-qing SHI1,2,Xiao-rong ZHANG2,Guan-wei LUO2
- 1.School of Mechatronic Engineering,Lanzhou Jiaotong University,Lanzhou 730070,China
2.Gansu Provincial Key Laboratory of System Dynamics and Reliability of Rail Transport Equipment,Lanzhou 730070,China
摘要:
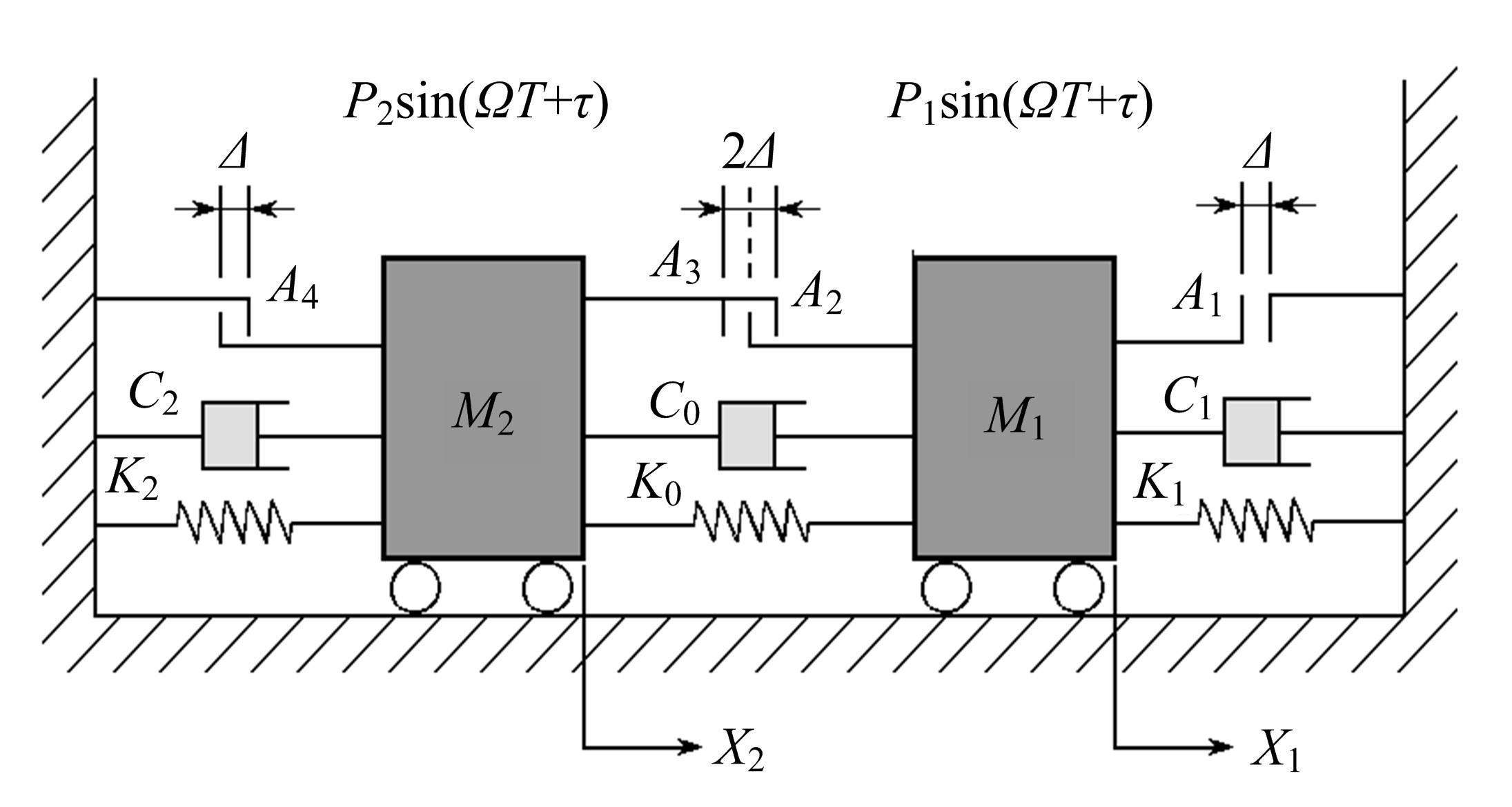
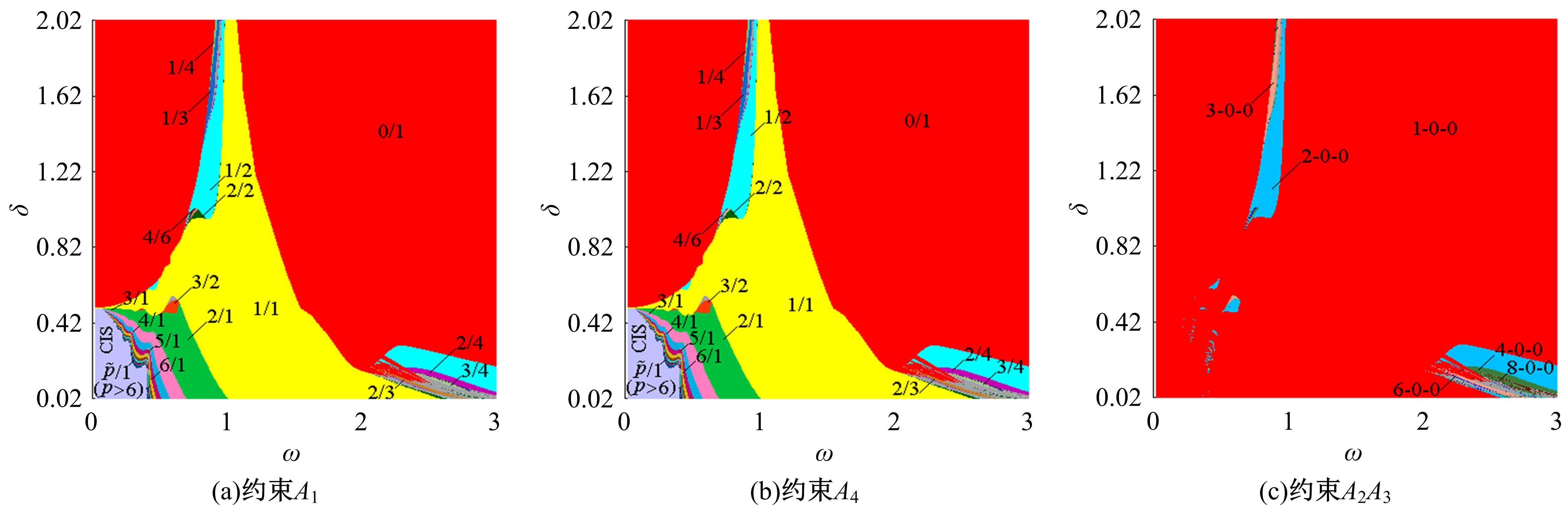
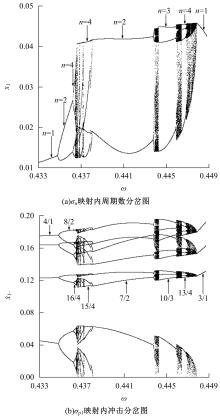
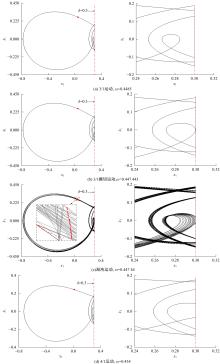
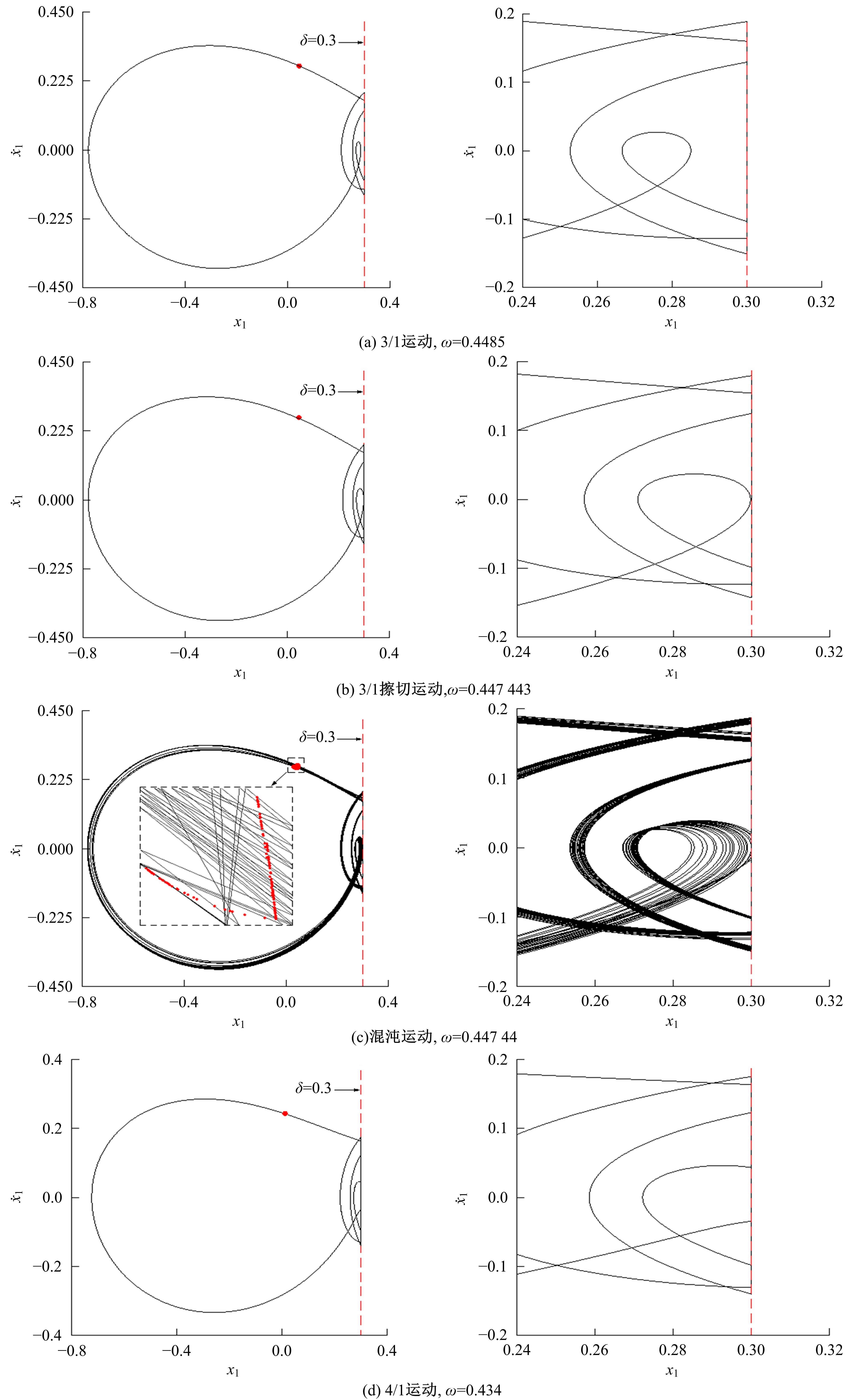
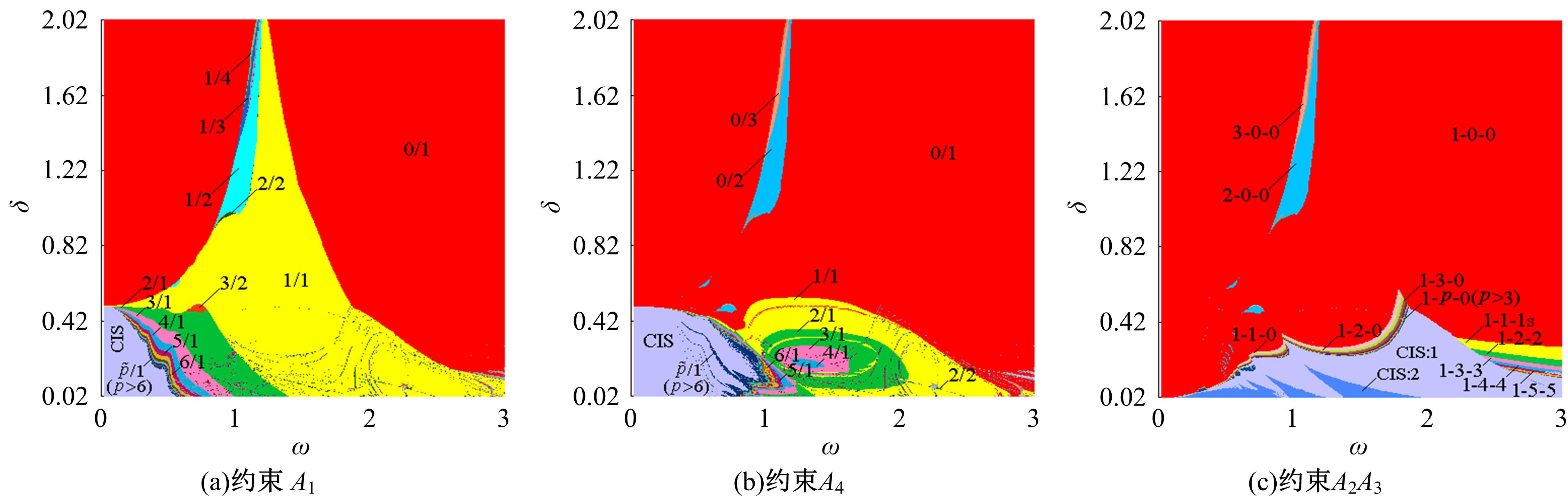
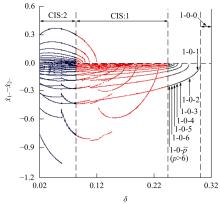
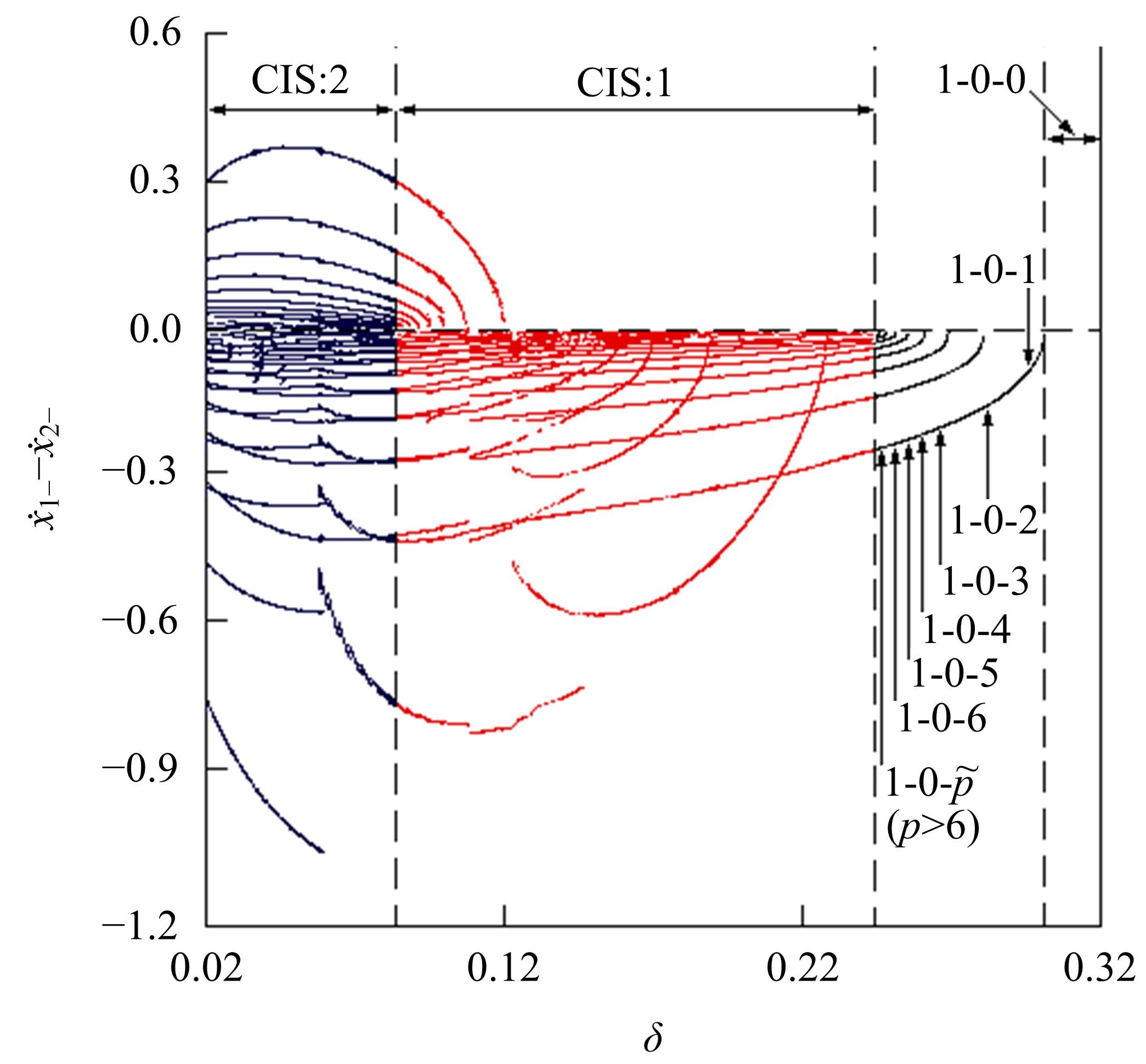
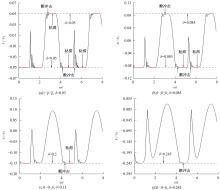
建立了一类含有多刚性限幅约束的两自由度受迫振动系统的力学模型。结合系统周期和冲击Poincaré映射,在双参数系内识别出周期冲击运动的模式多样性及其发生域。伴随不连续的擦切分岔,在相邻单周期冲击运动分布域的临界线上存在一系列奇异点、迟滞和舌形转迁域。根据动力学参数的采样范围,分析了极端质量分布工况下振动系统的动态响应。仿真结果表明:在低频区域的单周期冲击运动序列随频率递减,经连续的擦切分岔、滑移分岔等非光滑分岔行为,存在向非完全颤振冲击、完全颤振冲击转迁的形成规律。冲击瞬时的能量损失过大会导致粘滞现象,从而改变振动系统的原结构,降低系统自由度。由于多处刚性约束完全颤振冲击的同时作用,系统在极端参数控制域内会呈现短暂的停滞状态。
中图分类号:
- TH113.1
| 1 | Wan Qi, Liu Geng, Song Chun-yu, et al. Study on the dynamic interaction of multiple clearance joints for flap actuation system with a modified contact force model[J]. Journal of Mechanical Science and Technology, 2020, 34(7): 2701-2713. |
| 2 | 张树培,夏明悦,张玮,等. 考虑非线性刚度的间隙球铰碰撞动力学建模与仿真[J/OL]. [2022-02-07]. |
| 3 | 张发军,张烽,宋钰青,等. 铰碰与臂重激振下串联机械臂参数的抑振优化[J]. 哈尔滨工程大学学报, 2019, 40(5): 1000-1005. |
| Zhang Fa-jun, Zhang Feng, Song Yu-qing, et al. Parameters optimization based on vibration suppression of manipulator undergoing internal collision of hinges and heavy vibration of arms[J]. Journal of Harbin Engineering University, 2019, 40(5): 1000-1005. | |
| 4 | Alok S, Chaudhary K, Guha A. A study on critical order of joints with clearances and its effect on kinematic performance of multiloop planar mechanisms[J]. Sadhana-Academy Proceedings In Engineering Sciences, 2020, 45(1): 126. |
| 5 | Jiang S, Chen X L. Reducing undesirable effects of clearances on dynamic and wear of planar multi-link mechanism[J]. Nonlinear Dynamics, 2020, 100(3): 1-29. |
| 6 | Shin D, Palazzolo A. Nonlinear analysis of a geared rotor system supported by fluid film journal bearings[J]. Journal of Sound and Vibration, 2020, 475: 115269. |
| 7 | 林何,屈文宽, Rätsch Matthias,等. 齿轮-轴承系统非线性振动混沌吸引子周期轨道控制[J]. 振动与冲击, 2020, 39(13): 1-6, 15. |
| Lin He, Qu Wen-kuan, Rätsch Matthias, et al. Periodic orbit control of nonlinear vibration chaotic attractors of a gear-bearing system[J]. Journal of Vibration and Shock, 2020, 39(13): 1-6, 15. | |
| 8 | 石怀龙,郭金莹,王勇. 变轨距高速列车的动力学[J]. 机械工程学报, 2020, 56(20): 98-105. |
| Shi Huai-long, Guo Jin-ying, Wang Yong. Dynamic performance of high-speed gauge-changeable railway vehicle[J]. Journal of Mechanical Engineering, 2020, 56(20): 98-105. | |
| 9 | Lv Xiao-hong, Gao Quan-fu, Luo Guan-wei. Dynamic characteristics of a mechanical impact oscillator with a clearance[J]. International Journal of Mechanical Sciences, 2020, 178: 105605. |
| 10 | 申永康,殷珊,徐慧东,等. 碰撞振动系统不连续擦边分岔的线性反馈控制[J]. 应用力学学报, 2018, 35(4): 688-693. |
| Shen Yong-kang, Yin Shan, Xu Hui-dong, et al.Linear feedback control of the discontinuous grazing bifurcation in a vibro-impact system[J]. Chinese Journal of Applied Mechanics, 2018, 35(4): 688-693. | |
| 11 | Wei Dao-gao, Wang Wei-jia, Wang Bo, et al. Bifurcation and chaotic behaviors of vehicle brake system under low speed braking condition[J]. Journal of Vibration Engineering and Technologies, 2021, 9(8):2107-2120. |
| 12 | Thi H L, Junca S, Legrand M. Periodic solutions of a two-degree-of-freedom autonomous vibro-impact oscillator with sticking phases[J]. Nonlinear Analysis-Hybrid Systems, 2018, 28: 54-74. |
| 13 | Alzate R, Piiroinen P T, Bernardo M D. From complete to incomplete chattering: a novel route to chaos in impacting cam-follower systems[J]. International Journal of Bifurcation and Chaos, 2012, 22(5): 1250102. |
| 14 | Hős C, Champneys A R. Grazing bifurcations and chatter in a pressure relief valve model[J]. Physica D: Nonlinear Phenomena, 2012, 241(22): 2068-2076. |
| 15 | Baranyai T, Várkonyi P L. Zeno chattering of rigid bodies with multiple point contacts[J]. Nonlinear Dynamics, 2017, 92(4): 1857-1879. |
| 16 | Costa D, Vaziri V, Kapitaniak M, et al. Chaos in impact oscillators not in vain: dynamics of new mass excited oscillator[J]. Nonlinear Dynamics, 2020, 102: 835-861. |
| 17 | 王锐,田晓超,杨志刚,等. 压电低频旋转驱动器的设计与实验[J]. 吉林大学学报: 工学版, 2020, 50(4): 1283-1287. |
| Wang Rui, Tian Xiao-chao, Yang Zhi-gang, et al. Design and experimental of piezoelectric low-frequency driven rotary actuator[J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(4): 1283-1287. | |
| 18 | Oruganti P S, Krak M D, Singh R. Step responses of a torsional system with multiple clearances: Study of vibro-impact phenomenon using experimental and computational methods[J]. Mechanical Systems and Signal Processing, 2018, 99: 83-106. |
| 19 | Liu Y, Chávez P J, Guo B Y, et al. Bifurcation analysis of a vibro-impact experimental rig with two-sided constraint[J]. Meccanica, 2020, 55: 2505-2521. |
| 20 | Dumanli A, Sencer B. Active control of high frequency chatter with machine tool feed drives in turning[J]. CIRP Annals-Manufacturing Technology, 2021, 70(1): 309-312. |
| 21 | 陈云,侯亮,刘文志,等.基于时域仿真法的断续铣削颤振预测[J]. 机械工程学报, 2021, 57(3): 98-106. |
| Chen Yun, Hou Liang, Liu Wen-zhi, et al. Chatter stability prediction in low immersion milling based on time-domain simulation[J]. Journal of Mechanical Engineering, 2021, 57(3): 98-106. | |
| 22 | Luo G W, Lv X H, Shi Y Q. Vibro-impact dynamics of a two-degree-of freedom periodically-forced system with a clearance: diversity and parameter matching of periodic-impact motions[J]. International Journal of Non-Linear Mechanics,2014,65:173-195. |
| [1] | 刘洋. 动臂塔机卸载冲击仿真及试验[J]. 吉林大学学报(工学版), 2022, 52(6): 1292-1300. |
| [2] | 程亚兵,陈璐翔,葛平玉,杨泽宇,曹鹏宇. 双相正时套筒链的动力学仿真分析及磨损失效[J]. 吉林大学学报(工学版), 2022, 52(4): 781-788. |
| [3] | 赵洋,肖洋,孙皓,霍文浩,冯松,廖勇. 基于围道积分的润滑接触齿轮微点蚀损伤特征模拟[J]. 吉林大学学报(工学版), 2022, 52(4): 799-810. |
| [4] | 张朋成,彭斌,张宇波. 基于圆渐开线建立变截面涡旋齿的方法与理论[J]. 吉林大学学报(工学版), 2022, 52(4): 789-798. |
| [5] | 张龙,徐天鹏,王朝兵,易剑昱,甄灿壮. 基于卷积门控循环网络的齿轮箱故障诊断[J]. 吉林大学学报(工学版), 2022, 52(2): 368-376. |
| [6] | 郑伟,孙见君,马晨波,於秋萍,张玉言,牛韬. 汽车轮毂加工夹具的研究现状及展望[J]. 吉林大学学报(工学版), 2022, 52(1): 25-36. |
| [7] | 田晓超,王海刚,王虎,王志聪,赵剑,杨志刚,吴越. 悬臂式压电振子宽频发电装置设计及测试[J]. 吉林大学学报(工学版), 2021, 51(6): 1953-1958. |
| [8] | 陈魏,雷雨龙,李兴忠,付尧,扈建龙,侯利国. 低速工况下渐开线圆柱直齿轮齿面粘着磨损计算[J]. 吉林大学学报(工学版), 2021, 51(5): 1628-1634. |
| [9] | 胡晶,李聪,张邦成,乔晓利,张心明,周笑平. 考虑离心膨胀的双级串联轴承油膜刚度和阻尼[J]. 吉林大学学报(工学版), 2021, 51(5): 1601-1611. |
| [10] | 姚宗伟,高旭东,刘刚,毕秋实. 基于数值仿真的大型塔式磨机工作特性分析[J]. 吉林大学学报(工学版), 2021, 51(5): 1642-1650. |
| [11] | 李晓韬,任金鹏,李晓旭,杨航. 双压电振子反相模态驱动的直线驱动器机理[J]. 吉林大学学报(工学版), 2021, 51(2): 468-471. |
| [12] | 侯才生,刘涛,郭李先. 一种新型变壁厚涡旋型线的构建理论[J]. 吉林大学学报(工学版), 2020, 50(5): 1627-1634. |
| [13] | 程功,肖科,王家序,蒲伟,韩彦峰. 混合润滑状态下齿轮接触刚度[J]. 吉林大学学报(工学版), 2020, 50(2): 494-503. |
| [14] | 毛艳,成凯. 基于Hopfield神经网络的单缸插销式伸缩臂伸缩路径优化[J]. 吉林大学学报(工学版), 2020, 50(1): 53-65. |
| [15] | 郭震,于红英,滑忠鑫,赵娣. 刚性折纸机构运动分析及折叠过程仿真[J]. 吉林大学学报(工学版), 2020, 50(1): 66-76. |
|