吉林大学学报(工学版) ›› 2020, Vol. 50 ›› Issue (5): 1905-1912.doi: 10.13229/j.cnki.jdxbgxb20190489
• 通信与控制工程 • 上一篇
基于极局部模型的机械臂自适应滑模控制
- 天津大学 电气自动化与信息工程学院,天津 300072
Adaptive sliding mode control based on ultra⁃local model for robotic manipulator
Ai-guo WU( ),Jun-qing HAN,Na DONG
),Jun-qing HAN,Na DONG
- School of Electrical and Information Engineering, Tianjin University, Tianjin 300072, China
摘要:
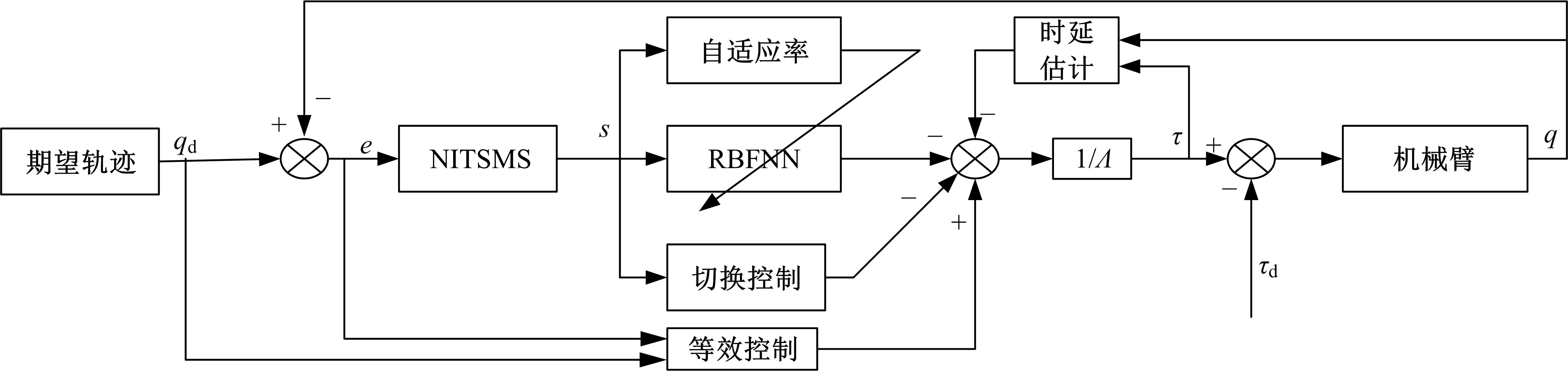
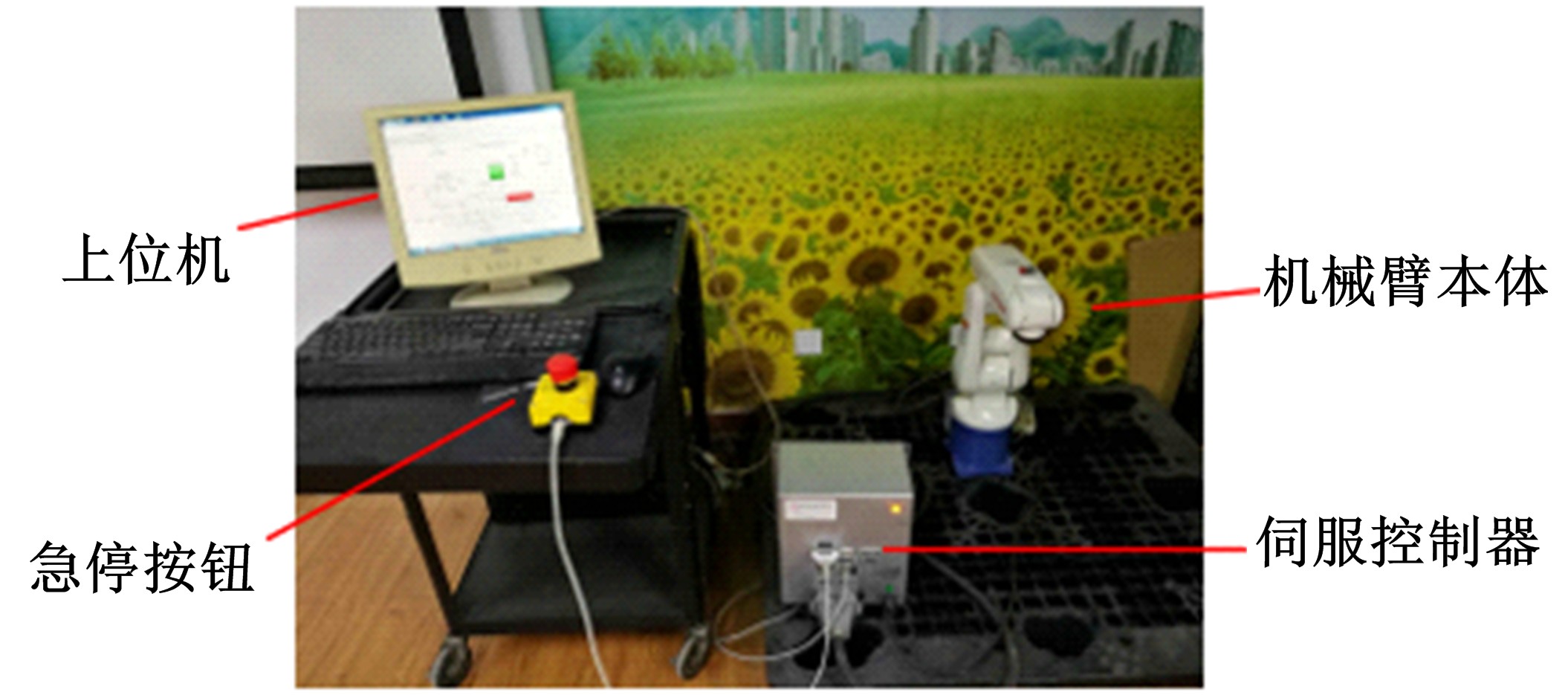
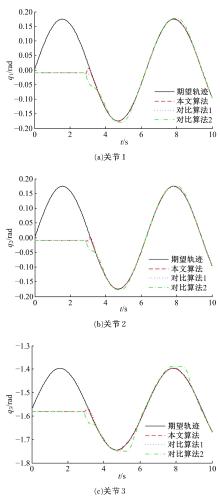
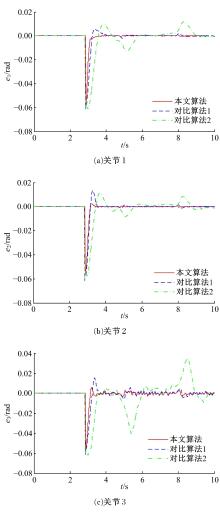
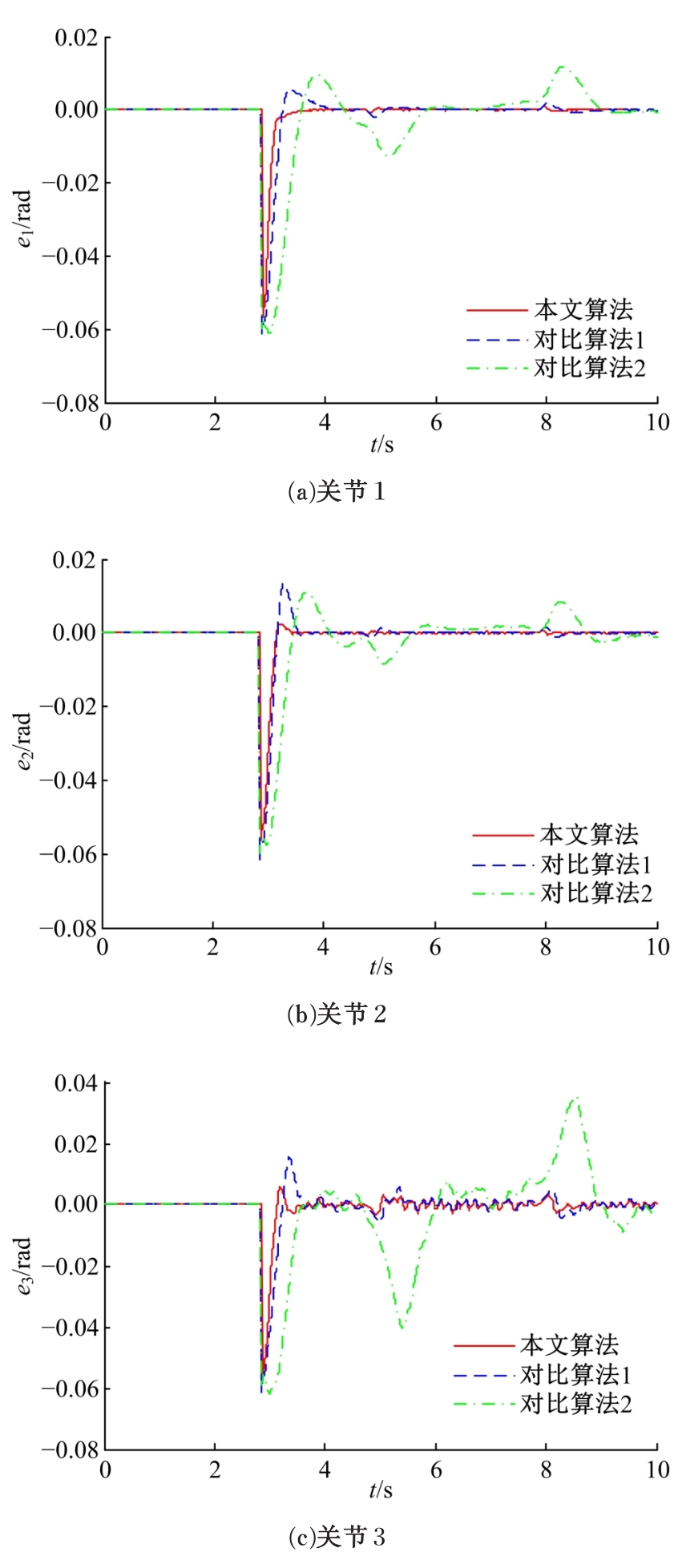
针对多自由度机械臂在实现轨迹跟踪控制时过于依赖机械臂的精确数学模型和跟踪精度低等问题,提出了一种将自适应神经网络、极局部模型与积分型终端滑模相结合的控制方法。该方法使用一种基于时延估计的极局部模型来近似机械臂的动力学模型,利用自适应神经网络的非线性逼近性能,补偿时延估计产生的误差;对极局部模型设计积分型滑模控制器提高系统的收敛速度和控制精度,实现不依靠动力学模型的机械臂高精度轨迹跟踪。通过李雅普诺夫理论证明系统的稳定性和有限时间收敛性。最后通过实验,验证了该控制方法可以在完全不依赖模型信息的前提下实现机械臂的高速度和高精度跟踪控制。
中图分类号:
- TP241
| 1 | Boulkroune A, Bouzeriba A, Bouden T. Fuzzy generalized projective synchronization of incommensurate fractional-order chaotic systems[J]. Neurcomputing, 2016, 173: 606-614. |
| 2 | Ortega R, Spong M W. Adaptive motion control of rigid robot: a tutorial[J]. Automatica, 1989, 25(6): 877-888. |
| 3 | Utkin V. Sliding Mode in Control and Optimization[M]. Berlin: Springer-Verlag, 1992. |
| 4 | Feng Y, Yu X, Man Z. Non-singular terminal sliding mode control of rigid manipulators[J]. Automatica, 2002, 38(12): 2159-2167. |
| 5 | Van C P, Nan W Y. Adaptive trajectory tracking neural network control with robust compensator for robot manipulators[J]. Neural Computing and Applications, 2016, 27(2): 525-536. |
| 6 | Fateh M M, Azargoshasb S. Discrete adaptive fuzzy control for asymptotic tracking of robotic manipulators[J]. Nonlinear Dynamics, 2014, 78(3): 2195-2204. |
| 7 | 胡立坤, 马文光, 赵鹏飞, 等. 六自由度机械臂的非奇异快速终端滑模控制[J]. 吉林大学学报: 工学版, 2014, 44(3): 734-741. |
| Hu Li-kun, Ma Wen-guang, Zhao Peng-fei, et al. Non-singular fast terminal sliding mode control method for 6-DOF manipulator[J]. Journal of Jilin University(Engineering and Technology Edition), 2014, 44(3): 734-741. | |
| 8 | 王伟, 赵健廷, 胡宽荣, 等. 基于快速非奇异终端滑模的机械臂轨迹跟踪方法[J]. 吉林大学学报: 工学版, 2020, 50(2): 464-471. |
| Wang Wei, Zhao Jian-ting, Hu Kuan-rong, et al. Trajectory tracking of robotic manipulators based on fast nonsingular terminal sliding mode[J]. Journal of Jilin University(Engineering and Technology Edition), 2020, 50(2): 464-471. | |
| 9 | 李元春, 王蒙, 盛立辉, 等. 液压机械臂基于反演的自适应二阶滑模控制[J]. 吉林大学学报: 工学报, 2015, 45(1): 193-201. |
| Li Yuan-chun, Wang Meng, Sheng Li-hui, et al. Adaptive second order sliding mode control for hydraulic manipulator based on backstepping[J]. Journal of Jilin University(Engineering and Technology Edition), 2015, 45(1): 193-201. | |
| 10 | Fliess M, Join C. Model-free control[J]. International Journal of Control, 2013, 86(12): 2228-2252. |
| 11 | Wang H, Ye X, Tian Y, et al. Model-free-based terminal SMC of quadrotor attitude and position[J]. IEEE Transactions on Aerospace and Electronic Systems, 2016, 52(5): 2519-2528. |
| 12 | Han S S, Wang H P, Tian Y. Model-free based adaptive nonsingular fast sliding mode control with time-delay estimation for a 12 DOF multi-functional lower limb exoskeleton[J]. Advances in Engineering Software, 2018, 119: 38-47. |
| 13 | 章心忆. 下肢外骨骼机器人的建模和控制策略研究[D]. 南京: 南京理工大学自动化学院, 2017. |
| Zhang Xin-yi. Modeling and control method reserch for lower limb exoskeleton robot[D]. Nanjing: Automatic College, Nanjing University of Technology, 2017. | |
| 14 | 霍伟. 机器人动力学与控制[M]. 北京: 高等教育出版社, 2005. |
| 15 | Maolin J, Lee J, Chang P H, et al. Practical nonsingular terminal sliding mode control of robot manipulators for high-accuracy tracking control[J]. IEEE Transactions on Industrial Electronics, 2009, 56(9): 3593-3601. |
| 16 | Bhat S P, Berstein D S. Finite time stability of homogeneous systems[C]∥Proceedings of the American Control Conference, Albuquerque, 1997: 2513-2514. |
| 17 | Park J, Sandberg I W. Universal approximation using radial basis function networks[J]. Neural Computation, 1991, 3(2): 246-257. |
| 18 | Bhat S, Berstein D. Finite-time stability of continuous autonomous system[J]. SIAM Journal of Control Optimization, 2000, 38(3): 751-766. |
| [1] | 王柯俨,王迪,赵熹,陈静怡,李云松. 基于卷积神经网络的联合估计图像去雾算法[J]. 吉林大学学报(工学版), 2020, 50(5): 1771-1777. |
| [2] | 李静,石求军,洪良,刘鹏. 基于车辆状态估计的商用车ESC神经网络滑模控制[J]. 吉林大学学报(工学版), 2020, 50(5): 1545-1555. |
| [3] | 刘国华,周文斌. 基于卷积神经网络的脉搏波时频域特征混叠分类[J]. 吉林大学学报(工学版), 2020, 50(5): 1818-1825. |
| [4] | 李志军,杨楚皙,刘丹,孙大洋. 基于深度卷积神经网络的信息流增强图像压缩方法[J]. 吉林大学学报(工学版), 2020, 50(5): 1788-1795. |
| [5] | 陈吉清,蓝庆生,兰凤崇,刘照麟. 基于轮胎力预判与拟合的轨迹跟踪控制[J]. 吉林大学学报(工学版), 2020, 50(5): 1565-1573. |
| [6] | 车翔玖,董有政. 基于多尺度信息融合的图像识别改进算法[J]. 吉林大学学报(工学版), 2020, 50(5): 1747-1754. |
| [7] | 谌华,郭伟,闫敬文,卓文浩,吴良斌. 基于深度学习的SAR图像道路识别新方法[J]. 吉林大学学报(工学版), 2020, 50(5): 1778-1787. |
| [8] | 张根保,李浩,冉琰,李裘进. 一种用于轴承故障诊断的迁移学习模型[J]. 吉林大学学报(工学版), 2020, 50(5): 1617-1626. |
| [9] | 王伟,赵健廷,胡宽荣,郭永仓. 基于快速非奇异终端滑模的机械臂轨迹跟踪方法[J]. 吉林大学学报(工学版), 2020, 50(2): 464-471. |
| [10] | 刘富,安毅,董博,李元春. 基于ADP的可重构机械臂能耗保代价分散最优控制[J]. 吉林大学学报(工学版), 2020, 50(1): 342-350. |
| [11] | 曲兴田,王学旭,孙慧超,张昆,闫龙威,王宏一. 熔融沉积成型技术3D打印机加热系统的模糊自适应PID控制[J]. 吉林大学学报(工学版), 2020, 50(1): 77-83. |
| [12] | 毛艳,成凯. 基于Hopfield神经网络的单缸插销式伸缩臂伸缩路径优化[J]. 吉林大学学报(工学版), 2020, 50(1): 53-65. |
| [13] | 周柚,杨森,李大琳,吴春国,王岩,王康平. 基于现场可编程门电路的人脸检测识别加速平台[J]. 吉林大学学报(工学版), 2019, 49(6): 2051-2057. |
| [14] | 赵宏伟,王鹏,范丽丽,胡黄水,刘萍萍. 相似性保持实例检索方法[J]. 吉林大学学报(工学版), 2019, 49(6): 2045-2050. |
| [15] | 李杰, 郭文翠, 赵旗, 谷盛丰. 基于车辆响应的路面不平度识别方法[J]. 吉林大学学报(工学版), 2019, 49(6): 1810-1817. |
|
||