吉林大学学报(工学版) ›› 2023, Vol. 53 ›› Issue (7): 1902-1910.doi: 10.13229/j.cnki.jdxbgxb.20210957
• 车辆工程·机械工程 • 上一篇
考虑偏转的斜齿轮啮合刚度及其振动分析
- 1.哈尔滨工业大学 机电工程学院,哈尔滨 150001
2.西安交通大学 航天航空学院,西安 710049
Meshing stiffness calculation and vibration analysis of helical gear considering deflection
Guo-hui CHEN1( ),Ye-yin XU2,Ying-hou JIAO1(
),Ye-yin XU2,Ying-hou JIAO1( )
)
- 1.School of Mechatronics Engineering,Harbin Institute of Technology,Harbin 150001,China
2.School of Aerospace Engineering,Xi′an Jiaotong University,Xi′an 710049,China
摘要:
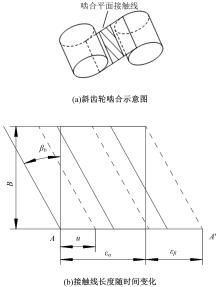
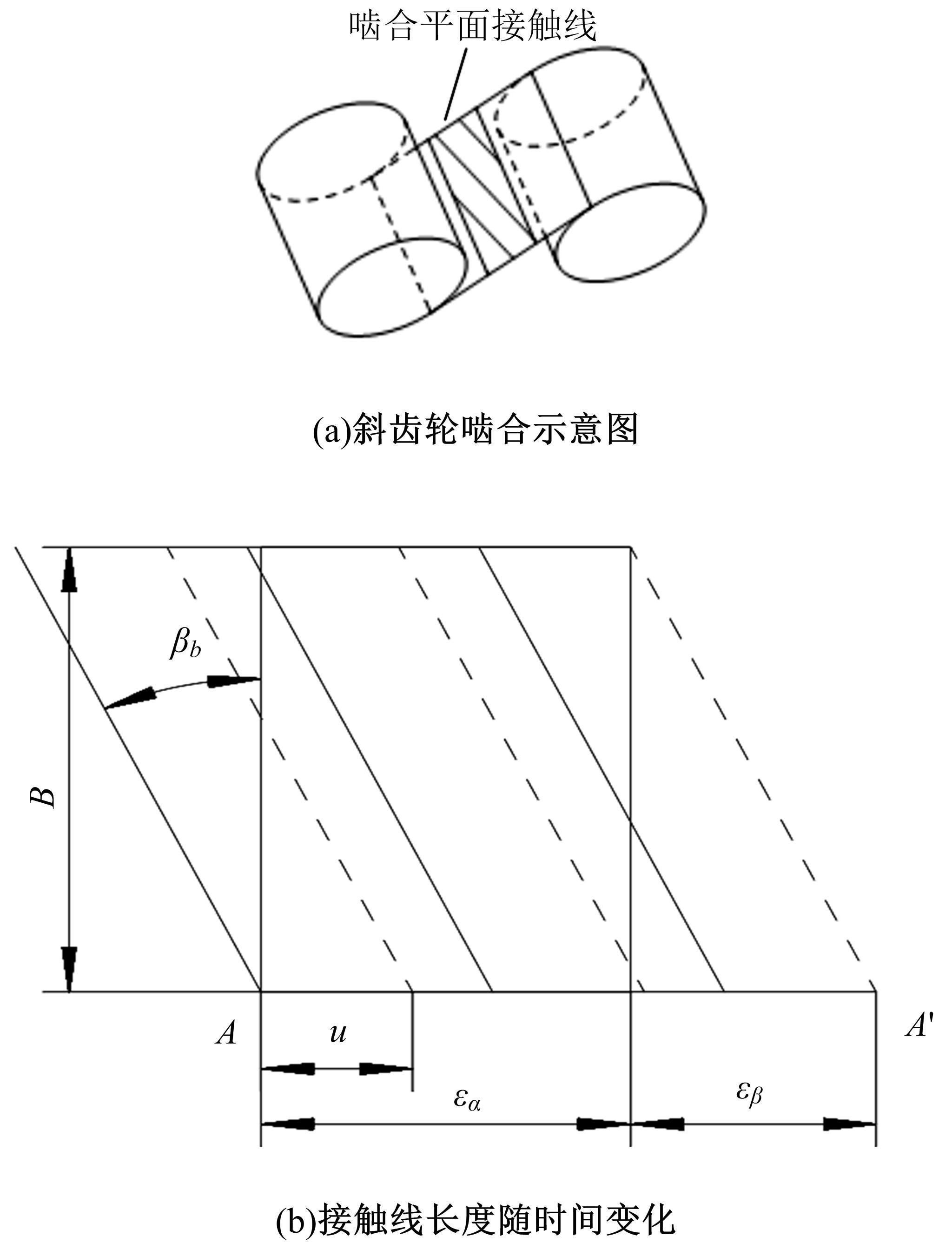
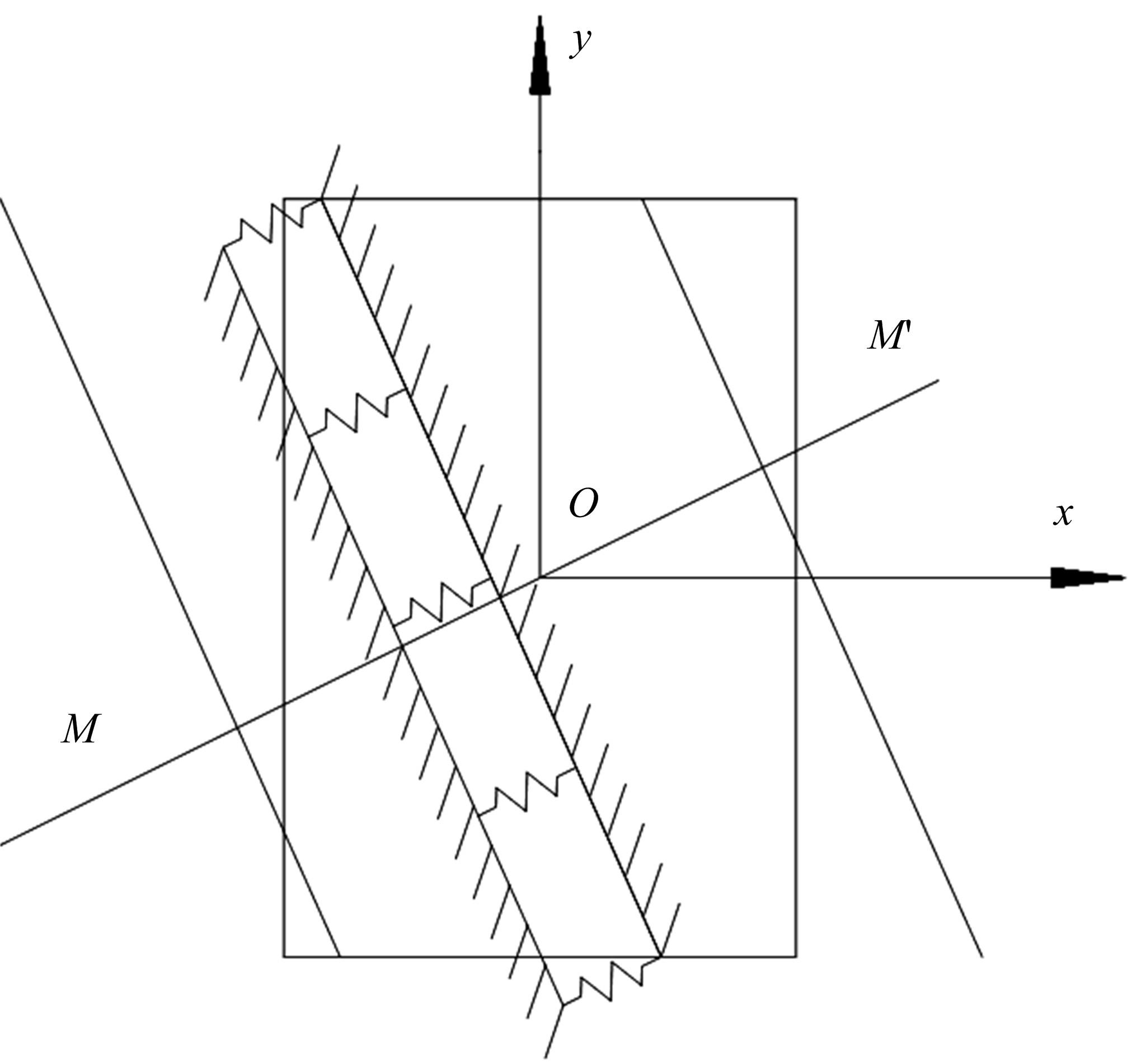
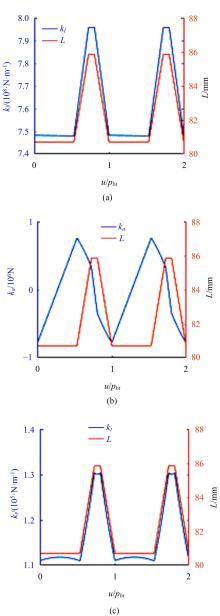
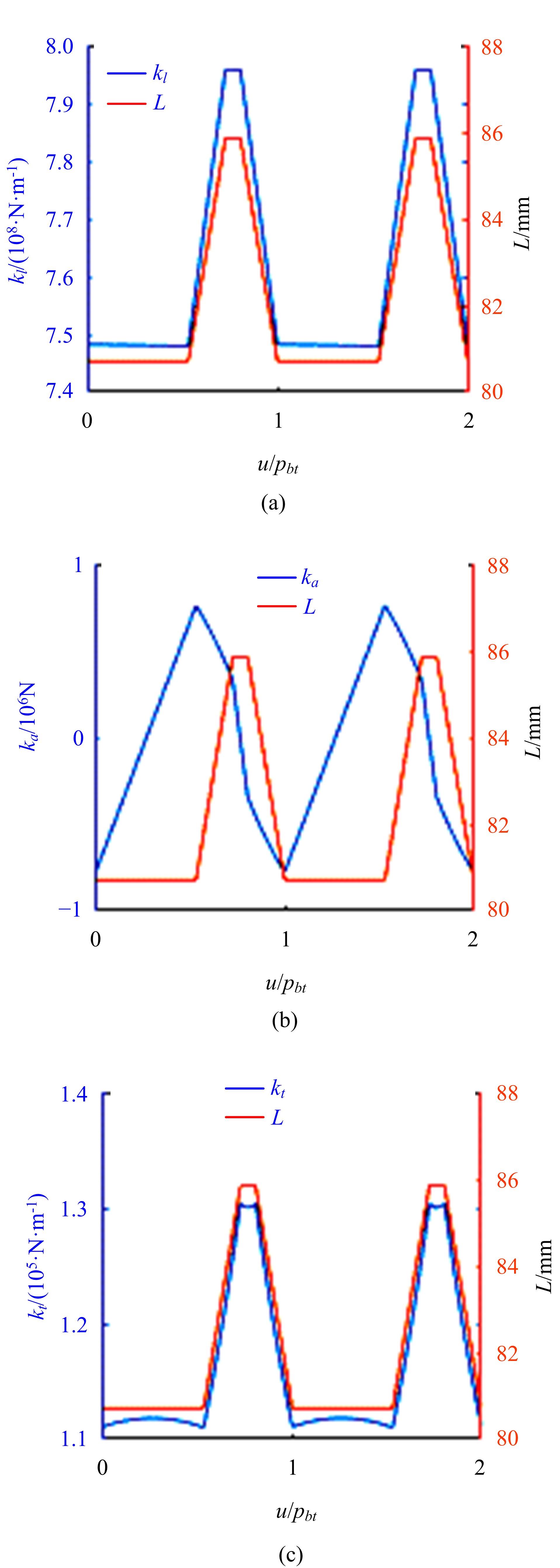
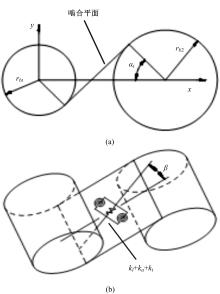
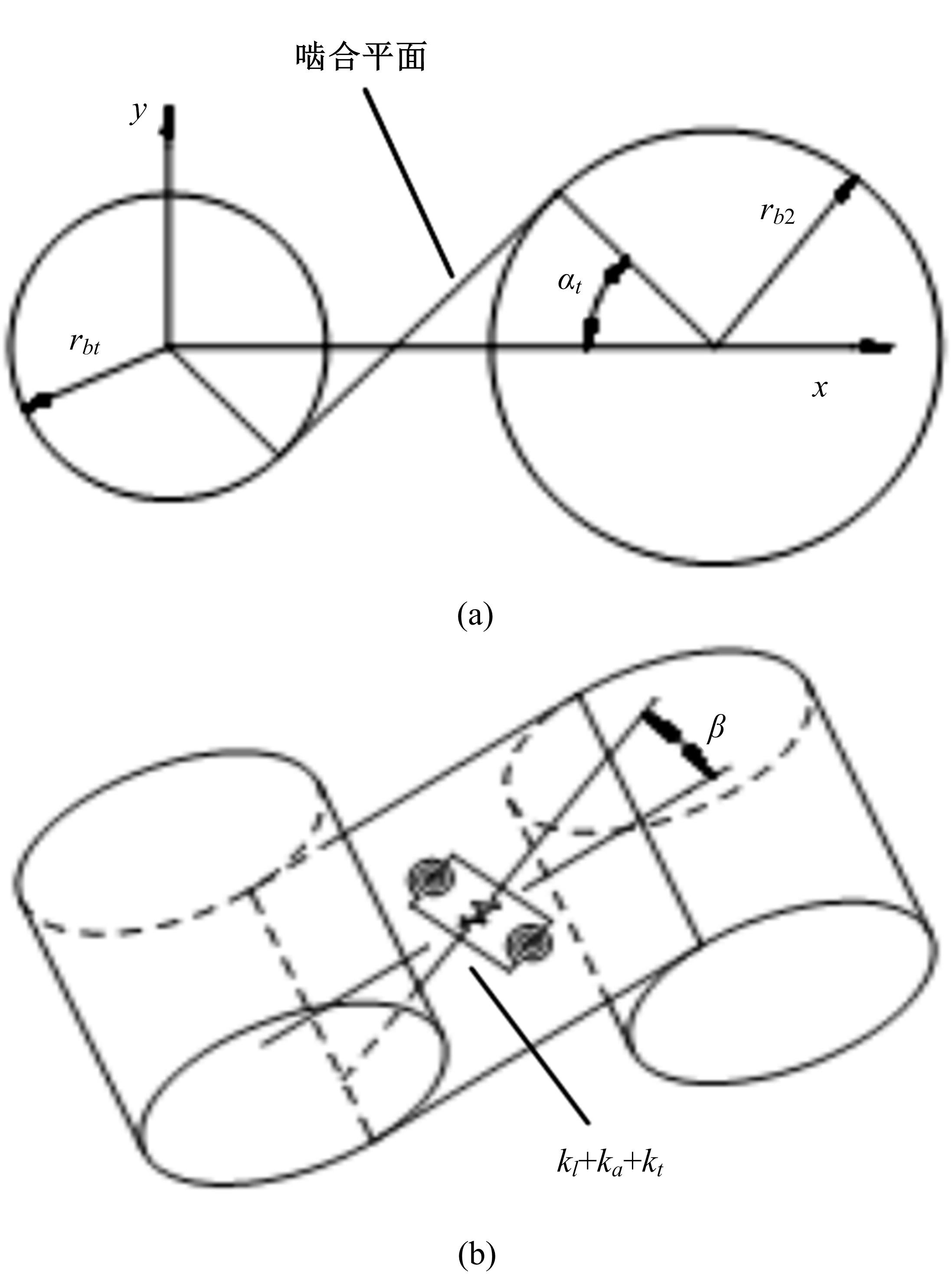
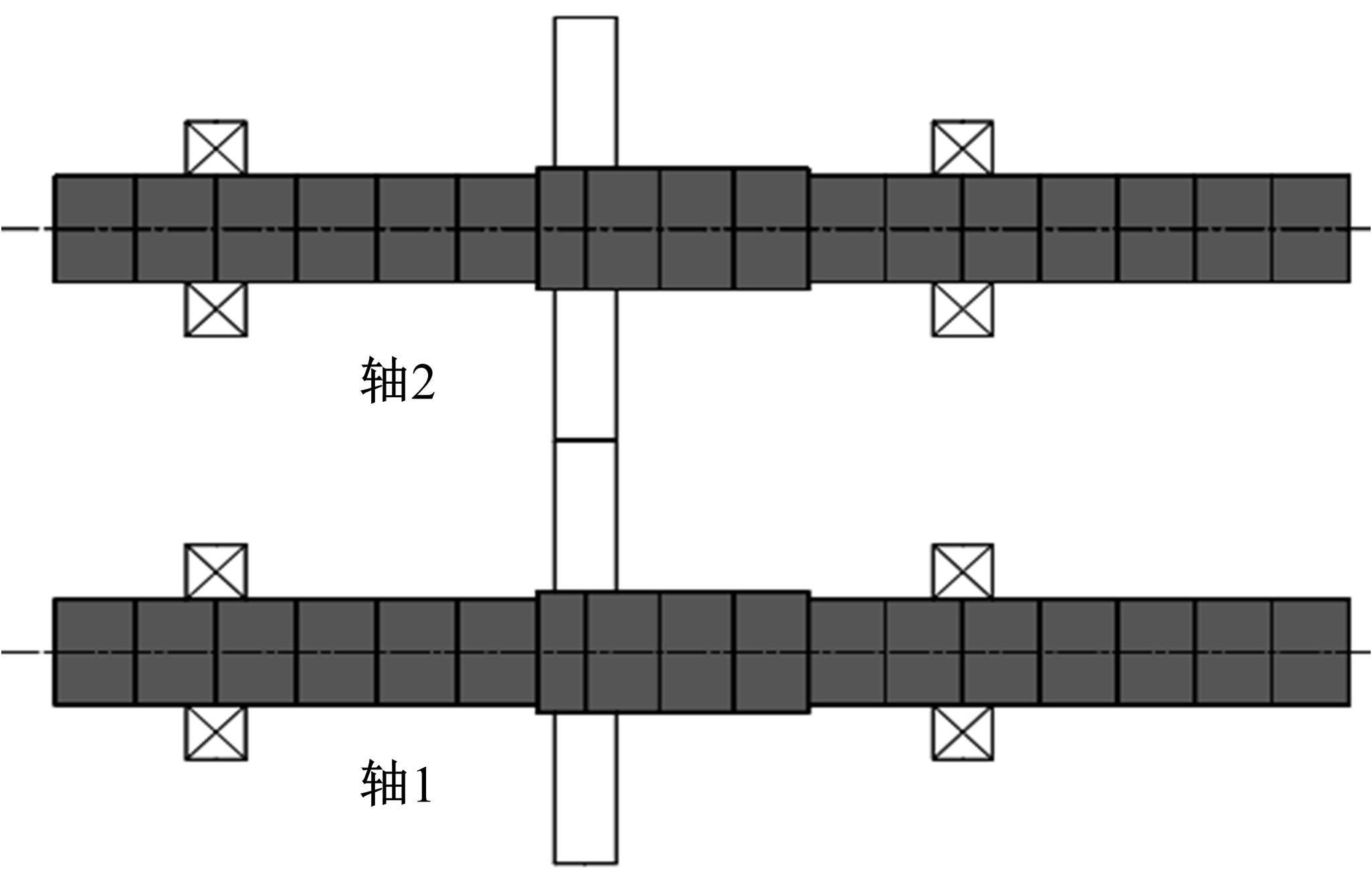
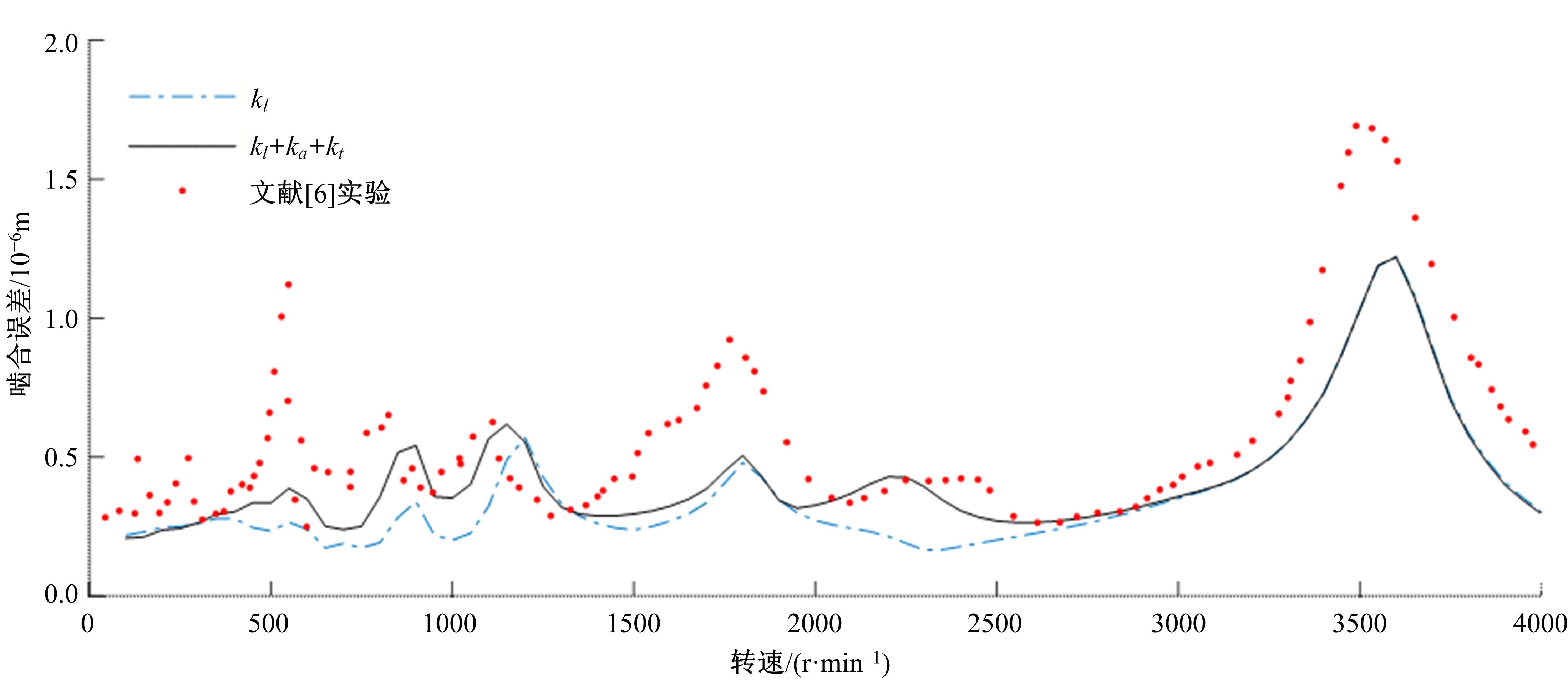
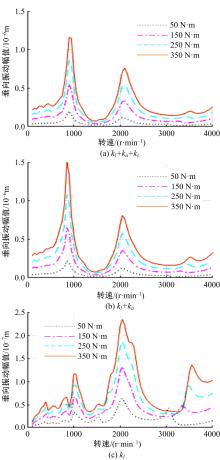
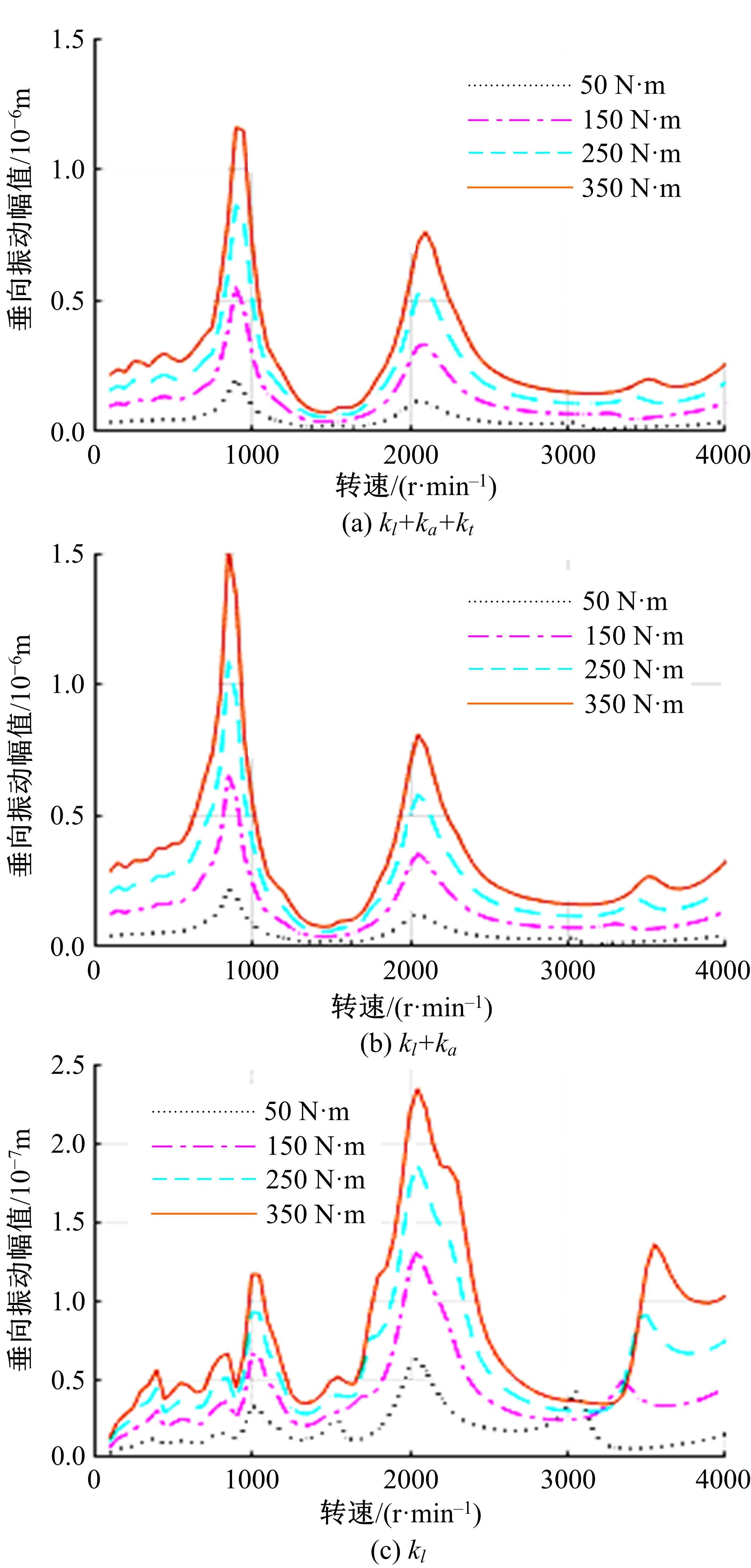
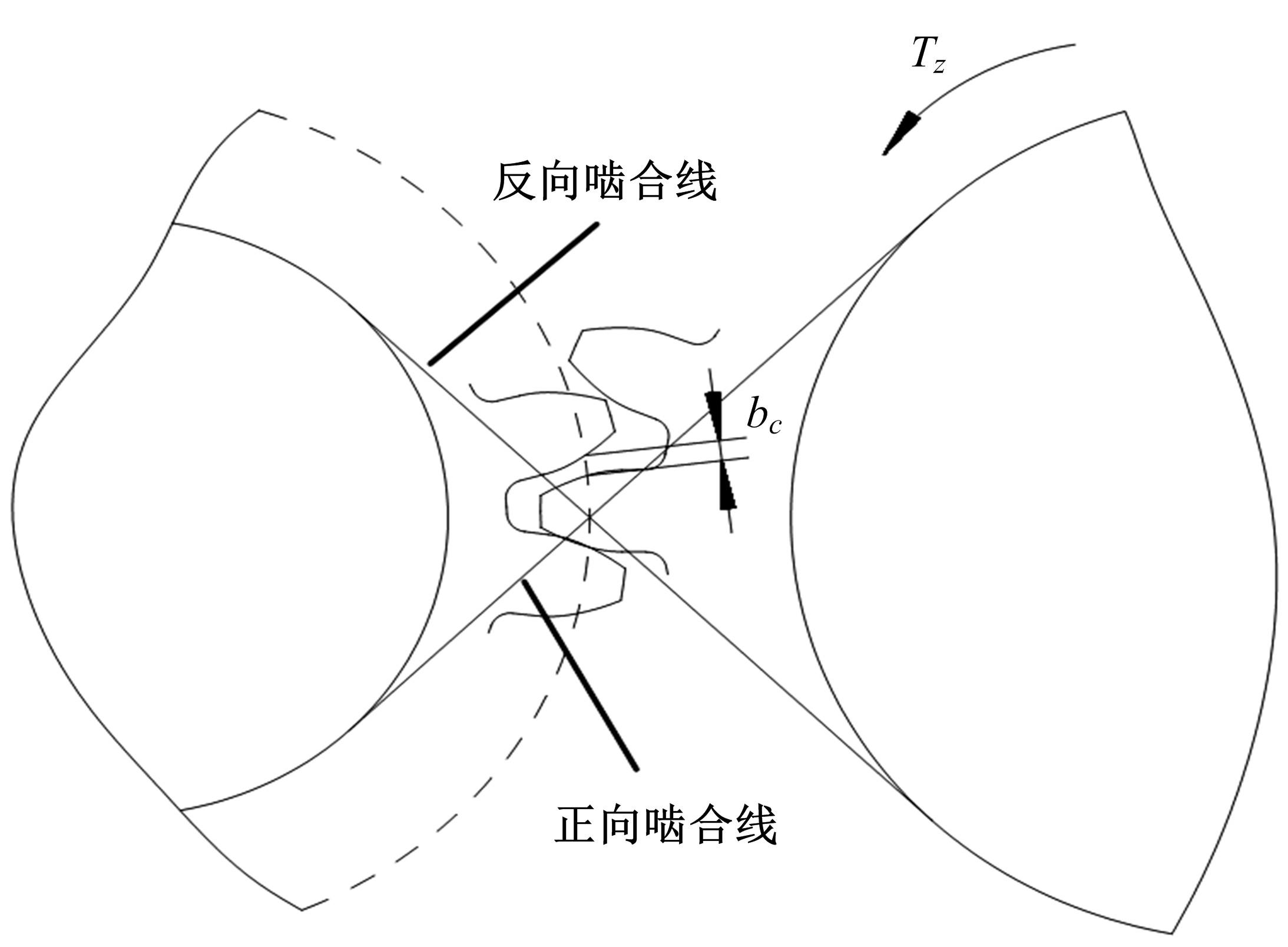
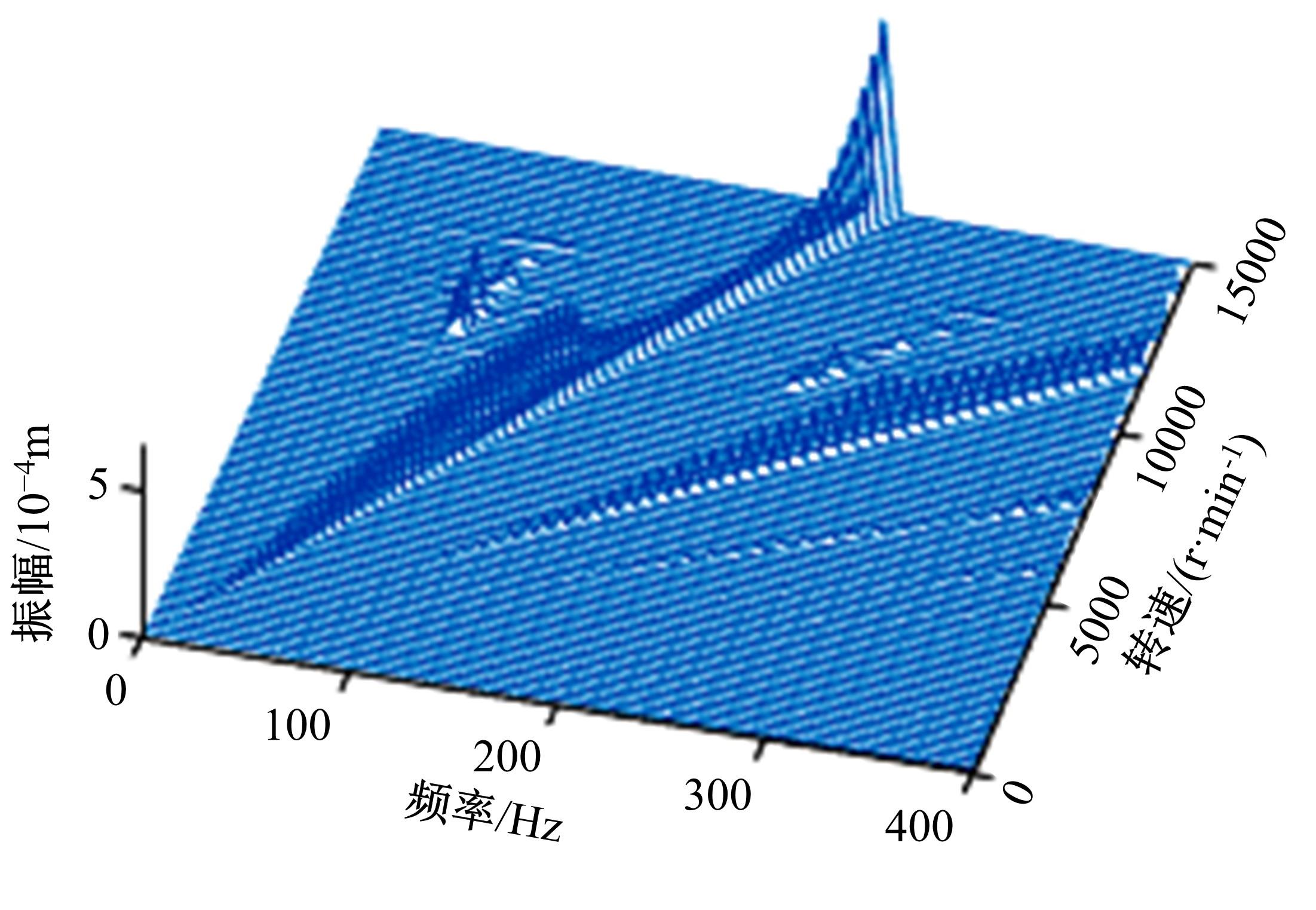
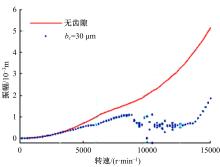
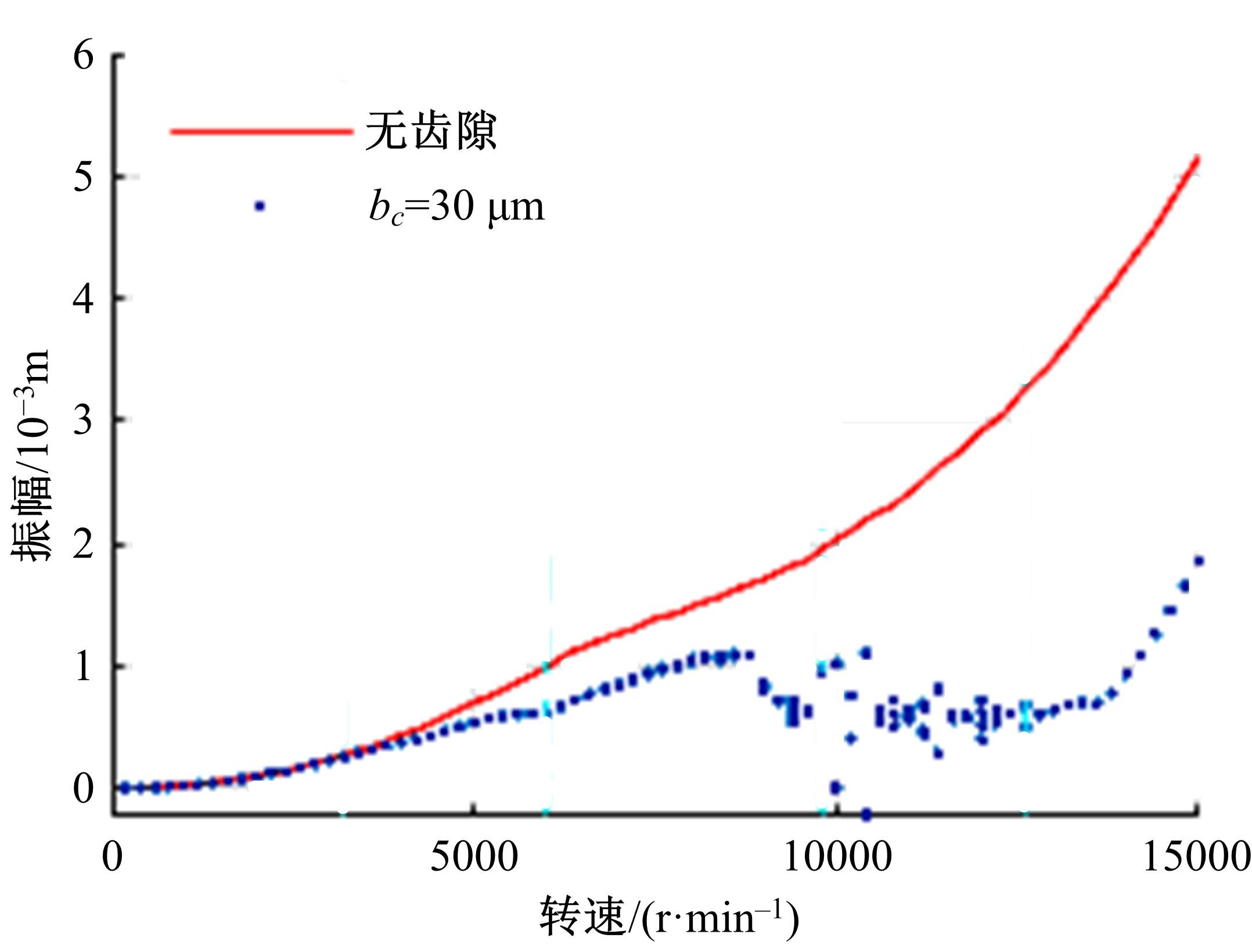
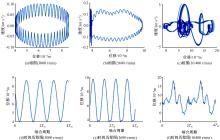
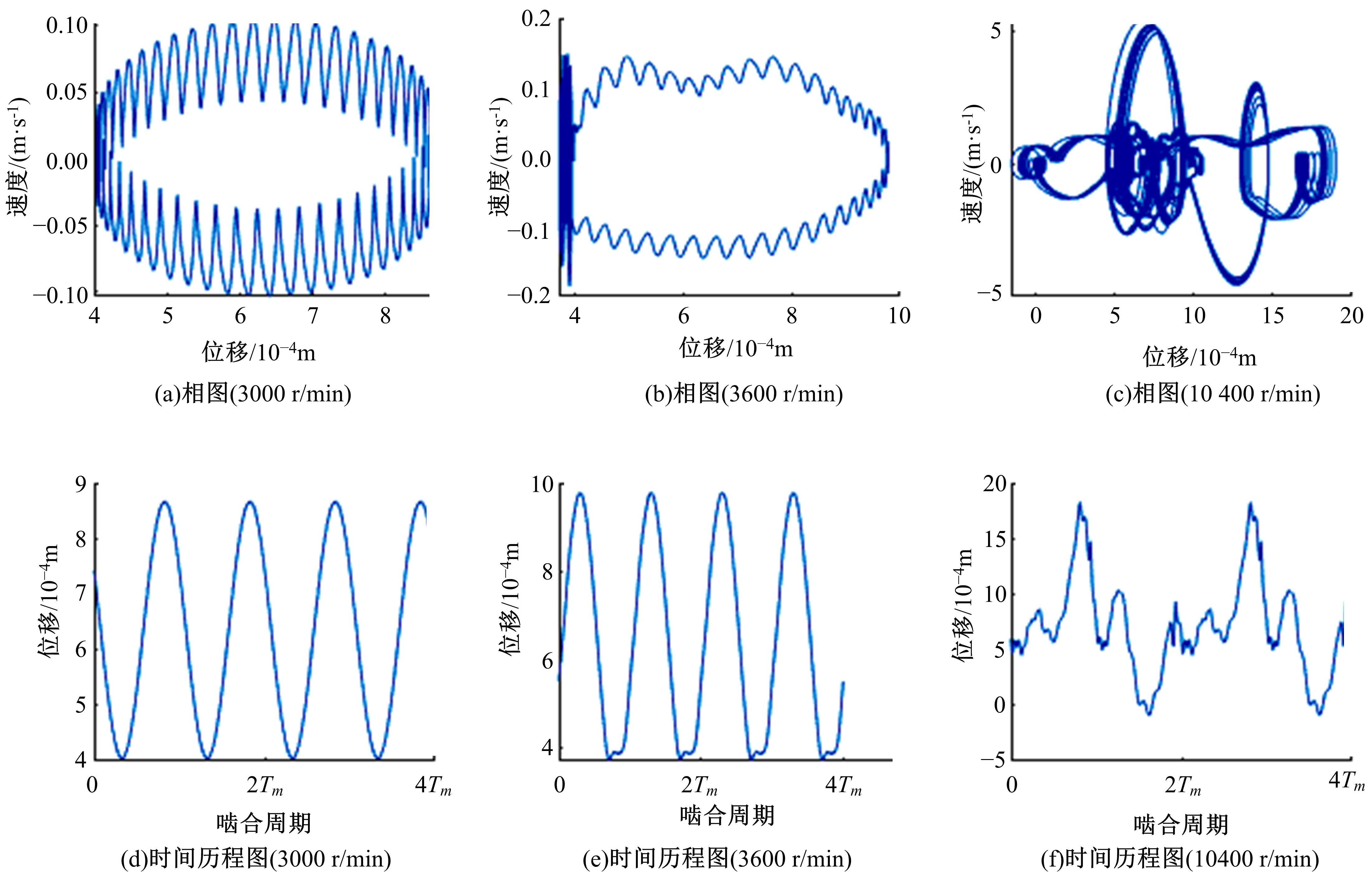
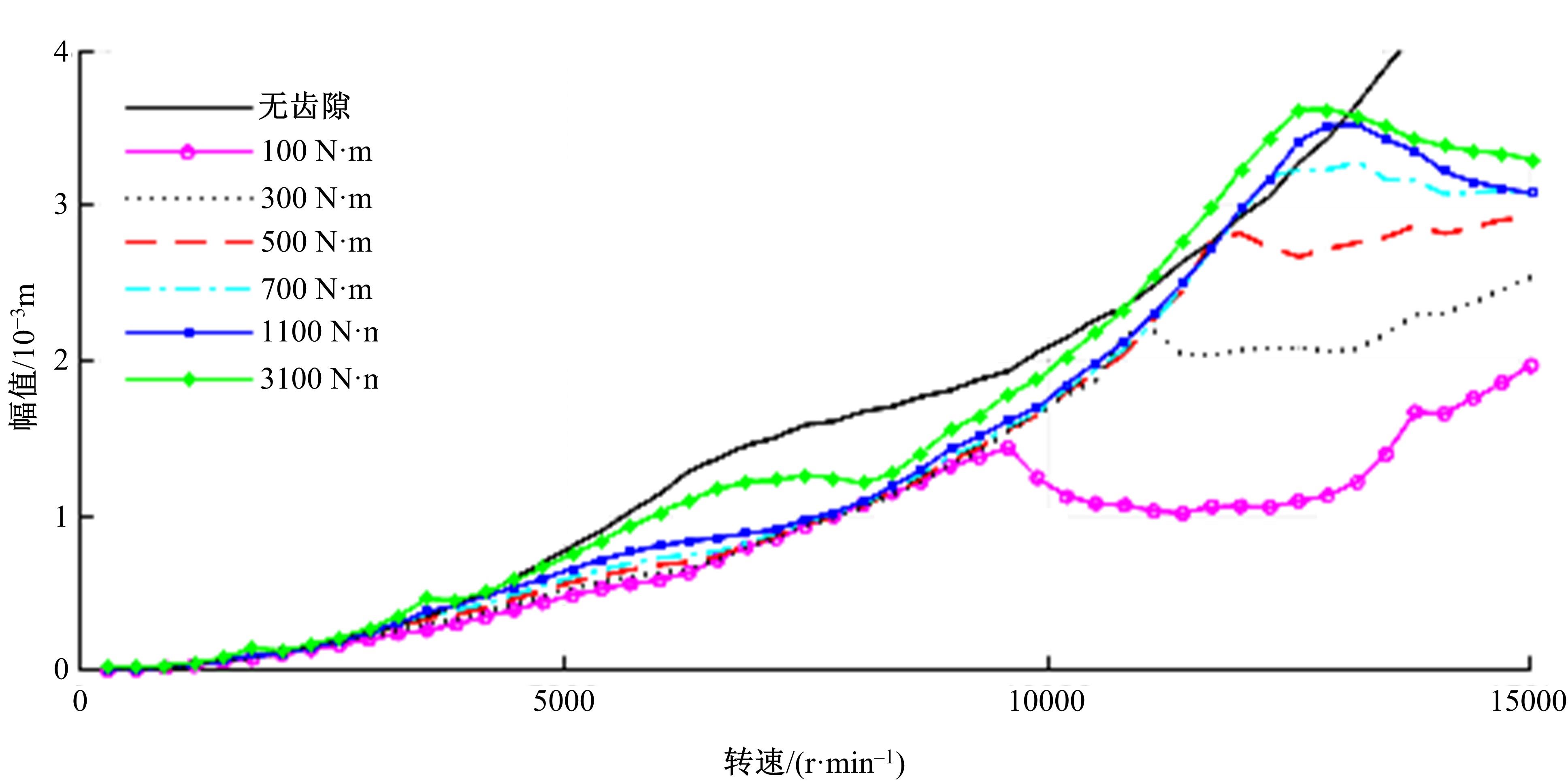
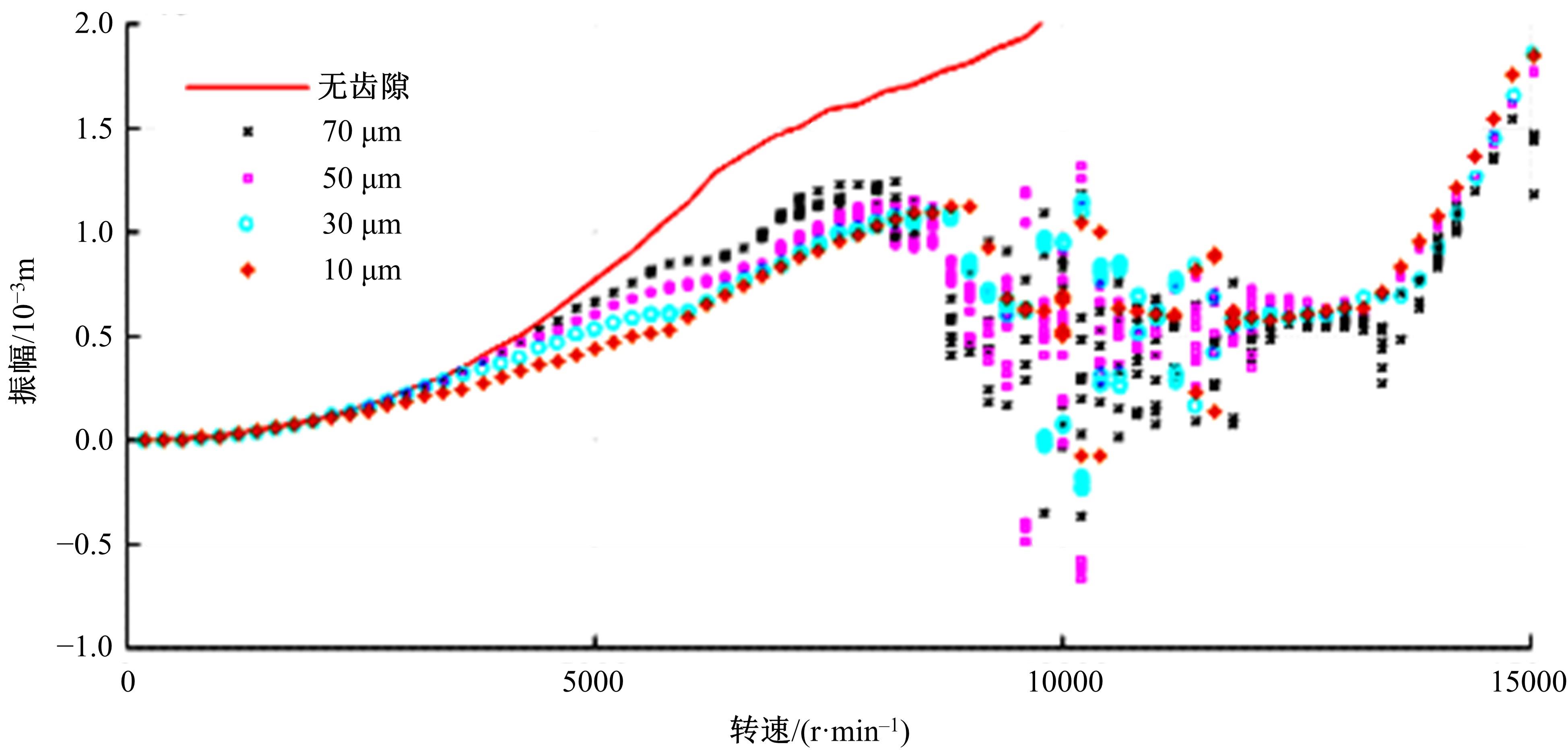
结合啮合接触线长度变化规律,根据斜齿轮啮合存在轴向偏转角时载荷沿轴向分布变化引起的啮合力矩的变化规律,推导了包含时变刚度和偏转刚度的斜齿轮啮合刚度解析表达式,并通过算例与现有实验数据对比验证。研究了不同齿宽和传递扭矩下,新啮合刚度模型的振动响应规律;进一步提出了考虑齿隙的斜齿轮啮合动力学方程,并研究了扭矩和齿隙大小的影响规律。结果显示,给出的斜齿轮啮合刚度解析公式能够较快速、准确地获取啮合刚度波动变化规律,能够很好地反映斜齿轮啮合振动;时变刚度和偏转刚度对斜齿轮传动系统的影响主要表现在具有工程意义的中低转速,随着齿宽增大其影响更明显,随着扭矩增大啮合刚度的时变引起的参数激振响应也随之增大;考虑齿隙的齿轮啮合振动,随着激励增大会经历稳定周期运动、概周期运动再回到稳定周期运动的过程。
中图分类号:
- TH113.2
| 1 | 林腾蛟, 陈梦寒, 杨金. 齿廓修形人字齿轮副时变啮合刚度计算方法[J]. 振动与冲击, 40(9):10-17. |
| Lin Teng-jiao, Chen Meng-han, Yang Jin. Calculation method of time-varying meshing stiffness of herringbone gear pair with tooth profile modification[J]. Journal of Vibration and Shock, 40(9):10-17. | |
| 2 | Wang Qi-bin, Ma Hong-bo, Kong Xian-guang, et al. A distributed dynamic mesh model of a helical gear pair with tooth profile errors[J]. Journal of Central South University, 2018, 25(2): 287-303. |
| 3 | Rincon A F D, Viadero F, Iglesias M, et al. A model for the study of meshing stiffness in spur gear transmissions[J]. Mechanism & Machine Theory, 2013, 61:30-58. |
| 4 | Gu X, Velex P, Sainsot P, et al. Analytical investigations on the mesh stiffness function of solid spur and helical gears[J]. Journal of Mechanical Design,2016,137(6):No.063301. |
| 5 | Kubur M, Kahraman A, Zini D M, et al. Dynamic analysis of a multi-shaft helical gear transmission by finite elements: model and experiment[J]. Journal of Vibration and Acoustics, 2004, 126(3): 398-406. |
| 6 | Marques P, Martins R, Seabra J. Analytical load sharing and mesh stiffness model for spur/helical and internal/external gears-towards constant mesh stiffness gear design[J]. Mechanism & Machine Theory, 2017, 113:126-140. |
| 7 | Tugan Eritenel, Parker R G. An investigation of tooth mesh nonlinearity and partial contact loss in gear pairs using a lumped-parameter model[J]. Mechanism & Machine Theory, 2012,56:28-51. |
| 8 | Margielewicz J, Gąska D, Litak G. Modelling of the gear backlash[J]. Nonlinear Dynamics, 2019, 97(1): 355-368. |
| 9 | Yang Y, Xu M, Du Y, et al. Dynamic analysis of nonlinear time-varying spur gear system subjected to multi-frequency excitation[J]. Journal of Vibration and Control, 2019, 25(6): 1210-1226. |
| [1] | 陈贵升,罗国焱,李靓雪,黄震,李一. 柴油机颗粒捕集器孔道流场及其高原环境下噪声特性分析[J]. 吉林大学学报(工学版), 2023, 53(7): 1892-1901. |
| [2] | 杨红波,史文库,陈志勇,郭年程,赵燕燕. 基于NSGA⁃II的斜齿轮宏观参数多目标优化[J]. 吉林大学学报(工学版), 2023, 53(4): 1007-1018. |
| [3] | 于立娟,安阳,何佳龙,李国发,王升旭. 机电装备载荷谱外推技术研究进展及发展趋势[J]. 吉林大学学报(工学版), 2023, 53(4): 941-953. |
| [4] | 王建,于威,王斌. 高原状态下甲醇替代率对柴油机燃烧与排放的影响[J]. 吉林大学学报(工学版), 2023, 53(4): 954-963. |
| [5] | 李胜,朱佳,黄德惠,陈存福,费洪庆,丰伟,胡兴军. 空冷中冷器百叶窗翅片结构参数优化[J]. 吉林大学学报(工学版), 2023, 53(4): 998-1006. |
| [6] | 朱凌,王秋成. 空间几何约束下新能源汽车驱动系统协调控制方法[J]. 吉林大学学报(工学版), 2022, 52(7): 1509-1514. |
| [7] | 金兆辉,谷乐祺,洪伟,解方喜,尤田. 液压可变气门系统压力波动的影响分析[J]. 吉林大学学报(工学版), 2022, 52(4): 773-780. |
| [8] | 张岩,刘玮,张树勇,裴毅强,董蒙蒙,秦静. 二/四冲程可变柴油机燃烧室热负荷的改善[J]. 吉林大学学报(工学版), 2022, 52(3): 504-514. |
| [9] | 李国发,王彦博,何佳龙,王继利. 机电装备健康状态评估研究进展及发展趋势[J]. 吉林大学学报(工学版), 2022, 52(2): 267-279. |
| [10] | 王磊,黄秉汉,丛家慧,回丽,周松,徐永臻. 超声冲击对搅拌摩擦焊缝疲劳性能的影响[J]. 吉林大学学报(工学版), 2022, 52(11): 2542-2548. |
| [11] | 赵文伯,李玉洁,邓俊,李理光,吴志军. 针阀运动规律及其对喷嘴内流和喷雾特性影响[J]. 吉林大学学报(工学版), 2022, 52(10): 2234-2243. |
| [12] | 胡兴军,张靖龙,辛俐,罗雨霏,王靖宇,余天明. 冷却管结构及风速对空冷中冷器性能的影响[J]. 吉林大学学报(工学版), 2021, 51(5): 1557-1564. |
| [13] | 赵庆武,程勇,杨雪,王宁. 高重频纳秒脉冲放电点火系统设计[J]. 吉林大学学报(工学版), 2021, 51(2): 414-421. |
| [14] | 翟富刚,尹燕斌,李超,田纬,乔子石. 伺服电动缸传动系统刚度建模与前馈控制[J]. 吉林大学学报(工学版), 2021, 51(2): 442-449. |
| [15] | 李志军,刘浩,张立鹏,李振国,邵元凯,李智洋. 过滤壁结构对颗粒捕集器深床过滤影响的模拟[J]. 吉林大学学报(工学版), 2021, 51(2): 422-434. |
|
||