基于Ease off的准双曲面齿轮多目标优化

doi:10.13229/j.cnki.jdxbgxb.20220251

摘要/Abstract

摘要：

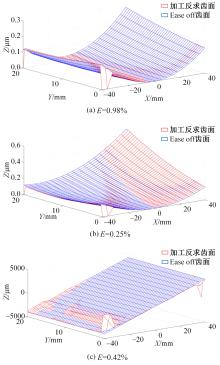
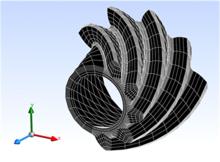
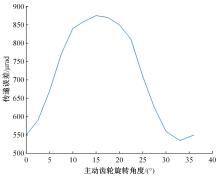
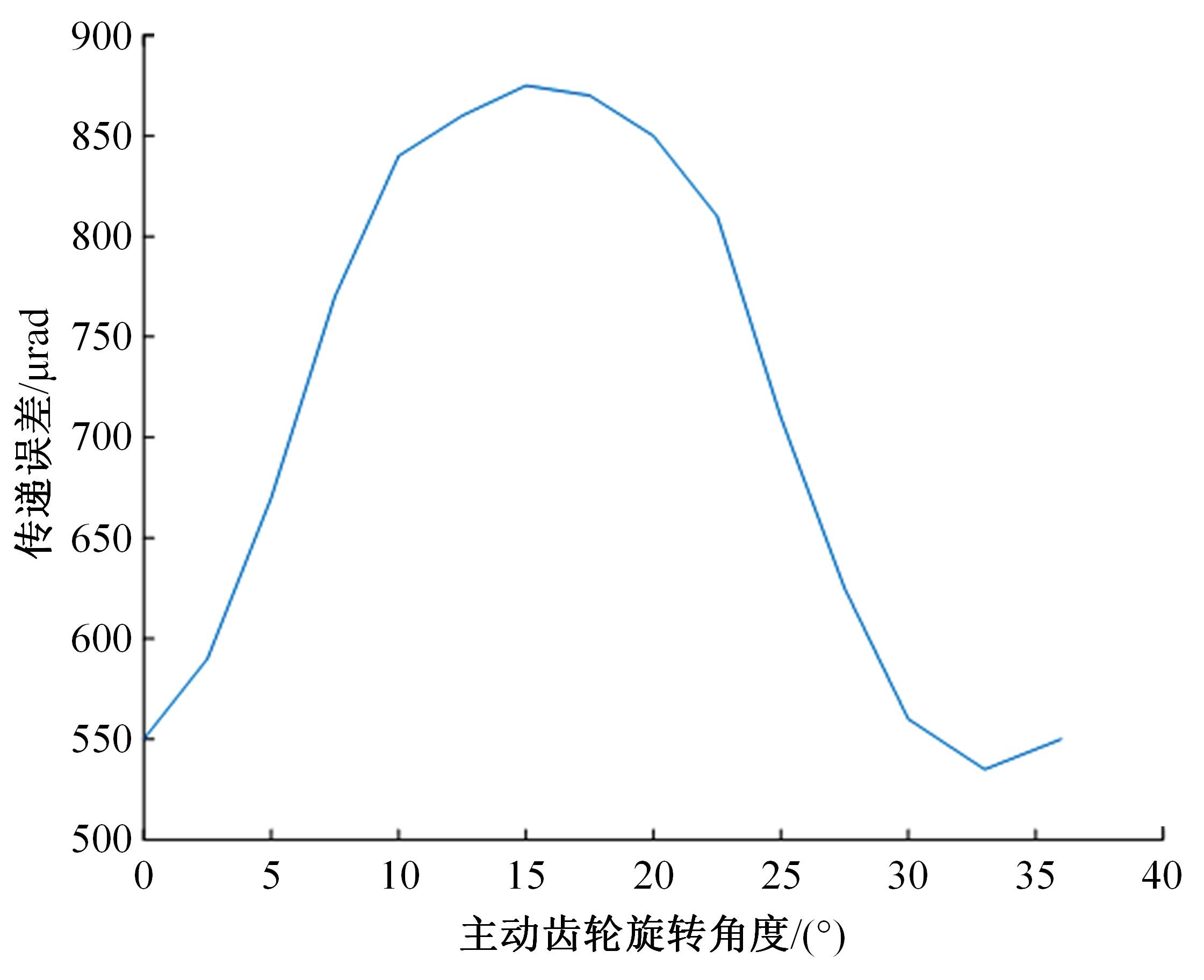
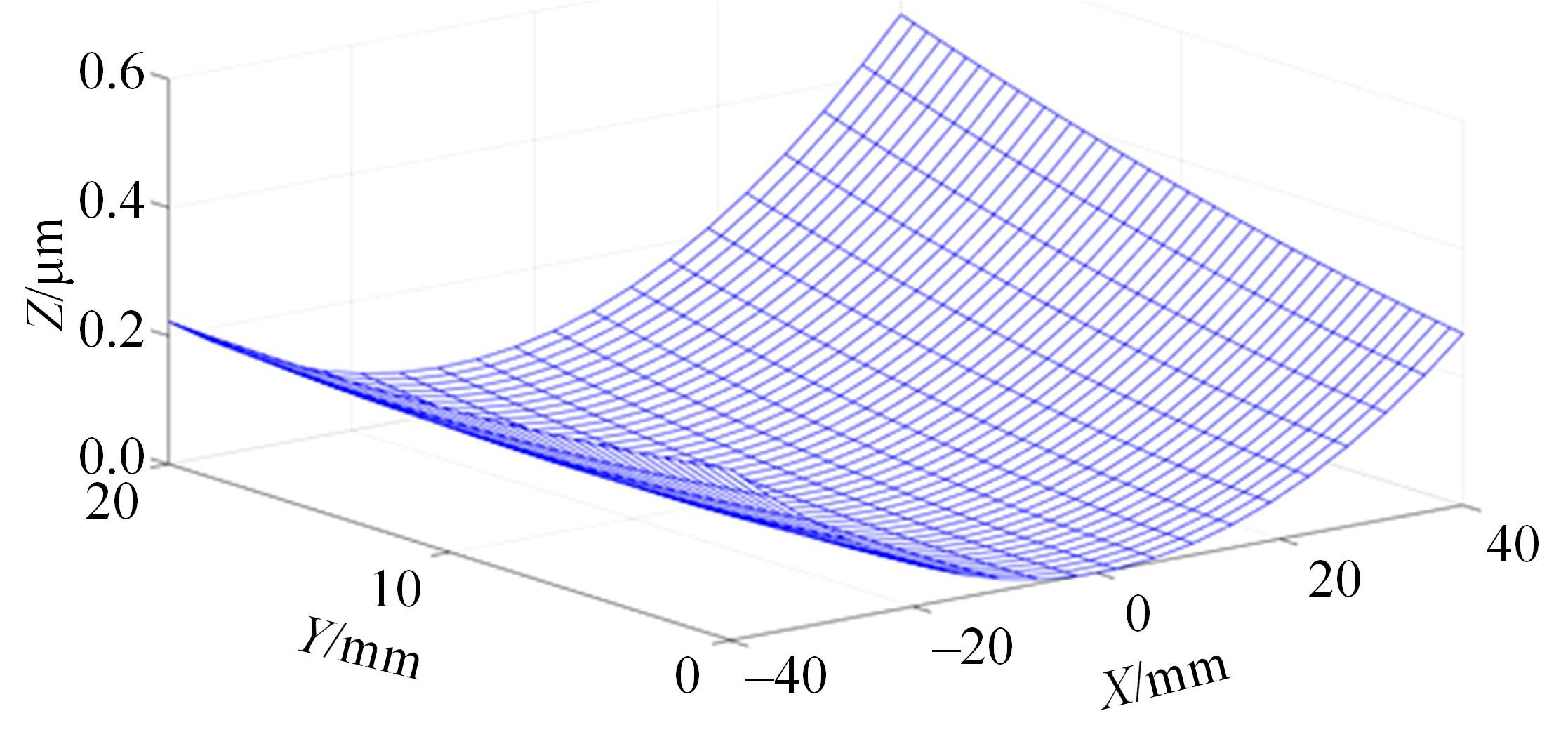
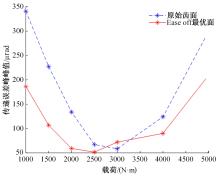
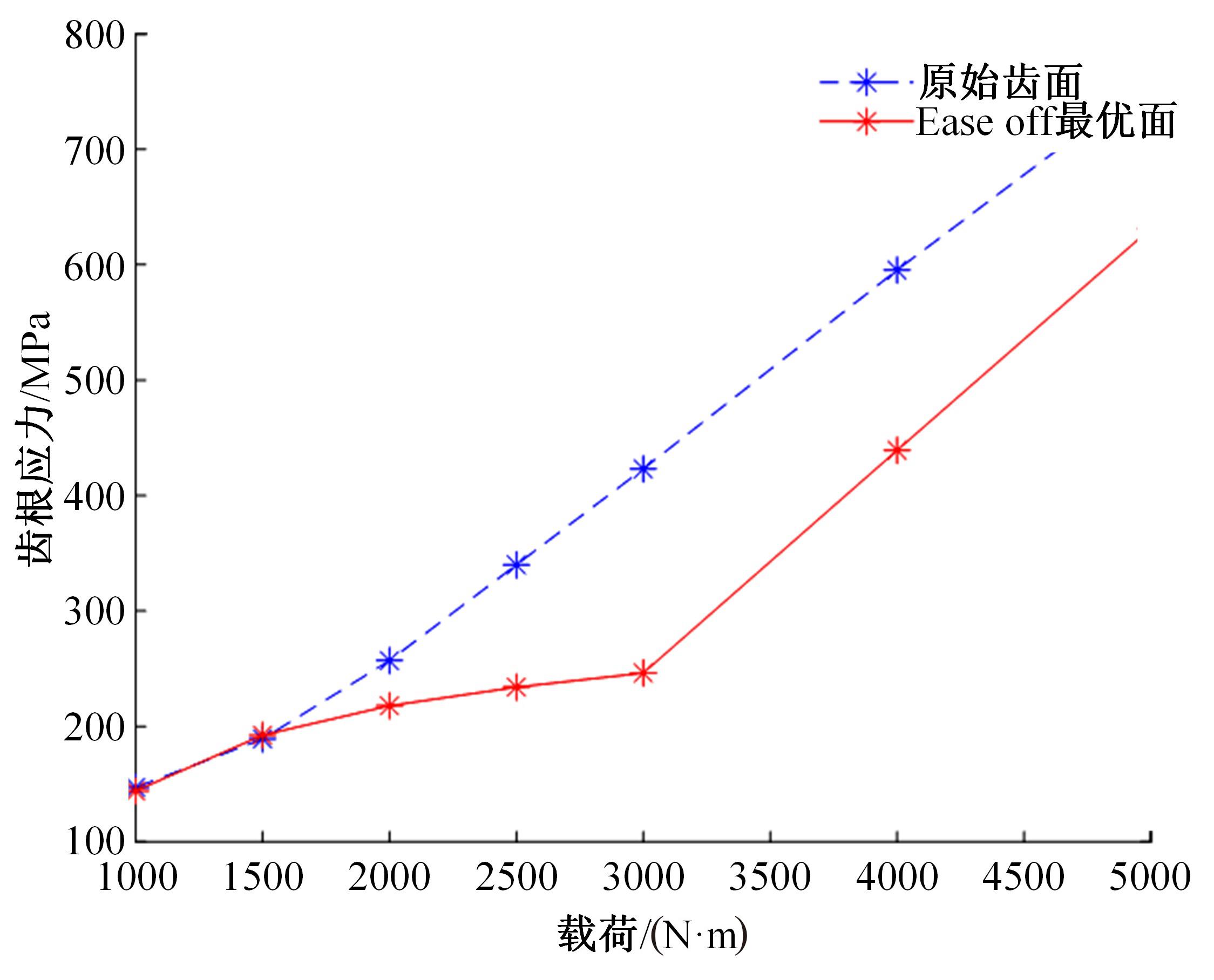
为实现准双曲面齿轮的多目标优化，建立了神经网络代理模型，用以描述Ease off修形参数和传递误差、齿根应力、啮合损失功率的关系。首先，利用动力学软件MASTA建立准双曲面齿轮驱动桥模型，基于敏感度系数矩阵，推导出齿面偏差二阶泰勒展开式对应的机床修形加工参数，建立修形齿轮模型。其次，通过MASTA的加载齿面接触分析功能计算修形齿轮模型的传递误差、齿根应力、啮合损失功率，最终建立神经网络代理模型。最后，采用NSGA-Ⅱ多目标优化算法优化代理模型，进行对比验证。结果表明：采用本多目标优化方法可有效降低准双曲面齿轮的传递误差、齿根应力、啮合损失功率。

关键词: 车辆工程, Ease off, 准双曲面齿轮, NSGA-Ⅱ, 多目标优化

Abstract:

In order to realize the multi-objective optimization of hypoid gears， a neural network surrogate model was established to describe the relationship between Ease off modification parameters and transfer error， tooth root stress and meshing loss power. Firstly， the dynamics software MASTA was used to establish the hypoid gear drive axle model. Based on the sensitivity coefficient matrix， the machine tool modification parameters corresponding to the second-order Taylor expansion of tooth surface deviation were derived， and the modified gear model was established. Secondly， the transmission error， tooth root stress and meshing loss power of the modified gear model were calculated through MASTA's loading tooth surface contact analysis function， and the neural network agent model was finally established. NSGA-II multi-objective optimization algorithm was used to optimize the surrogate model for comparative verification. The results show that the proposed multi-objective optimization method can effectively reduce transmission error， tooth root stress and meshing power loss of hypoid gears.

Key words: automotive engineering, Ease off, hypoid gear, NSGA-Ⅱ, multi-objective optimization

中图分类号:

U463.2

吴骁,史文库,郭年程,赵燕燕,陈志勇,李鑫鹏,孙卓,刘健. 基于Ease off的准双曲面齿轮多目标优化[J]. 吉林大学学报(工学版), 2024, 54(1): 76-85.

Xiao WU,Wen-ku SHI,Nian-cheng GUO,Yan-yan ZHAO,Zhi-yong CHEN,Xin-peng LI,Zhuo SUN,Jian LIU. Multi-objective optimization of hypoid gears based on Ease off[J]. Journal of Jilin University(Engineering and Technology Edition), 2024, 54(1): 76-85.

图/表 28

图1

图2

图3

表1

表2

图4

图5

图6

表3

图7

图8

图9

图10

图11

表4

1000 N·m传递误差峰峰值结果"

参数

数值

主动齿轮载荷/（N·m）

被动齿轮载荷/（N·m）

主动齿轮峰峰值误差/ $μ r a d$

从动齿轮峰峰值误差/ $μ r a d$

1000.00

4100.00

340.8482

83.1337

表4

表5

1000 N·m最大齿根应力结果"

参数

主动齿轮

被动齿轮

最大米氏应力（拉伸）/MPa

最大米氏应力（压缩）/MPa

最大主应力（拉伸）/ $μ r a d$

最大主应力（压缩）/ $μ r a d$

147.0513

130.5012

166.4879

89.5532

91.5480

124.0980

111.8853

74.3783

表5

图12

表6

主动齿轮传动效率计算参数"

参数	单位	数值
中点螺旋角 $β m$	rad	0.61
润滑油运动粘度v	cst	50
接触面宽度 $b w$	mm	10.23
小轮中点分度圆半径 $r m 1$	mm	54.88
端面压力角 $α t m$	rad	0.39
中点锥距 $R m$	mm	197.81
外锥距 $R e$	mm	235.15
节圆半径r	mm	56.30
分锥角 $δ$	rad	0.29
齿顶高系数 $h a e$	mm	18.12
齿面角 $δ a$	rad	0.35
齿宽b	mm	79.05

表6

表7

灵敏度分析表"

项目	组合（1）	组合（2）	组合（3）	组合（4）	组合（5）
螺旋角误差系数 $a 0$	0.01	0	0	0	0
压力角误差系数 $a 1$	0	0.01	0	0	0
齿长曲率系数 $a 2$	0	0	0.001	0	0
齿廓曲率系数系数 $a 3$	0	0	0	0.01	0
齿面挠率系数 $a 4$	0	0	0	0	0.001
传递误差平均值	1602.6	499.1	1981.1	3674.4	2021.1

表7

表8

代理模型计算参数"

模型	厚度取值/mm
螺旋角误差系数 $a 0$	0，0.0002
压力角误差系数 $a 1$	0，0.0002
齿长曲率系数 $a 2$	0，0.0001，0.0002，0.0003
齿廓曲率系数系数 $a 3$	0，0.0005，0.001
齿面挠率系数 $a 4$	0，0.0001，0.0002，0.0003，0.0004，0.0005

表8

表9

模型数据验证表"

输入集/10^-5					MASTA模型计算结果			代理模型计算结果			相对误差/%
$a 0$	$a 1$	$a 2$	$a 3$	$a 4$	TE/μRad	RS/MPa	PMi/W	TE/μrad	RS/MPa	PMi/W	TE	RS	PMi
10	10	5	5	5	202.492	268.010	199.561	199.761	261.973	204.972	-1.349	-2.253	+2.712
10	10	15	15	15	248.317	378.304	213.967	240.194	384.523	207.173	-3.271	+1.644	-3.175
5	5	5	5	5	200.561	375.798	198.673	219.913	368.846	204.691	+9.659	-1.850	+3.029
5	15	25	25	25	435.081	354.052	238.767	428.970	349.637	250.111	-1.405	-1.248	+4.751
5	5	15	15	15	245.919	319.348	210.820	261.183	330.652	216.814	+6.207	+3.540	+2.843

表9

图13

表10

模型最优解及验证"

最优解集/10^-5					MASTA模型计算结果			代理模型计算结果			相对误差/%
$a 0$	$a 1$	$a 2$	$a 3$	$a 4$	TE/μrad	RS/MPa	PMi/W	TE/μrad	RS/MPa	PMi/W	TE	RS	PMi
0	0	16.7	0	38.9	112.681	292.985	185.378	107.947	297.218	182.961	-4.201	+1.445	-1.304
0	0	16.7	22.2	16.7	107.935	300.674	183.095	115.236	289.028	179.898	+6.764	-3.873	-1.746
0	4.4	16.7	22.2	16.7	121.356	245.574	190.279	115.263	261.931	185.196	-5.021	+6.661	-2.671
4.4	20	16.7	0	33.3	119.601	253.077	189.586	111.027	259.501	182.787	-7.169	+2.538	-3.586
2.2	0	16.7	11.1	33.3	151.667	275.755	192.056	145.458	288.334	193.534	-4.094	+4.562	+0.770

表10

图14

图15

图16

图17

表11

参考文献 15

1	Park Nogill. A generalized hypoid gear synthesized with common crown rack positioned between pinion and gear blanks[J]. Journal of Mechanical Design, 2017, 139(8): No. 085001.
2	魏冰阳, 杨建军, 仝昂鑫, 等. 基于等距Ease-off曲面的轮齿啮合仿真分析[J]. 航空动力学报, 2017, 32(5): 1259-1265.
	Wei Bing-yang, Yang Jian-jun, Tong Ang-xin, et al. Simulation analysis of gear tooth meshing based on isometric ease-off surface[J]. Journal of Aerospace Power, 2017, 32(5): 1259-1265.
3	Li G, Zhu W. An active ease-off topography modification approach for hypoid pinions based on a modified error sensitivity analysis method[J]. Journal of Mechanical Design, 2019, 141(9): 1-10.
4	Wang Q, Zhou C, Gui L, et al. An ease-off based approach to designing a high-order transmission motion for face-milled spiral bevel gears[J]. International Journal of Vehicle Design, 2019, 81(3/4): 241-252.
5	Shih Y P. A novel ease-off flank modification methodology for spiral bevel and hypoid gears[J]. Mechanism & Machine Theory, 2010, 45(8): 1108-1124.
6	Peng X L, Zhang L, Fang Z D. Manufacturing process for a face gear drive with local bearing contact and controllable unloaded meshing performance based on ease-off surface modification[J]. Journal of Mechanical Design, 2016, 138(4): No.043302.
7	Fan Q. Ease-Off and Application in Tooth Contact Analysis for Face-Milled and Face-Hobbed Spiral Bevel and Hypoid Gears[M]. Berlin: Springer International Publishing, 2016.
8	聂少武, 唐思成, 黄菊花, 等. 基于刀倾法的弧齿锥齿轮齿面失配啮合分析[J]. 机械传动, 2019, 43(9): 96-102.
	Nie Shao-wu, Tang Si-cheng, Huang Ju-hua, et al. Analysis of tooth surface mismatch meshing of spiral bevel gear based on cutter tilt method[J]. Mechanical Transmission, 2019, 43(9): 96-102.
9	聂少武, 邓静, 邓效忠, 等. 基于齿面Ease off拓扑的弧齿锥齿轮齿面偏差等效修正方法[J]. 中国机械工程, 2017, 28(20): 2434-2440.
	Nie Shao-wu, Deng Jing, Deng Xiao-zhong, et al. Equivalent correction method for tooth surface deviation of spiral bevel gears based on ease off topology[J]. China Mechanical Engineering, 2017, 28(20): 2434-2440.
10	曹雪梅, 邓效忠, 聂少武. 基于共轭齿面修正的航空弧齿锥齿轮高阶传动误差齿面拓扑结构设计[J]. 航空动力学报, 2015, 30(1): 195-200.
	Cao Xue-mei, Deng Xiao-zhong, Nie Shao-wu. Topological structure design of aeronautical spiral bevel gear high order transmission error based on conjugate tooth surface modification[J]. Journal of Aerospace Power, 2015, 30(1): 195-200.
11	聂少武, 邓静, 邓效忠, 等. 弧齿锥齿轮齿面拓扑修形及加工参数计算[J]. 航空动力学报, 2017, 32(08): 2009-2016.
	Nie Shao-wu, Deng Jing, Deng Xiao-zhong, et al. Topological modification and machining parameters calculation of spiral bevel gears[J]. Journal of Aerospace Power, 2017, 32(8): 2009-2016.
12	聂少武, 蒋闯, 邓效忠, 等. Ease-off拓扑修正的准双曲面齿轮齿面修形方法[J]. 中国机械工程, 2019, 30(22): 2709-2715, 2740.
	Nie Shao-wu, Jiang Chuang, Deng Xiao-zhong, et al. Tooth surface modification method for hypoid gears with ease-off topology modification[J]. China Mechanical Engineering, 2019, 30(22): 2709-2715, 2740.
13	Cinelli C, Hazlett C. Making sense of sensitivity: extending omitted variable bias[J]. Journal of the Royal Statistical Society Series B, 2020, 82(1): 39-67.
14	Deb K, Pratap A, Agarwal S, et al. A fast and elitist multi-objective genetic algorithm: NSGA-II[J]. IEEE Transactions on Evolutionary Computation, 2002, 6(2): 182-197.
15	Srinivas N, Deb K. Multi-objective function optimization using non-dominated sorting genetic algorithms[J]. Evolutionary Computation, 1994, 2(3): 1301-1308.

相关文章 15

[1]	李旭东,王新宇,田程,张新峰,牛治慧,赵志强. 基于用户关联的车辆耐久性载荷谱编制[J]. 吉林大学学报(工学版), 2024, 54(1): 66-75.
[2]	陈兆玮,蒲前华. 弹性车轮对大跨斜拉桥车桥耦合振动的抑制特性[J]. 吉林大学学报(工学版), 2023, 53(9): 2519-2532.
[3]	刘平义,李晓婷,高偌霖,李海涛,魏文军,王亚. 车辆侧倾驱动机构设计与试验[J]. 吉林大学学报(工学版), 2023, 53(8): 2185-2192.
[4]	黄学劲,钟锦星,路京雨,赵霁,肖伟,袁新枚. 基于用户画像的电动汽车充电负荷预测方法[J]. 吉林大学学报(工学版), 2023, 53(8): 2193-2200.
[5]	周晓,梁燚杰,奚中轩,王宇涛. 白车身B柱焊接变形模拟及预变形控制方法[J]. 吉林大学学报(工学版), 2023, 53(8): 2212-2218.
[6]	张树培,夏明悦,张玮,陈钊,陈义祥. 考虑非线性刚度的间隙球铰碰撞动力学建模与仿真[J]. 吉林大学学报(工学版), 2023, 53(8): 2227-2235.
[7]	陈辉,邵亚军. 基于惯性基准多传感器耦合的路面谱测量方法[J]. 吉林大学学报(工学版), 2023, 53(8): 2254-2262.
[8]	陈磊,王杨,董志圣,宋亚奇. 一种基于转向意图的车辆敏捷性控制策略[J]. 吉林大学学报(工学版), 2023, 53(5): 1257-1263.
[9]	陈鑫,张冠宸,赵康明,王佳宁,杨立飞,司徒德蓉. 搭接焊缝对铝合金焊接结构轻量化设计的影响[J]. 吉林大学学报(工学版), 2023, 53(5): 1282-1288.
[10]	张勇,毛凤朝,刘水长,王青妤,潘神功,曾广胜. 基于Laplacian算法的汽车外流场畸变网格优化[J]. 吉林大学学报(工学版), 2023, 53(5): 1289-1296.
[11]	汪少华,储堃,施德华,殷春芳,李春. 基于有限时间扩张状态观测的HEV鲁棒复合协调控制[J]. 吉林大学学报(工学版), 2023, 53(5): 1272-1281.
[12]	尹燕莉,黄学江,潘小亮,王利团,詹森,张鑫新. 基于PID与Q⁃Learning的混合动力汽车队列分层控制[J]. 吉林大学学报(工学版), 2023, 53(5): 1481-1489.
[13]	于贵申,陈鑫,武子涛,陈轶雄,张冠宸. AA6061⁃T6铝薄板无针搅拌摩擦点焊接头结构及性能分析[J]. 吉林大学学报(工学版), 2023, 53(5): 1338-1344.
[14]	田彦涛,黄兴,卢辉遒,王凯歌,许富强. 基于注意力与深度交互的周车多模态行为轨迹预测[J]. 吉林大学学报(工学版), 2023, 53(5): 1474-1480.
[15]	杨红波,史文库,陈志勇,郭年程,赵燕燕. 基于NSGA⁃II的斜齿轮宏观参数多目标优化[J]. 吉林大学学报(工学版), 2023, 53(4): 1007-1018.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

参数	数值
主减速比	41
桥壳网格单元最大尺寸/mm	10
桥壳网格单元最小尺寸/mm	4
桥壳网格单元坍塌比最小值	0.2
桥壳网格单元总数	3 071 501
桥壳密度/（kg·m^-3）	7 850
桥壳弹性模量/MPa	206 800
桥壳泊松比	0.29
轴系密度/（kg·m^-3）	7 850
轴系弹性模量/MPa	212 000
轴系泊松比	0.28
齿轮密度/（kg·m^-3）	7 800
齿轮弹性模量/MPa	207 000
齿轮泊松比	0.29

参数/单位	主动齿轮	被动齿轮
齿数	10	41
旋向	左	右
轴夹角/（°）	90	90
节圆模数/mm	10.98	10.98
中点螺旋角/（°）	35	35
平均压力角/（°）	22.50	22.50
齿宽/mm	79.02	72.00
全齿高/mm	20.45	20.69
齿顶高/mm	18.12	18.35
平均节圆直径/mm	112.26	380.90
节锥角/（°）	16.48	73.10
面锥角/（°）	20.24	73.89
根锥角/（°）	15.71	69.36

参数	主动齿轮	被动齿轮
网格大小/mm	5.95	27.14
轮廓网格密度	4	4
表面网格数	8	8
圆角网格数	8	8
径向网格数	4	4

模型	TE/μrad	RS/MPa	PMi/W
性能提升百分比/%	39.600	22.395	19.964
原始齿面	178.701	387.444	228.767
Ease off最优面	107.935	300.674	183.095