剪力钉连接件拉剪共同作用抗剪性能

doi:10.13229/j.cnki.jdxbgxb.20211243

摘要/Abstract

摘要：

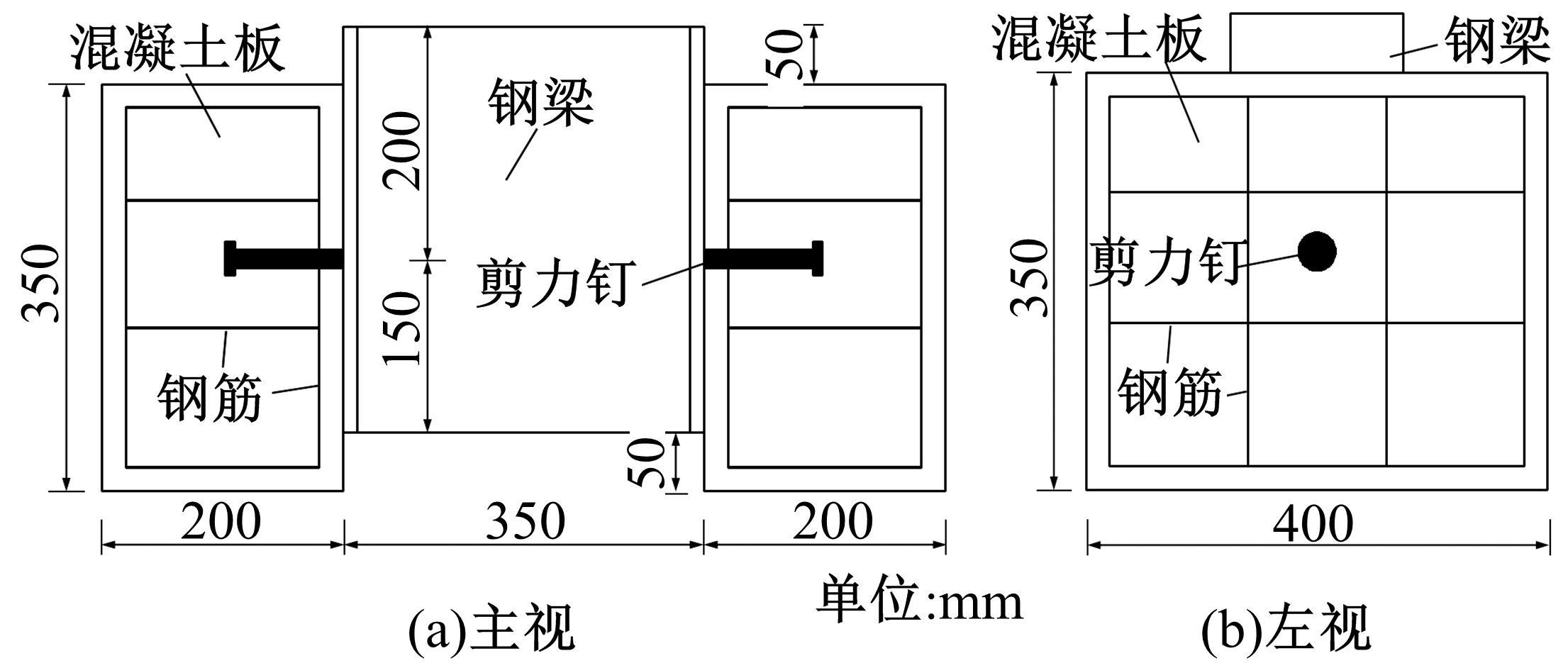
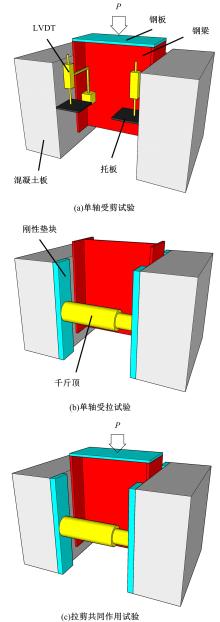
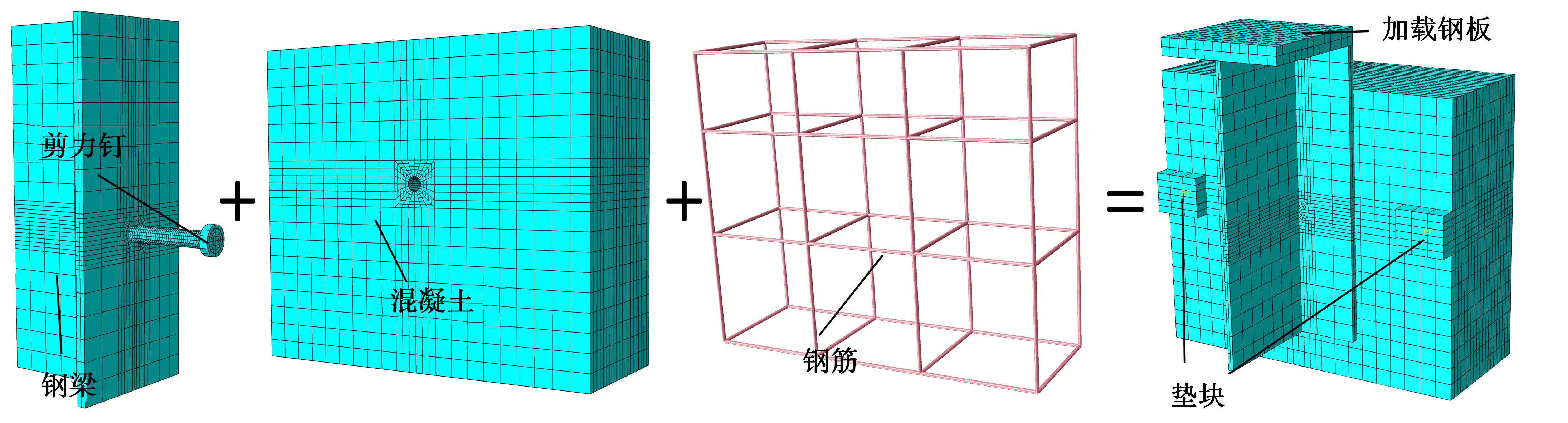
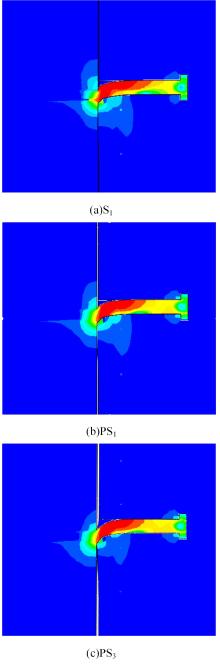
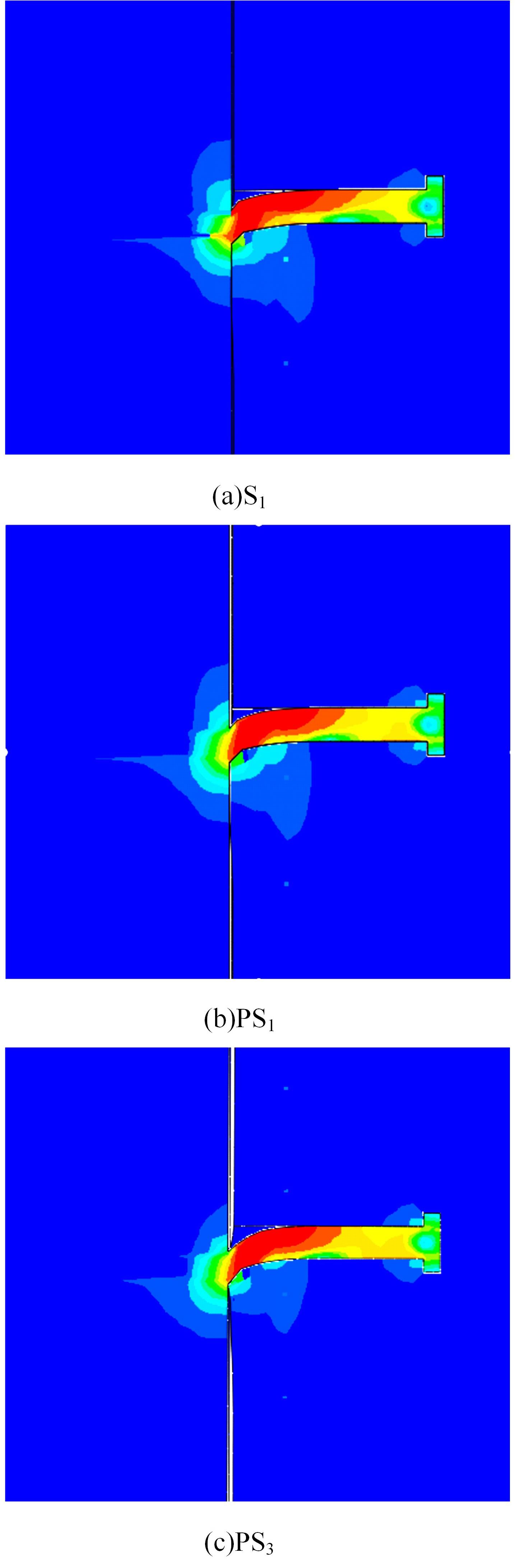
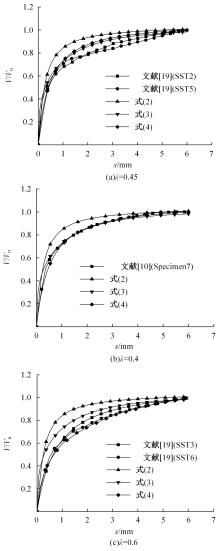
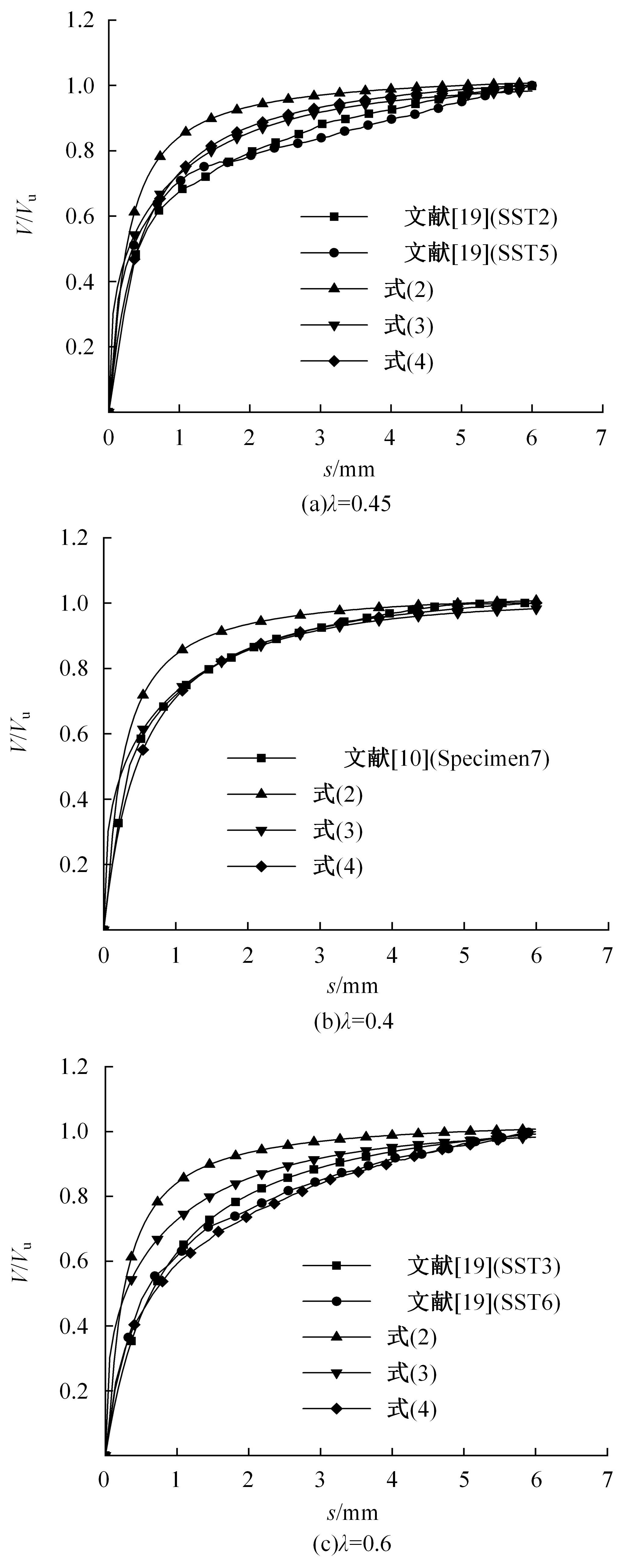
为明确剪力钉连接件在拉剪荷载作用下的力学性能，开展了5组受拉、受剪和拉剪共同作用模型试验，结合有限元模型，探讨了混凝土强度和剪力钉尺寸对抗剪承载力的影响，提出了拉剪共同作用剪力钉连接件荷载-滑移关系式和抗剪承载力计算公式。结果表明：当拉力施力比从0增到0.6时，剪切刚度下降69%，弹性极限荷载与抗剪承载力的比值从0.55下降至0.24，抗剪承载力下降31%；混凝土强度和拉力施力比对剪力钉抗剪承载力的影响具有相关性。本文提出的拉剪共同作用剪力钉连接件荷载-滑移关系式和抗剪承载力计算公式可应用于工程设计和计算。

关键词: 桥梁工程, 钢-混组合梁, 剪力钉连接件, 剪切刚度, 荷载-滑移曲线, 抗剪承载力

Abstract:

In order to understand the mechanical properties of shear stud connectors under combined tension and shear loading， five groups of model tests were tested， including uniaxial pull-out tests， uniaxial push-out tests， and combined tension and shear loading tests. Combined with finite element models， influence of concrete strength and stud size on shear capacity were analyzed， and the load-slip relationship， the ultimate shear capacity formula of shear stud connectors under tension and shear loading were proposed. The results show that as the applied tensile force ratio increase to 0.6， the shear stiffness reduces 69%； and the ratio of ultimate elastic capacity to ultimate shear capacity reduces from 0.55 to 0.24， and the ultimate shear capacity reduces 31%. The effects of concrete strength and tensile force ratio on the shear capacity of shear stud connectors are correlated. The proposed load-slip relationship and ultimate shear capacity formula under tension and shear loading can be applied to engineering design and calculation.

Key words: bridge engineering, steel concrete composite beam, shear stud connector, shear stiffness, load-slip curve, ultimate shear capacity

中图分类号:

U443.3

安然,王有志. 剪力钉连接件拉剪共同作用抗剪性能[J]. 吉林大学学报(工学版), 2023, 53(9): 2554-2562.

Ran AN,You-zhi WANG. Shear properties of shear stud connectors under combined tension and shear loading[J]. Journal of Jilin University(Engineering and Technology Edition), 2023, 53(9): 2554-2562.

图/表 13

图1

表1

图2

图3

表2

图4

图5

图6

图7

表3

图8

表4

剪力钉连接件拉剪相关关系式"

文献来源	拉剪相关关系式	公式编号
文献［14］	$V V u 53 + T T u 53 ≤ 1$	（6）
文献［19］	$V = V u, T ≤ 0.1 T u V V u + 0.5 2 + T T u + 0.5 2 ≤ 2.61, ????? T > 0.1 T u$	（7）
文献［22］	$V V u 2 + T T u 2 ≤ 1$	（8）
文献［23］	$V = V u, T ≤ 0.2 T u V V u + T T u ≤ 1.2, T > 0.2 T u$	（9）

表4

表5

参考文献 24

1	王倩, 刘玉擎. 焊钉连接件抗剪承载力试验研究[J]. 同济大学学报: 自然科学版, 2013, 41(5): 659-663.
	Wang Qian, Liu Yu-qing. Experimental study of shear stud connectors[J]. Journal of Tongji University (Natural Science Edition), 2013, 41(5): 659-663.
2	匡亚川, 陈立斌, 李超举, 等. 栓钉剪力连接件力学性能分析[J]. 吉林大学学报:工学版, 2023, 53(2): 538-546.
	Kuang Ya-chuan, Chen Li-bin, Li Chao-ju, et al. Analysis of mechanical properties of stud shear connectors[J]. Journal of Jilin University (Engineering and Technology Edition), 2023, 53(2): 538-546.
3	鞠晓臣, 赵欣欣, 刘晓光, 等. 火灾作用下剪力钉抗拔性能研究[J]. 桥梁建设, 2018, 48(2): 55-60.
	Ju Xiao-chen, Zhao Xin-xin, Liu Xiao-guang, et al. Study of pullout performance of shear stud under fire[J]. Bridge Construction, 2018, 48(2): 55-60.
4	蔺钊飞, 刘玉擎. 焊钉连接件抗拉承载力试验[J]. 同济大学学报: 自然科学版, 2015, 43(9): 1313-1319, 1331.
	Lin Zhao-fei, Liu Yu-qing. Experimental study on static behavior of headed studs under tension force[J]. Journal of Tongji University (Natural Science Edition), 2015, 43(9): 1313-1319, 1331.
5	Luis P, Jerome F. Headed steel stud anchors in composite structures,part II: tension and interaction[J]. Journal of Constructional Steel Research, 2010, 66(2): 213-228.
6	薛东焱, 宛青云, 潘志宏, 等. 剪力与拉拔力共同作用下焊钉受力性能分析[J]. 江苏科技大学学报:自然科学版, 2020, 34(2): 97-102.
	Xue Dong-yan, Wan Qing-yun, Pan Zhi-hong, et al. Mechanical behavior analysis of headed studs under shear and tension loads[J]. Journal of Jiangsu University of Science and Technology (Natural Science Edition), 2020, 34(2): 97-102.
7	刘沐宇, 赖苑林, 邓晓光, 等. 集束式长短剪力钉抗剪承载力计算方法[J]. 中国公路学报, 2018, 31(12): 97-105.
	Liu Mu-yu, Lai Yuan-lin, Deng Xiao-guang, et al. Calculation method of shear capacity of clustered stud connectors with different lengths and diameters[J]. China Journal of Highway and Transport, 2018, 31(12): 97-105.
8	张阳, 吴洁, 邵旭东, 等. 超高性能混凝土-普通混凝土界面抗剪性能试验研究[J]. 土木工程学报, 2021, 54(7): 81-89.
	Zhang Yang, Wu Jie, Shao Xu-dong, et al. Experiment on interfacial shear properties between ultra-high performance concrete and normal strength concrete[J]. China Civil Engineering Journal, 2021, 54(7): 81-89.
9	Shen M H, Chung K F. An investigation into shear resistances of headed shear studs in solid concrete slabs with local aggregates in Hong Kong[J]. Procedia Engineering, 2011: 1098-1105.
10	Saari W K, Hajjar J F, Schultz A E, et al. Behavior of shear studs in steel frames with reinforced concrete infill walls[J]. Journal of Constructional Steel Research, 2004, 60(10): 1453-1480.
11	蔺钊飞, 刘玉擎. 焊钉连接件拉剪相关关系模型试验[J]. 中国公路学报, 2015, 28(1): 80-86.
	Lin Zhao-fei, Liu Yu-qing. Model experiment on shear-tension interaction relationship of headed studs[J]. China Journal of Highway and Transport, 2015, 28(1): 80-86.
12	Shen M H, Chung K F. Experimental investigation into stud shear connections under combined shear and tension forces[J]. Journal of Constructional Steel Research, 2017, 133(6): 434-447.
13	Mirza O, Uy B. Effects of the combination of axial and shear loading on the behavior of headed stud steel anchors[J]. Engineering Structures, 2010, 32(1): 93-105.
14	Mcmackin P J, Slutter R G, Fisher J W. Headed steel anchor under combined loading[J]. AISC Engineering Journal, 1973, 2: 43-52.
15	蒲黔辉, 谢宏伟, 樊书文, 等. 拔出破坏的钢混组合结构栓钉连接件承载力的分析方法[J]. 工程科学与技术, 2019, 51(1): 89-95.
	Pu Qian-hui, Xie Hong-wei, Fan Shu-wen, et al. Analysis method of bearing capacity of stud connector in steel concrete composite structures under push-out failure[J]. Advanced Engineering Sciences, 2019, 51(1): 89-95.
16	Luis P, Jerome F. Headed steel stud anchors in composite structures, part I: shear[J]. Journal of Constructional Steel Research, 2010, 66(2): 198-212.
17	EN 1994-1-1 :2004. Eurocode4: design of composite structures, part 1-1: general rules and rules for buildings [S].
18	Han Q H, Wang Y H, Xu J, et al. Static behavior of stud shear connectors in elastic concrete-steel composite beams[J]. Journal of Constructional Steel Research, 2015, 113: 115-126.
19	Lin Z F, Liu Y Q, He J. Behavior of stud connectors under combined shear and tension loads[J]. Engineering Structures, 2014, 81: 362-376.
20	Xue D Y, Liu Y Q, Yu Z, et al. Static behavior of multi-stud shear connectors for steel-concrete composite bridge[J]. Steel Construction, 2012, 74(8): 1-7.
21	苏庆田, 韩旭, 任飞. 多排焊钉推出试验试件力学性能[J]. 同济大学学报: 自然科学版, 2014, 42(7): 1011-1016.
	Su Qing-tian, Han Xu, Ren Fei. Static behavior of push-out specimen with multi-row stud connectors[J]. Journal of Tongji University (Natural Science Edition), 2014, 42(7): 1011-1016.
22	Katsuhiko T, Katsuyuki N, Sumio H. Shear strength of headed stud shear connector subjected to tensile load[J]. Journal of Constructional Steel, 1999, 7: 233-240.
23	Bode H, Hanenkamp W. Capacity of headed studs under tension load[J]. Bauingenieur, 1985, 60: 361-367.
24	安然, 王有志, 周磊, 等. 剪力钉连接件拉剪复合作用试验及计算模型[J]. 长安大学学报: 自然科学版, 2020, 40(3): 42-52.
	An Ran, Wang You-zhi, Zhou Lei, et al. Experiment and mechanical model of sheer stud connectors under combined tension and shear force[J]. Journal of chang'an University (Natural Science Edition), 2020, 40(3): 42-52.

Metrics

Viewed

Full text

Abstract

Cited

Shared

Discussed

试件编号	混凝土抗压强度f_c/MPa	剪力钉规格d×h	配筋	λ
S_1-1，S_1-2	66.34	16 mm×90 mm	?12@ 110	0
PS_1-1，PS_1-2	63.02			0.4
PS_2-1，PS_2-2	58.93			0.5
PS_3-1，PS_3-2	60.44			0.6
P₁	62.07			—

试件	T/kN	T_u/kN	λ（λ=T/T_u）	V/kN	V_u/kN	V/V_u	s_u/mm	k_e/（kN·mm^-1）	V_e/V
S_1-1	—	— — — — — — — — —	0.00	199.8	205.0 205.0 205.0 205.0 205.0 205.0 205.0 205.0 205.0	1.00	4.982	557.8	0.54
S_1-2	—		0.00	210.0		1.00	4.122	565.5	0.56
PS_1-1	33		0.40	176.2		0.86	4.686	299.5	0.34
PS_1-2	33		0.40	168.4		0.82	6.015	290.2	0.35
PS_2-1	41		0.50	162.3		0.79	5.672	210.6	0.26
PS_2-2	41		0.50	154.7		0.75	4.714	232.0	0.30
PS_3-1	50		0.60	145.3		0.70	3.948	167.1	0.22
PS_3-2	50		0.60	140.4		0.68	5.312	182.5	0.26
P₁	—	82.3	—	—	—	—	—	—	—

混凝土强度/MPa	λ
混凝土强度/MPa	0.2	0.3	0.4	0.5	0.6
30	0.95	0.94	0.93	0.92	0.90
40	0.96	0.95	0.94	0.93	0.91
50	0.98	0.97	0.95	0.94	0.93
60	1.00	1.00	1.00	0.99	0.98

数据来源	试件/有限元数量	式（6）		式（7）		式（8）		式（9）		式（10）
数据来源	试件/有限元数量	μ	η	μ	η	μ	η	μ	η	μ	η
文献［10］	1	0.909	0.000	0.848	0.000	1.376	0.000	0.938	0.000	1.050	0.000
文献［11］	12	0.917	0.032	0.862	0.074	1.274	0.274	0.941	0.014	0.983	0.021
文献［13］	32	0.840	0.054	0.740	0.054	1.608	0.054	0.887	0.054	1.021	0.051
本文	30	1.007	0.046	0.926	0.028	1.620	0.107	1.044	0.009	1.101	0.022

[1]	左新黛,张劲泉,赵尚传. 在役混凝土T梁疲劳刚度退化及寿命预测方法[J]. 吉林大学学报(工学版), 2023, 53(9): 2563-2572.
[2]	顾正伟,张攀,吕东冶,吴春利,杨忠,谭国金,黄晓明. 基于数值仿真的简支梁桥震致残余位移分析[J]. 吉林大学学报(工学版), 2023, 53(6): 1711-1718.
[3]	吴春利,黄诗茗,李魁,顾正伟,黄晓明,张炳涛,杨润超. 基于数值仿真和统计分析的洪水作用下桥墩作用效应分析[J]. 吉林大学学报(工学版), 2023, 53(6): 1612-1620.
[4]	谭国金,孔庆雯,何昕,张攀,杨润超,朝阳军,杨忠. 基于动力特性和改进粒子群优化算法的桥梁冲刷深度识别[J]. 吉林大学学报(工学版), 2023, 53(6): 1592-1600.
[5]	江辉,李新,白晓宇. 桥梁抗震结构体系发展述评：从延性到韧性[J]. 吉林大学学报(工学版), 2023, 53(6): 1550-1565.
[6]	王华,王龙林,张子墨,何昕. 基于裂缝宽度变化的连续刚构桥安全性预警技术[J]. 吉林大学学报(工学版), 2023, 53(6): 1650-1657.
[7]	冯宇,郝键铭,王峰,张久鹏,黄晓明. 非平稳极端风作用下大跨桥梁瞬态风致效应分析[J]. 吉林大学学报(工学版), 2023, 53(6): 1638-1649.
[8]	卜建清,郭至博,张吉仁,荀敬川,黄晓明. 多损伤钢-混组合梁桥力学性能有限元分析方法[J]. 吉林大学学报(工学版), 2023, 53(6): 1621-1637.
[9]	王峰,刘双瑞,王佳盈,宋佳玲,王俊,张久鹏,黄晓明. 尺寸和形状效应对多孔结构风阻系数的影响[J]. 吉林大学学报(工学版), 2023, 53(6): 1677-1685.
[10]	王俊,李加武,王峰,张久鹏,黄晓明. 简化U形峡谷风速分布及其对悬索桥抖振响应的影响[J]. 吉林大学学报(工学版), 2023, 53(6): 1658-1668.
[11]	袁野. 温度和车辆作用下梁式桥梁结构固有频率分析方法[J]. 吉林大学学报(工学版), 2023, 53(6): 1702-1710.
[12]	刘子玉,陈士通,支墨墨,黄晓明,陈哲心. 可“临-永”转换抢修钢墩应急使用极限承载力[J]. 吉林大学学报(工学版), 2023, 53(6): 1601-1611.
[13]	张玥,刘传森,宋飞. 桥台背墙对连续梁桥地震易损性的影响[J]. 吉林大学学报(工学版), 2023, 53(5): 1372-1380.
[14]	兰树伟,周东华,陈旭,莫南明. 双柱式高墩桥梁整体稳定性的实用算法[J]. 吉林大学学报(工学版), 2023, 53(4): 1105-1111.
[15]	匡亚川,陈立斌,李超举,贺宇豪. 栓钉剪力连接件力学性能分析[J]. 吉林大学学报(工学版), 2023, 53(2): 538-546.